Managerial Economics 1.1: Derivative Rules

SebastianWaiEcon
17 Aug 202006:16
EducationalLearning
32 Likes 10 Comments

TLDRIn this introductory video on Managerial Economics, Sebastian Y outlines the fundamental mathematical techniques, specifically focusing on the rules of derivatives. He introduces the notation for derivatives, distinguishing between Leibniz and Lagrange notations. The video covers five basic rules: (1) the derivative of a constant is zero, (2) constants can be factored out of derivatives, (3) the power rule for differentiating x to any power, (4) the sum rule for differentiating the sum of functions, and (5) the product rule for differentiating the product of two functions. The emphasis is on understanding these rules, especially the power rule and the sum rule, as they are most frequently used in calculating derivatives of polynomial functions. The video concludes by encouraging viewers to seek clarification if they are not comfortable with any of the rules, and hints at the application of derivatives in optimization in the next video.

Takeaways
  • 📚 Derivatives are mathematical tools used to analyze the rate of change of a function with respect to its variable.
  • 📝 Two common notations for derivatives are Leibniz (df/dx) and Lagrange (f'(x)). Both represent the rate of change of function f with respect to x.
  • 🔢 The derivative of a constant (da/dx) is always zero because a constant does not change.
  • 🔑 Constants can be factored out of derivatives, simplifying the expression to 'a times the derivative of f(x)'.
  • ⚙️ The power rule states that the derivative of x to the power of a is 'a times x to the power of (a - 1)', applicable to any exponent.
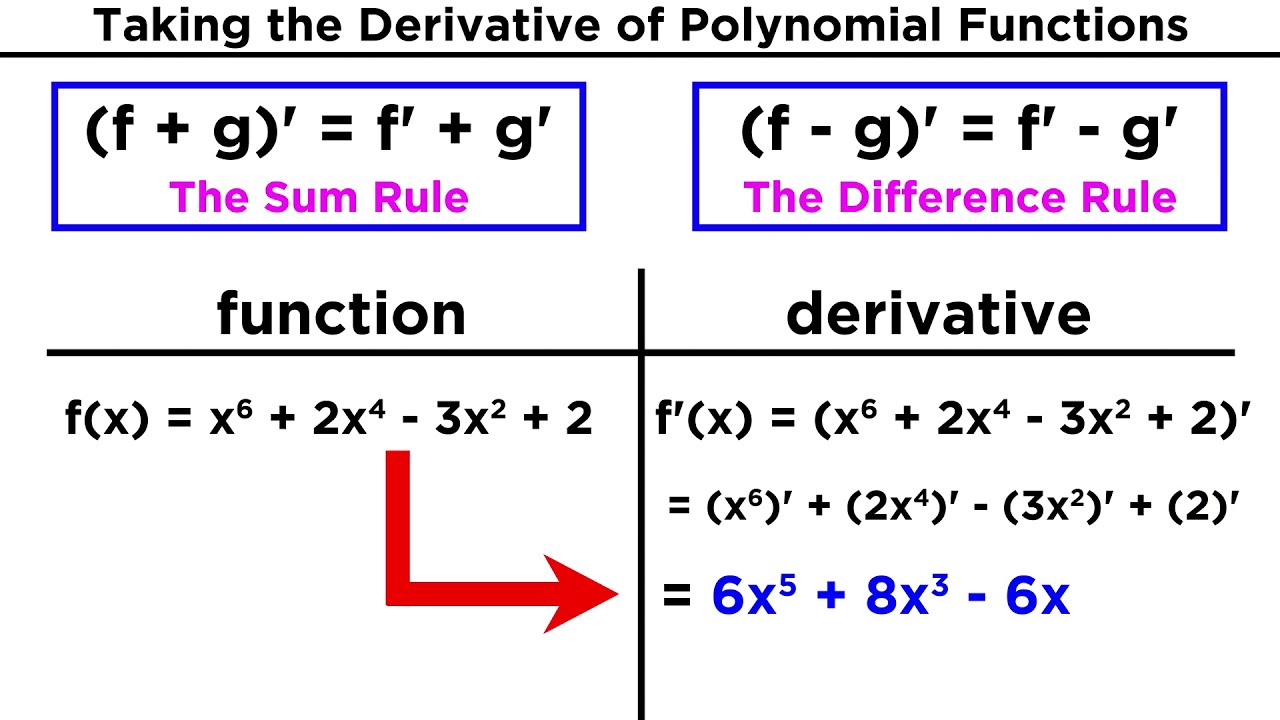
  • 📈 The sum rule allows us to find the derivative of a sum of functions by taking the derivative of each function separately and then adding the results.
  • 📌 The product rule is used for finding the derivative of a product of two functions, combining the derivative of one times the other function, and vice versa.
  • 🧮 Derivatives are essential for calculating the rate of change in managerial economics, which is crucial for optimization problems.
  • 📐 The power rule is the most frequently used for calculating derivatives of polynomial functions.
  • 🤔 It's important to be comfortable with these rules, especially the power rule and sum rule, as they are fundamental for more complex calculations.
  • ❓ Encouragement is given for learners to ask questions if they are not comfortable with any of the rules, ensuring a solid understanding before moving on.
  • 🚀 The next step is to apply the knowledge of derivatives to solve optimization problems, which is a key application in the field of economics.
Q & A

  • What are the two notations used to denote the derivative of a function?

    -The two notations are Leibniz notation, represented as df/dx, and Lagrange notation, represented as f'(x).

  • Why is the derivative of a constant always zero?

    -The derivative of a constant is zero because a constant does not change as x changes, so the rate of change is 0.

  • How can you factor out constants when taking the derivative of a function?

    -You can factor out constants by taking the constant out of the derivative notation, as in a times the derivative of f(x), written as a * (df/dx).

  • What is the power rule for derivatives?

    -The power rule states that the derivative of x to the power of a is equal to a times x to the power of (a - 1), written as d(x^a)/dx = a * x^(a - 1).

  • How does the sum rule for derivatives apply when finding the derivative of a sum of functions?

    -The sum rule allows you to find the derivative of the sum of two functions by taking the derivative of each function separately and then adding the results, written as d(f(x) + g(x))/dx = df/dx + dg/dx.

  • What is the product rule for derivatives and how is it applied?

    -The product rule states that the derivative of the product of two functions is the first function times the derivative of the second plus the second function times the derivative of the first, written as d(f(x) * g(x))/dx = f(x) * (dg/dx) + g(x) * (df/dx).

  • Can the power rule be applied to negative or fractional exponents?

    -Yes, the power rule applies to any exponent, including negative and fractional exponents.

  • What is the derivative of x to the fourth power using the power rule?

    -The derivative of x to the fourth power is four times x to the third power, written as d(x^4)/dx = 4 * x^3.

  • How do you find the derivative of a polynomial function?

    -You find the derivative of a polynomial by separating it into its individual terms, applying the power rule to each term, and then summing the results.

  • In the context of the video, which two rules are said to be used most frequently?

    -The power rule (rule number three) and the sum rule (rule number four) are said to be used most frequently.

  • What is the next topic that will be covered in the series of videos?

    -The next topic to be covered is the application of derivatives to optimization problems.

  • What should one do if they are not feeling comfortable with any of the derivative rules presented in the video?

    -If one is not comfortable with the rules, they should feel free to ask questions, likely in the comments section or related discussion platform for the video.

Outlines
00:00
📚 Introduction to Derivatives in Managerial Economics

Sebastian Y introduces the topic of managerial economics and outlines the mathematical techniques that will be covered in the course. The focus of the first video is on the basic rules for derivatives. Notation is explained, with 'x' as the choice variable and 'f(x)' as a generic function. Two notations for derivatives are presented: Leibniz (df/dx) and Lagrange (f'(x)), with a preference for Leibniz notation. Four key rules for derivatives are discussed: 1) the derivative of a constant is zero, 2) constants can be factored out, 3) the power rule for derivatives of x to any power, and 4) the sum rule for derivatives of functions. An example is given to illustrate the application of these rules, specifically the power rule and the sum rule in calculating the derivative of a polynomial function.

05:01
🔢 Applying Derivatives: The Product Rule and Polynomial Functions

The second paragraph delves into the fifth rule, known as the product rule, which is used for finding the derivative of a product of two functions, f(x) and g(x). An example calculation is provided for the derivative of 2x times 3x squared, demonstrating the use of the product rule. The paragraph emphasizes the importance of comfort with the power rule and the sum rule, as they are the most frequently used in calculating derivatives, particularly for polynomial functions. The speaker encourages questions if there's any discomfort with the rules and previews that the next video will apply derivative knowledge to optimization problems.

Mindmap
Keywords
💡Derivatives
Derivatives in the context of the video refer to the mathematical concept that describes the rate at which a function changes with respect to its variable. It is a fundamental concept in calculus and is used to analyze the behavior of economic functions. In the video, derivatives are used to review mathematical techniques essential for understanding managerial economics.
💡Leibniz Notation
Leibniz notation is a way to represent the derivative of a function, denoted as df/dx, which signifies the derivative of the function f with respect to x. It is one of the two notations for derivatives discussed in the video and is used to illustrate the process of differentiating functions.
💡Lagrange Notation
Lagrange notation, also known as the prime notation, is another method to denote derivatives, represented as f'(x). It is mentioned in the video as an alternative to Leibniz notation and is used when it is more convenient for the context of the problem being solved.
💡Constant
In the context of derivatives, a constant is a value that does not change. The video explains that the derivative of a constant is zero, which is a basic rule of calculus. This is because a constant has no variability with respect to the variable x, hence its rate of change is non-existent.
💡Factoring Constants
Factoring constants is a technique used in calculus where a constant factor can be taken out of a derivative expression. The video demonstrates this by showing that the derivative of a constant times a function can be simplified by factoring the constant out, making the calculation more straightforward.
💡Power Rule
The power rule is a fundamental rule in calculus for finding the derivative of a variable raised to a power. As explained in the video, the rule states that to find the derivative of x^a, you multiply by the exponent (a) and subtract 1 from the exponent. This rule applies regardless of whether the exponent is positive, negative, or fractional.
💡Sum of Derivatives
The sum of derivatives rule, as discussed in the video, allows for the derivative of a sum of functions to be broken down into the sum of their individual derivatives. This means that for a function f(x) + g(x), the derivative is the derivative of f(x) plus the derivative of g(x), simplifying the process of finding the derivative of complex expressions.
💡Product Rule
The product rule is a specific formula in calculus for differentiating the product of two functions. The video explains that the derivative of f(x) times g(x) is found by multiplying f(x) by the derivative of g(x) and adding it to g(x) times the derivative of f(x). This rule is essential for differentiating more complex functions that are products of simpler ones.
💡Polynomial Function
A polynomial function is a function involving a finite number of terms, each term consisting of a constant multiplied by a power of the variable. The video emphasizes that polynomial functions are the most common type of functions for which derivatives are calculated. The process involves applying the power rule to each term of the polynomial.
💡Optimization
While not explicitly defined in the transcript, optimization is a process that involves finding the best solution or maximum/minimum value within a set of possible solutions. The video hints at applying knowledge of derivatives to perform optimization, which is a significant application of derivatives in managerial economics.
💡Managerial Economics
Managerial economics is the application of economic theory and quantitative methods to solve management problems. The video is a review of mathematical techniques, specifically derivatives, which are crucial for understanding and applying economic principles in a managerial context.
Highlights

Introduction to the mathematical techniques required for Managerial Economics.

Explanation of notation: 'x' as the choice variable and two ways to denote derivatives - Leibniz and Lagrange notations.

Derivative of a constant is zero, as constants do not change.

Constants can be factored out of derivatives, simplifying the calculation process.

The power rule for derivatives: multiply by the exponent and subtract one, applicable to any exponent including negative and fractional.

Derivative of a sum of functions is the sum of the derivatives of the individual functions.

Product rule for derivatives of the product of two functions, involving both functions' derivatives.

Example calculation: derivative of 3x^2 using the power rule.

Example calculation: derivative of 3x^3 + 4x^2, applying both power rule and sum rule.

Example calculation: derivative of 2x * 3x^2, demonstrating the product rule.

Emphasis on the importance of being comfortable with the power rule and sum rule for polynomial functions.

The most common application of derivatives in the course will be calculating derivatives of polynomial functions.

Encouragement for students to ask questions if they are not comfortable with any of the derivative rules.

Upcoming application of derivatives to optimization problems in the next video.

Derivatives provide the rate of change of a function as the variable changes.

Leibniz notation is preferred, but Lagrange notation may be used when convenient.

The derivative of a function f(x) with respect to x is represented as df/dx or f'(x).

The importance of understanding the basic rules of derivatives for the course.

The transcript serves as a review and introduction to the mathematical foundation of Managerial Economics.

Transcripts

Browse More Related Video

Derivatives of Polynomial Functions: Power Rule, Product Rule, and Quotient Rule

Techniques for Finding Derivatives

Calculus - The basic rules for derivatives

Math 1325 Lecture 9 4 - Derivative Formulas

BusCalc 08 Derivative Basics

Basic Derivative Rules - Part 2: Combining the Rules

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesManagerial EconomicsMathematical TechniquesLeibniz NotationLagrange NotationPower RuleProduct RuleConstant FactorRate of ChangeOptimizationPolynomial FunctionsEducational Content