10. Ampere's Law
TLDRThe provided script is a detailed lecture on electromagnetism, focusing on Ampere's Law and its applications, the concept of magnetic flux, and Faraday's Law of electromagnetic induction. The professor begins by reviewing Ampere's Law, which relates the integral of the magnetic field around a closed loop to the currents passing through the loop. The lecture then delves into the nuances of calculating magnetic fields using symmetry and the implications of a changing magnetic field on a stationary loop, highlighting the paradox of a magnetic field doing work despite the common understanding that it does not. The script progresses to Faraday's Law, which introduces the idea that a changing magnetic field induces an electromotive force (emf), leading to the generation of an electric field. The professor uses the concept of magnetic flux to explain how this induced emf can drive a current opposing the change in magnetic flux, a phenomenon known as Lenz's Law. The lecture concludes with a discussion on the implications of these laws for the understanding of electromagnetic fields and their interaction, emphasizing that the derived equations are not only applicable to conductors but are intrinsic properties of electric and magnetic fields themselves.
Takeaways
- 🧲 Ampere's Law states that the integral of the magnetic field around a closed loop is equal to μ₀ times the total current passing through the loop.
- 🔍 The direction of the current is important in Ampere's Law, with currents entering the loop considered negative and those leaving considered positive.
- 📐 In three dimensions, the surface bounded by a loop is not unique, but Ampere's Law holds true for any surface that shares the same loop as its boundary.
- 💡 The magnetic field due to a long straight wire is found using Ampere's Law and symmetry considerations, resulting in a field that is perpendicular to the wire and varies with the distance from it.
- 🌀 For an infinite solenoid, the magnetic field is constant inside the solenoid and zero outside, due to the continuous nature of the magnetic field lines.
- 🔌 The magnetic field inside a finite solenoid can be found using Ampere's Law, considering the number of turns per unit length (n) and the current (I), resulting in a formula B = μ₀nI.
- 🍩 A toroidal solenoid, or a solenoid in the shape of a doughnut, has a magnetic field that varies depending on the radius, with the field being μ₀NI/2πr, where N is the total number of turns and r is the radius.
- ⚡ The work done by the magnetic force v × B on a moving wire loop results in an electromotive force (emf), which can power a light bulb, despite the magnetic field not doing work in the traditional sense.
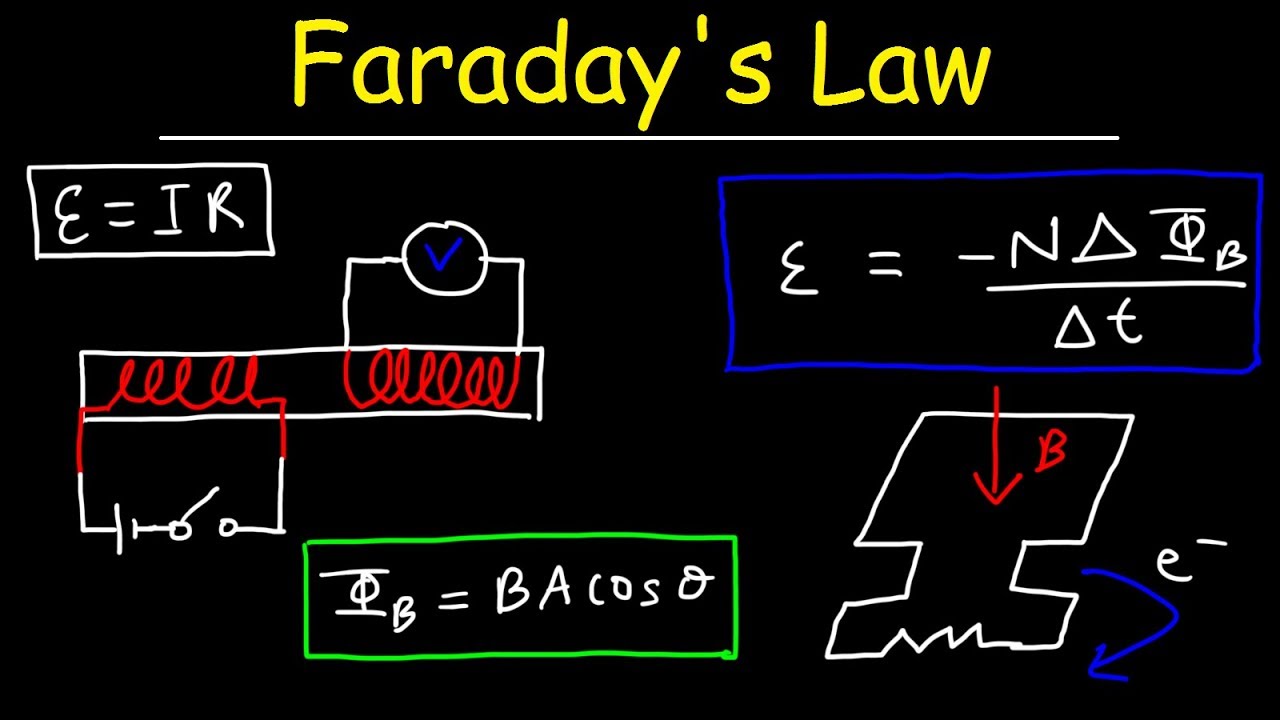
- 🔄 Faraday's Law of Electromagnetic Induction states that the electromotive force induced in a closed loop is equal to the negative rate of change of the magnetic flux through the loop, emf = -dΦ/dt.
- ⚖️ Lenz's Law, associated with the negative sign in Faraday's Law, states that the induced emf and resulting current will always act to oppose the change in magnetic flux that created it.
- 🔧 The concept of electromagnetic induction is fundamental to understanding how electric generators work, converting mechanical energy into electrical energy through the movement of a loop in a magnetic field.
Q & A
What is Ampere's law and how does it relate to the integration of the magnetic field around a closed loop?
-Ampere's law states that the integral of the magnetic field around a closed loop is equal to μ₀ times the sum of all currents that penetrate the loop. It means that only the currents passing through the loop (not those outside of it) contribute to the magnetic field's integral. The law is crucial for calculating magnetic fields created by currents.
How does the convention of line integral and the direction of current affect the calculation of magnetic fields using Ampere's law?
-The convention for the line integral in Ampere's law is to perform it counterclockwise. If you follow the line integral with your fingers, your thumb points in the direction of the positive current (outside the board), and any current going into the board is considered negative. This convention is essential for correctly calculating the magnetic field's contribution from different currents.
What is the significance of the surface bounded by a loop in Ampere's law, and how does it relate to the concept of magnetic field lines?
-The surface bounded by a loop in Ampere's law is significant because it defines the area through which currents contribute to the magnetic field. The theorem holds true even if the surface is non-planar or bulging. Magnetic field lines, like currents, do not terminate and will cross any surface that has the same boundary loop, making the choice of surface for the calculation irrelevant.
How can Ampere's law be used to solve problems involving highly symmetric configurations, such as a solenoid?
-Ampere's law can be used to solve problems with high symmetry by taking advantage of the symmetry to simplify the magnetic field's configuration. For example, in the case of a solenoid, the symmetry of the coil allows us to deduce that the magnetic field inside the solenoid is constant and parallel to the axis of the solenoid, which simplifies the calculation of the magnetic field.
What is the relationship between the magnetic field inside a solenoid and the number of turns per unit length of the wire?
-The magnetic field inside a solenoid is directly proportional to the number of turns per unit length of the wire (n) and the current (I) flowing through the wire. The relationship is given by the formula B = μ₀ * n * I, where B is the magnetic field, μ₀ is the permeability of free space, and n is the number of turns per unit length.
How does the magnetic field inside a toroidal solenoid (doughnut-shaped) vary with the radius of the loop?
-The magnetic field inside a toroidal solenoid varies inversely with the radius of the loop. The formula for the magnetic field inside the toroid is B = μ₀ * N * I / (2πr), where N is the total number of turns, I is the current, and r is the radius of the loop. As the radius increases, the magnetic field decreases.
What is the role of the magnetic field in a circuit when a loop of wire is moved through a magnetic field?
-When a loop of wire is moved through a magnetic field, the magnetic field induces an electromotive force (emf) in the loop, which can cause a current to flow if the loop is part of a closed circuit. This induced emf is the work done per unit charge around the loop, which can power a device like a light bulb.
How does the concept of relativity apply to the scenario where a loop of wire is moved through a magnetic field?
-According to the principle of relativity, the physical laws should be the same in all inertial frames of reference. In the scenario where a loop is moved through a magnetic field, an observer moving with the loop would still observe the light bulb glowing, even though from their perspective, it's the magnetic field that is moving. This implies that there must be an electric field generated by the changing magnetic field, even in the frame of reference where the loop is stationary.
What is the physical interpretation of the negative sign in Faraday's law of electromagnetic induction?
-The negative sign in Faraday's law, often associated with Lenz's law, indicates that the induced electromotive force (emf) and the resulting current will act in a direction to oppose the change in magnetic flux that produced it. This means that the induced effects work to maintain the status quo of the magnetic flux through the loop.
How does the changing magnetic field produce an electric field, and what is the mathematical relationship between them?
-A changing magnetic field induces an electric field, as described by Faraday's law of electromagnetic induction. The mathematical relationship is given by the equation emf = -dΦ/dt, where Φ is the magnetic flux through a surface bounded by a loop, and emf is the electromotive force around the loop. The negative sign indicates the direction of the induced emf opposes the change in magnetic flux.
What is the significance of Faraday's law in the context of electromagnetic fields and how does it modify the Maxwell's equations for time-varying fields?
-Faraday's law signifies that a time-varying magnetic field can induce an electric field, even in the absence of charges. This is a departure from the static case where the line integral of the electric field around a closed loop is zero. In the context of Maxwell's equations, Faraday's law introduces a term that accounts for the time derivative of the magnetic field, indicating that the electric field is not conservative when magnetic flux is changing.
Outlines
🧲 Introduction to Ampere's Law
The professor begins by revisiting Ampere's law, which relates the integrated magnetic field around a closed loop to the currents passing through the loop. The law is crucial for understanding magnetostatics. The convention for the line integral is counterclockwise, with a positive current being outside the loop and a negative current inside. The law accounts for the algebraic sum of currents, not the absolute value. The subtlety of the current penetrating a surface bounded by the loop is also discussed, emphasizing that the surface's shape does not affect the outcome, as long as it has the same boundary loop.
🔍 Symmetry and Ampere's Law
The professor illustrates how symmetry can simplify problems involving Ampere's law, such as finding the magnetic field around a long straight wire. By considering the field's symmetry, one can deduce the field's configuration and use Ampere's law to calculate the magnetic field's magnitude. The example of a thick wire with finite thickness is also discussed, showing how the magnetic field varies within and outside the wire.
🌀 Magnetic Field of a Solenoid
The focus shifts to the solenoid, a coil of wire that generates a magnetic field. The professor explains how to use Ampere's law to find the magnetic field inside and outside the solenoid. By considering an Amperian loop within the solenoid, it is argued that the magnetic field is constant inside and zero outside for an ideal solenoid. The right-hand rule is used to determine the direction of the magnetic field.
🔗 Current Through a Solenoid
The professor clarifies a common mistake regarding the current through a solenoid. The current is not simply the current in the wire; instead, it is the product of the number of turns per unit length (n) and the length of the loop (l). This results in the formula for the magnetic field inside a solenoid being μ₀ times n times the current (I). The concept of an ideal solenoid, where the magnetic field lines are perpendicular and do not intersect, is also discussed.
🍩 Magnetic Field of a Toroid
The toroid, a solenoid wound in the shape of a doughnut, is introduced. The professor explains that the magnetic field inside the toroid is weaker towards the outer rim and stronger towards the inner rim, with the field being zero outside the toroid. The magnetic field's formula inside the toroid is derived, showing its dependence on the radius and the number of turns in the solenoid.
📐 Maxwell's Equations for Static Fields
The professor summarizes electrostatics and magnetostatics with Maxwell's equations, which describe the relationship between electric and magnetic fields and their sources (charges and currents). The force experienced by a charged particle in an electromagnetic field is also discussed, emphasizing the importance of understanding these fields as they are responsible for the motion of charged particles.
🏃♂️ Moving a Wire Loop in a Magnetic Field
The scenario of a wire loop moving through a magnetic field is explored, leading to the light bulb glowing due to the induced electromotive force (emf). The professor explains that the work done by the magnetic field on the moving charges within the loop results in an emf, which can be calculated using the force exerted by the magnetic field on the moving charges.
💡 The Role of Magnetic Fields in Energy Transfer
The professor discusses the apparent paradox of magnetic fields doing work despite the established principle that they do not. The resolution lies in understanding that while the magnetic field does not do work in the sense of moving charges along the direction of the magnetic field, it does facilitate the transfer of energy from macroscopic mechanical power to microscopic energy in the electrons. This is illustrated through the example of a generator, where the work done by pulling the loop results in the light bulb glowing.
🧐 The Paradox of a Glowing Light Bulb
The professor presents a thought experiment involving a light bulb glowing due to a magnetic field. The paradox arises when considering the situation from the frame of reference of the loop, where the loop is stationary, and the magnetic field is moving. The solution to this paradox involves the concept of a changing magnetic field inducing an electric field, which can drive a current around the loop, even in the stationary frame of reference.
🌀 Induced Electric Field by a Changing Magnetic Field
The professor explains that a changing magnetic field induces an electric field, which can be understood through Faraday's law of electromagnetic induction. This law states that the electromotive force (emf) around a closed loop is equal to the negative rate of change of the magnetic flux through that loop. The induced electric field is not conservative and can drive a current around the loop, even in the absence of a conducting wire.
🔄 Lenz's Law and the Direction of Induced Current
Lenz's law is introduced, which describes the direction of the induced current in response to a changing magnetic flux. The induced current will flow in a direction that opposes the change in magnetic flux. This is demonstrated through examples, showing how the induced current can either increase or decrease the flux, depending on the situation. Lenz's law is a fundamental principle that helps determine the direction of induced currents in electromagnetic induction scenarios.
📉 The Rate of Change of Flux and emf
The professor delves into the mathematical relationship between the rate of change of magnetic flux and the induced electromotive force (emf). By considering the flux through a surface that changes over time due to the motion of a loop and the change in the magnetic field, the professor derives an expression for the emf that accounts for both the changing magnetic field and the motion of the loop. This leads to a deeper understanding of Faraday's law of electromagnetic induction.
🔗 Faraday's Law and the Intrinsic Relation Between E and B
The professor concludes with Faraday's law, which establishes an intrinsic relationship between electric and magnetic fields. The law states that the line integral of the electric field around a closed loop is equal to the negative rate of change of the magnetic flux through that loop. This law reveals that a changing magnetic field can produce a circulating electric field, even in the absence of a conducting loop, and is a fundamental principle of electromagnetism.
Mindmap
Keywords
💡Ampere's Law
💡Magnetic Field
💡Line Integral
💡Current Density
💡Surface Integral
💡Electromotive Force (EMF)
💡Magnetic Flux
💡Faraday's Law of Electromagnetic Induction
💡Lenz's Law
💡Cross Product
💡Relativity
Highlights
Ampere's law is fundamental for understanding the relationship between magnetic fields and currents, stating that the integral of the magnetic field around a closed loop equals μ₀ times the total current passing through the loop.
The convention for calculating the line integral in Ampere's law involves a counterclockwise direction, with positive current being outside the loop and negative current entering the loop.
Ampere's law applies to any surface bounded by a given loop, even in three-dimensional scenarios, emphasizing the law's versatility.
The algebraic sum of currents is considered in Ampere's law, not the absolute value, which is crucial for understanding the magnetic field's contribution.
Gauss's law and Ampere's law are both useful for solving problems with high symmetry, such as the electric field of a spherical charge distribution.
The magnetic field around a current-carrying wire can be determined using Ampere's law, with the field configuration being symmetrical and dependent on the distance from the wire.
For a finite solenoid, the magnetic field grows linearly inside and falls off like 1 over r outside, which differs from the field of an idealized point wire.
The magnetic field inside an infinite solenoid is constant and can be calculated using Ampere's law, with the field outside being zero.
The magnetic field inside a toroidal solenoid (doughnut-shaped) varies depending on the radius, with the field being stronger at the inner rim and weaker at the outer rim.
Maxwell's equations, as presented, are valid for static cases where currents and charge densities are constant.
The force exerted by a magnetic field on a moving charge is given by the cross product v x B, which is responsible for the induced emf in a loop of wire moving through a magnetic field.
The work done by the magnetic field in a moving loop is not a violation of the principle that magnetic fields do no work, as the magnetic field facilitates the transfer of energy from macroscopic to microscopic scales.
Faraday's law of electromagnetic induction states that a changing magnetic flux through a loop induces an electromotive force (emf), which can drive a current in the loop.
Lenz's law, associated with the negative sign in Faraday's law, indicates that the induced emf and current work to oppose the change in magnetic flux, not the magnetic field itself.
The direction of the induced current can be determined by understanding that it will flow in a way that opposes the change in magnetic flux, as per Lenz's law.
Faraday's law reveals an intrinsic relationship between electric and magnetic fields, showing that a changing magnetic field can produce a circulating electric field, even in the absence of a physical loop.
The line integral of the electric field around a closed loop is equal to the negative rate of change of the magnetic flux through any surface bounded by that loop, highlighting the interplay between E and B fields.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: