2022 AP Calculus AB Free Response #5
TLDRThe video script discusses the process of solving a differential equation using various mathematical techniques. The presenter begins by sketching the slope field for the given differential equation and then illustrates a particular solution curve through a specific point. They derive the equation of the tangent line to the solution curve at a given point (x=1, y=2) and use it to approximate the value of y when x=0.8. The presenter explains that if the second derivative is positive, indicating the curve is concave up, the tangent line approximation will be an underestimate. They then employ separation of variables to find a particular solution to the differential equation, integrating both sides and applying initial conditions to solve for the constant 'c'. The final solution is presented, and the importance of considering the positive square root for the solution is emphasized to avoid extraneous solutions.
Takeaways
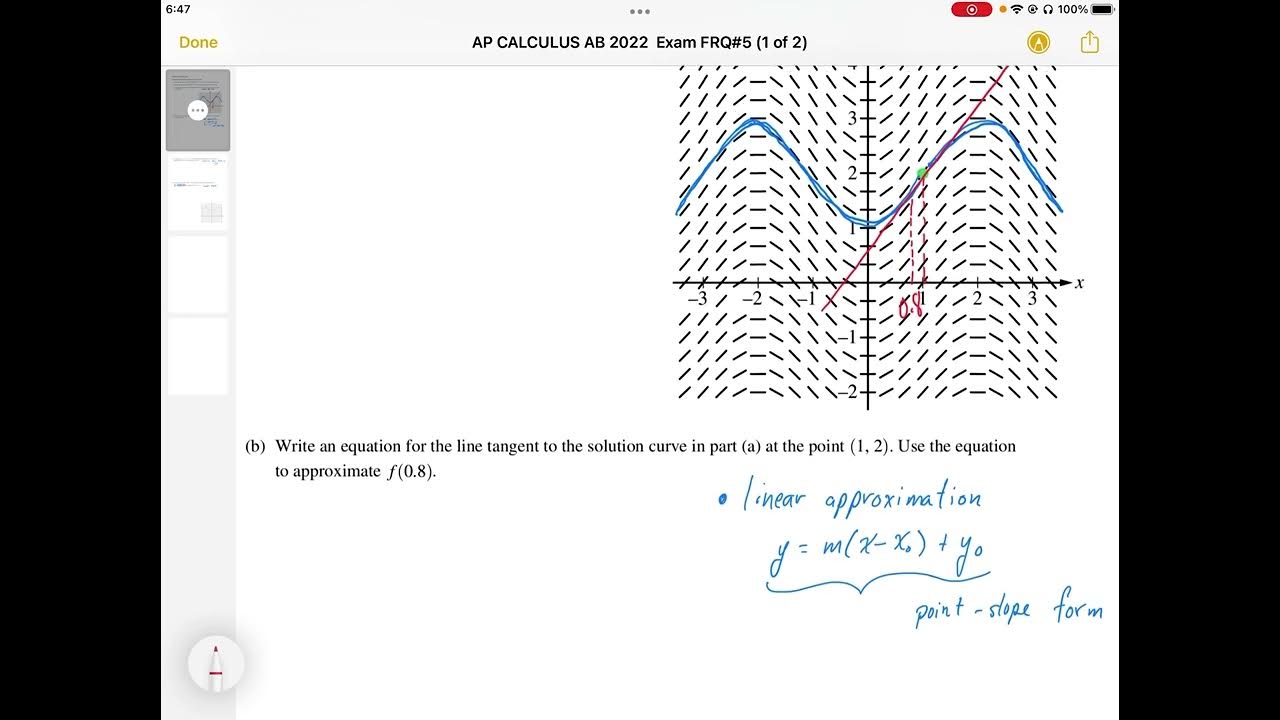
- 📐 Sketching the solution curve for a differential equation involves connecting the dots to represent the slope field and does not require exact precision.
- 📝 The tangent line equation can be formulated using the slope at a specific point, which is derived from the differential equation itself.
- 🔍 The derivative needed for the tangent line equation is calculated using the function's value at a specific point, involving trigonometric and algebraic manipulation.
- 🧮 For the tangent line approximation, the value of the function at a given x can be estimated using the derived tangent line equation.
- 📈 Positive second derivatives indicate a concave up curve, and when using a tangent line to approximate the function value, this setup typically results in an underestimate.
- 🔢 Separation of variables is used to find the particular solution to the differential equation, involving moving differentials to opposite sides and integrating.
- ✏️ Integration of both sides involves specific techniques like U-substitution or power rule, and sometimes adjustments for integration constants.
- 🔑 The integration result is manipulated algebraically to express the function in terms of y, and constants are adjusted accordingly.
- 🌐 The initial condition is used to solve for the integration constant, demonstrating how boundary conditions help pinpoint specific solutions in differential equations.
- ✨ Correct identification of the constant from possible values is crucial, especially when redundant solutions appear from operations like squaring, highlighting the importance of maintaining the correct signs and values through calculations.
Q & A
What is the purpose of considering a particular solution to a differential equation?
-A particular solution to a differential equation is used to find a specific solution that satisfies the given differential equation, often in the context of an initial value problem or a boundary value problem.
How does one sketch the slope field for a differential equation?
-The slope field is sketched by plotting points with the slope given by the differential equation at various points in the domain. This helps visualize the behavior of solutions to the differential equation.
What is the process of finding the equation of the tangent line to a curve at a given point?
-The equation of the tangent line is found using the point-slope form, which is y - y0 = m(x - x0), where m is the slope (derivative) at the point (x0, y0).
What does the second derivative being greater than zero indicate about the curve?
-If the second derivative is greater than zero, it indicates that the curve is concave up, meaning the tangent line will always lie below the curve, leading to an underestimate of the actual curve's value at a given point.
How does one use separation of variables to solve a differential equation?
-Separation of variables involves rearranging the differential equation so that all terms involving one variable are on one side and the other variable on the other side, then integrating both sides with respect to their respective variables.
What is the significance of the initial condition in finding a particular solution to a differential equation?
-The initial condition provides a specific value that the solution must satisfy, which is used to determine any arbitrary constants in the general solution, thus finding the particular solution that meets the condition.
Why is it important to consider the sign of the square root when solving differential equations?
-The sign of the square root is important because it affects the domain of the solution. In many physical applications, the solution must be real and positive, hence the square root must also be positive.
What is the role of the constant 'c' in the general solution of a differential equation?
-The constant 'c' represents an arbitrary constant that arises from the integration process. It is typically determined by applying initial or boundary conditions to the general solution.
How does the direction of concavity affect the approximation of a function using a tangent line?
-The direction of concavity (whether the function is concave up or down) determines whether the tangent line will overestimate or underestimate the function's value at a given point. If the function is concave up, the tangent line will underestimate the value.
What is the process of using an integral to solve for the general solution of a differential equation?
-Integration is used to 'undo' the differentiation process in a differential equation. By integrating both sides of the equation with respect to the appropriate variable, one can find the general solution, which may include an arbitrary constant.
Why is it necessary to check for extraneous solutions when solving differential equations?
-Extraneous solutions can arise when operations such as squaring both sides of an equation are performed. These solutions do not satisfy the original differential equation and must be discarded to ensure the solution is valid.
What is the importance of the sign of the constant 'c' in the context of the given differential equation?
-The sign of the constant 'c' is crucial because it affects the behavior of the solution. In the given context, 'c' must be positive to ensure that the square root term remains real and positive, which is necessary for the solution to be physically meaningful.
Outlines
📐 Differential Equation Solution and Tangent Line Approximation
The video script begins with the presenter discussing a differential equation and introduces a particular solution, \( y(x) \). They proceed to sketch the solution curve and highlight the process of finding the equation of the tangent line at a given point, \( (1, 2) \). The presenter uses the slope of the tangent line, which is derived from the derivative of the function at that point, to approximate the value of \( y \) when \( x = 0.8 \). They then discuss the implications of the second derivative being positive, indicating that the curve is concave down, and therefore, the tangent line approximation will be an underestimate. The presenter concludes this section by using separation of variables to find the particular solution to the given differential equation, integrating both sides, and applying the initial condition \( f(1) = 2 \) to solve for the constant \( c \).
🔍 Squaring for Initial Condition and Positive Root Determination
The second paragraph delves into using the initial condition to find the value of the constant \( c \) in the solution to the differential equation. The presenter squares both sides of the equation, subtracts seven, and then uses the initial condition \( f(1) = 2 \) to solve for \( c \). They find that \( c \) must be positive 3 to satisfy the initial condition and the algebraic manipulations performed. The presenter emphasizes the importance of considering the positive square root due to the potential for extraneous solutions when squaring both sides of an equation. They also discuss the rationale behind choosing the positive value for \( c \), based on the algebraic properties and the context of the problem.
Mindmap
Keywords
💡Differential Equation
💡Particular Solution
💡Slope Field
💡Tangent Line
💡Second Derivative
💡Separation of Variables
💡Initial Condition
💡Concave Up/Down
💡Integration
💡Cosine Function
💡Arbitrary Constant
Highlights
The video discusses solving a differential equation using various methods like sketching the solution curve, tangent line approximation, and separation of variables.
The differential equation is dy/dx = (1/2)sin(pi/2x) * sqrt(y + 7).
A particular solution y(x) is considered to define a portion of the slope field for the given differential equation.
The solution curve is sketched through a given point, with the curve approximated by connecting the slope of the lines.
The equation for the tangent line to the solution curve at a specific point (x0, y0) is derived using the point-slope form.
The derivative dy/dx at the point (1, 2) is calculated to find the slope m for the tangent line equation.
The tangent line equation is simplified by factoring out terms and avoiding unnecessary distribution.
The tangent line approximation is used to estimate the value of y at x = 0.8, resulting in an approximate value of 1.7.
It is determined that the tangent line approximation is an underestimate since the second derivative is positive, indicating the curve is concave down.
Separation of variables is used to find the particular solution to the differential equation, by rearranging and integrating both sides.
The integral on the left side is solved using power rules, resulting in (2/sqrt(y + 7)) as the integrating factor.
The integral on the right side involves a u-substitution with u = sin(pi/2x), leading to a cosine term in the solution.
The arbitrary constant C is included in the final solution, with both sides divided by 2 for simplicity.
The initial condition y(1) = 2 is used to solve for the value of C, resulting in C = 3.
The possibility of extraneous solutions due to squaring is acknowledged, with the positive value of C being justified.
The final particular solution is expressed as y = -1/(2pi) * cos(pi/2x) + 3^2 - 7.
The importance of considering the positive square root in the solution is emphasized to avoid extraneous solutions.
Transcripts
Browse More Related Video
AP CALCULUS AB 2022 Exam Full Solution FRQ#5(a,b)

2022 AP Calculus AB Exam FRQ #5

AP Calculus AB Crash Course Day 5 - Integration and Differential Equations

2010 AP Calculus AB Free Response #6

2004 AP Calculus AB Free Response #6

Separable differential equations introduction | First order differential equations | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: