AP Calculus AB Crash Course Day 5 - Integration and Differential Equations
TLDRThe video script delves into various calculus problems, focusing on integrals and differential equations. It begins with a substitution method for integrating 1/(xlnx), leading to a natural logarithm solution. Next, it solves a differential equation dy/dx = x^2/y^4, using separation of variables to find y as a root function of x. The video then tackles the integral of 8/(1+x^2), simplifying it to an inverse tangent function plus a constant. Common inverse trigonometric integrals are also highlighted. A differential equation dy/dx = xy + 3y is solved using separation of variables, with an initial condition to find the particular solution. The script also evaluates a definite integral using the chain rule and fundamental theorem of calculus, applying it to a provided function table. Finally, it approximates a function's value using the tangent line at a specific point on a curve, derived from another differential equation. The video provides a comprehensive walkthrough of calculus techniques, offering both conceptual explanations and step-by-step solutions.
Takeaways
- 📌 Integral of 1/(x * ln(x)) can be solved by substitution, setting u = ln(x), which leads to du = (1/x) dx.
- 📘 The integral simplifies to ∫(1/u) du, which is ln(|u|) + C, and substituting back ln(x) for u gives ln(|ln(x)|) + C.
- 🔍 For the differential equation dy/dx = x^2/y^4 with y(3) = 0, separation of variables and integration yields y^(5/4) = (x^3/3) + C, and solving for C gives y = (5 * x^3/(3 - 9))^(1/5).
- 🧮 The integral of 8/(1 + x^2) dx is 8 * arctan(x) + C, where the constant 8 is factored out, and the integral of the remaining term is the inverse tangent of x.
- 📐 The integral of 1/√(1 - x^2) dx is given by the inverse sine function, sin^(-1)(x) + C.
- 🤔 For the differential equation dy/dx = xy + 3y, separation of variables and integration yields ln(|y|) = (x^2/2) + 3x + C, leading to y = ±e^(x^2/2 + 3x + C).
- 🔑 Using the initial condition y(0) = 5, we find the constant k = 5, and thus y = 5e^(x^2/2 + 3x).
- 📉 To evaluate the integral of g'(f(x)) * f'(x) dx from 0 to 3, the chain rule is applied, and the fundamental theorem of calculus is used with the given table to find the result as -3.
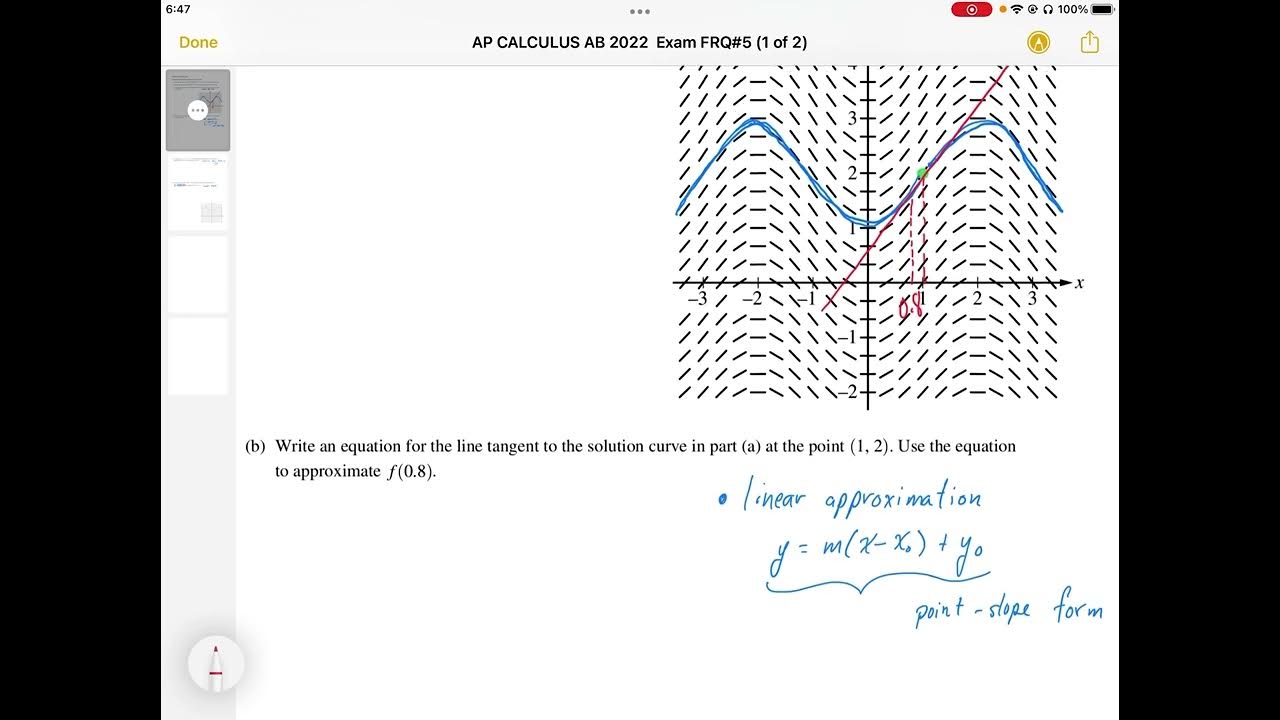
- 🧹 For the differential equation dy/dx = y + 1/x, the solution curve's tangent line at (-2, 0) has a slope of -1/2, leading to the equation y = -1/2x - 1.
- 🔢 Approximating f(-1.5) using the tangent line equation gives a value of approximately -1.25.
- 📐 The particular solution to the differential equation dy/dx = y + 1/x with the initial condition y(-2) = 0 is found by separation of variables, leading to y = -1/2x - 1.
Q & A
What is the integral of 1 over x times the natural logarithm of x with respect to x?
-The integral of 1/(x * ln(x)) dx is found by substitution, where u = ln(x), resulting in du = (1/x) dx. The integral then becomes ∫(1/u) du, which is ln(|u|) + C. Substituting back, we get ln(|ln(x)|) + C.
How do you solve the differential equation dy/dx = x^2/y^4 with the initial condition y(3) = 0?
-By separating variables, we get y^4 dy = x^2 dx. Integrating both sides gives y^5/5 = x^3/3 + C. Using the initial condition y(3) = 0, we find C = -9. Thus, the solution is y^5/5 = x^3/3 - 9.
What is the integral of 8/(1 + x^2) dx?
-Since 8 is a constant, we can pull it out of the integral. The integral of 1/(1 + x^2) dx is the inverse tangent of x, so the final result is 8 * arctan(x) + C.
What is the integral of 1/√(1 - x^2) dx?
-The integral of 1/√(1 - x^2) dx is the inverse sine of x, which is written as sin^(-1)(x) + C.
How do you find the particular solution to the differential equation dy/dx = xy + 3y given y(0) = 5?
-By separating variables, we get dy/y = (x + 3) dx. Integrating both sides gives ln(|y|) = (x^2/2) + 3x + C. Exponentiating both sides and using the initial condition y(0) = 5, we find k = 5. Thus, the solution is y = 5e^(x^2/2 + 3x).
How do you evaluate the integral from 0 to 3 of g'(f(x)) * f'(x) dx using the given table of values?
-By recognizing that g'(f(x)) * f'(x) is the derivative of g(f(x)) by the chain rule, we can apply the fundamental theorem of calculus to find g(f(3)) - g(f(0)) using the table values, which results in g(1) - g(2) = -5 - (-2) = -3.
What is the equation for the line tangent to the solution curve of dy/dx = y + 1/x at the point (-2, 0)?
-The slope of the tangent line at (-2, 0) is the derivative at that point, which is -1/2. Using point-slope form, the equation of the tangent line is y = -1/2 * x + 1.
How do you approximate f(-1.5) using the tangent line equation for the differential equation dy/dx = y + 1/x?
-By substituting x = -1.5 into the tangent line equation y = -1/2 * x + 1, we get f(-1.5) ≈ -1/2 * (-1.5) + 1 = 0.75 + 1 = 1.75.
What is the particular solution to the differential equation dy/dx = y + 1/x with the initial condition f(-2) = 0?
-Separating variables, we get dy/(y + 1) = dx/x. Integrating both sides gives ln(|y + 1|) = ln(|x|) + C. Using the initial condition f(-2) = 0, we find d = -1/2. Thus, the solution is y + 1 = -1/2 * x, or y = -1/2 * x - 1.
What are the common integrals of inverse trigonometric functions that should be known?
-Some common integrals of inverse trigonometric functions are: ∫(1/√(1 - x^2)) dx = sin^(-1)(x) + C, ∫(1/(1 + x^2)) dx = arctan(x) + C, and ∫(1/(√(x^2 - 1))) dx = sec^(-1)(x) + C.
How do you find the constant of integration, C, in the solution to a differential equation?
-To find the constant of integration, C, you typically use an initial condition or boundary condition provided with the differential equation. By substituting the given values into the solution, you can solve for C algebraically.
Outlines
📚 Integral Calculations and Differential Equations
This paragraph covers a range of mathematical topics including integral calculus and differential equations. It begins with the integration of 1/(xlnx) using substitution, where u is set to ln(x), leading to the result ln(|ln(x)|) + C. The solution to the differential equation dy/dx = x^2/y^4 with y(3) = 0 is found using separation of variables, resulting in y^(5/5) = x^(3/3) + C, and then solving for y to get y as the fifth root of (5x^3/(3-9)). The integral of 8/(1+x^2) dx is evaluated as 8arctan(x) + C. Several standard integrals of inverse trigonometric functions are also listed. Lastly, a differential equation dy/dx = xy + 3y is solved using separation of variables, leading to y = ±e^(c)e^(x^2/2+3x), where c is determined using the initial condition y(0) = 5, resulting in y = 5e^(x^2/2+3x).
🔍 Evaluating Definite Integrals and Approximating Functions
The second paragraph involves evaluating a definite integral using both direct computation and substitution methods. The integral in question is ∫ from 0 to 3 of (g'(f(x)) * f'(x) dx), which is recognized as the chain rule derivative of g(f(x)). Using the fundamental theorem of calculus and given values from a table, the integral is computed as g(1) - g(2) = -5 - (-2) = -3. The paragraph also discusses approximating the value of a function at a given point using the tangent line to its graph. For the differential equation dy/dx = y + 1/x, with the initial condition y(-2) = 0, the tangent line at the point (-2, 0) is derived, and used to approximate y at x = -1.5, resulting in an approximate value of -1.5 for f(-1.5). Lastly, the particular solution to the differential equation dy/dx = y + 1/x with the same initial condition is found by separating variables and integrating, leading to y + 1/x = ±e^c, and using the initial condition to solve for c, which gives y = -1/2x - 1.
🧮 Solving Differential Equations with Initial Conditions
The final paragraph focuses on solving a specific differential equation dy/dx = y + 1/x with the initial condition y(-2) = 0. The solution process involves separating variables, integrating both sides, and applying the initial condition to find the constant of integration. The integral of dy/(y + 1) dx results in ln|y + 1| = ln|x| + C, which is then simplified using logarithmic properties to ln|(y + 1)/x| = C. Exponentiating both sides leads to (y + 1)/x = ±e^C, and with the given initial condition, the constant C (referred to as d in the text) is found to be -1/2. The particular solution is then expressed as y + 1 = -1/2x, which simplifies to y = -1/2x - 1.
Mindmap
Keywords
💡Substitution
💡Natural Logarithm
💡Separation of Variables
💡Differential Equation
💡Integration
💡Inverse Trigonometric Functions
💡Chain Rule
💡Fundamental Theorem of Calculus
💡Exponential Function
💡Initial Condition
💡Point-Slope Form
Highlights
Substitution method is used to solve the integral of 1/x * ln(x) dx.
Let u = ln(x), then du = 1/x dx, to transform the integral into ∫1/u du.
The integral ∫1/u du evaluates to ln|u| + C, which simplifies to ln|ln(x)| + C after substitution.
Separation of variables technique is applied to solve the differential equation dy/dx = x^2/y^4.
After integrating, the solution is y^(5/4) = (x^3)/(3) + C, with C found using the initial condition y(3) = 0.
The particular solution y = (5x^3)/(3 - 9) is obtained after finding the constant C.
The integral ∫8/(1+x^2) dx is solved by pulling out the constant 8 and using the arctan integral.
Key inverse trig integrals are summarized: 1/√(1-x^2) = arcsin(x) + C, 1/(1+x^2) = arctan(x) + C, etc.
Separation of variables is again used to solve dy/dx = xy + 3y, factoring out y first.
Integrating both sides gives ln|y| = (x^2)/2 + 3x + C, which is exponentiated to find y.
The particular solution y = 5e^((x^2)/2 + 3x) is found using the initial condition y(0) = 5.
The integral ∫g'(f(x))f'(x) dx from 0 to 3 is evaluated using the chain rule and fundamental theorem.
Alternatively, substitution u = f(x) is used to change the limits and solve the integral as ∫g'(u) du from 2 to 1.
The tangent line equation at (-2, 0) for dy/dx = y + 1/x is derived using point-slope form.
The tangent line is used to approximate f(-1.5) ≈ -0.25 for the solution curve of dy/dx = y + 1/x.
The particular solution y = f(x) = -0.5x - 1 to the differential equation dy/dx = y + 1/x is found.
Initial condition f(-2) = 0 is used to determine the constant d in the general solution y + 1/x = d.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: