AP Calculus BC 2008 Multiple Choice (calculator) - Questions 76 - 92

vinteachesmath

31 Mar 202234:43

EducationalLearning

32 Likes 10 Comments

TLDRThis video script is a comprehensive review of the 2008 BC Calculus Multiple Choice Calculator section by Vin. It covers a range of topics, including the interpretation of derivatives, integrals, limits, and the application of various calculus theorems. Vin provides step-by-step solutions to questions, emphasizing the importance of understanding concepts like the integral test, Taylor polynomials, and the first derivative test. The script is designed to prepare students for the AP Calculus exam, highlighting common pitfalls and offering strategies for tackling complex problems efficiently.

Takeaways

📚 The script is a detailed walkthrough of the 2008 BC Calculus Multiple Choice Calculator section, aiming to help students understand and solve calculus problems.
📉 The speaker explains how to interpret the graph of a derivative function to identify local maxima, minima, and points of inflection, highlighting common pitfalls and the importance of careful analysis.
💧 The concept of integrating a rate function over time to find the total volume of water pumped out of a lake is discussed, with an example calculation provided.
🔍 The importance of understanding limits and how they approach a certain value is emphasized, with the speaker demonstrating how to determine the correct values of 'c' for which the limit exists.
📊 The script covers the integral test for convergence or divergence of series, cautioning against common misconceptions and explaining the correct application of the test.
∫ The process of finding the area between two curves using definite integrals is explained, with a focus on using the calculator to simplify the process.
🔢 The speaker demonstrates how to use the calculator to find points of inflection by taking the second derivative of a given function and analyzing where it changes sign.
📌 The concept of breaking an integral into parts to find the value of an unknown segment is illustrated, using the given integrals and their corresponding areas.
🔄 The direct comparison test for series convergence and divergence is explained, with an example showing how a smaller divergent series implies the divergence of a larger series.
📐 The script includes a problem involving finding the area between curves by setting up integrals and using the absolute value to account for the changing roles of the top and bottom functions.
📈 The importance of knowing formulas for Taylor polynomials and how to apply them to find a polynomial that approximates a given function is discussed, with a step-by-step example provided.

Q & A

What is the main topic of the video?
-The main topic of the video is a walkthrough of the 2008 BC Calculus Multiple Choice Calculator section, explaining the solutions to various calculus problems.
How does the speaker determine that there is a local maximum at x equals c in question 76?
-The speaker determines that there is a local maximum at x equals c by observing that f' (the derivative of f) goes from positive to negative, which indicates a local maximum for the original function f, not f'.
What is the significance of the integral of the rate in question 77?
-The integral of the rate is significant because it represents the total amount of water pumped out of the lake from time zero to five, as the rate is given in cubic meters per minute and the integral calculates the total volume over time.
Why does the speaker caution about the graph of f' being a point of inflection in question 78?
-The speaker cautions because a point of inflection refers to a change in concavity of the original function f, not its derivative f'. The speaker clarifies that f' changing from negative to positive at x equals a indicates a local minimum for f, not a point of inflection.
What theorem does the speaker refer to in question 79 regarding the series convergence?
-The speaker refers to the Integral Test for convergence or divergence of a series, which is applicable when the terms of the series are positive, continuous, and decreasing.
How does the speaker approach finding the points of inflection in question 80?
-The speaker approaches finding the points of inflection by taking the second derivative of the given function f' and looking for where it changes sign over the interval from negative two to two.
What concept does the speaker use to solve the integral problem in question 81?
-The speaker uses the concept that the whole integral from a to b is equal to the sum of its parts, calculating the integral from a to c by subtracting the integral from c to b from the integral from a to b.
What test does the speaker apply in question 82 to determine the divergence of the series b_sub_n?
-The speaker applies the Direct Comparison Test to determine that if a smaller series a_sub_n diverges, then the larger series b_sub_n, which is greater than or equal to a_sub_n, must also diverge.
How does the speaker solve the area between curves problem in question 83?
-The speaker solves the problem by using the absolute value of the difference between the two functions over the interval from one to five, which accounts for the changing roles of the top and bottom functions.
What is the key formula the speaker uses in question 84 for the Taylor polynomial?
-The key formula used for the Taylor polynomial is f(x) ≈ f(3) + f'(3)(x-3) + f''(3)(x-3)^2/2! + f'''(3)(x-3)^3/3!, where f', f'', and f''' represent the first, second, and third derivatives of f evaluated at x=3.

Outlines

00:00

📚 AP Calculus BC Section Review

In this segment, Vin delves into the 2008 BC Calculus multiple-choice section, starting with Question 76, which involves analyzing a graph of a derivative to determine properties of the original function. He clarifies common misconceptions about local maxima and minima, points of inflection, and the concavity of a function. Vin also explains how to calculate the volume of water pumped from a lake over time using integration and highlights the importance of understanding the difference between the integral of a rate and the rate itself.

05:00

🔍 Limit Analysis and Theorems Application

Vin continues with an in-depth analysis of limits, integral tests for convergence or divergence of series, and the application of the integral test. He emphasizes the importance of recognizing when a series converges and understanding the implications for related series. Additionally, he discusses the use of the calculator to find points of inflection and the strategy for solving integrals involving the difference of two functions.

10:01

📉 Calculus Concepts and Theorems Clarification

This paragraph focuses on the application of calculus theorems and concepts, such as the integral test, direct comparison test, and the area between curves. Vin explains how to use the calculator to find the area between two functions and the importance of understanding the relationship between the integral of a function and the sum of its series representation.

15:02

📝 Taylor Polynomials and Particle Motion Analysis

Vin discusses the concept of Taylor polynomials, specifically a third-degree Taylor polynomial, and how to construct it using given derivatives at a center point. He also addresses the motion of a particle on the x-axis, explaining how to determine the number of times the particle changes direction by analyzing the velocity function.

20:02

📈 Derivative Applications and Function Analysis

In this section, Vin examines the use of derivatives to analyze the slope of a function at any point and to determine subsequent function values given an initial condition. He also explains how to find the position of an object at a specific time using the fundamental theorem of calculus and the concept of antiderivatives.

25:03

🤔 Theorems of Calculus and Their Implications

Vin wraps up the script with a discussion on the implications of calculus theorems, such as the Mean Value Theorem and Rolle's Theorem, and how they relate to the behavior of functions and their derivatives. He also covers the use of the chain rule and the first derivative test to find relative maxima and the application of the fundamental theorem of calculus to find the slope of a composite function.

Mindmap

Keywords

💡Derivative

The derivative is a fundamental concept in calculus representing the rate of change of a function with respect to one of its variables. In the context of the video, the derivative of a function, denoted as f'(x), is used to analyze the behavior of a function, such as identifying local maxima and minima, points of inflection, and the concavity of a function. For example, the script discusses how a change from positive to negative in the derivative indicates a local maximum at a certain point x=c.

💡Integral

An integral is a mathematical concept that represents the area under the curve of a function, or the accumulated quantity of a variable. In the video, integrals are used to calculate the total volume of water pumped out of a lake over time and to determine the area between curves. The script illustrates the process of integrating a rate function to find the total volume pumped out from time zero to five.

💡Limit

A limit is a value that a function or sequence approaches as the input approaches some value. In the script, the concept of a limit is used to determine the values of x for which the function f(x) approaches a certain y-value, such as one. The video explains how to analyze the behavior of a function as x approaches a specific value, c, and how this relates to the limit of the function at that point.

💡Convergence

Convergence in the context of series refers to the property where the sum of the series approaches a finite limit. The video discusses the integral test for convergence, which is a method to determine whether an infinite series converges by comparing it to an improper integral. The script warns against a common misconception about the relationship between the sum of a series and its corresponding improper integral.

💡Diverges

Diverges refers to the property of a series where the sum does not approach a finite limit and instead grows without bound. The script explains the direct comparison test for divergence, which states that if a smaller series diverges, then a larger series must also diverge. This concept is used to determine the behavior of a series based on the behavior of another series that is known to diverge.

💡Taylor Polynomial

A Taylor polynomial is an approximation of a function near a specific point using the function's derivatives at that point. In the video, a third-degree Taylor polynomial is discussed, which is used to approximate a function based on its value and the values of its first three derivatives at the center point x=3. The script demonstrates how to construct a Taylor polynomial using given derivatives and the center point.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for differentiating composite functions. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. In the script, the chain rule is used to find the derivative of a function composed of two other functions, such as h(x) = f(g(x)), and to calculate the derivative of h at a specific point.

💡First Derivative Test

The first derivative test is a method used to determine the relative extrema (maximum or minimum) of a function by analyzing the sign changes of its first derivative. The script explains how to use the first derivative test to find where a function attains a relative maximum by looking for points where the first derivative changes from positive to negative.

💡Second Derivative

The second derivative of a function is the derivative of its first derivative and provides information about the concavity of the function. In the video, the second derivative is used to find points of inflection, where the function changes concavity. The script demonstrates how to calculate the second derivative and use it to determine the number of points of inflection on a given interval.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus is a key theorem that links the concept of the integral to that of the derivative, showing that the definite integral of a function can be computed by finding the antiderivative of the function and evaluating it at the limits of integration. In the script, the theorem is used to relate the area under the curve of a function to the antiderivative of the function's rate, and to find the position of an object given its velocity function.

Highlights

Introduction to the 2008 BC Calculus Multiple Choice Calculator section review.

Explanation of how to interpret the graph of a derivative to identify local maxima and minima.

Clarification of the difference between a local maximum of a function and its derivative.

Identification of points of inflection by analyzing changes in the second derivative's sign.

Use of integrals to calculate the volume of water pumped out of a lake over time.

Discussion on the importance of understanding limits and their values in calculus problems.

Application of the Integral Test for convergence or divergence of series.

The misconception about the relationship between improper integrals and series clarified.

Finding points of inflection using the second derivative without manual calculation.

Use of the concept 'the whole is equal to the sum of its parts' in integral calculus.

Explanation of the Direct Comparison Test for divergence of series.

Calculation of the area between curves using integrals and absolute value.

Understanding Taylor polynomials and their application in approximating functions.

Analysis of a particle's motion to determine the number of direction changes based on velocity function.

Derivation of a function's equation from its derivative using initial conditions.

Application of the Fundamental Theorem of Calculus to find the position of an object.

Differentiation of an area function to analyze the behavior of a related function.

Use of Mean Value Theorem and Rolle's Theorem to deduce properties of a function.

Application of the Chain Rule to find the derivative of a composite function.

Utilization of the First Derivative Test to identify relative maxima.

Calculation of a derivative using the Chain Rule for a composite function involving two functions.

Transcripts

Browse More Related Video

AP Calculus AB 2003 Multiple Choice (no calculator) - Questions 1-28

AP Calculus AB 2012 Multiple Choice (no calculator) - Questions 1-28

AP Calculus Multiple Choice Practice Test (2020 AP CED Problems)

AP Calculus AB/BC Multiple Choice Practice Test (2012 AP CED Problems)

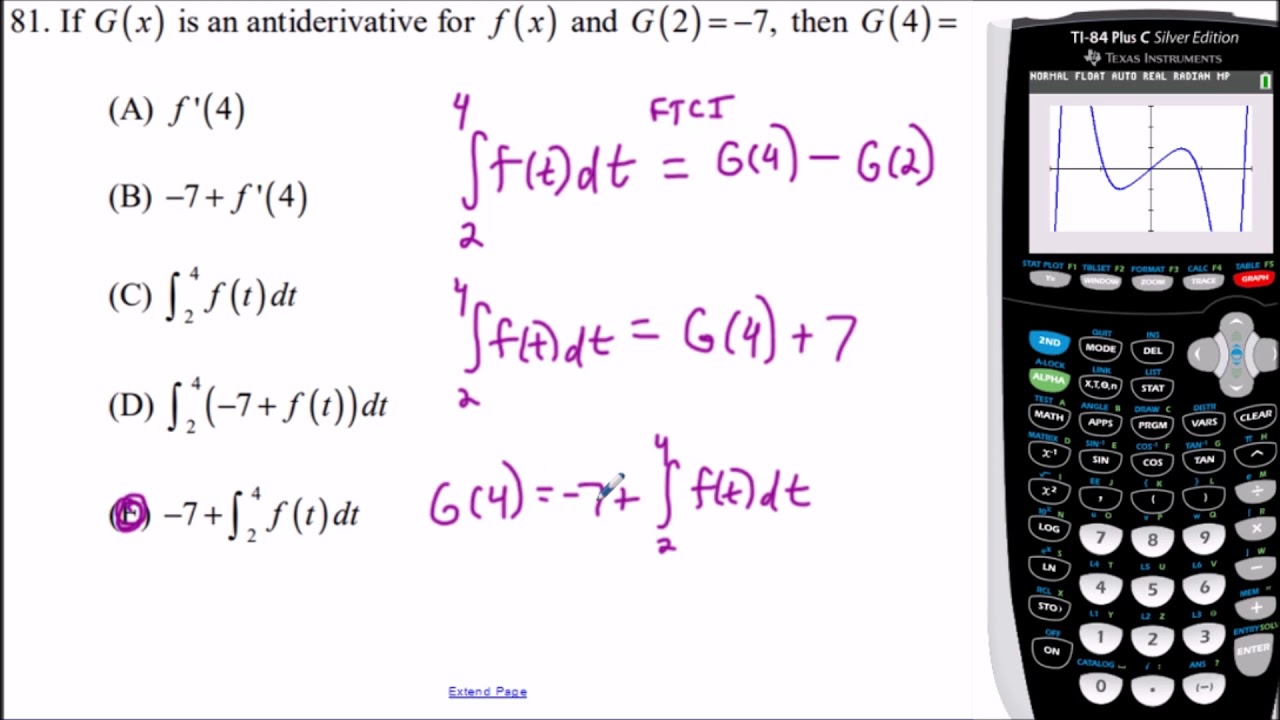
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28

Related Tags

Calculus Tutorial AP Exam Derivatives Integrals Math Analysis Educational Content Test Preparation Theorems Chain Rule Fundamental Theorem Mathematics Education