Atomic Mass: How to Calculate Isotope Abundance

Tyler DeWitt
3 Oct 201211:48
EducationalLearning
32 Likes 10 Comments

TLDRThe video script is an educational walkthrough on calculating the percent abundance of isotopes given the atomic mass of an element. Using chlorine as an example, the narrator demonstrates how to set up variables for the abundance of two isotopes, Cl-35 and Cl-37, and solve for their respective percentages. The key insight is that the sum of the abundances must equal 100%, leading to the expression of one isotope's abundance in terms of the other. The process involves multiplying the mass of each isotope by its abundance, summing these products, and equating the result to the known relative atomic mass of the element. The script also illustrates a similar calculation for lithium isotopes, emphasizing the step-by-step mathematical approach and the conversion of final decimals to percentages. This methodical explanation empowers viewers to tackle such problems independently, providing a clear understanding of isotopic abundance calculations.

Takeaways
  • 🧠 **Understanding Isotopes**: The video discusses how to calculate the percent abundance of isotopes given their atomic masses and the element's average atomic mass.
  • 📊 **Assigning Variables**: Introduces variables X and Y to represent the unknown percent abundances of two isotopes, with Y expressed as 1 - X to simplify calculations.
  • 🔍 **Observing Patterns**: Highlights the pattern that the sum of the decimal abundances of two isotopes must equal 1, which helps in expressing one isotope's abundance in terms of the other.
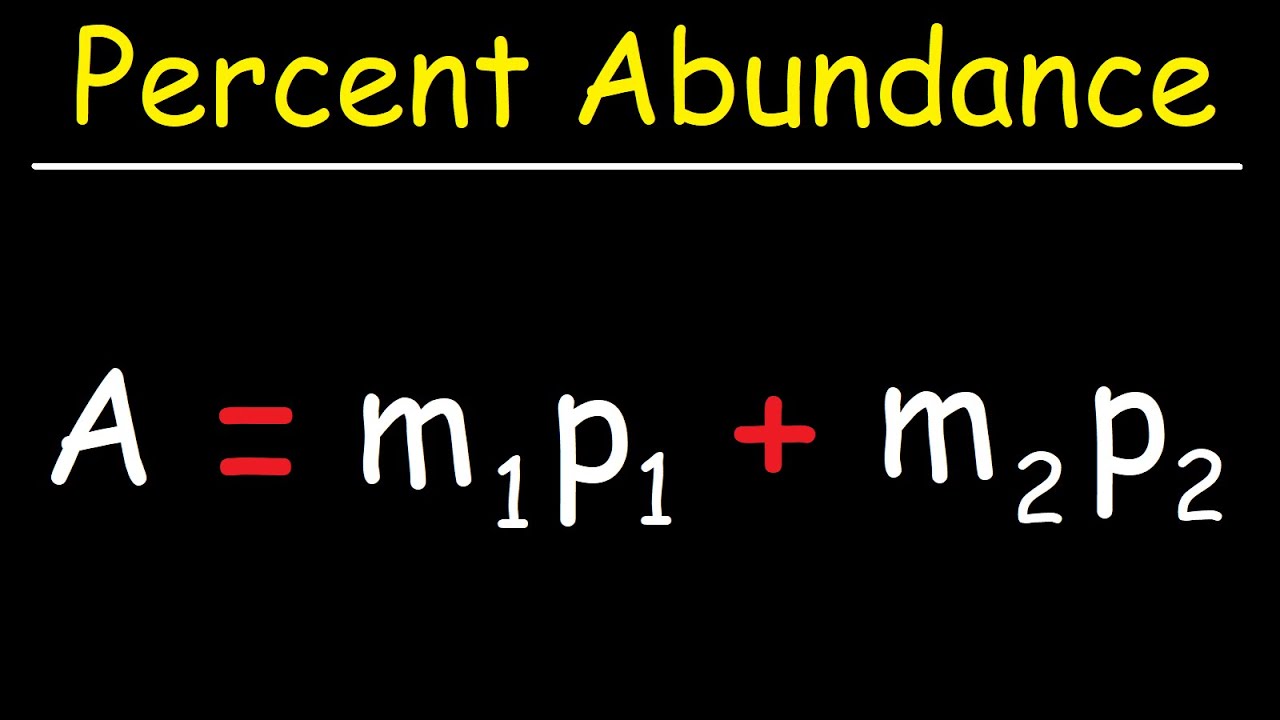
  • 📐 **Setting Up the Equation**: Demonstrates setting up an equation using the known atomic mass of an element and the masses of its isotopes multiplied by their respective abundances.
  • 🧮 **Solving for Abundance**: Shows the step-by-step algebraic process to solve for the variable representing one isotope's abundance, which then allows finding the other isotope's abundance.
  • ⚖️ **Atomic Mass Unit (AMU)**: Emphasizes the use of AMU as the unit for expressing atomic mass and how it's used in the calculation of isotopes' abundance.
  • 🔢 **Converting Decimals to Percentages**: Explains the conversion of decimal abundances to percentages by multiplying by 100, which is essential for expressing the results.
  • 🌐 **Periodic Table Reference**: Mentions the use of the periodic table to find the relative atomic mass of an element when it's not provided in the problem.
  • 📝 **Step-by-Step Calculation**: Provides a clear, step-by-step method to perform the calculations, making it easier for viewers to follow and replicate the process.
  • 📚 **Educational Approach**: The script is educational, designed to teach the concept of isotope abundance calculation through a detailed example.
  • ✅ **Verification of Results**: Suggests the importance of verifying the results by ensuring the sum of the calculated abundances equals 100% or 1 when expressed as decimals.
Q & A

  • What is the relative atomic mass of chlorine?

    -The relative atomic mass of chlorine is 35.45 AMU.

  • What are the two stable isotopes of chlorine mentioned in the script?

    -The two stable isotopes of chlorine mentioned are chlorine-35 and chlorine-37.

  • What is the mass of chlorine-35 in AMU?

    -The mass of chlorine-35 is 34.97 AMU.

  • What is the mass of chlorine-37 in AMU?

    -The mass of chlorine-37 is 36.97 AMU.

  • How are the variables X and Y used to represent the abundance of chlorine-35 and chlorine-37?

    -X is used to represent the percent abundance of chlorine-35, and Y (which is expressed as 1 - X) is used for chlorine-37, since the total abundance must equal 100%.

  • What is the equation used to calculate the relative atomic mass of an element based on its isotopes?

    -The equation is the sum of the products of each isotope's mass and its respective abundance (expressed as a decimal).

  • How do you express the abundance of chlorine-37 in terms of X?

    -The abundance of chlorine-37 is expressed as 1 - X, where X is the abundance of chlorine-35.

  • What is the percent abundance of chlorine-35 calculated to be in the script?

    -The percent abundance of chlorine-35 is calculated to be 76.0%.

  • What is the percent abundance of chlorine-37 calculated to be in the script?

    -The percent abundance of chlorine-37 is calculated to be 24.0%.

  • What is the mass of lithium-6 in AMU?

    -The mass of lithium-6 is 6.015 AMU.

  • What is the mass of lithium-7 in AMU?

    -The mass of lithium-7 is 7.016 AMU.

  • How do you find the atomic mass of lithium if it's not given in the problem?

    -You can find the atomic mass of lithium by looking it up on the periodic table, where the relative atomic mass is provided.

Outlines
00:00
🔍 Determining Isotope Abundances from Atomic Mass

The first paragraph introduces a method for calculating the percent abundance of isotopes when the atomic mass of an element is known. It uses chlorine as an example with its two stable isotopes, chlorine-35 and chlorine-37, having masses of 34.97 AMU and 36.97 AMU respectively. The relative atomic mass of chlorine is given as 35.45 AMU. The paragraph walks through the process of setting up variables for the abundance of each isotope (X for chlorine-35 and 1-X for chlorine-37), considering their sum must equal 100%. It then forms an equation using the masses and variable abundances to solve for the actual percent abundances, resulting in 76.0% for chlorine-35 and 24.0% for chlorine-37.

05:02
🧮 Solving for Isotope Abundances with Algebra

The second paragraph demonstrates algebraic manipulation to solve for the abundance of isotopes. It begins by setting up an equation using the masses of the isotopes and their respective abundances, which are represented by variables X and 1-X. The paragraph shows the step-by-step algebraic process, including distributing, combining like terms, and isolating the variable to find the abundance of lithium-6 as 7.5% and lithium-7 as 92.5%. The key takeaway is the method of expressing one isotope's abundance in terms of the other to simplify calculations, and the importance of converting final decimals to percentages.

10:06
📚 Applying the Method to Lithium Isotopes

The third paragraph applies the previously explained method to find the abundance of lithium's naturally occurring isotopes, lithium-6 and lithium-7, with given masses of 6.015 AMU and 7.016 AMU. It emphasizes the need to find lithium's atomic mass from the periodic table, which is 6.941. The paragraph outlines the algebraic steps to set up an equation with the known masses and solve for the variable representing the abundance of lithium-6 (X). It concludes by calculating the abundance of lithium-6 as 7.5% and lithium-7 as 92.5%, reiterating the process of converting the final decimal result into a percentage.

Mindmap
Keywords
💡Isotopes
Isotopes are variants of a particular chemical element which differ in neutron number, and hence in nucleon number. The concept is central to the video as it discusses calculating the abundance of different isotopes of chlorine and lithium. In the script, chlorine-35 and chlorine-37, as well as lithium-6 and lithium-7, are used to illustrate the process of determining isotopic abundance.
💡Atomic Mass Unit (AMU)
An atomic mass unit is a unit of mass that quantifies mass on an atomic or molecular scale. It is defined as one twelfth of the mass of an unbound carbon-12 atom. The video uses AMU to express the mass of different isotopes, which is essential for calculating their percent abundance.
💡Percent Abundance
Percent abundance refers to the percentage of a particular isotope in a naturally occurring element. The video's primary objective is to teach how to calculate the percent abundance of isotopes, using the known relative atomic mass of the element and the masses of its isotopes.
💡Relative Atomic Mass
The relative atomic mass is a dimensionless value that compares the mass of an atom to 1/12 of the mass of a carbon-12 atom. It is used in the video to determine the overall atomic mass of elements like chlorine and lithium based on the abundance of their isotopes.
💡Variables
In the context of the video, variables are used to represent the unknown percent abundances of isotopes, such as X for chlorine-35 and Y (or 1-X in this case) for chlorine-37. The video demonstrates how to use these variables in equations to solve for the abundances.
💡Equations
Equations are used to express the relationship between the masses of isotopes, their abundances, and the relative atomic mass. The video involves setting up and solving equations to find the percent abundance of each isotope, showcasing the mathematical process involved.
💡Decimals and Percentages
Decimals and percentages are used to represent the abundances of isotopes. The video explains how to convert between these two forms, emphasizing that the sum of the abundances in decimal form equals one, and in percentage form equals 100%.
💡Solving for Abundance
The process of determining the isotopic abundance involves setting up equations and solving for the variables representing the percent abundances. The video provides a step-by-step guide on how to perform these calculations for chlorine and lithium isotopes.
💡Element and Isotope
An element is a substance made of atoms with the same number of protons, while an isotope is a variant of an element with a different number of neutrons. The video focuses on isotopes of chlorine and lithium, demonstrating how to calculate their abundance in the context of the element as a whole.
💡Periodic Table
The periodic table is a tabular arrangement of the chemical elements, ordered by their atomic number, electron configuration, and recurring chemical properties. It is referenced in the video to find the relative atomic mass of lithium, which is necessary for the abundance calculation.
💡Mathematical Operations
The video involves various mathematical operations such as multiplication, division, addition, and subtraction to solve for isotopic abundance. These operations are applied to the masses of isotopes and their respective abundances to find the overall atomic mass and the individual percent abundances.
💡Natural Occurrence
Natural occurrence refers to the presence of isotopes in nature. The video discusses naturally occurring isotopes of chlorine and lithium, emphasizing that the calculation of their abundance is based on their natural occurrence and not synthetic or artificial creation.
Highlights

The process involves determining the percent abundance of isotopes given the atomic mass of an element.

Two stable isotopes of chlorine, chlorine-35 and chlorine-37, are used as an example with their respective masses of 34.97 AMU and 36.97 AMU.

The relative atomic mass of chlorine is given as 35.45 AMU, which is used to find the isotopes' abundance.

Variables are assigned to represent the unknown percent abundances, with chlorine-35 as 'X' and chlorine-37 expressed as '1-X'.

A pattern is observed where the sum of the abundances of the two isotopes equals 100% or 1 when expressed as decimals.

An equation is formed using the masses of the isotopes multiplied by their respective abundances, which equals the relative atomic mass of chlorine.

The equation is solved algebraically to find the value of 'X', which represents the percent abundance of chlorine-35.

After finding 'X', the percent abundance of chlorine-37 is calculated using the relationship '1-X'.

The calculated abundance of chlorine-35 is found to be 76.0%, and chlorine-37 is 24.0%.

A similar method is applied to lithium isotopes, lithium-6 and lithium-7, using their masses and the atomic mass of lithium from the periodic table.

The abundances of lithium-6 and lithium-7 are represented by 'X' and '1-X' respectively, and an equation is set up accordingly.

The atomic mass of lithium is used to solve for 'X', which represents the percent abundance of lithium-6.

The abundance of lithium-6 is calculated to be 7.5%, and by subtraction, lithium-7 is found to be 92.5%.

The key to solving these problems is setting up the correct algebraic equation based on the given masses and atomic mass, and solving for the variable representing abundance.

The process requires converting the final decimal results into percentages by multiplying by 100.

The method demonstrated is applicable to any element with known isotopes and their masses, providing a systematic way to determine isotopic abundance.

The problem-solving approach emphasizes the importance of understanding the relationship between isotopic masses, their abundances, and the element's atomic mass.

Transcripts

Browse More Related Video

How To Find The Percent Abundance of Each Isotope - Chemistry

Isotopes, Percent Abundance, Atomic Mass | How to Pass Chemistry

How To Calculate The Average Atomic Mass

Atomic Mass: Introduction

13.04 Isotopic Abundance in Mass Spectrometry

Protons Neutrons Electrons Isotopes - Average Mass Number & Atomic Structure - Atoms vs Ions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Isotope AbundanceAtomic MassChemical CalculationElemental AnalysisChlorine IsotopesLithium IsotopesPeriodic TableMathematical ProblemEducational ContentScience Tutorial