Basic Derivative Rules - Part 3: Applications
TLDRThis video script is the third part of a lecture series focusing on basic derivative rules and their applications. The lecturer begins by illustrating how to find the equation of a tangent line to a given function at a specific point, using the example of a parabola at x equals two. The process involves calculating the derivative of the function, evaluating it at the given point to find the slope of the tangent line, and then using the point-slope form to derive the equation of the tangent line. The script also covers the concept of the derivative as an instantaneous rate of change, with applications including estimating the rate of change of power with respect to velocity for a windmill and calculating the instantaneous velocity from a position function. The lecturer emphasizes the importance of understanding the derivative as both a slope and a rate of change, and provides step-by-step calculations to ensure clarity and accuracy.
Takeaways
- 📚 The lecture focuses on applications of basic derivative rules, specifically finding the equation of a tangent line to a function at a given point.
- 📈 The process involves finding the derivative of the function, which represents the slope of the tangent line at a specific point.
- 🔍 For the first example, the function is a parabola with an upturning shape, and the tangent line is to be found at x = 2.
- 🧮 The derivative of the given function is found to be 6x, and it is evaluated at x = 2 to get the slope of the tangent line, which is 12.
- 📐 Using the point-slope form of a line (y - y1 = m(x - x1)), the equation of the tangent line is derived with the known slope and a specific point on the function.
- 📝 The function value at x = 2 is calculated to be 7, which is used as y1 in the point-slope form to find the equation of the tangent line.
- 🔑 The final equation of the tangent line is simplified to y = 12x - 17, which is in slope-intercept form, a standard way to express a linear equation.
- ⚙️ The second part of the lecture deals with the concept of the instantaneous rate of change, exemplified by estimating the rate of change of power with respect to velocity for a windmill.
- 🌪️ The derivative of the power function with respect to velocity is calculated, and then evaluated at a velocity of 8.3 miles per hour to find the instantaneous rate of change.
- 🚀 The derivative of the position function gives the velocity function, which is used to find the instantaneous velocity at a specific time.
- ⏱️ At t = 2 seconds, the instantaneous velocity is found to be 26, by evaluating the velocity function at that time.
- 🔗 The lecture connects the concept of derivatives to real-world applications, such as power generation and velocity, emphasizing the practical importance of understanding these mathematical concepts.
Q & A
What is the main focus of this video lecture?
-The video lecture focuses on applications of basic derivative rules, specifically finding the equation of a tangent line to a function at a given point and estimating the instantaneous rate of change.
What is the shape of the function discussed in the first example?
-The function in the first example is described as an upturning parabola.
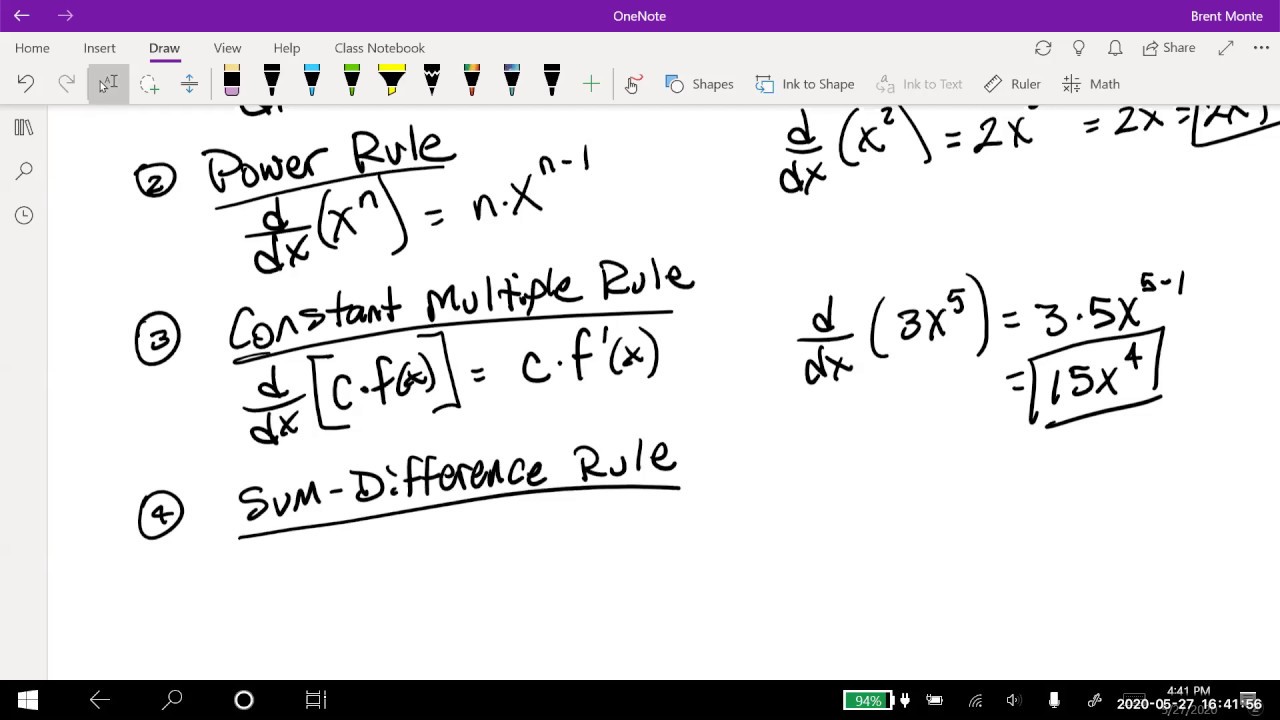
How is the derivative of the function calculated in the first example?
-The derivative of the function is calculated by taking the derivative of each term: six times x for the term '3x^2', and zero for the constant term '-5', resulting in the derivative being '6x'.
What does the value 12 represent in the context of the first example?
-The value 12 represents the slope of the tangent line at the point x equals two.
What is the point-slope form of a line and how is it used in the first example?
-The point-slope form of a line is 'y - y1 = m * (x - x1)' where m is the slope and (x1, y1) is a point on the line. It is used to find the equation of the tangent line by substituting the slope and the coordinates of the given point.
What is the function value at x equals two in the first example?
-The function value at x equals two is 7, which is calculated as 3 times (2^2) minus 5, resulting in 12 - 5.
How is the equation of the tangent line expressed in the first example?
-The equation of the tangent line is expressed in slope-intercept form as 'y - 7 = 12 * (x - 2)' and then simplified to 'y = 12x - 17'.
What does the instantaneous rate of change represent in the context of the second example?
-The instantaneous rate of change represents the rate at which the power generated by a windmill changes with respect to velocity at a specific velocity of 8.3 miles per hour.
How is the derivative of the power function with respect to velocity calculated in the second example?
-The derivative is calculated by multiplying the constant 0.021 by 3 and then by the square of the velocity (v^2), resulting in '0.063 * v^2'.
What is the instantaneous rate of change of power with respect to velocity at a velocity of 8.3 miles per hour?
-The instantaneous rate of change at a velocity of 8.3 miles per hour is 4.34, obtained by evaluating '0.063 * (8.3)^2'.
How is the instantaneous velocity defined in the context of the third example?
-The instantaneous velocity is defined as the derivative of the position function with respect to time, which gives the velocity function.
What is the instantaneous velocity at 2 seconds in the third example?
-The instantaneous velocity at 2 seconds is 26, calculated by evaluating the velocity function at t equals 2, which results in '-32 * 2 + 90'.
Outlines
📈 Derivative Applications: Finding Tangent Line Equations
This paragraph focuses on applying derivative rules to find the equation of a tangent line to a given function at a specific point. The lecturer illustrates the process by using a parabola-shaped function and finding the tangent line at x = 2 without needing a graph. The derivative of the function, which is 6x, is calculated, and its value at x = 2 (f'(2) = 12) is used to determine the slope of the tangent line. The point-slope form of a line is then employed to derive the equation of the tangent line. Additionally, the function value at x = 2 is calculated to be 7, which, along with the slope, allows the equation to be written in slope-intercept form: y - 7 = 12(x - 2), simplifying to y = 12x - 17. The lecturer also touches on the concept of the derivative as the instantaneous rate of change, providing an example of estimating the rate of change of power with respect to velocity for a windmill at a velocity of 8.3 miles per hour.
🚀 Instantaneous Rate of Change: Power and Velocity
The second paragraph deals with the concept of the instantaneous rate of change, specifically in the context of power with respect to velocity. The lecturer presents a function P(v) that represents the power generated by a windmill in terms of its velocity. To estimate the instantaneous rate of change at a velocity of 8.3 miles per hour, the derivative of the power function is calculated, resulting in P'(v) = 0.063v^2. By substituting the velocity value into the derivative, the instantaneous rate of change is found to be 0.063 * (8.3)^2 = 4.34. The paragraph also addresses another instantaneous rate of change problem related to velocity, which is the derivative of the position function. The position function is given as s(t), and its derivative, representing velocity, is found to be -32t + 90. The instantaneous velocity at t = 2 seconds is then calculated to be -32(2) + 90 = 26.
Mindmap
Keywords
💡Derivative
💡Tangent Line
💡Point-Slope Form
💡Instantaneous Rate of Change
💡Slope Intercept Form
💡Parabolas
💡Function Evaluation
💡Position Function
💡Velocity Function
💡Arithmetic Check
💡Contextualization
Highlights
The video focuses on applications of basic derivative rules.
The first example involves finding the equation of a tangent line to a parabola at x=2.
The function is described as an upturning parabola without the need to draw a graph.
The derivative of the function is found to be 6x, representing the slope of the tangent line.
The derivative at the point x=2 is evaluated to be 12, which is the slope of the tangent line at that point.
The point-slope form of a line is used to find the equation of the tangent line.
The function value at x=2 is calculated to be 7, providing the y-coordinate for the point of tangency.
The equation of the tangent line is written in slope-intercept form as y = 12x - 17.
The instantaneous rate of change is discussed as another interpretation of the derivative.
An example of estimating the instantaneous rate of change of power with respect to velocity at 8.3 miles per hour is given.
The derivative of the power function with respect to velocity is calculated to be 0.063v^2.
The instantaneous rate of change of power at a velocity of 8.3 mph is found to be 4.34.
Velocity is defined as the derivative of the position function, which is discussed in the context of a position function s(t).
The instantaneous velocity at 2 seconds is determined by evaluating the velocity function at that time.
The velocity function is derived from the position function, resulting in -32t + 90.
The instantaneous velocity at t=2 seconds is calculated to be 26.
The video concludes with the final example, summarizing the concepts taught.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: