Elasticity Resulting From Infinite Change In Quantity Demanded [Express Revenue As A Function Of P]

mathmontrealmath
23 Jun 202205:44
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a detailed mathematical explanation on isolating a variable 'x' in an equation, expressing it in terms of 'p', and then deriving a revenue function based on 'p'. The focus then shifts to calculating the price elasticity of demand, which involves taking the derivative of the revenue function. The process includes simplifying the function and applying the elasticity formula, which is the negative ratio of the percentage change in quantity demanded to the percentage change in price. The script concludes with a hypothetical scenario where the price elasticity is evaluated at a specific price point, illustrating the concepts of elastic and inelastic demand.

Takeaways
  • 🧮 To isolate x, you can derive it from the equation by dividing both sides by the coefficient of x, in this case, 0.03.
  • 📉 The resulting function in terms of p is derived from the initial equation by substituting x with the expression in terms of p.
  • 💰 Revenue can be expressed as a function of either x or p, as it is the product of quantity (x) and price (p).
  • 🔢 Simplification of the revenue function in terms of p is not necessary for the next steps, but it can be done for clarity.
  • 📌 The derivative of the revenue function with respect to p is required for calculating price elasticity of demand.
  • 📐 The elasticity formula uses the derivative of the revenue function and the original revenue function itself.
  • 🚫 There's no need to simplify the derivative further before applying it to the elasticity formula.
  • 🔄 The elasticity calculation involves multiplying the derivative by -p and then dividing by the revenue function.
  • ➗ The elasticity formula results in a simplified expression that can be further simplified by dividing both the numerator and the denominator by the same number.
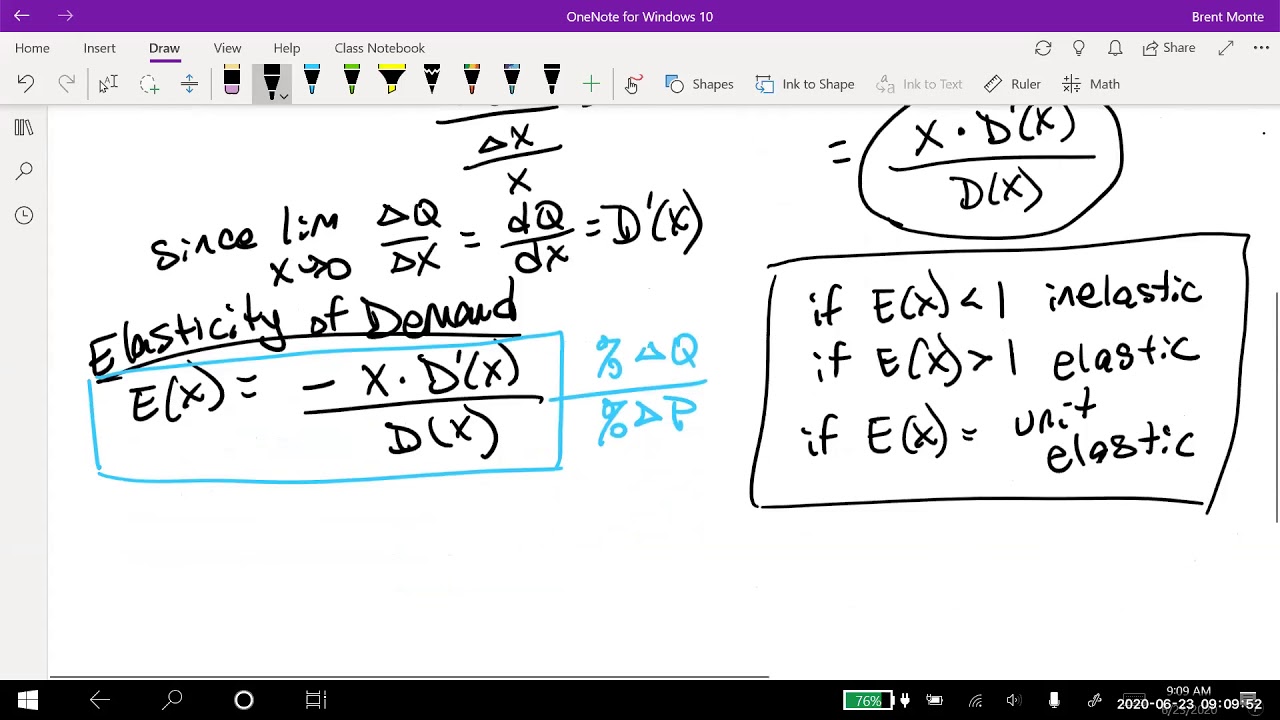
  • 📈 Elasticity greater than 1 indicates elastic demand, less than 1 indicates inelastic demand, and equal to 1 indicates unitary elastic demand.
  • ❓ If a price is given, you can plug it into the elasticity formula to determine the nature of demand at that price point.
Q & A

  • What is the initial step to isolate x in the given equation?

    -The initial step to isolate x is to divide both sides of the equation by 0.03.

  • How is the revenue function expressed in terms of p?

    -Revenue is expressed as a function of p by substituting the value of x in terms of p into the revenue formula, which is quantity times price.

  • What is the formula for revenue in terms of x?

    -The formula for revenue in terms of x is not explicitly given in the transcript, but it would typically be the product of x (quantity) and the price (p).

  • Why is it necessary to simplify the revenue function before calculating elasticity?

    -Simplifying the revenue function makes it easier to take the derivative, which is a required step in calculating the price elasticity of demand.

  • What is the derivative of the revenue function with respect to p?

    -The derivative of the revenue function with respect to p is -40, as derived from the simplified revenue function 30p - 40p^2.

  • How is the price elasticity of demand calculated?

    -The price elasticity of demand is calculated using the formula: (-p * derivative of revenue function with respect to p) / (revenue function itself).

  • What does the term 'elasticity' refer to in the context of economics?

    -In economics, 'elasticity' refers to the measure of how sensitive the quantity demanded or supplied of a good is to a change in the price of that good.

  • What does it mean if the price elasticity of demand is greater than one?

    -If the price elasticity of demand is greater than one, it indicates that the good is elastic, meaning that the percentage change in quantity demanded is greater than the percentage change in price.

  • What is the implication if the price elasticity of demand is less than one?

    -If the price elasticity of demand is less than one, it suggests that the good is inelastic, meaning that the percentage change in quantity demanded is less than the percentage change in price.

  • How can you determine the price elasticity of demand at a specific price point?

    -You can determine the price elasticity of demand at a specific price point by plugging the price into the elasticity formula and calculating the resulting value.

  • What is the significance of simplifying the elasticity formula to its simplest form?

    -Simplifying the elasticity formula to its simplest form makes it easier to interpret and compare the elasticity values across different goods or markets.

  • What does the term 'inelastic' imply about the responsiveness of quantity demanded to price changes?

    -The term 'inelastic' implies that the quantity demanded of a good is not very responsive to changes in the price, meaning that large price changes will result in relatively small changes in quantity demanded.

Outlines
00:00
📚 Isolating Variables and Calculating Revenue Functions

The first paragraph introduces the process of isolating the variable 'x' from an equation, which is equal to 30 minus 4 times 'p' divided by 0.03. The result is a function in 'p'. The speaker then explains that revenue can be expressed as a function of either 'x' or 'p', since revenue is the product of quantity and price. The focus is on expressing revenue as a function of 'p'. The paragraph concludes with a simplification of the revenue function and a brief mention of the next step, which is to calculate the derivative for elasticity.

05:02
📉 Calculating Price Elasticity of Demand

The second paragraph delves into the concept of price elasticity of demand. It outlines the formula for elasticity, which involves multiplying the price 'p' by the derivative of the revenue function with respect to 'p', and then dividing by the revenue function itself. The paragraph demonstrates the calculation of the derivative of the revenue function and then uses it to find the elasticity. The final step is to simplify the elasticity formula, resulting in an expression that can be used to determine whether demand is elastic or inelastic by comparing it to the value of 1. The paragraph also mentions that typically, an additional question might ask to calculate the necessity of the product at a given price.

Mindmap
Keywords
💡Isolate x
The process of solving an equation to express the variable x by itself. In the script, the speaker is trying to isolate x by starting with an equation that includes x and other variables, such as p. This is a fundamental step in algebra and is crucial for understanding the relationship between different variables in the given context.
💡Function in p
A mathematical expression where one variable, p, is treated as the independent variable, and the rest are expressed in terms of p. In the video, the speaker is focusing on expressing the revenue function purely in terms of the price variable p, which is essential for analyzing the economic model being discussed.
💡Revenue
Revenue refers to the total income generated from sales of goods or services. In the context of the video, revenue is calculated as the product of quantity (x) and price (p). It is a key concept in the discussion as the speaker is trying to express revenue as a function of the price variable (p) to understand its dependency and behavior.
💡Derivative
In calculus, the derivative of a function measures the rate at which the function value changes with respect to a change in its variable. In the script, the speaker calculates the derivative of the revenue function with respect to the price variable (p), which is a step towards finding the price elasticity of demand.
💡Price Elasticity of Demand
Price elasticity of demand measures the responsiveness of the quantity demanded of a good to a change in its price. It is a crucial concept in economics and business. In the video, the speaker is calculating the elasticity to understand how sensitive the demand is to price changes, which is done by taking the derivative of the revenue function and applying the elasticity formula.
💡Simplification
Simplification in mathematics involves reducing a complex expression to a more straightforward form. The speaker in the video simplifies expressions to make them easier to work with and understand. Simplification is important for clarity and for performing further calculations, such as taking derivatives or calculating elasticity.
💡Elasticity Formula
The elasticity formula is used to calculate the price elasticity of demand. It is given by the percentage change in quantity demanded divided by the percentage change in price. In the script, the speaker applies this formula by using the derivative of the revenue function to find the elasticity, which helps determine if the demand is elastic or inelastic.
💡Inelastic and Elastic Demand
Inelastic demand means that the quantity demanded does not respond much to a change in price, whereas elastic demand means that the quantity demanded responds significantly to a price change. The speaker discusses this concept in the context of applying the elasticity formula, noting that if the elasticity value is greater than one, demand is elastic; if it's less than one, demand is inelastic.
💡Unit of Measure
A unit of measure is a standard quantity that is used to express the magnitude of other quantities. In the video, the speaker simplifies the elasticity expression to a form that is easier to interpret, such as expressing it as a decimal (0.75) rather than a fraction (3/4), which makes it more accessible for understanding the economic concept.
💡Algebraic Manipulation
Algebraic manipulation refers to the process of rearranging and combining mathematical expressions according to algebraic rules to achieve a desired outcome. The speaker uses algebraic manipulation throughout the video to isolate variables, express functions in terms of other variables, and simplify expressions, which is essential for solving the economic problem presented.
💡Economic Model
An economic model is a theoretical construct representing economic processes by a set of variables and a set of equations that show the relationship between those variables. In the video, the speaker is working with an economic model that involves variables such as price (p) and quantity (x) to understand and predict the behavior of revenue and demand in response to price changes.
Highlights

The process of isolating x results in an equation with 0.03x equal to 30 minus 4p.

To express revenue as a function of p, you need to replace x with the expression in terms of p.

Revenue can be expressed as a function in x or p, since it's quantity times price, and quantity is x while price is p.

The transcript emphasizes the importance of expressing revenue solely in terms of p for the given problem.

A simplification step is suggested but deemed unnecessary for the current task.

The derivative of the revenue function is calculated to find elasticity.

The elasticity formula involves the price times the derivative of the function divided by the function itself.

The derivative of the revenue function in p is found to be minus 40 after simplification.

Elasticity is calculated using the formula, resulting in a positive value indicating elastic demand.

The elasticity calculation simplifies to 40p divided by (30 - 40p).

Further simplification of the elasticity formula is performed by dividing all terms by 40.

The final elasticity value is expressed as 0.75, indicating the degree of responsiveness of quantity demanded to price changes.

The concept of price elasticity of demand is discussed, highlighting the difference between elastic and inelastic demand.

A hypothetical scenario is presented where the price is given, and elasticity is used to determine the nature of demand.

The transcript explains how to interpret elasticity values in the context of demand responsiveness.

The process concludes without needing to apply the elasticity calculation to a specific price point.

The transcript provides a comprehensive guide on how to calculate and interpret price elasticity of demand.

Transcripts

Browse More Related Video

Elasticity of Demand - Example

Math 11 - Section 3.7

What is Elasticity?

Managerial Economics 2.5: More Elasticity

Elasticity of Demand

3.7 - Elasticity of Demand

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
EconomicsDerivationRevenue FunctionPrice ElasticityMathematical AnalysisElasticity CalculationSimplificationReal-world ApplicationEconomic TheoryQuantitative Methods