Cramer's Rule - 2x2 & 3x3 Matrices - Solving Systems of Linear Equations - 2 & 3 Variables

The Organic Chemistry Tutor

10 Oct 201638:02

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on applying Kramer's Rule to solve systems of linear equations with two and three variables. The process involves calculating determinants to find the values of x, y, and z for the given equations. The script provides step-by-step instructions, including setting up matrices, calculating determinants for 2x2 and 3x3 systems, and verifying the solutions. It emphasizes the importance of accuracy and patience in this method, suggesting practice for proficiency.

Takeaways

📚 Kramer's Rule is a method used to solve systems of linear equations with multiple variables.
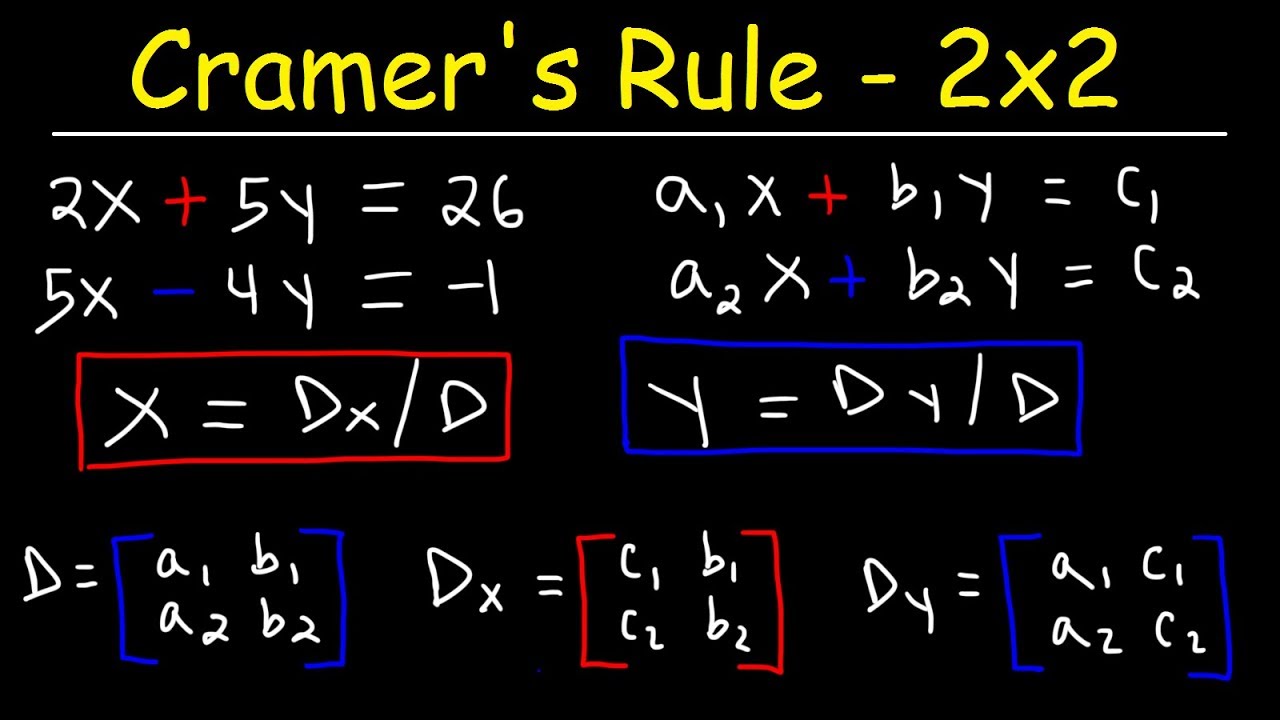
🔢 For a two-variable system, the value of x and y can be calculated using determinants.
🎯 The determinant of a 2x2 matrix (d) is found by multiplying the diagonal elements and subtracting the product of the off-diagonal elements.
📐 To find dx and dy, replace the coefficients of x and y in the matrix with the constants from the equations and calculate the determinants.
🌟 The values of x and y are found by dividing dx and dy by the determinant d.
📈 The script provides an example of solving a two-variable system using Kramer's Rule, resulting in the solution (x=2, y=3).
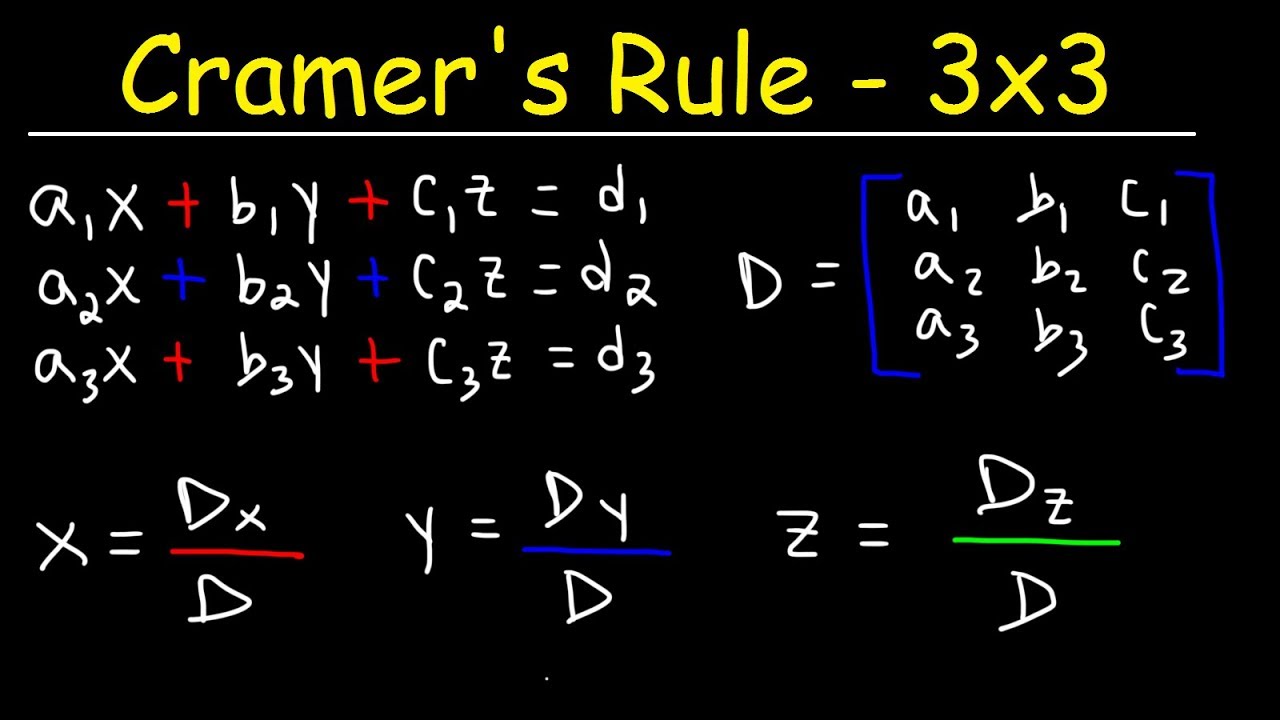
🔑 Kramer's Rule can also be applied to systems with three variables, involving a 3x3 matrix.
📊 For a three-variable system, the determinant d and the determinants for dx, dy, and dz are calculated using a similar process but with more complex matrices.
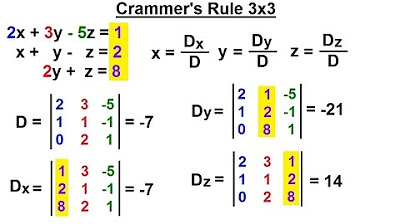
🌐 The script includes an example of solving a three-variable system, resulting in the solution (x=1, y=2, z=3).
🛠️ Kramer's Rule is a method that requires patience and careful calculations, as one mistake can invalidate the entire solution.
📖 Practice is essential to master Kramer's Rule and apply it efficiently to solve various systems of equations.

Q & A

What is Kramer's Rule and how is it used?
-Kramer's Rule is a method used to solve systems of linear equations with multiple variables. It is particularly useful for systems with two or three variables. The rule involves calculating the determinant of matrices formed by the coefficients of the variables and then using these determinants to find the values of the variables.
How do you calculate the determinant of a 2x2 matrix?
-The determinant of a 2x2 matrix is calculated by multiplying the diagonal elements and then subtracting the product of the off-diagonal elements. For a matrix [a1, b1; b2, a2], the determinant d is given by d = a1*a2 - b1*b2.
What are dx, dy, and the determinant d in the context of Kramer's Rule?
-In Kramer's Rule, dx, dy, and d are determinants used to find the solution of a system of linear equations. dx and dy are the determinants formed by replacing the coefficients of x and y in the original matrix with the constants from the equations. The determinant d is the determinant of the original matrix formed by the coefficients of the variables.
How do you find the values of x and y using Kramer's Rule?
-Once you have calculated the determinants dx, dy, and d, you can find the values of x and y by dividing dx and dy by d. The values are given by x = dx/d and y = dy/d. If the determinant d is zero, the system may be dependent or inconsistent, and a unique solution may not exist.
What is the process for solving a system of equations with three variables using Kramer's Rule?
-To solve a system with three variables using Kramer's Rule, you first calculate the determinant d of the 3x3 matrix formed by the coefficients of the variables. Then, you calculate the determinants dx, dy, and dz by replacing the coefficients of x, y, and z with the constants from the equations, respectively. The values of the variables x, y, and z are then found by dividing the corresponding determinants by d (x = dx/d, y = dy/d, z = dz/d).
How do you verify the solution of a system of equations?
-To verify the solution, you plug the calculated values of the variables back into the original equations and check if both sides of each equation are equal. If they are, then the solution is correct.
What are some alternative methods to Kramer's Rule for solving systems of linear equations?
-Alternative methods to Kramer's Rule include the substitution method and the elimination method. These methods can be simpler and quicker to use, especially for smaller systems of equations.
Why might a teacher require the use of Kramer's Rule over other methods?
-A teacher might require the use of Kramer's Rule to ensure that students understand the concept of determinants and how they can be applied to solve systems of linear equations. It also provides practice in handling more complex systems with multiple variables.
What is the significance of the determinant d being zero in the context of the system of equations?
-If the determinant d is zero, it suggests that the system of equations may not have a unique solution. The system could be dependent (infinitely many solutions) or inconsistent (no solution). Further analysis is needed to determine the nature of the system.
How does the process of calculating the determinant for a 3x3 matrix differ from that of a 2x2 matrix?
-Calculating the determinant for a 3x3 matrix is more complex than for a 2x2 matrix. For a 3x3 matrix, you break down the larger matrix into three smaller 2x2 matrices, calculate their determinants, and then combine these results following a specific pattern that involves adding and subtracting the products of the elements along the main diagonals.
What are the steps involved in calculating the determinant of a 3x3 matrix?
-To calculate the determinant of a 3x3 matrix, you first eliminate one row and one column from the matrix, then calculate the determinants of the three resulting 2x2 matrices. You then combine these determinants by multiplying the elements of the eliminated row and column by the determinants of the 2x2 matrices and summing them up according to the pattern of addition and subtraction along the main diagonals.
How does Kramer's Rule help in understanding the relationship between the coefficients and constants in a system of equations?
-Kramer's Rule emphasizes the relationship between the coefficients of the variables and the constants in a system of equations by requiring you to form matrices with these elements. The process of calculating the determinants dx, dy, dz, and d highlights how these elements interact to produce the solution to the system, enhancing the understanding of the underlying structure of the equations.

Outlines

00:00

📚 Introduction to Kramer's Rule

This paragraph introduces Kramer's Rule, a method for solving systems of linear equations with multiple variables. It explains the basic concept of applying the rule to systems with two and three variables, and sets up the foundation for understanding how to calculate the determinant of a matrix, which is crucial for this method. The paragraph outlines the process of finding the values of x and y in a two-variable system using determinants.

05:01

🧮 Calculation of Determinants and Coefficients

This paragraph delves into the specifics of calculating determinants for 2x2 matrices and how they are used to find the coefficients dx, dy, and d. It provides a step-by-step walkthrough of the process, including replacing coefficients with constants from the equations and computing the determinant to find the values of x and y. The paragraph also presents an example to illustrate the calculation, reinforcing the method with practical application.

10:05

🔢 Application of Kramer's Rule to a Two-Variable System

This paragraph demonstrates the application of Kramer's Rule to a practical example involving a two-variable system. It shows how to calculate the determinant (d), dx, and dy using the coefficients from the given equations. The paragraph then uses these values to solve for x and y, verifying the solution by plugging the values back into the original equations. This example serves to clarify the process and validate the method for solving systems of equations.

15:06

📈 Extending Kramer's Rule to Three-Variable Systems

The paragraph discusses the extension of Kramer's Rule to systems involving three variables. It explains the setup for calculating the determinant (d) for a 3x3 matrix and how to find the coefficients dx, dy, and dz. The explanation includes the process of replacing the coefficients of each variable with the constants from the equations and setting up the appropriate matrices for calculation. This section lays the groundwork for solving more complex systems involving three variables.

20:06

📊 Detailed Calculation for a Three-Variable System

This paragraph provides a detailed example of applying Kramer's Rule to a three-variable system. It outlines the steps for calculating the determinant (d), dx, dy, and dz for the given system of equations. The paragraph breaks down the process of eliminating variables and calculating the determinants for the smaller 2x2 matrices, leading to the final values for x, y, and z. The comprehensive explanation ensures a clear understanding of the method for solving three-variable systems.

25:07

🎓 Verification of the Solution

The paragraph concludes the application of Kramer's Rule by verifying the calculated values of x, y, and z. It demonstrates how to plug these values back into the original equations to ensure they satisfy all three equations in the system. The verification process confirms the accuracy of the solution and reinforces the reliability of Kramer's Rule for solving systems of linear equations with multiple variables.

Mindmap

Keywords

💡Kramer's Rule

Kramer's Rule is a method used to solve systems of linear equations with multiple variables. It is particularly useful when dealing with systems where the number of equations equals the number of variables. The rule involves calculating the determinant of matrices to find the values of the variables. In the video, Kramer's Rule is applied to both two-variable and three-variable systems, demonstrating how to find the determinant and use it to solve for the unknowns.

💡System of Equations

A system of equations refers to a collection of multiple equations that need to be solved simultaneously. Each equation represents a relationship between different variables, and finding the solution to the system involves determining the values of these variables that satisfy all equations at once. In the context of the video, the focus is on linear systems with two and three variables, which are solved using Kramer's Rule.

💡Determinant

The determinant is a scalar value that can be computed from the elements of a square matrix and is used in various areas of mathematics, including linear algebra. In the context of solving systems of linear equations, the determinant helps in finding the inverse of a matrix and is used in Kramer's Rule to calculate the values of the variables. A determinant of zero indicates that the system of equations may be dependent, meaning there are infinitely many solutions or no solution at all.

💡Two-Variable System

A two-variable system consists of two linear equations with two unknowns, typically denoted as x and y. Solving such a system involves finding the values of x and y that satisfy both equations simultaneously. The video demonstrates the use of Kramer's Rule to solve these systems by calculating the determinant and the values of dx, dy, and d, which are used to find the values of x and y.

💡Three-Variable System

A three-variable system is a set of three linear equations with three unknowns, typically denoted as x, y, and z. Solving this system requires finding the values of x, y, and z that satisfy all three equations at the same time. The video shows how to apply Kramer's Rule to such systems by setting up and calculating the determinants for d, dx, dy, and dz, which are then used to find the values of the three variables.

💡Matrix

A matrix is a rectangular array of numbers, symbols, or expressions, arranged in rows and columns. In the context of the video, matrices are used to represent systems of linear equations and to calculate determinants, which are essential for applying Kramer's Rule. The coefficients of the variables in the system of equations form the matrix, and the constants are used to form the determinants for dx, dy, and dz.

💡Coefficients

Coefficients are the numerical factors multiplying the variables in a linear equation. They determine the slope or the rate of change in the relationship between the variables. In the video, the coefficients are the numbers in front of the variables x, y, and z in the given system of equations. These coefficients are used to form the matrices and calculate the determinants necessary for solving the system using Kramer's Rule.

💡Variables

Variables are symbols, often letters, that represent unknown quantities in an equation or a system of equations. They are the values that the system is trying to find. In the video, the variables discussed are x, y, and z, which are used in both two-variable and three-variable systems of linear equations.

💡Equilibrium

In the context of a system of equations, equilibrium refers to the set of values for the variables that satisfy all equations simultaneously. These values represent the state where the relationships defined by the equations are in balance. The video's main goal is to find this equilibrium for both two-variable and three-variable systems using Kramer's Rule.

💡Substitution Method

The substitution method is a technique used to solve systems of linear equations by expressing one variable in terms of another from one equation and then substituting it into the other equation(s) to find the solution. While the video focuses on Kramer's Rule, it mentions that the substitution method is another approach to solving systems of equations, which may be preferred or required in certain situations.

💡Elimination Method

The elimination method is a technique for solving systems of linear equations by adding or subtracting equations to eliminate one variable, making it easier to solve for the remaining variables. Like the substitution method, the elimination method is an alternative to Kramer's Rule and is mentioned in the video as another way to solve systems of equations. This method is particularly useful when the coefficients of a variable in one or more equations are the same or negatives of each other.

Highlights

Introduction to Kramer's Rule for solving systems of equations with two and three variables.

Explanation of how to calculate the value of x and y using a two-variable system.

Method for finding the determinant of a two by two matrix using the formula a1*b2 - a2*b1.

Procedure for calculating dx and dy by replacing the coefficients of x and y with the constants from the equations.

Illustration of how to apply Kramer's Rule through the solution of a practical two-variable system example.

Verification of the solution by plugging the values of x and y back into the original equations.

Introduction to the application of Kramer's Rule for a three-variable system.

Explanation of setting up the determinant for a three by three matrix with coefficients of x, y, and z.

Breakdown of how to calculate the determinant of a three by three matrix using smaller two by two matrices.

Example of solving a three-variable system by finding d, dx, dy, and dz, and the method for checking the solution.

Discussion on the practicality and patience required when using Kramer's Rule as opposed to other methods like substitution or elimination.

Emphasis on the importance of accuracy and attention to detail when performing the calculations in Kramer's Rule.

Encouragement for practice and mastery of Kramer's Rule for educational purposes, despite the availability of other solving techniques.

Transcripts

Browse More Related Video

Cramer's Rule - 3x3 Linear System

Cramer's Rule - 2x2 Linear System

Cramer's Rule Explained

PreCalculus - Matrices & Matrix Applications (33 of 33) Using Cramer's Rule to Find x=? y=? z=?

PreCalculus - Matrices & Matrix Applications (30 of 33) Find the Determinant of a 3x3 Matrix 1

7.1.1 Solving a System of Equations Using Substitution

Related Tags

Kramer's Rule Linear Equations Mathematics Tutorial Educational Video Variable Systems Matrix Determinant Equation Solving Math Techniques Substitution Method Elimination Method