Related Rates Cone Example (Calculus)

Mario's Math Tutoring

25 Jul 201605:30

EducationalLearning

32 Likes 10 Comments

TLDRIn this informative video, Mario from Mario's Math Tutoring explains how to tackle a related rate problem involving a conical tank filling up with water. He guides viewers through drawing a diagram, identifying known variables, and forming equations using geometry and calculus. Mario demonstrates the step-by-step process of solving for the rate of change of water depth, ultimately providing a clear solution and encouraging viewers to explore more related rate problems for a deeper understanding.

Takeaways

📚 The video is a tutorial on solving a related rate problem involving a cone.
🎓 Mario's Math provides a step-by-step approach to tackle calculus problems.
📈 The problem involves a conical tank filling up with water at a rate of 10 ft³/min.
📐 The tank is 6 feet across the top and the water level is 3 feet deep when the rate is to be determined.
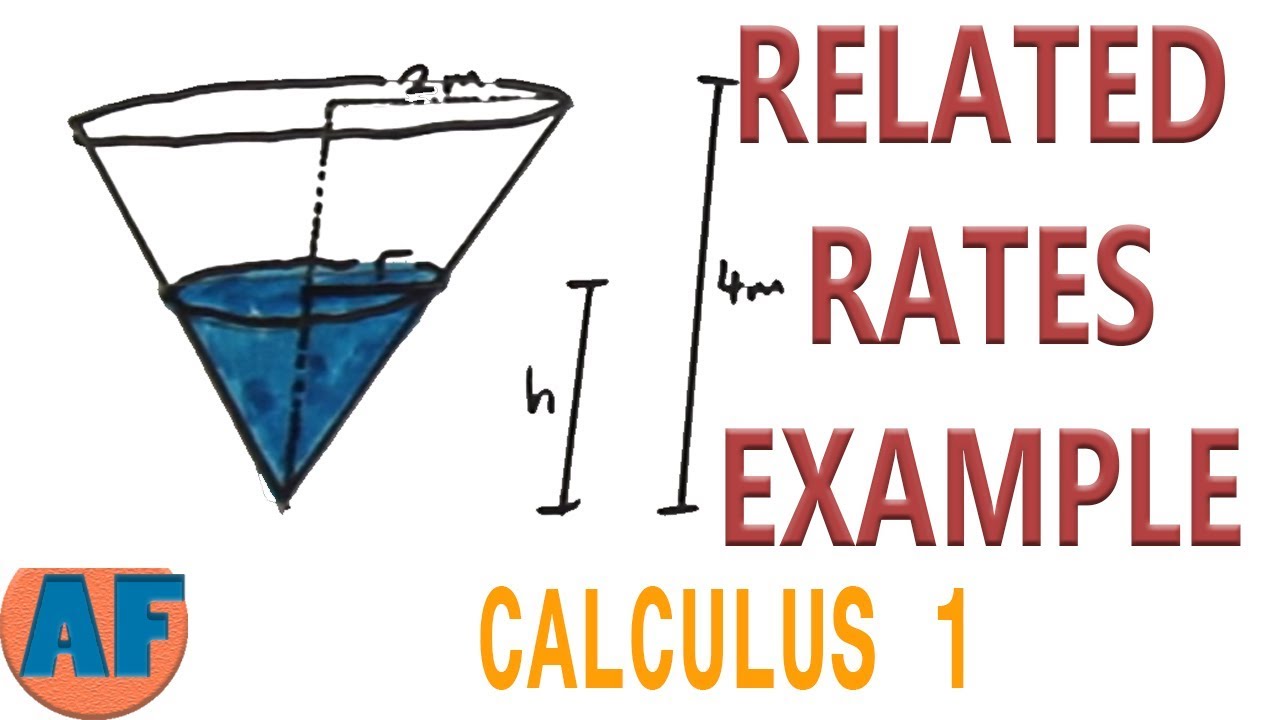
🖼️ Step one is to draw a diagram to visualize the problem and understand the geometry involved.
🔢 Identify known values and the unknown value (dh/dt when h = 3 feet).
📐 Use the properties of similar triangles to establish a relationship between the radius and height of the water in the tank.
📝 Formulate an equation for the volume of the cone using the volume formula (1/3)πr²h.
💡 Simplify the volume equation to express it in terms of H only, using the similar triangles ratio.
📶 Perform implicit differentiation with respect to time (t) to find the rate of change of height (dh/dt).
🔢 Solve for the unknown rate (dh/dt) when the height is 3 feet using the given volume rate.
🎉 The final answer is the rate of change of the height with respect to time, expressed in feet per minute.

Q & A

What is the main topic of the video?
-The main topic of the video is solving a related rate problem involving a conical tank filling up with water.
What are the dimensions of the conical tank mentioned in the video?
-The conical tank is 6 feet across the top and 4 feet deep.
What is the rate at which water is flowing into the tank?
-Water is flowing into the tank at a rate of 10 cubic feet per minute.
What specific rate is being sought in the problem?
-The problem seeks to find the rate of change of the depth of the water (dh/dt) when the water is 3 feet deep.
How does Mario suggest approaching related rate problems initially?
-Mario suggests initially drawing a diagram to visualize the problem and understand the given information and what needs to be found.
What is the relationship between the two triangles formed in the conical tank?
-The two triangles formed in the conical tank are similar due to the angle-angle similarity from geometry, as they share the same angles and have right angles.
What is the formula for the volume of a cone?
-The formula for the volume of a cone is (1/3)πr^2h, where r is the radius and h is the height.
How does Mario relate the similar triangles to find an equation for the volume of the cone?
-Mario sets up a proportion using the similar triangles (3 is to 2R as 4 is to 2h), which leads to the equation 3H = 4R. He then substitutes R = (3H/4) into the volume formula to get a volume equation in terms of H only.
What is the derivative taken with respect to time in this problem?
-The derivative taken with respect to time is dV/dt, which represents the rate of change of the volume of the tank as water flows in.
What is the final formula derived for the rate of change of the height of the water?
-The final formula derived for the rate of change of the height of the water is (9π/16)H^2(dH/dt) = dV/dt, where H is the height of the water and dV/dt is the known rate of change of volume.
What is the final answer for the rate of change of the height of the water when the water is 3 feet deep?
-When the water is 3 feet deep, the rate of change of the height (dH/dt) is (160/81π) feet per minute.
What advice does Mario give for understanding related rate problems better?
-Mario advises watching other videos on related topics, such as the ladder problem, to gain a better understanding of how to work through related rate problems.

Outlines

00:00

📚 Introduction to Related Rates Problem

This paragraph introduces Mario's math tutoring video focused on solving a related rates problem involving a conical tank. Mario explains that the problem involves a tank with a vertex down, 6 feet across at the top, and water is flowing into it at a rate of 10 cubic feet per minute. The goal is to find the rate of change of the water's depth when it is 3 feet deep. Mario emphasizes the importance of drawing a diagram to visualize the problem and outlines the steps needed to solve it, including identifying known and unknown variables and forming equations based on the geometry of the cone and similar triangles.

05:02

🧮 Solving the Related Rates Problem

In this paragraph, Mario dives into the solution of the related rates problem. He starts by setting up an equation for the volume of the cone using the given dimensions and the rate of water flow. Mario then uses the concept of similar triangles to relate the radius and height of the cone, which allows him to express the volume in terms of the height alone. He proceeds to differentiate this volume equation with respect to time to find the rate of change of the height (dh/dt). After substituting the given rate of volume change and the height of 3 feet, Mario simplifies the equation to find the exact rate at which the height of the water is increasing, providing a clear and detailed explanation of each step in the process.

Mindmap

Keywords

💡Related rate problem

A related rate problem is a type of mathematical challenge that involves finding the rate of change of one quantity with respect to another, given certain conditions. In the context of the video, it refers to calculating the rate at which the depth of water in a conical tank changes over time as water flows into it. The problem is set within a real-world scenario of a tank filling up, which makes it relatable and practical.

💡Cone

A cone is a three-dimensional geometric shape that tapers smoothly from a flat base, called the base radius, to a single point called the vertex. In the video, the conical tank is described with a vertex pointing downwards and a base radius of 6 feet, which is crucial for calculating the volume and subsequently the rate of change of water depth.

💡Volume

Volume refers to the amount of space occupied by a three-dimensional object. In mathematics, it is often calculated using formulas specific to the shape of the object. For a cone, the volume is given by the formula (1/3)πr²h, where r is the radius of the base and h is the height. In the video, the volume of the conical tank is central to determining the rate of change of the water depth.

💡

A diagram is a visual representation or sketch used to illustrate the key components and relationships in a problem. In the video, drawing a diagram of the conical tank helps to visualize the geometry involved and to identify the similar triangles that are crucial for setting up the related rate problem.

💡Similar triangles

Similar triangles are two triangles that have the same shape but not necessarily the same size, with their corresponding angles being equal and the sides being in proportion. In the video, Mario identifies two similar triangles formed within the conical tank to establish a proportion that relates the radius of the base to the height of the water.

💡Equation

An equation is a mathematical statement that asserts the equality of two expressions. In the context of the video, Mario sets up an equation that relates the known volume change rate to the unknown rate of change of the water's depth. This equation is essential for solving the related rate problem.

💡Implicit differentiation

Implicit differentiation is a method used in calculus to differentiate an equation with respect to a variable when the relationship between the variables is not explicitly given in terms of one variable. In the video, Mario uses implicit differentiation to find the derivative of the volume with respect to time, which helps to determine the rate of change of the water's depth.

💡Derivative

A derivative in calculus represents the rate of change of a function with respect to its independent variable. It provides information about the function's slope or how it changes at a given point. In the video, the derivative is used to find the rate of change of the water's depth in the conical tank over time.

💡Rate of change

The rate of change is a measure of how quickly a quantity changes with respect to another quantity. It is often represented as the derivative of a function and is used to analyze the behavior of functions and their rates of growth or decay. In the video, the rate of change is central to finding out how fast the water depth is increasing as the tank fills up.

💡Geometry

Geometry is a branch of mathematics concerned with the properties and relations of points, lines, surfaces, and solids. In the video, geometry is used to analyze the conical tank and the triangles formed within it, which is essential for setting up and solving the related rate problem.

💡Calculus

Calculus is a branch of mathematics that deals with rates of change and accumulation. It is used to study the behavior of functions and their derivatives, which can be applied to a wide range of problems, including those involving physical and geometric quantities. In the video, calculus is the mathematical tool used to solve the related rate problem involving the filling of a conical tank.

Highlights

Introduction to a related rate problem involving a cone.

The problem involves a conical tank with a vertex down, 6 feet across at the top, and 4 feet deep.

Water is flowing into the tank at a rate of 10 cubic feet per minute.

The goal is to find the rate of change of the water's depth when it is 3 feet deep.

The first step in solving the problem is to draw a diagram of the cone.

Identify known information and what is being sought, such as the change in volume (DV/DT) and the change in depth (dh/dt).

Use the given information to establish a relationship between the radius and height of the water in the tank using similar triangles.

Develop an equation for the volume of the cone using the relationship between the radius, height, and volume.

Perform implicit differentiation to find the derivative of the volume with respect to time.

Simplify the derivative to express the change in height (dh/dt) in terms of the given volume rate (DV/DT).

Substitute the known volume rate and the height of the water to solve for the rate of change of height.

The final result gives the rate of increase in height of the water in the tank as it fills.

The solution involves positive values indicating the water level is rising.

The final answer is given in feet per minute, reflecting the units of the problem.

The video also mentions other related rate problems, such as the ladder problem, for further understanding.

The video aims to help viewers better understand how to work with related rates and apply the concepts to various problems.

The presenter encourages viewers to subscribe to the channel for more educational content.

Transcripts

Browse More Related Video

Step by Step Method of Solving Related Rates Problems - Conical Example

Calculus AB Homework 4.2: Related Rates

Related Rates Cone Example (Calculus)

Takeaways

Q & A

What is the main topic of the video?

What are the dimensions of the conical tank mentioned in the video?

What is the rate at which water is flowing into the tank?

What specific rate is being sought in the problem?

How does Mario suggest approaching related rate problems initially?

What is the relationship between the two triangles formed in the conical tank?

What is the formula for the volume of a cone?

How does Mario relate the similar triangles to find an equation for the volume of the cone?

What is the derivative taken with respect to time in this problem?

What is the final formula derived for the rate of change of the height of the water?

What is the final answer for the rate of change of the height of the water when the water is 3 feet deep?

What advice does Mario give for understanding related rate problems better?