13 - Instantaneous Acceleration Explained (Average Vs. Instantaneous Acceleration)

Math and Science
30 Oct 201817:49
EducationalLearning
32 Likes 10 Comments

TLDRIn this lesson, the concept of instantaneous acceleration is explored, drawing parallels with instantaneous velocity. The discussion revolves around the idea that acceleration is the rate of change of velocity, just as velocity is the rate of change of position. By examining the slope of the tangent to the velocity curve at any given point, one can determine the instantaneous acceleration. The lesson emphasizes understanding the physical intuition behind calculus concepts and applies these ideas to analyze the motion depicted in a velocity versus time graph, highlighting how the steepest slope indicates the greatest acceleration.

Takeaways
  • πŸ“ˆ Instantaneous acceleration is the slope of the line tangent to the velocity curve at a specific point.
  • πŸ”„ The concept of instantaneous acceleration mirrors that of instantaneous velocity, with the slope representing the rate of change.
  • πŸ“Œ To find the instantaneous velocity, a line tangent to the position curve at a point is used, and similarly, for acceleration, a tangent to the velocity curve is used.
  • πŸ€” The process of finding the slope of a tangent line involves taking two points on the curve and calculating the change in position (Ξ”x) over the change in time (Ξ”t).
  • πŸ“‰ When the slope is positive, it indicates that the acceleration is in the positive direction, meaning the speed is increasing.
  • πŸ“Š A zero slope on the velocity curve indicates zero acceleration, which means the speed of the object is not changing at that instant.
  • πŸ“ˆπŸ“‰ The steepest part of the velocity curve will have the highest absolute value of acceleration, indicating the fastest change in speed.
  • πŸ”„ The process of finding the slope of a tangent line is analogous to the concept of a derivative in calculus.
  • πŸš€ Understanding the relationship between position, velocity, and acceleration is crucial for solving problems in physics involving motion.
  • πŸ“š The concepts discussed are fundamental to the study of kinematics and dynamics in physics.
Q & A

  • What is instantaneous acceleration?

    -Instantaneous acceleration is the rate of change of velocity at a specific point in time. It is the slope of the line tangent to the velocity curve at a particular point and is measured in meters per second squared (m/s^2).

  • How is instantaneous acceleration related to instantaneous velocity?

    -Instantaneous velocity is the slope of the line tangent to the position curve at a specific point, while instantaneous acceleration is the slope of the line tangent to the velocity curve at a specific point. Both are concepts that describe the motion of an object at a particular instant in time, with velocity focusing on speed and direction, and acceleration focusing on the change in velocity.

  • What is the mathematical representation of instantaneous acceleration?

    -The mathematical representation of instantaneous acceleration is given by the limit of the change in velocity (Δv) divided by the change in time (Δt) as Δt approaches zero: a = lim(Δt→0) (Δv/Δt).

  • How do you determine the sign of acceleration on a velocity curve?

    -The sign of acceleration on a velocity curve is determined by the direction of the slope of the tangent line at a given point. A positive slope indicates positive acceleration (speeding up), while a negative slope indicates negative acceleration (slowing down).

  • What happens at the point of maximum speed on a velocity curve?

    -At the point of maximum speed on a velocity curve, the acceleration is zero because there is no change in speed at that instant. The object is still moving at its fastest, but it is no longer increasing its speed.

  • How can you estimate the instantaneous acceleration at a point on a velocity curve?

    -You can estimate the instantaneous acceleration at a point on a velocity curve by finding the slope of the line segment between two points on the curve and then repeatedly selecting closer points to the desired point, calculating the slope for each segment, and taking the limit as the distance between the points approaches zero.

  • What is the relationship between the slope of a curve and the physical quantities in motion?

    -The slope of a displacement curve (position vs. time) represents velocity, and the slope of a velocity curve (velocity vs. time) represents acceleration. This relationship is fundamental in kinematics and is used to analyze and predict the motion of objects.

  • What are the units for instantaneous acceleration?

    -The units for instantaneous acceleration are meters per second squared (m/s^2), indicating the rate of change of velocity per unit of time.

  • How does the steepness of the tangent line on a velocity curve relate to the magnitude of acceleration?

    -The steepness of the tangent line on a velocity curve is directly related to the magnitude of acceleration. A steeper tangent line indicates a larger rate of change of velocity, meaning a larger acceleration, while a shallower tangent line indicates a smaller acceleration.

  • What is the significance of the derivative in calculus in relation to motion?

    -The derivative in calculus is used to find the instantaneous rate of change of a function, such as velocity or position, with respect to another variable, like time. In the context of motion, the derivative of the position function gives the velocity, and the derivative of the velocity function gives the acceleration.

  • How can you compare the magnitude of acceleration at different points on a velocity curve?

    -To compare the magnitude of acceleration at different points on a velocity curve, you look at the steepness of the tangent lines at those points. The steeper the tangent line, the greater the magnitude of acceleration, regardless of whether it is positive or negative.

Outlines
00:00
πŸ“ˆ Introduction to Instantaneous Acceleration and Velocity

This paragraph introduces the concept of instantaneous acceleration, drawing parallels to the previously discussed concept of instantaneous velocity. The speaker explains that just as velocity is the slope of the tangent line to the position curve at a specific point, acceleration is the slope of the tangent line to the velocity curve. The speaker uses a hypothetical parabolic position curve to illustrate the process of finding the instantaneous velocity at a point by drawing a tangent line and discussing the mathematical representation of this concept.

05:01
πŸ“Š Derivation of Instantaneous Acceleration

In this paragraph, the speaker delves deeper into the concept of instantaneous acceleration by explaining how it is derived from the velocity curve. The process involves estimating the slope of the tangent line at a point by finding the difference in velocity (Ξ”v) over the difference in time (Ξ”T). The speaker emphasizes the importance of taking the limit as Ξ”T approaches zero to find the instantaneous acceleration, which is analogous to the process used in calculus to find derivatives. This paragraph also establishes the connection between the slope of the velocity curve and the acceleration, highlighting that the direction of the slope indicates whether the acceleration is positive or negative.

10:01
πŸ” Analyzing the Sign and Magnitude of Acceleration

The speaker continues the discussion on acceleration by examining the sign and magnitude of acceleration at different points on a velocity curve. The paragraph focuses on understanding the physical meaning behind positive and negative accelerations, using a detailed analysis of a velocity versus time graph with labeled points A, B, C, and D. The speaker explains that the sign of the acceleration (positive or negative) indicates the direction of the change in velocity, while the magnitude represents how quickly the velocity is changing. The speaker also provides a visual representation of the acceleration at each point, helping to intuitively understand the concept.

15:02
🌟 Understanding Acceleration through Velocity and Displacement

In the final paragraph, the speaker wraps up the lesson by reinforcing the fundamental concepts of displacement, velocity, and acceleration. The speaker emphasizes that the slope of the tangent line to the displacement curve represents velocity, while the slope of the tangent line to the velocity curve represents acceleration. The speaker encourages the audience to focus on these intuitive concepts rather than just the mathematical formulas. The paragraph concludes with a brief mention of solving problems related to motion, setting the stage for further exploration of physics and the equations of motion.

Mindmap
Keywords
πŸ’‘Instantaneous Acceleration
Instantaneous acceleration refers to the rate of change of velocity at a specific moment in time. It is the slope of the line tangent to the velocity curve at a particular point and is used to determine how quickly the speed of an object is changing at that instant. In the context of the video, this concept is crucial for understanding the dynamics of motion and is analogous to the previously discussed concept of instantaneous velocity.
πŸ’‘Position Curve
A position curve is a graphical representation of an object's position as a function of time. It plots the position of the object on one axis (usually the vertical axis) against time on the other axis (usually the horizontal axis). The shape of the curve provides information about the object's motion, such as its speed and direction. In the video, the position curve is used to introduce the concept of instantaneous velocity and acceleration.
πŸ’‘Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. The slope of the tangent line at any point on a curve represents the instantaneous rate of change of the function at that point. In the context of the video, tangent lines are used to find the instantaneous velocity and acceleration by determining their slopes.
πŸ’‘Slope
The slope of a line is a measure of its steepness, indicating the rate of change between the variables represented on the two axes. In the context of the video, the slope is used to describe the rate of change of position (resulting in velocity) and the rate of change of velocity (resulting in acceleration).
πŸ’‘Derivative
In calculus, the derivative is a fundamental concept that represents the rate of change of a function with respect to its independent variable. It is the mathematical operation that finds the slope of the tangent line to a curve at any given point. The derivative is used in physics to describe how a quantity changes as another quantity varies, such as velocity changing with time in the case of motion.
πŸ’‘Limit
In mathematics, a limit is the value that a function or sequence approaches as the input (or index) approaches some value. In the context of the video, the limit is used to define instantaneous velocity and acceleration as the time interval approaches zero, which gives the precise rate of change at a specific instant.
πŸ’‘Velocity Curve
A velocity curve is a graphical representation of an object's velocity as a function of time. It plots the velocity of the object on one axis against time on the other axis. The shape of the curve indicates how the object's speed changes over time, including whether it is speeding up, slowing down, or maintaining a constant speed.
πŸ’‘Acceleration
Acceleration is a physical quantity that describes how quickly an object's velocity changes. It is equal to the rate of change of velocity with respect to time. Positive acceleration indicates an increase in speed, while negative acceleration (or deceleration) indicates a decrease in speed.
πŸ’‘Meters per Second (m/s)
Meters per second (m/s) is the unit of speed in the International System of Units (SI), indicating the distance an object travels in one second. It is commonly used in physics to describe the velocity of an object.
πŸ’‘Meters per Second Squared (m/s^2)
Meters per second squared (m/s^2) is the unit of acceleration in the International System of Units (SI), representing the change in speed per second. It describes how quickly an object's velocity changes, with positive values indicating an increase in speed and negative values indicating a decrease in speed.
Highlights

Instantaneous acceleration is closely related to instantaneous velocity, both being fundamental concepts in understanding motion.

The concept of instantaneous acceleration is introduced as the slope of the line tangent to the position curve at a specific point.

A position curve is a plot of how far an object is from the origin in a single direction (X) as a function of time.

To find the instantaneous velocity at a point, one draws a tangent line to the position curve at that point, with the slope of this tangent line representing the velocity.

Mathematically, instantaneous velocity at a point is defined using the limit as the time interval approaches zero, represented as the ratio of change in position (Ξ”x) to change in time (Ξ”T).

A velocity curve is a plot of velocity versus time, which can be used to analyze changes in an object's speed over time.

Instantaneous acceleration is the slope of the line tangent to the velocity curve at a specific point, indicating how quickly the velocity is changing.

The formula for instantaneous acceleration involves the limit as Ξ”T approaches zero, which is analogous to the formula for instantaneous velocity.

In the context of calculus, the process of finding the slope of the tangent line to a curve is known as finding the derivative.

The direction of the slope (positive or negative) in the velocity curve indicates whether the acceleration is increasing or decreasing speed.

A horizontal tangent line on a velocity curve signifies zero acceleration, as it represents a moment when the speed is not changing.

A negative slope on the velocity curve indicates negative acceleration, or deceleration, where the speed of the object is decreasing.

The steepest slope on the velocity curve represents the highest acceleration, while the shallowest slope represents the lowest.

Comparing two negative accelerations involves looking at the absolute values to determine which is the greatest, indicating the fastest deceleration.

Understanding the relationship between position, velocity, and acceleration is crucial for solving problems in physics involving motion.

The concepts of instantaneous velocity and acceleration are not only theoretical but also have practical applications in analyzing real-world motion.

The lesson emphasizes the importance of visual analysis of curves to intuitively understand the motion of an object in terms of velocity and acceleration.

Transcripts

Browse More Related Video

Kinematics Graphs

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics

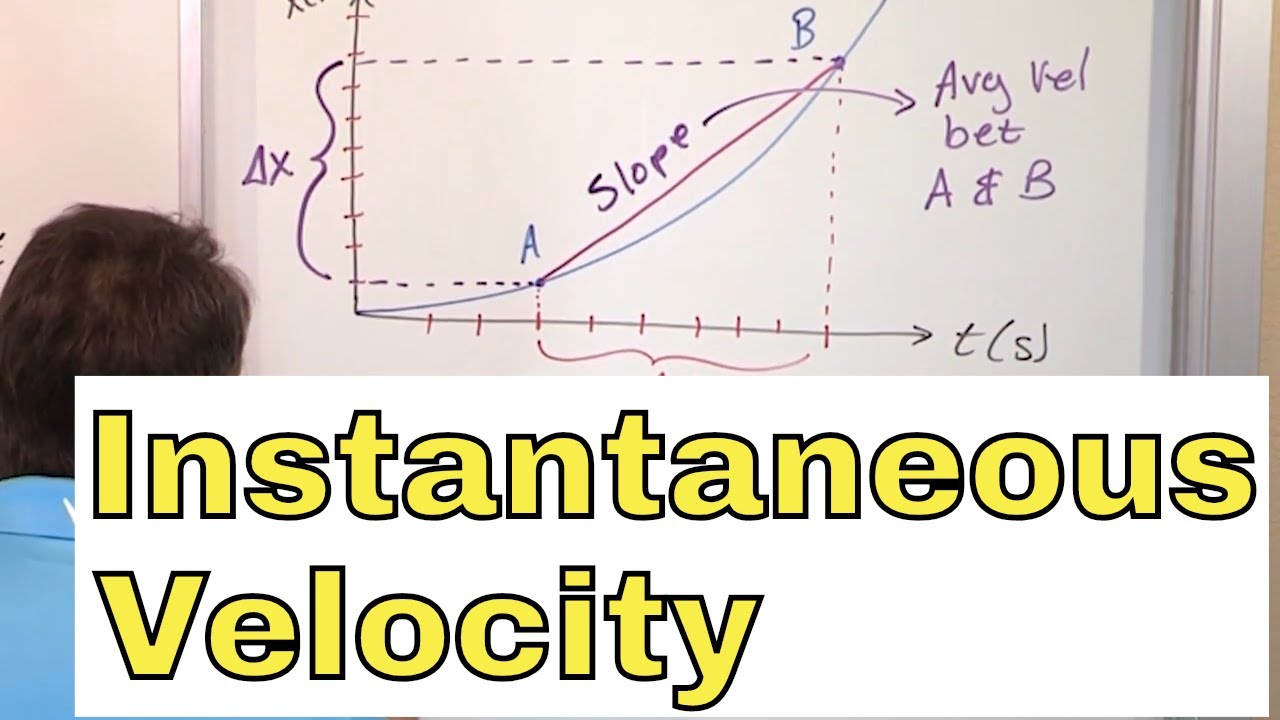
07 - What is Instantaneous Velocity?, Part 1 (Instantaneous Velocity Formula & Definition)

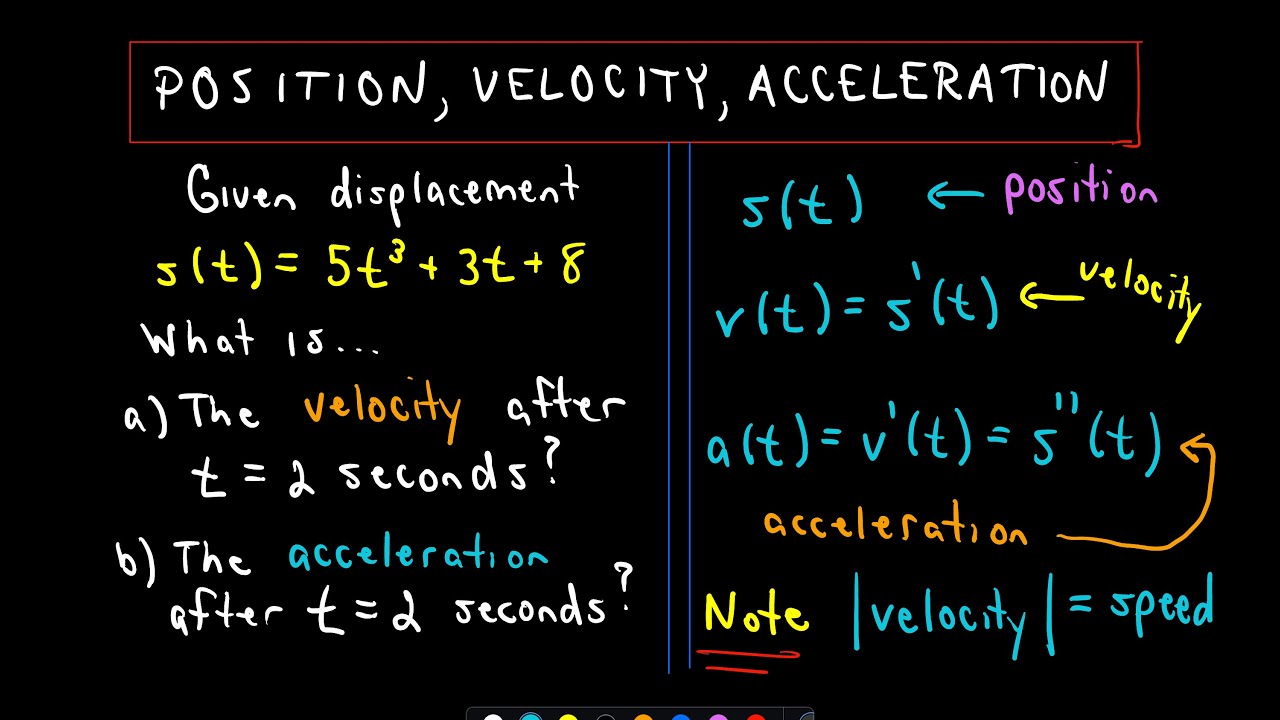
Position, Velocity, Acceleration using Derivatives

Motion Graphs - AP Physics 1: Kinematics Review Supplement

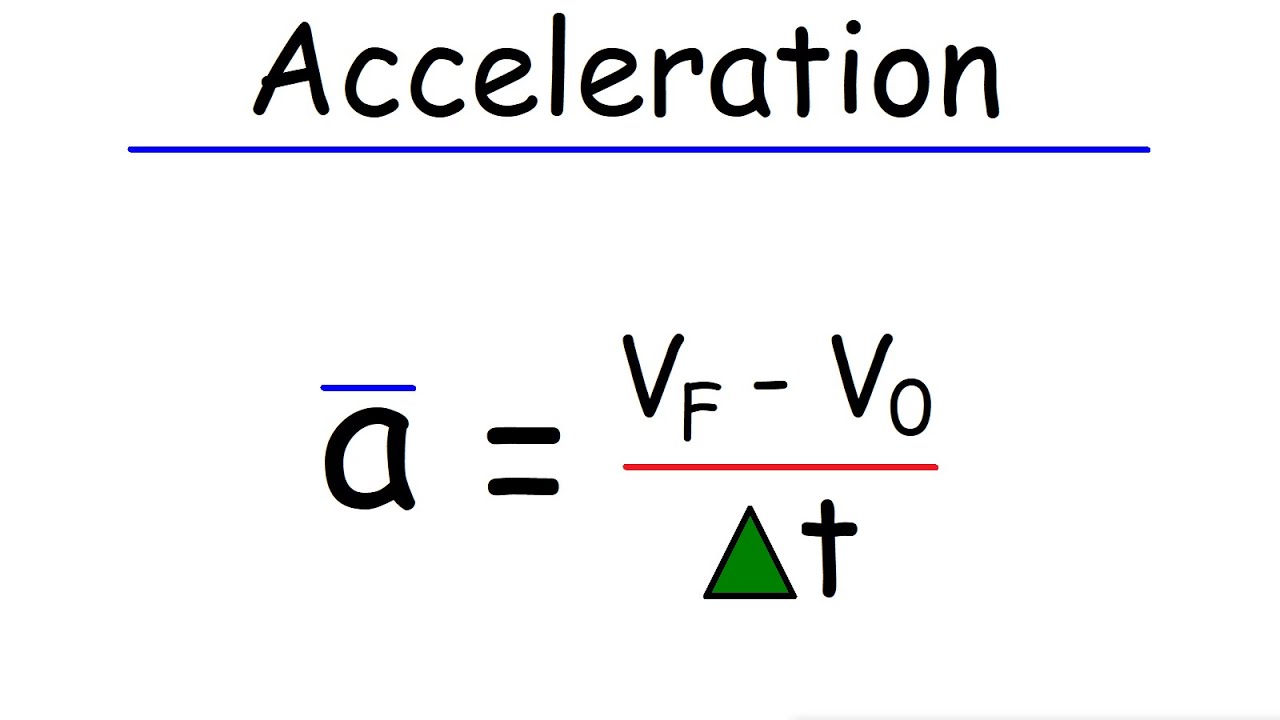
Average Acceleration and Instantaneous Acceleration

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsConceptsInstantaneousAccelerationVelocityAnalysisCalculusApplicationsMotionDynamicsSlopeInterpretationGraphInterpretationAccelerationSignificanceLearningPhysicsEducationalContent