GCSE Physics Revision "Acceleration"

Freesciencelessons
19 Feb 201805:52
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the concept of acceleration, explaining it as the change in velocity over time. It guides students through the process of calculating acceleration using the formula and provides examples involving a car and a cyclist to illustrate the concept. The script further explores velocity-time graphs, explaining how to determine acceleration from the graph's gradient and how to calculate distance traveled by examining the total area under the graph. The content is tailored to different student tiers, with advanced concepts for high-tier students involving non-constant acceleration and complex graph analysis.

Takeaways
  • 📚 Acceleration is the change in velocity of an object over a given time period.
  • 🔢 The formula for calculating acceleration is acceleration (m/s²) = (change in velocity (m/s)) / time (s).
  • 🚗 Example problem: A car accelerates from 50 m/s to 35 m/s in 20 seconds, resulting in an acceleration of 1 m/s².
  • 🚴‍♂️ Deceleration is represented by a negative acceleration, such as a cyclist slowing down from 6 m/s to 0 m/s in 12 seconds, which is -5 m/s².
  • 📈 Velocity-time graphs can be used to determine an object's acceleration by examining the gradient of the line.
  • 🏁 Horizontal lines on a velocity-time graph indicate constant velocity, while upward or downward slopes indicate acceleration or deceleration.
  • 🔄 To calculate the distance traveled from a velocity-time graph, the graph can be divided into shapes (triangles, rectangles) and the areas calculated.
  • 🔢 For non-constant acceleration, the total area under the graph is found by counting and estimating the area of complete and partial squares.
  • 📚 Understanding acceleration and its representation on velocity-time graphs is crucial for high-tier students.
  • 📈 The total area under a velocity-time graph represents the displacement in a specific direction.
  • 💡 Practice problems involving acceleration on velocity-time graphs can be found in the accompanying workbook.
Q & A

  • What is the definition of acceleration?

    -Acceleration is the change in velocity of an object over a given time period.

  • How is acceleration calculated?

    -Acceleration is calculated using the formula: acceleration (in meters per second squared) equals the change in velocity (in meters per second) divided by the time (in seconds).

  • What is the difference between velocity and acceleration?

    -Velocity is the speed of an object in a given direction, while acceleration refers to the change in velocity over time.

  • How do you calculate the acceleration of a car that changes velocity from 50 m/s to 35 m/s in 20 seconds?

    -The acceleration is calculated by (35 m/s - 50 m/s) / 20 s, which equals -1 m/s^2.

  • What does a negative acceleration value indicate?

    -A negative acceleration value indicates deceleration, meaning the object is slowing down.

  • What does the gradient of a velocity-time graph represent?

    -The gradient of a velocity-time graph represents the acceleration of the object. A horizontal line indicates constant velocity, an upward slope indicates acceleration, and a downward slope indicates deceleration.

  • How do you calculate the distance traveled by an object using a velocity-time graph?

    -The total area under the velocity-time graph represents the distance traveled. For constant acceleration or deceleration, the graph can be divided into shapes like triangles and rectangles, and the areas can be calculated and summed up.

  • In the example given, how do you calculate the distance traveled by a cyclist whose velocity decreases from 6 m/s to 0 m/s in 12 seconds?

    -The distance traveled is calculated by dividing the velocity-time graph into a triangle and a rectangle, finding the areas of these shapes, and adding them up to get the total distance.

  • What is the significance of the total area under a velocity-time graph in the context of motion?

    -The total area under a velocity-time graph represents the displacement, or the total distance traveled by the object in a specific direction.

  • How do you handle a velocity-time graph with non-constant acceleration or deceleration?

    -For a velocity-time graph with non-constant acceleration or deceleration, the total area under the graph is calculated by counting squares and estimating the areas of partial squares, then summing these areas to find the total distance or displacement.

  • What is the formula for calculating the area of a triangle in the context of a velocity-time graph?

    -The area of a triangle in a velocity-time graph is calculated using the formula: 0.5 * base * height, where the base is the difference in velocity and the height is the time interval.

  • How can one use the information from a velocity-time graph to solve problems in physics?

    -By understanding the relationship between the gradient of the graph and acceleration, as well as the area under the graph and displacement, one can solve a variety of problems in physics.

Outlines
00:00
📚 Introduction to Acceleration and Velocity Time Graphs

This paragraph introduces the concept of acceleration and its relationship with velocity. It explains that acceleration is the change in velocity over time, and provides the formula for calculating it: acceleration (in m/s^2) = (change in velocity in m/s) / time (in s). The paragraph also reviews the concept of velocity from a previous lesson, emphasizing that velocity is a vector quantity with both magnitude and direction. It presents a typical problem involving a car's acceleration and guides the audience to solve it by applying the formula. Additionally, it introduces the concept of deceleration and explains how to calculate an object's acceleration using a velocity-time graph. The paragraph concludes with an example of calculating acceleration from a graph and the meaning of the graph's gradient.

05:00
📐 Calculating Acceleration and Distance from Velocity-Time Graphs

This paragraph delves deeper into the application of velocity-time graphs for calculating both acceleration and distance traveled by an object. It explains how the gradient of a velocity-time graph represents the object's acceleration, with a horizontal line indicating constant velocity, an upward slope indicating acceleration, and a downward slope indicating deceleration. The paragraph provides a step-by-step guide on how to calculate acceleration from the graph by using the formula and subtracting initial and final velocities. It also introduces the concept of calculating distance traveled by examining the total area under the graph. The paragraph presents a method for calculating the area when acceleration or deceleration is not constant, instructing the audience to count squares under the graph and estimate the area of partial squares. The example given illustrates how to find the total distance or displacement by summing the areas of different geometric shapes formed under the graph. The paragraph concludes by mentioning additional resources for practice, directing the audience to a vision workbook available through a link.

Mindmap
Keywords
💡Acceleration
Acceleration is a physical quantity that describes the rate of change of velocity of an object over time. It is measured in meters per second squared (m/s^2). In the context of the video, acceleration is central to understanding how quickly an object changes its speed and direction. For example, the car's acceleration is calculated as the change in velocity (from 50 m/s to 35 m/s) divided by the time taken (20 seconds), resulting in an acceleration of 1 m/s^2.
💡Velocity
Velocity is a vector quantity that describes the speed of an object in a specific direction. It is measured in meters per second (m/s). In the video, velocity is used to describe the motion of objects like cars and cyclists, and understanding it is crucial for calculating acceleration and displacement. The video explains that the velocity of the car was 50 m/s north and it changed to 35 m/s north, indicating a change in velocity.
💡Change in Velocity
Change in velocity refers to the difference between an object's final velocity and its initial velocity. It is a key component in calculating acceleration, as it represents how much the velocity has changed over a given time period. In the video, the change in velocity is used to determine the acceleration of the car and the cyclist.
💡Time
Time is the duration over which an event occurs or a process takes place. In the context of the video, time is a crucial factor in calculating acceleration, as it is one of the components in the acceleration equation (change in velocity divided by time). The video provides examples of calculating acceleration over specific time intervals, such as 20 seconds for the car and 12 seconds for the cyclist.
💡Deceleration
Deceleration is the rate at which an object's velocity decreases over time. It is a type of acceleration with a negative value, indicating that the object is slowing down. In the video, deceleration is exemplified by the cyclist who reduces their velocity to zero over a period of 12 seconds, resulting in a negative acceleration.
💡Velocity-Time Graph
A velocity-time graph is a graphical representation of an object's velocity against time. The slope or gradient of the graph represents the acceleration, while the total area under the graph indicates the displacement or distance traveled in a specific direction. The video explains how to interpret and use velocity-time graphs to calculate acceleration and distance.
💡Gradient
In the context of a velocity-time graph, the gradient represents the rate of change of velocity with respect to time, which is the acceleration of the object. A positive gradient indicates acceleration, while a negative gradient indicates deceleration. The video emphasizes the importance of understanding gradients to determine the object's acceleration from the graph.
💡Displacement
Displacement refers to the change in position of an object, usually represented as a distance in a specific direction. In the video, displacement is calculated by determining the area under the velocity-time graph, which represents the total distance traveled by the object in that direction.
💡Contextual Understanding
Contextual understanding in this video script refers to the ability to comprehend the concepts of acceleration, velocity, and their calculations within the specific scenarios presented, such as the car and cyclist examples. It involves recognizing how these physical quantities apply to real-world situations and how they can be manipulated to solve problems.
💡Problem Solving
Problem solving in the video involves applying the concepts of acceleration, velocity, and their related equations to solve for unknown quantities in given scenarios. It requires the ability to analyze the information provided, apply the correct formulas, and calculate the answers to questions about the motion of objects.
Highlights

Welcome back to free size lessons, where this video aims to teach the concept of acceleration and its calculation.

Acceleration is the change in an object's velocity over a given time period.

The equation for calculating acceleration is given as 'acceleration in meters per second squared equals the change in velocity in meters per second divided by the time in seconds'.

A typical example is provided, where a car accelerates from 50 m/s to 35 m/s in 20 seconds, resulting in an acceleration of 1 m/s^2.

Another example involves a cyclist who decelerates from 6 m/s to 0 m/s in 12 seconds, with an acceleration of -2.5 m/s^2, demonstrating the concept of deceleration.

Velocity-time graphs can also be used to calculate an object's acceleration, where the gradient of the graph indicates the rate of change of velocity.

A horizontal line on a velocity-time graph indicates constant velocity, while upward and downward sloping lines indicate acceleration and deceleration, respectively.

The first part of the graph shows an acceleration calculation with an initial velocity of 0 m/s, a final velocity of 15 m/s, and a time of 100 seconds, resulting in an acceleration of 0.15 m/s^2.

In the last part of the graph, the object decelerates from 15 m/s to 0 m/s in 300 seconds, with an acceleration of -0.05 m/s^2.

The total area under a velocity-time graph represents the distance traveled in a specific direction, which is the displacement.

When there is constant acceleration or deceleration, the graph can be divided into shapes like triangles and rectangles to calculate the total area and thus the displacement.

For non-constant acceleration or deceleration, the total area under the graph is calculated by counting squares, with each square representing a unit of area.

An example is given where the total number of squares under the graph is 20, and with each square having an area of 250, the total displacement is 5,000 meters.

The video mentions a vision workbook with plenty of questions on acceleration on velocity-time graphs, which can be accessed by clicking on a link.

The video concludes with a recap of the importance of understanding acceleration and its calculation, as well as the application of velocity-time graphs in problem-solving.

Transcripts

Browse More Related Video

GCSE Physics - Velocity Time Graphs #54

High School Physics: Graphing Motion

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics

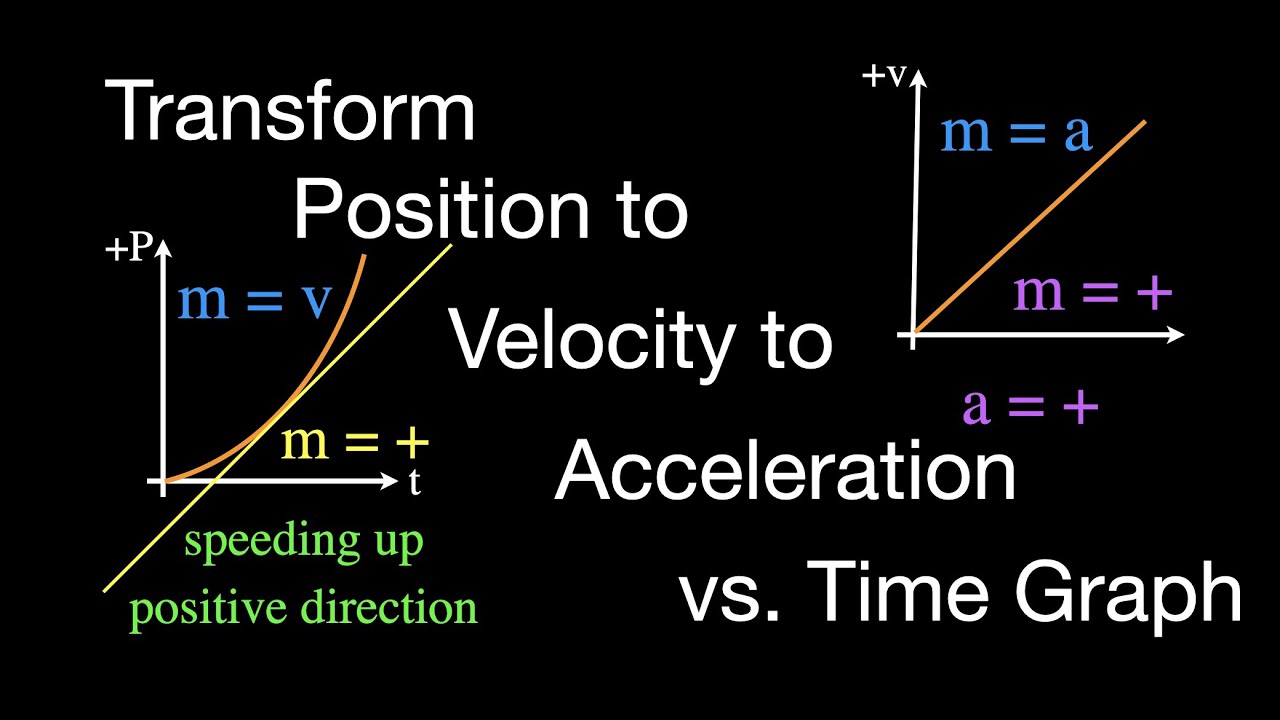
Motion Graphs: Transforming Position to Velocity to Acceleration vs Time

College Physics 1: Lecture 8 - Acceleration

Motion Graphs - AP Physics 1: Kinematics Review Supplement

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationAccelerationCalculationVelocityTimeGraphsKinematicsLearningPhysicsScienceLessonMathematicalConceptsEducationalContentPhysicsProblemSolvingInteractiveTeaching