Calculating Acceleration From a Velocity-Time Graph - GCSE Physics | kayscience.com

KayScience
24 Aug 202005:23
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script explains how to calculate the acceleration of an object using a velocity-time graph. It begins with the car's initial stationary state, details the formula for acceleration (a = (v - u) / t), and demonstrates its application at different stages of the car's journey, including constant velocity and deceleration. The script encourages viewers to practice these concepts and seek additional resources for further understanding.

Takeaways
  • 📈 The script introduces a method to solve problems using a velocity-time graph.
  • ⏱️ The equation for acceleration is a = (v - u) / t, where 'a' is acceleration, 'v' is final velocity, 'u' is initial velocity, and 't' is time.
  • 🚗 For the first part, a car starts from rest (u = 0 m/s) and reaches a velocity of 30 m/s in 6 seconds, resulting in an acceleration of 5 m/s².
  • 🔄 Between 6 and 18 seconds, the car maintains a constant velocity of 30 m/s, which means the acceleration is 0 m/s² as there is no change in velocity.
  • 📉 From 18 to 20 seconds, the car decelerates from 30 m/s to 0 m/s in 2 seconds, leading to a negative acceleration of -15 m/s².
  • 🔢 The velocity-time graph helps to visualize the change in velocity over time and to calculate acceleration at different stages of an object's motion.
  • 🎯 The key to solving these problems is identifying the correct initial and final velocities, as well as the time interval for each segment of motion.
  • 🚦 A positive acceleration indicates speeding up, while a negative acceleration indicates slowing down.
  • 📝 Practice is encouraged to solidify understanding, with prompts to press pause and attempt questions independently.
  • 💡 The script suggests revisiting the video material for correction and further understanding if the viewer gets stuck.
  • 📚 Additional resources for learning are available at ksites.com, including free videos, worksheets, and quizzes at kscience.com.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is to teach how to calculate the acceleration of an object using a velocity-time graph.

  • What is the formula used to calculate acceleration?

    -The formula used to calculate acceleration is a = (v - u) / t, where 'a' is acceleration, 'v' is final velocity, 'u' is initial velocity, and 't' is the time taken for the change in velocity.

  • What was the initial velocity (u) of the car at the beginning of the journey?

    -The initial velocity (u) of the car at the beginning of the journey was 0 meters per second.

  • What was the final velocity (v) the car reached in the first part of the journey?

    -The car reached a final velocity (v) of 30 meters per second in the first part of the journey.

  • How long did it take for the car to accelerate from 0 to 30 meters per second?

    -It took the car 6 seconds to accelerate from 0 to 30 meters per second.

  • What is the acceleration of the car during the constant velocity phase between 6 and 18 seconds?

    -The acceleration of the car during the constant velocity phase between 6 and 18 seconds is 0 meters per second squared because the velocity does not change.

  • What is the initial velocity (u) and final velocity (v) of the car during the deceleration phase between 18 and 20 seconds?

    -The initial velocity (u) of the car during the deceleration phase is 30 meters per second, and the final velocity (v) is 0 meters per second.

  • What is the time duration of the deceleration phase from 30 meters per second to a complete stop?

    -The time duration of the deceleration phase is 2 seconds (from 18 seconds to 20 seconds).

  • What is the acceleration of the car during the deceleration phase?

    -The acceleration of the car during the deceleration phase is -15 meters per second squared, indicating that the car is slowing down or decelerating.

  • Why is the acceleration negative during the deceleration phase?

    -The acceleration is negative during the deceleration phase because the final velocity is less than the initial velocity, indicating a decrease in speed.

  • How can one determine if an object is accelerating or decelerating using the velocity-time graph?

    -One can determine if an object is accelerating or decelerating by observing the change in velocity over time on the graph. If the velocity increases, it indicates acceleration; if it decreases, it indicates deceleration.

  • What is the significance of the slope on the velocity-time graph?

    -The slope on the velocity-time graph represents the acceleration of the object. A positive slope indicates acceleration, while a negative slope indicates deceleration. A flat line indicates constant velocity with no acceleration.

Outlines
00:00
📊 Velocity Time Graph Analysis

This paragraph introduces the concept of a velocity time graph and explains how to use it to calculate the acceleration of an object. It begins with identifying the initial and final velocities (u and v) from the graph and applying the formula a = (v - u) / t. The example given involves a car accelerating from 0 to 30 m/s in 6 seconds, resulting in an acceleration of 5 m/s². The explanation continues with calculating the acceleration during a constant velocity phase, which is found to be zero since there's no change in velocity. Lastly, it covers calculating the deceleration when the car goes from 30 m/s to 0 m/s in 2 seconds, yielding a negative acceleration of -15 m/s².

05:02
📝 Practice and Additional Resources

The second paragraph emphasizes the importance of practicing the concepts learned by attempting the questions provided in the video. It encourages viewers to pause the video to check their answers and make corrections as needed. Additionally, it directs viewers to visit ksites.com for more educational resources such as free videos, worksheets, and quizzes related to the topic. The paragraph concludes with a reminder to engage with the content by liking and subscribing to the channel.

Mindmap
Keywords
💡Velocity
Velocity is a physical quantity that describes the rate of change of an object's position with respect to time, given in meters per second (m/s). In the video, velocity is represented on the y-axis of the velocity-time graph, showing how the car's speed changes over time. For instance, the car starts with a velocity of zero m/s and accelerates to 30 m/s within six seconds.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time, and it is typically measured in meters per second squared (m/s^2). In the context of the video, the concept of acceleration is used to calculate how quickly the car's velocity changes at different stages of its journey, as depicted on the velocity-time graph.
💡Time
Time is a fundamental physical quantity that corresponds to the progression of events and is used to measure the duration of these events. In the video, time is represented on the x-axis of the velocity-time graph and is crucial in calculating the car's acceleration at different intervals, such as the six seconds it takes for the car to accelerate from rest to 30 m/s.
💡Graph
A graph is a visual representation of data, showing the relationship between two or more variables. In the video, a velocity-time graph is used to illustrate the car's velocity changes over time. The graph helps in visualizing and calculating the car's acceleration and constant velocity segments.
💡Equation
An equation is a mathematical statement that asserts the equality of two expressions. In the video, the equation a = (v - u) / t is used to calculate the acceleration of the car at different points during its journey. This equation relates the change in velocity to the time taken, providing a quantitative measure of the car's acceleration.
💡Deceleration
Deceleration is the decrease in the speed of an object, which is a type of acceleration but with a negative value. In the video, the car experiences deceleration when it reduces its velocity from 30 m/s to a stop within two seconds, indicating a negative acceleration.
💡Constant Velocity
Constant velocity refers to the state where an object moves at a steady speed, meaning there is no acceleration or deceleration. In the video, the car maintains a constant velocity of 30 m/s for a period of 12 seconds, indicating no change in speed during this interval.
💡Initial Velocity (u)
Initial velocity, often denoted as 'u', is the speed of an object at the beginning of a motion or a time interval. In the video, the initial velocity of the car is zero m/s at the start, and it changes to 30 m/s after six seconds of acceleration.
💡Final Velocity (v)
Final velocity, often denoted as 'v', is the speed of an object at the end of a motion or a time interval. In the video, the car's final velocity varies at different stages, such as 30 m/s after accelerating for six seconds and 0 m/s after decelerating for two seconds.
💡Practice
Practice refers to the act of repeatedly performing an activity to improve one's understanding or skill in a particular area. In the video, the viewer is encouraged to practice using the key concepts and equations to solve problems related to velocity and acceleration.
💡Understanding
Understanding is the ability to comprehend the meaning, significance, and application of information or a concept. In the video, the goal is for viewers to gain an understanding of the principles of velocity and acceleration, as well as how to apply these principles to analyze motion as depicted on a graph.
💡Concepts
Concepts are abstract ideas or general notions that represent the core understanding of a subject area. In the video, key concepts such as velocity, acceleration, and the relationship between them are introduced and explained to help the viewer grasp the fundamental principles of motion.
Highlights

The problem introduces a method to solve velocity-time graph related questions.

The equation a = (v - u) / t is used to calculate acceleration from a velocity-time graph.

The initial velocity (u) of the car is zero meters per second.

The car accelerates to a final velocity (v) of 30 meters per second.

The time (t) taken to reach 30 meters per second is six seconds.

The acceleration during the first part of the journey is calculated to be 5 meters per second squared.

Between 6 and 18 seconds, the car travels at a constant velocity with zero acceleration.

The car's final velocity for the constant velocity segment is 30 meters per second.

The car decelerates from 30 meters per second to zero in two seconds.

The deceleration is calculated to be -15 meters per second squared.

Negative acceleration indicates deceleration.

The transcript provides a clear example of how velocity changes with time.

The method can be applied to calculate acceleration at different stages of an object's journey.

The importance of understanding the difference between acceleration and constant velocity is emphasized.

The use of the velocity-time graph as a tool for analyzing motion is demonstrated.

The transcript encourages practice and self-correction through pausing and reviewing answers.

Transcripts

Browse More Related Video

AP Physics Workbook 1.H Relationship between Position,Velocity and Acceleration

Equations of Motion by Graphical Method

Velocity time graph conversion to Position time graph

AP Physics Workbook 1.G Graphs of Velocity

AP Physics B Kinematics Presentation #03

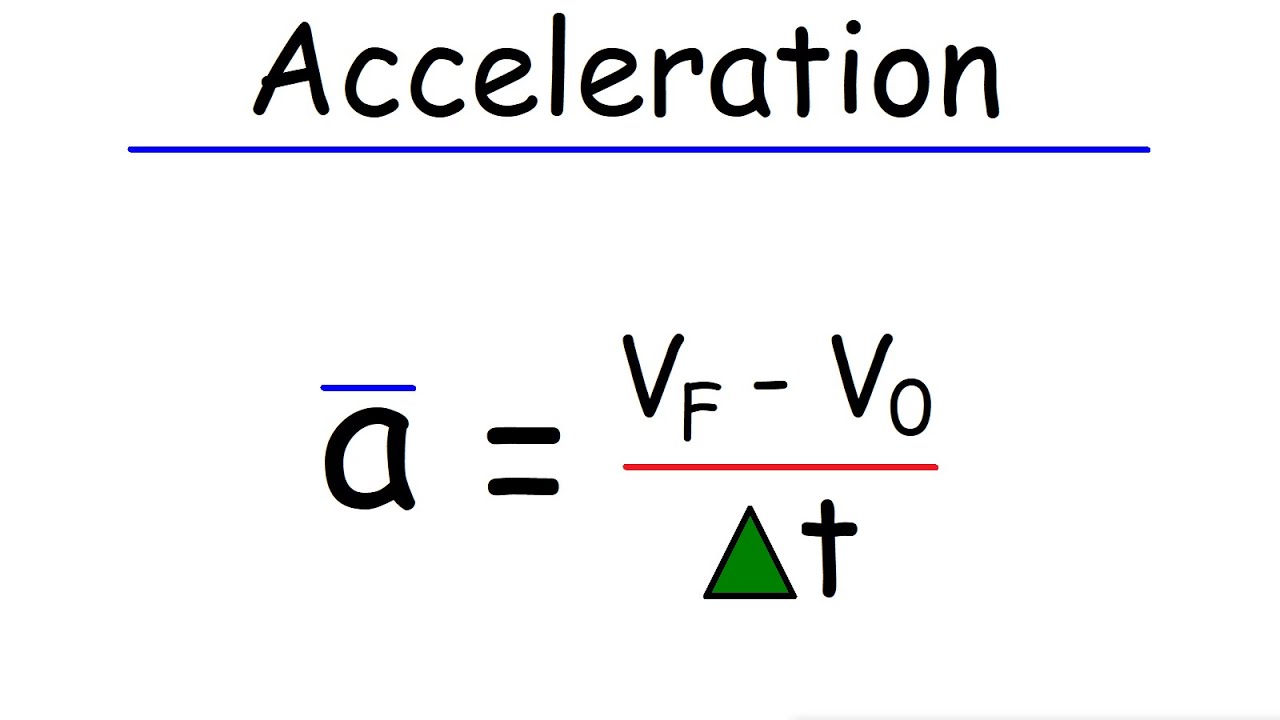
Average Acceleration and Instantaneous Acceleration

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationAcceleration CalculationVelocity-Time GraphKinematicsMechanicsLearning ResourceInteractive LearningConcept ClarificationFree VideoWorksheets