Electric Current & Circuits Explained, Ohm's Law, Charge, Power, Physics Problems, Basic Electricity

The Organic Chemistry Tutor
20 Feb 201718:09
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers an insightful exploration of fundamental electrical concepts, including Ohm's Law, electric current, and power calculations. It clarifies the difference between conventional current and electron flow, and demonstrates how voltage, current, and resistance interrelate. The script presents a series of practical problems to reinforce understanding, covering charge calculation, power dissipation in resistors, and the cost of operating electrical devices over time. The comprehensive walkthrough of problem-solving techniques enhances the viewer's grasp of electrical principles and their real-world applications.

Takeaways
  • πŸ”‹ Conventional current flows from the positive terminal to the negative terminal of a battery, but electron flow is opposite.
  • πŸ”§ Current (I) is defined as the rate of charge flow, calculated as the electric charge (Q) divided by time (t), or Ξ”Q/Ξ”t.
  • ⚑ The unit for electric charge is the coulomb, and the charge of an electron is approximately -1.6 Γ— 10^(-19) coulombs.
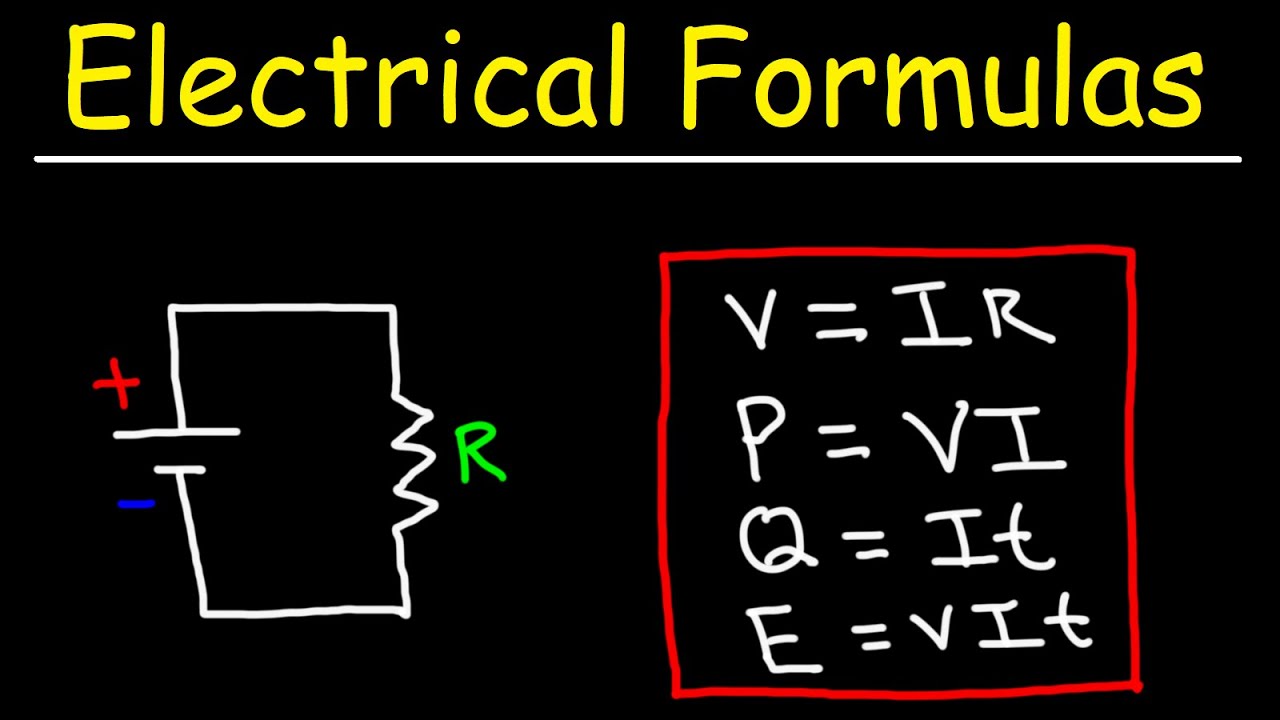
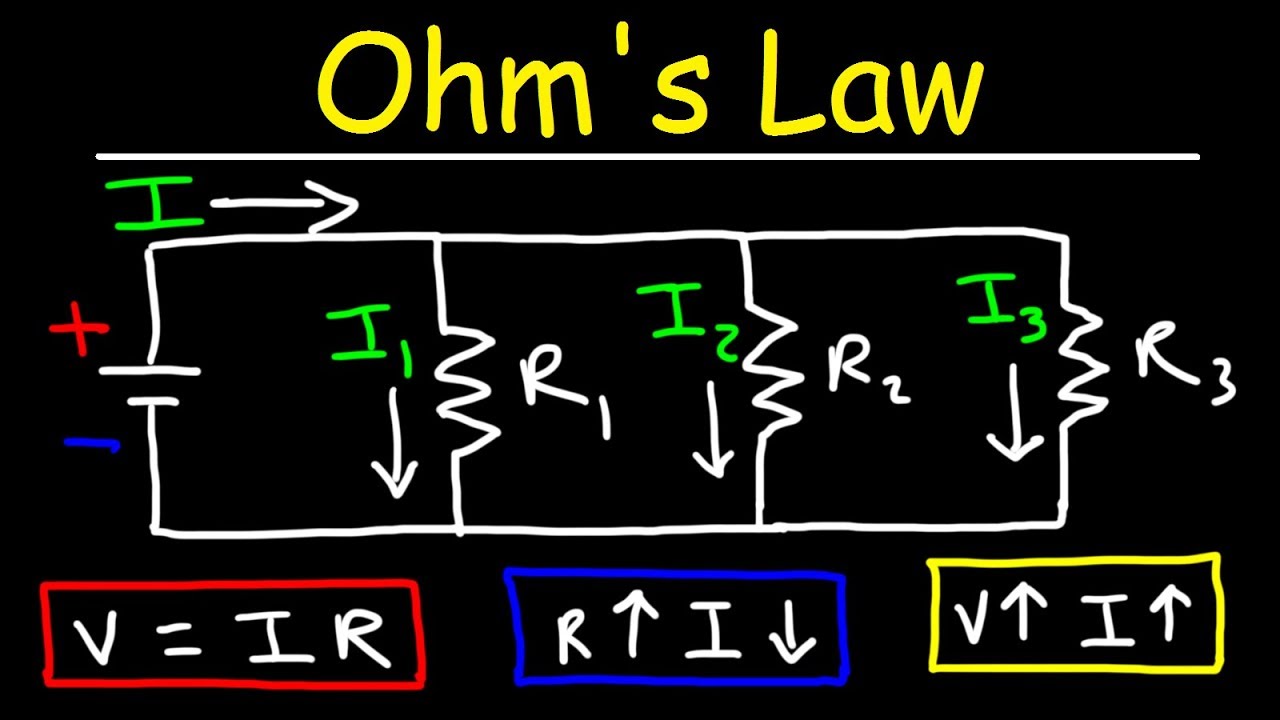
  • πŸ“ˆ Ohm's Law (V = IR) describes the relationship between voltage (V), current (I), and resistance (R), with resistance measured in ohms.
  • πŸ”„ Increasing voltage while keeping resistance constant results in increased current; increasing resistance decreases current.
  • πŸ’‘ Electric power (P) is the product of voltage and current (P = VI) and is measured in watts, with 1 watt equal to 1 joule per second.
  • πŸ”Œ To calculate electric charge (Q), multiply current (I) by time (t) in seconds, accounting for unit conversions.
  • 🌐 The number of electrons can be determined by dividing the total charge by the charge of a single electron.
  • πŸ”¦ When calculating power dissipated by a resistor, use the formula P = I^2R or P = VI, where V is the voltage across the resistor.
  • πŸ’° The cost of operating an electrical device can be calculated by determining its power consumption and multiplying by the cost per kilowatt-hour.
  • πŸ•° To find the electric current that flows through a resistor, use the charge passed and the total time in seconds, and account for unit conversions.
Q & A

  • What is the basic definition of conventional current?

    -Conventional current is defined as the flow of positive charge. It is the movement of charge from the positive terminal to the negative terminal of a power source, following the path of high voltage to low voltage, similar to the flow of water from a higher to a lower position.

  • How does electron flow differ from conventional current?

    -Electron flow is opposite to conventional current. In reality, electrons, which carry a negative charge, emanate from the negative terminal and flow towards the positive terminal, contrary to the direction of conventional current.

  • What is the formula for calculating electric current?

    -The formula for calculating electric current (I) is the electric charge (Q) divided by the time (t), or Ξ”Q/Ξ”t. The electric charge (Q) is measured in coulombs, and the time (t) is in seconds. The unit for current is the ampere (amp), where 1 amp is equal to 1 coulomb per second.

  • What is the charge of an electron and how does it relate to the unit of current?

    -An electron has a charge that is equal to approximately 1.6 times 10 to the negative 19 coulombs, which is negative. This charge is the fundamental unit of electric charge, and the ampere, the unit of current, is defined such that 1 amp is the flow of 1 coulomb of charge per second.

  • Explain the relationship between voltage, current, and resistance as described by Ohm's Law.

    -Ohm's Law describes the relationship between voltage (V), current (I), and resistance (R) as V = IR. This means that voltage is the product of the current and resistance. When resistance is kept constant, increasing the current results in an increase in voltage, and vice versa. Increasing resistance decreases the current, while decreasing resistance increases the current.

  • What are the three forms of the electric power equation and how are they derived?

    -The three forms of the electric power equation are: (1) P = VI, which is the product of voltage and current; (2) P = I^2R, which is the square of the current times resistance; and (3) P = V^2/R, which is the square of the voltage divided by resistance. These forms are derived from each other by substituting the Ohm's Law relationship (V = IR) into the power equation.

  • How is electric power related to the transfer of energy?

    -Electric power is the rate at which energy is transferred or converted. It is measured in watts, where 1 watt is equal to 1 joule per second. This indicates the amount of energy that can be transferred or converted in one second.

  • In the given script, how is the electric charge calculated in the first problem?

    -In the first problem, the electric charge (Q) is calculated by multiplying the current (I) by the time (t) in seconds. The current is 3.8 amps, and the time is 12 minutes, which is converted to 720 seconds (12 minutes * 60 seconds/minute). Thus, Q = I * t = 3.8 amps * 720 seconds = 2736 coulombs.

  • What is the relationship between the number of electrons and the amount of charge?

    -The number of electrons is directly proportional to the amount of charge. The charge of a single electron is 1.6 times 10 to the negative 19 coulombs. By dividing the total charge by the charge of a single electron, you can find the number of electrons represented by that charge.

  • How is the current calculated in the second problem with a 9-volt battery and a 250-ohm resistor?

    -The current (I) is calculated using Ohm's Law (V = IR). The voltage (V) is 9 volts, and the resistance (R) is 250 ohms. Solving for I, we get I = V/R = 9 volts / 250 ohms = 0.036 amps or 36 milliamps.

  • What is the cost calculation for operating a 1.8-watt light bulb for a month, given the electricity cost of 11 cents per kilowatt-hour?

    -First, convert the power from watts to kilowatts by dividing by 1000 (1.8 watts / 1000 = 0.0018 kilowatts). Then, calculate the energy consumed in a month by multiplying the power in kilowatts by the number of hours in a month (0.0018 kilowatts * 30 days * 24 hours/day = 12.96 kilowatt-hours). Finally, find the cost by multiplying the energy by the cost per kilowatt-hour (12.96 kilowatt-hours * $0.11/kilowatt-hour = $1.4256). The approximate cost is 14 cents.

  • How can you find the voltage across a resistor given the charge and resistance?

    -The voltage (V) across a resistor can be found using Ohm's Law (V = IR). If you know the charge (Q) that flows through the resistor and the resistance (R), you can calculate the current (I) as Q divided by the product of the resistance and the time in seconds (I = Q / (R * time)). Once you have the current, you can then find the voltage by multiplying the current by the resistance (V = I * R).

Outlines
00:00
πŸ”‹ Understanding Basic Electric Concepts and Ohm's Law

This paragraph introduces fundamental concepts related to electric current and Ohm's Law. It explains the direction of conventional current and electron flow, emphasizing that conventionally, current is thought to flow from the positive to the negative terminal, akin to water flowing from high to low. The actual electron flow is opposite. The paragraph defines current as the rate of charge flow (measured in amperes) and explains the relationship between voltage, current, and resistance as described by Ohm's Law (V=IR). It highlights the direct relationship between voltage and current and the inverse relationship between current and resistance. The concept of electric power, measured in watts, is also introduced, with power being the rate of energy transfer.

05:00
πŸ”§ Calculating Electric Charge and Electron Count

The second paragraph focuses on practical problem-solving related to electric charge and the number of electrons represented by a given charge. It provides a step-by-step calculation of electric charge (in coulombs) using the current (3.8 amps) and time (12 minutes converted to seconds). The paragraph then explains how to convert this charge to the number of electrons, using the charge of a single electron (1.6 x 10^-19 coulombs). The result is approximately 1.71 x 10^22 electrons, illustrating the proportionality between the amount of charge and the number of electrons.

10:01
πŸ’‘ Applying Ohm's Law to Circuit Analysis

This paragraph delves into applying Ohm's Law to analyze simple circuits involving a battery, a resistor, and a light bulb. It demonstrates how to calculate the current passing through a resistor connected to a 9-volt battery and how to determine the power dissipated by the resistor. The analysis extends to calculating the power delivered by the battery, emphasizing the balance of power in the circuit. The paragraph also covers calculating the resistance of a light bulb using Ohm's Law and determining the power consumed by the bulb. Additionally, it explores the cost of operating the light bulb for a month, given the electricity rate of 11 cents per kilowatt-hour.

15:02
🏎️ Determining Voltage and Resistance in an Electric Motor

The fourth paragraph discusses the calculation of voltage and internal resistance in an electric motor. It uses the given power consumption (50 watts) and current (400 milliamps converted to amps) to find the voltage across the motor using the power formula (P = VI). The paragraph then applies Ohm's Law to determine the motor's internal resistance. The detailed calculations provide insights into the relationships between power, voltage, current, and resistance in the context of an electric motor.

⚑️ Calculating Electric Current, Power, and Voltage in a Resistor

The final paragraph presents a scenario where a specific amount of charge (12.5 coulombs) flows through a resistor (5 kiloohms) over a period of eight minutes. It explains how to calculate the electric current using the charge, resistance, and time. The paragraph then uses the derived current to calculate the power consumed by the resistor, applying the formula for power in terms of current and resistance (P = I^2R). Additionally, it shows how to find the voltage across the resistor using Ohm's Law (V = IR), completing the analysis of the resistor's behavior under the given conditions.

Mindmap
Keywords
πŸ’‘Conventional Current
Conventional current is a fundamental concept in electrical circuits, referring to the hypothetical flow of positive charge from the positive terminal to the negative terminal of a power source, such as a battery. This concept is rooted in historical convention, dating back to a time before the actual charge carriers (electrons) were well understood. Despite electrons carrying a negative charge and moving in the opposite direction, conventional current provides a simplified way to analyze and understand circuit behavior. In the video, this concept is used to explain current flow through circuits, illustrating the directionality assumed for analysis and design purposes.
πŸ’‘Ohm's Law
Ohm's Law is a foundational principle in the study of electricity, describing the relationship between voltage (V), current (I), and resistance (R) in an electrical circuit. It states that the current through a conductor between two points is directly proportional to the voltage across the two points, and inversely proportional to the resistance between them (V = IR). This equation allows for the calculation of one electrical parameter if the other two are known, offering a critical tool for electrical engineering and physics. The video uses Ohm's Law to solve problems related to voltage, current, and resistance, demonstrating its practical application in analyzing electrical circuits.
πŸ’‘Resistance
Resistance, measured in ohms (Ξ©), is a property of materials that impedes the flow of electric current. It is a critical concept in the study of electricity and electronics, reflecting how difficult it is for current to flow through a component. The video uses resistance to explain the behavior of circuits, such as comparing it to the flow of cars on a highway, where a higher resistance is akin to a narrower road that limits the flow of traffic. By manipulating resistance, one can control the amount of current in a circuit, demonstrating the concept's relevance in designing and analyzing electrical systems.
πŸ’‘Electric Power
Electric power, measured in watts (W), represents the rate at which electrical energy is converted into another form of energy, such as light, heat, or mechanical power. It can be calculated using the formula P = VI, where P is power, V is voltage, and I is current. The video explores different formulas for calculating power based on known quantities, emphasizing its importance in determining how much energy electrical devices consume and produce. Understanding electric power is essential for designing efficient electrical systems and calculating energy costs.
πŸ’‘Voltage
Voltage, or electric potential difference, measured in volts (V), is a fundamental electrical quantity that describes the electric potential energy per unit charge between two points. It is the driving force that pushes electrons through a circuit. In the context of the video, voltage is discussed as a key factor in determining the flow of current and the operation of circuits according to Ohm's Law (V = IR), illustrating its central role in the function and analysis of electrical systems.
πŸ’‘Coulomb
The coulomb (C) is the SI unit of electric charge, named after Charles-Augustin de Coulomb. One coulomb is equivalent to the charge of approximately 6.242 x 10^18 electrons. The video mentions this unit in the context of calculating the amount of charge that flows through a circuit over time (Q = It), providing a foundation for understanding how electric current is quantified and the relationship between charge, current, and time.
πŸ’‘Ampere
The ampere (amp, A) is the unit of electric current in the International System of Units (SI). It is defined as the flow of one coulomb of charge per second. The video discusses the ampere in the context of measuring electric current, illustrating how current represents the rate of charge flow through a conductor. Understanding the ampere is crucial for analyzing electrical circuits, including calculating the quantity of charge transferred and the effects of varying current levels on circuit operation.
πŸ’‘Electron Flow
Electron flow refers to the movement of electrons from the negative terminal to the positive terminal of an electrical power source, opposite to the direction of conventional current. This concept is based on the physical behavior of electrons, which carry a negative charge, within a conductor when a potential difference (voltage) is applied. The video mentions electron flow to clarify the actual direction of charge carriers in a circuit, contrasting it with the simplified model of conventional current used for circuit analysis.
πŸ’‘Electric Charge
Electric charge is a fundamental property of particles that determines their electromagnetic interaction. Measured in coulombs (C), charge can be positive or negative, with electrons carrying a negative charge. The video discusses electric charge in the context of current and power calculations, highlighting how the flow of charge through a circuit over time relates to the concept of electric current (I = Q/t). Understanding electric charge is essential for analyzing the movement of electrons in circuits and the resulting electrical phenomena.
πŸ’‘Watt
The watt (W) is the unit of power in the International System of Units (SI), equivalent to one joule per second. It measures the rate of energy transfer or conversion. In the video, the watt is discussed in the context of electric power calculation formulas (P = VI, P = I^2R, P = V^2/R), demonstrating how to determine the energy usage of electrical components. Understanding watts is vital for evaluating the efficiency and energy consumption of electrical systems and devices.
Highlights

Introduction to basic equations and practice problems involving electric current and Ohm's Law.

Explanation of conventional current flow from the positive to the negative terminal, analogous to water flow from high to low positions.

Clarification that electron flow is opposite to conventional current, emanating from the negative terminal and flowing towards the positive terminal.

Definition of current as the rate of charge flow, measured in amperes (amps), with the formula of charge (in coulombs) divided by time (in seconds).

Discussion of Ohm's Law, which describes the relationship between voltage, current, and resistance, with the formula V=IR.

Explanation of how increasing voltage or decreasing resistance results in an increase in current, and vice versa.

Introduction to electric power, which is the product of voltage and current, with power measured in watts and the formula P=VI, I^2R, or V^2/R.

Solution to a problem calculating the electric charge (in coulombs) that passes through a circuit using the formula Q=It.

Conversion of charge to the number of electrons using the charge of a single electron (1.6 x 10^-19 coulombs).

Calculation of current passing through a resistor using Ohm's Law with a 9-volt battery and a 250-ohm resistor.

Determination of power dissipated by a resistor using the formula P=I^2R and the concept of power in watts.

Explanation of how power delivered by a battery equals the power absorbed by the resistor in a simple circuit.

Calculation of a light bulb's electrical resistance using Ohm's Law with a 12-volt battery and the measured current.

Estimation of the cost to operate a light bulb for a month based on electrical power consumption and the cost of electricity.

Determination of voltage across a motor using the power and current values with the formula V=P/I.

Calculation of a motor's internal resistance using Ohm's Law with the known voltage and current values.

Calculation of the electric current flowing through a resistor using the charge, resistance, and time with the formula I=Q/t.

Determination of the power consumed by a resistor using the formula P=I^2R and the concept of power in watts.

Calculation of the voltage across a resistor using the current through it and its resistance with the formula V=IR.

Transcripts

Browse More Related Video

Electrical Formulas - Basic Electricity For Beginners

Electricity - Basic Introduction

Voltage Current and Resistance

Circuits (AP Physics SuperCram Review)

Ohm's Law

Basic Electricity - Resistance and Ohm's law

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Electrical EngineeringOhm's LawPhysics EducationCircuit AnalysisVoltage CalculationCurrent FlowResistance ImpactPower DissipationEnergy CostProblem Solving