Kirchhoff's Current Law, Junction Rule, KCl Circuits - Physics Problems

The Organic Chemistry Tutor
13 Dec 201711:59
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explains Kirchhoff's Current Law (KCL), a fundamental principle in electrical circuits stating that the algebraic sum of currents at a junction is zero. It illustrates this concept with several examples, demonstrating how to assign positive and negative values to currents based on their direction relative to the junction and how to calculate unknown currents using the law. The examples reinforce the understanding of KCL and its application in analyzing electrical networks.

Takeaways
  • 📝 Kirchhoff's Current Law (KCL) states that the total current flowing into a junction must equal the total current flowing out of it.
  • 🔧 When applying KCL, currents entering a junction are considered positive, and currents leaving a junction are considered negative.
  • 🌐 KCL ensures that the sum of all individual currents at a junction equals zero, demonstrating the conservation of electric charge at the junction.
  • 🔢 In the first example, 10 amps entering and 10 amps leaving the junction balance each other out, resulting in a net current of zero.
  • 🔌 In the second example, with 10 amps entering and 14 amps leaving, the calculation shows that i3 must be a positive 4 amps entering the junction to maintain the balance.
  • 🛠️ The third example involves a more complex junction with four currents. The calculation yields i4 as a negative 8 amps, indicating it leaves the junction.
  • 🔄 The final example requires calculating two unknown currents (i3 and i4) based on given conditions. It results in i3 being -8 amps and i4 being -16 amps.
  • 📊 By solving for the unknown currents, the balance of currents entering and leaving the junctions can be verified, ensuring the application of KCL is correct.
  • 🎯 KCL is a fundamental principle used to analyze electrical circuits and networks, and it's crucial for understanding circuit behavior.
  • 💡 The examples provided in the script are practical applications of KCL, demonstrating how to calculate unknown currents based on the law and the given conditions of a circuit.
Q & A

  • What is Kirchhoff's Current Law (KCL)?

    -Kirchhoff's Current Law, abbreviated KCL, states that the total current flowing into a junction must equal the total current flowing out of the junction. Essentially, the algebraic sum of currents at any node in a circuit is zero.

  • How does one determine if a current is positive or negative according to KCL?

    -A current is considered positive if it flows into a junction or node, and negative if it flows out of the junction. The assignment of positive and negative signs is based on the direction of conventional current flow, which is opposite to the flow of electrons.

  • In the example where 10 amps flow into the junction and 10 amps flow out, what is the sum of the currents?

    -In this case, the sum of the currents is zero. This is because the positive 10 amps flowing into the junction is balanced by the negative 10 amps (conventionally opposite direction) flowing out of the junction.

  • How can you calculate the missing current (i3) in a circuit with two known currents, i1 and i2?

    -You can calculate the missing current (i3) by applying KCL. If you know i1 and i2 and the fact that the sum of all currents at the junction must be zero, you can set up an equation: i1 + i2 + i3 = 0. Then solve for i3 by subtracting the sum of i1 and i2 from both sides of the equation.

  • In the example with 10 amps and 14 amps, what is the direction of i3?

    -In this example, i3 is positive, which indicates that the current is flowing into the junction. This is because the sum of the currents (i1 + i2 + i3) must equal zero, and the negative value of i2 (14 amps out of the junction) is balanced by the positive values of i1 (10 amps in) and i3 (4 amps in).

  • What is the significance of the net current being zero in a junction?

    -The net current being zero in a junction is a fundamental principle of KCL and reflects the conservation of charge in electrical circuits. It ensures that no charge is accumulated or lost at the junction, which is consistent with the physical laws governing the behavior of electric current.

  • How does the magnitude and direction of i4 relate to i3 if i4 is twice the value of i3?

    -If i4 is twice the value of i3 in magnitude, it means that the absolute value of i4 is twice as large as that of i3. The direction of i4 may or may not be the same as i3. If it is assumed to have the same direction, then i4 will be either twice as positive or twice as negative as i3, depending on the actual direction of i3.

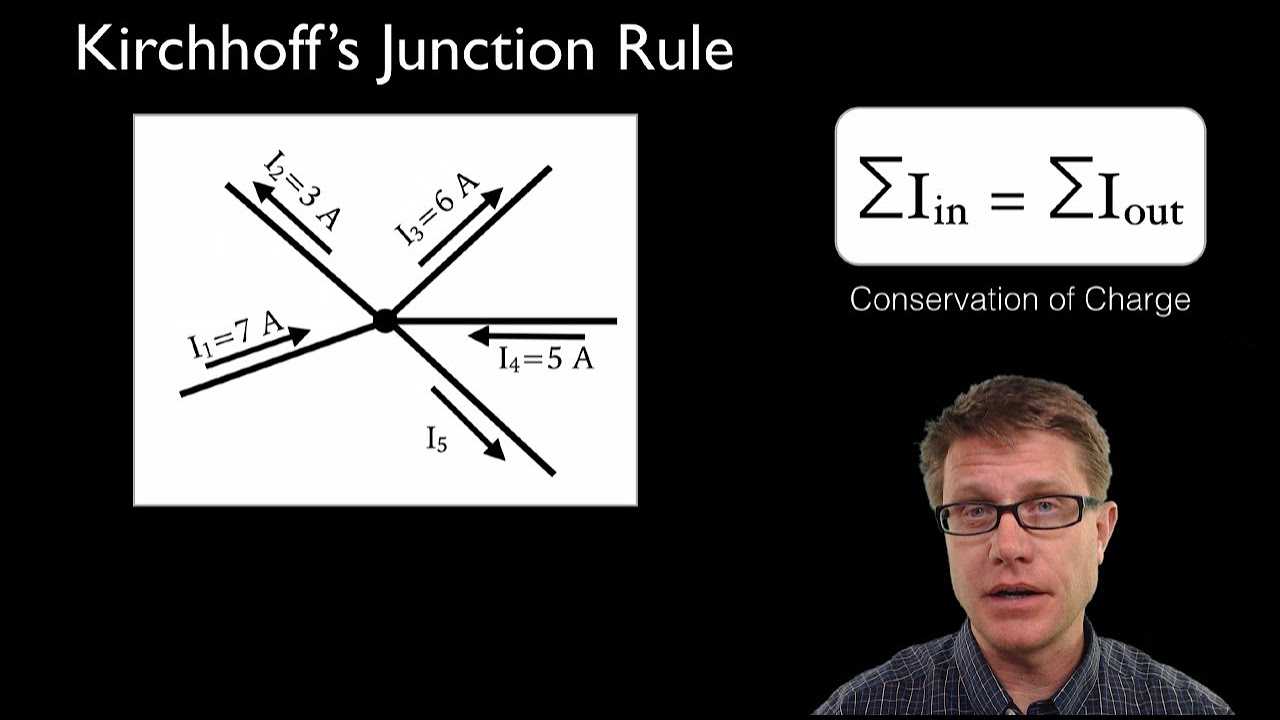
  • What is the total current flowing into and out of the circuit in the final example with currents i1 through i5?

    -In the final example, the total current flowing into the circuit is the sum of the positive currents: 12 amps (i1) and 20 amps (i5), which equals 32 amps. The total current flowing out of the circuit is the sum of the negative currents: 8 amps (i2), 16 amps (2*i3, which is -16), and 8 amps (-i3), which equals -32 amps. The net current, as expected, is zero.

  • What does it mean if a current is negative according to KCL?

    -A negative current according to KCL indicates that the current is flowing out of the junction or node in the direction opposite to the conventional current flow (opposite to the flow of electrons). This is a mathematical representation and does not imply an actual reversal of electron flow.

  • How can you verify if your answers to KCL problems are correct?

    -You can verify the correctness of your answers by ensuring that the sum of all currents at the junction equals zero, as per KCL. If the net current is not zero, it indicates an error in the calculations or the assignment of positive and negative signs.

  • What is the practical application of Kirchhoff's Current Law?

    -Kirchhoff's Current Law is fundamental in the analysis and design of electrical circuits. It helps in understanding the distribution of current in complex networks and is essential for troubleshooting, testing, and ensuring the proper functioning of electrical and electronic systems.

  • Can Kirchhoff's Current Law be applied to a single loop or a closed loop in a circuit?

    -While Kirchhoff's Current Law is specifically concerned with currents at a junction or node, it can also be applied to a closed loop in a circuit by considering the loop as a single path and applying Kirchhoff's Voltage Law (KVL), which states that the sum of voltages around any closed loop in a circuit is zero.

Outlines
00:00
🔧 Introduction to Kirchhoff's Current Law (KCL)

This paragraph introduces Kirchhoff's Current Law (KCL), a fundamental principle in electrical circuit analysis. It explains that the total current flowing into a junction must equal the total current flowing out of it. The concept is illustrated with an example where 10 amps enter and 10 amps leave the junction, summing up to zero. The paragraph further clarifies the assignment of positive and negative values to currents based on their direction relative to the junction. A basic example is worked out to demonstrate the calculation of an unknown current (i3), using the principle that the sum of all currents at a junction must equal zero.

05:02
📐 Solving for Unknown Currents in a Circuit

This paragraph delves into the process of solving for unknown currents in an electrical circuit using KCL. It presents a more complex example with four currents (i1, i2, i3, and i4) meeting at a junction. The paragraph explains how to assign positive or negative values to each current based on whether they are entering or leaving the junction. The method of setting up an equation where the sum of all currents equals zero is used to calculate the magnitude and direction of the unknown current i4. The paragraph emphasizes the importance of understanding the direction of currents in solving circuit problems.

10:02
🧠 Advanced KCL Problem with Multiple Unknowns

The paragraph presents an advanced example of applying KCL to a scenario with multiple unknown currents. It introduces a circuit with five currents (i1, i2, i3, i4, and i5) and provides specific details about their directions and relationships. The paragraph guides through the process of setting up an equation to solve for the unknown currents i3 and i4, taking into account the given information that i4 is twice the magnitude of i3. The solution involves simplifying the equation, solving for i3, and then determining i4 based on the relationship provided. The paragraph concludes by comparing the total currents entering and leaving the circuit, reinforcing the KCL principle that the net current at a junction is zero.

Mindmap
Keywords
💡Kirchhoff's Current Law (KCL)
Kirchhoff's Current Law, abbreviated as KCL, is a fundamental principle in electrical circuit analysis which states that the algebraic sum of all currents entering and exiting a junction or node in a circuit is zero. This law is based on the conservation of electric charge, implying that charge can neither be created nor destroyed. In the video, KCL is the central concept around which the examples and explanations revolve, demonstrating how to apply this law to analyze the flow of current in various hypothetical circuits.
💡Current
Current, measured in amperes (amps), is the flow of electric charge in a circuit. It represents the rate at which charge moves through a conductor. In the context of the video, understanding current is crucial for applying KCL, as it involves identifying the direction and magnitude of currents at a junction. The direction of current is significant: a positive value indicates current flowing into the junction, while a negative value indicates current flowing out.
💡Junction
A junction, also known as a node, is a point in a circuit where two or more circuit elements or branches connect. In the context of the video, the analysis of the currents is focused on these junctions, where the application of Kirchhoff's Current Law helps determine the flow of current through the branches of the circuit. The law ensures that the total current entering a junction equals the total current leaving it.
💡Positive and Negative Current
In the context of the video, positive and negative currents refer to the direction of the flow of electric charge relative to the junction. A positive current indicates that charge is moving into the junction, while a negative current indicates that charge is moving away from the junction. This convention is essential for applying Kirchhoff's Current Law, as it helps in determining the algebraic sum of currents at the junction, which must equal zero.
💡Circuit Analysis
Circuit analysis is the process of determining the behavior of electrical circuits, typically by analyzing the flow of current and voltage within the circuit. In the video, circuit analysis is performed using Kirchhoff's Current Law to understand how currents interact at a junction. This analysis is crucial for the design, testing, and troubleshooting of electrical and electronic systems.
💡Algebraic Sum
The algebraic sum refers to the result of adding numbers considering their signs. In the context of the video, the algebraic sum of currents is used to apply Kirchhoff's Current Law, which states that the sum of currents entering a junction must equal the sum of currents leaving the junction. This concept is essential for ensuring that the conservation of charge is maintained within the circuit.
💡Charge Conservation
Charge conservation is a fundamental principle of physics stating that the total electric charge in an isolated system remains constant over time. In the context of the video, this principle underlies Kirchhoff's Current Law, which asserts that the total current entering a junction must equal the total current leaving it, reflecting the idea that charge cannot be created or destroyed in a circuit.
💡Circuit Branches
Circuit branches are the individual paths that current can take within a circuit. They split from the main path and can recombine or form loops. In the video, the analysis of currents at a junction involves understanding how currents split into branches and how they recombine, which is crucial for applying Kirchhoff's Current Law to determine the unknown currents.
💡Ohm's Law
Ohm's Law is a fundamental relationship in electrical engineering that states that the current through a conductor between two points is directly proportional to the voltage across the two points and inversely proportional to the resistance between them. While not explicitly mentioned in the video script, Ohm's Law is a related concept that is often used in conjunction with Kirchhoff's laws to analyze and solve for unknown values in electrical circuits.
💡Electrical Charge
Electrical charge is a fundamental property of matter that gives rise to one of the four fundamental forces of nature—the electrostatic force. In the context of the video, the flow of electrical charge constitutes the current in a circuit, and the conservation of charge is the underlying principle that Kirchhoff's Current Law is based on.
💡Net Current
Net current refers to the overall or resultant current in a circuit or at a junction after accounting for all individual currents. It is calculated by summing the currents flowing in different directions, considering their signs. In the context of the video, the net current at a junction must be zero, as per Kirchhoff's Current Law, indicating that the total incoming current is balanced by the total outgoing current.
Highlights

Kirchhoff's Current Law (KCL) is introduced, which states that the total current flowing into a junction equals the total current flowing out.

Current values flowing into a junction are considered positive, while those leaving the junction are considered negative.

The sum of all individual currents at a junction must add up to zero, following the convention of positive and negative assignments.

A basic example is provided where 10 amps enter and 10 amps leave the junction, demonstrating the balance of current.

In the examples, the direction of current flow is used to determine whether the current value is positive or negative.

A calculation is shown where 10 amps and 4 amps flow into a junction, and it's determined that 6 amps must flow out.

Another example is given where 10 amps and 14 amps are considered, and 4 amps is calculated to flow into the junction.

A more complex example with four currents is solved, resulting in a net current of zero flowing into the junction.

The concept of current balance is emphasized, showing that the total current entering the circuit equals the total current leaving it.

A final example involves a junction with five currents, where it's given that i4 is twice the magnitude of i3.

In the final example, the calculated values for i3 and i4 confirm that the net current flowing into the circuit is zero.

The video provides a method to test the correctness of the answers by comparing the total current flowing in and out of the circuit.

The importance of understanding the direction of current flow for correctly applying KCL is stressed.

The video serves as an educational resource for understanding and applying Kirchhoff's Current Law in circuit analysis.

Transcripts

Browse More Related Video

Capacitors and Kirchhoff: Crash Course Physics #31

Kirchhoff's Junction Rule

Kirchhoff's Voltage Law - KVL Circuits, Loop Rule & Ohm's Law - Series Circuits, Physics

Solving Circuit Problems using Kirchhoff's Rules

Kirchhoff's Law, Junction & Loop Rule, Ohm's Law - KCl & KVl Circuit Analysis - Physics

Kirchhoff's Laws - A-level Physics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Kirchhoff's LawElectrical EngineeringCircuit AnalysisPhysics ConceptsEducational VideoCurrent CalculationJunction AnalysisPositive Negative CurrentsEngineering TutorialElectrical Currents