Projectile Motion (of an Object Fired at an Angle) - A Level Physics

Physics Online

19 Mar 201610:39

EducationalLearning

32 Likes 10 Comments

TLDRThe video script delves into the physics of projectile motion, particularly as it applies to indirect fire weaponry used by artillery units. It explains how factors like air resistance, wind, temperature, and the Earth's rotation can affect the trajectory of projectiles. The script uses the example of a light gun firing a 15-20 kg projectile at a 30-degree angle to illustrate the principles of projectile motion, including the calculations for range, maximum height, and time of flight. The presenter breaks down the projectile's path into two halves, highlighting the constant horizontal velocity and the changing vertical velocity, to provide a comprehensive understanding of how artillery shells reach their targets.

Takeaways

🎯 The script discusses projectile motion, particularly in the context of direct and indirect fire weapons.
🚀 Direct fire weapons, like rifles and tanks, fire at a target along the horizontal plane.
💣 Indirect fire, used by artillery, involves firing at a high angle to reach targets that are not in direct line-of-sight.
🏹 The video uses an example of a light gun firing a 15-20 kg projectile at 300 meters per second at a 30-degree angle.
🌀 In an ideal physics world without air resistance, the projectile's path can be modeled with simplified equations.
📈 The script explains the use of Suvat equations to calculate the projectile's motion in both horizontal and vertical directions.
🔢 The projectile's maximum height is calculated to be approximately 1146.79 meters.
🕒 The time taken for the projectile to reach its maximum height is about 15.29 seconds.
🏞️ The first half of the projectile's journey is mirrored in the second half due to constant horizontal velocity and symmetrical path.
📊 The total range of the projectile is roughly 8 kilometers, with a total time of flight of around 31 seconds.
🌐 The principles discussed apply to various types of projectile motion, not just military applications.

Q & A

What type of projectile motion is discussed in the video?
-The video discusses projectile motion related to direct fire weapons, such as those used in armored vehicles and rifles, as well as indirect fire, which is associated with artillery guns.
How does the video differentiate between direct fire and indirect fire weapons?
-Direct fire weapons, like rifles and some armored vehicles, fire at the enemy directly without any significant angle. Indirect fire weapons, such as artillery guns, are designed to fire at a high angle over long distances, with the projectile initially moving upwards before following a curved path towards the target.
What factors are ignored in the ideal physics world example provided in the video?
-In the ideal physics world example, factors such as air resistance, wind direction and speed, temperature, and the rotation of the Earth are ignored to simplify the projectile motion calculations.
What is the significance of the projectile's angle and initial velocity in determining its trajectory?
-The projectile's angle and initial velocity determine the shape and length of its trajectory. A higher angle and greater initial velocity will result in a higher and possibly longer flight path, affecting the distance and height the projectile reaches.
How does the absence of air resistance affect the projectile motion calculations?
-Without air resistance, the projectile's motion can be simplified using basic kinematic equations. This idealization assumes constant velocities and ignores the effects of drag, which in reality would decrease the projectile's range and alter its trajectory.
What are the key elements of the projectile motion equation used in the video?
-The key elements of the projectile motion equation used in the video are the initial velocity (u), the acceleration due to gravity (a), the time of flight (t), and the displacement or distance traveled (s). These elements are combined in the Suvat equations to calculate the projectile's motion.
What is the maximum height reached by the projectile in the example given?
-In the example provided, the projectile reaches a maximum height of approximately 1146.79 meters, or about 3,000 feet.
How does the time it takes for the projectile to reach its maximum height relate to the total time of flight?
-The time it takes for the projectile to reach its maximum height is approximately 15.3 seconds, which is also the time it takes to travel the same distance back down to the ground. Thus, the total time of flight is about 30.6 seconds.
What is the total range of the projectile in the ideal physics world scenario?
-In the ideal physics world scenario, the total range of the projectile is approximately 7,200 meters, or about 8 kilometers.
Why is the height reached by the projectile significant in a battlefield context?
-The height reached by the projectile is significant in a battlefield context because it can affect the use of airspace by other military assets, such as helicopters. Ensuring that projectiles do not intersect with the flight paths of helicopters is crucial for safety and operational efficiency.
How does the video's explanation of projectile motion apply to non-military scenarios?
-The principles of projectile motion explained in the video apply to non-military scenarios as well, such as sports or any situation where an object is thrown or launched into the air. The same physics govern the motion regardless of the context.

Outlines

00:00

💥 Direct and Indirect Fire Weapons

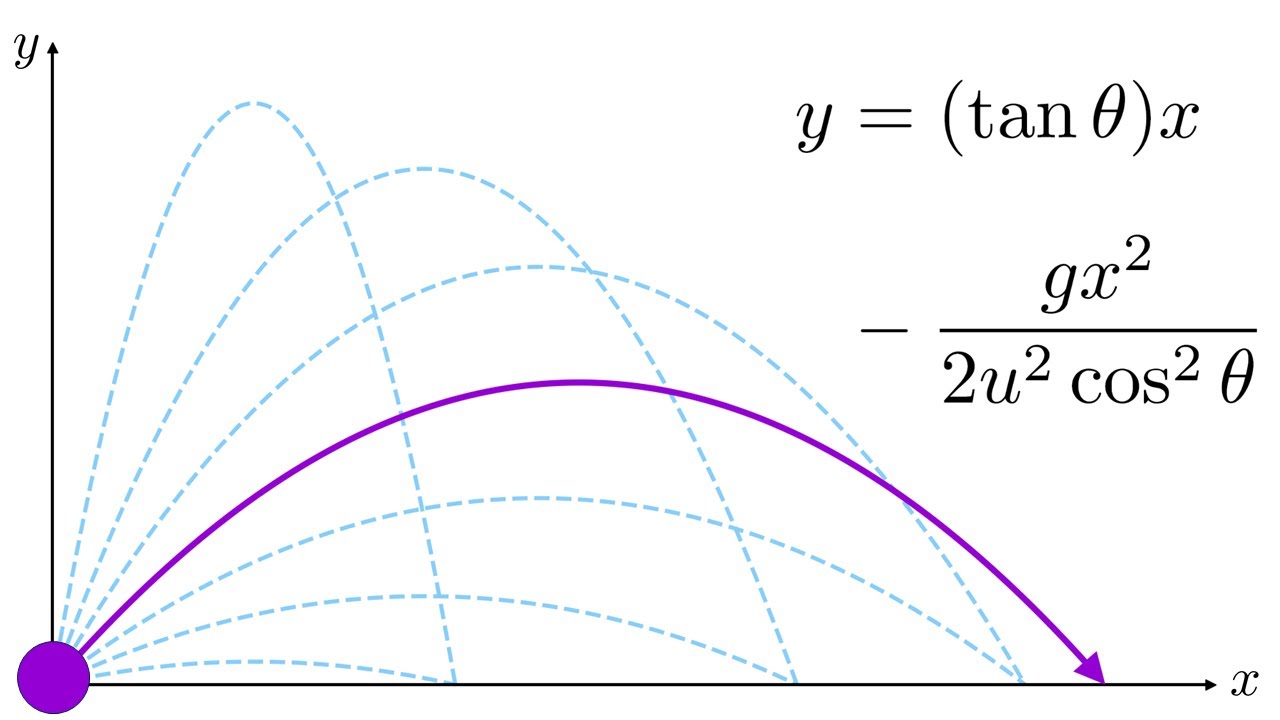
This paragraph discusses projectile motion in the context of direct and indirect fire weapons. The speaker begins by reviewing the concept of projectile motion from the previous video, specifically its application in direct fire weapons like rifles and armored vehicles. They then transition to indirect fire, highlighting the role of artillery guns, which are designed to fire at high angles over long distances. The speaker uses the example of a light gun, describing its versatility and historical use in conflicts. They introduce the concept of an ideal physics world without air resistance to simplify the analysis of projectile motion, focusing on a projectile's initial horizontal and vertical velocities and its parabolic trajectory. The speaker outlines the use of Suvat equations to calculate the projectile's path, emphasizing the importance of considering various real-world factors like wind, air temperature, and the Earth's rotation in actual artillery fire.

05:00

🚀 Projectile Motion Analysis

In this paragraph, the speaker delves into the specifics of projectile motion, using the example of a light gun firing a projectile. They calculate the projectile's initial velocity in both horizontal and vertical directions, considering the effects of a 30-degree angle and an initial speed of 300 meters per second. The speaker employs the Suvat equations to determine the projectile's maximum height and the time it takes to reach this point. They also calculate the distance traveled during the first half of the projectile's flight. The discussion emphasizes the practical implications of understanding projectile motion, such as ensuring safety in airspace and the importance of considering various factors like wind and temperature in real-world scenarios.

10:01

📈 Breakdown of Projectile's Trajectory

The speaker concludes the video script by summarizing the projectile's trajectory, highlighting the key points discussed in the previous paragraphs. They reiterate the separation of the projectile's path into two halves, with the first half focusing on the vertical and horizontal motion and the second half mirroring the first in terms of distance and time. The speaker emphasizes the projectile's constant horizontal velocity and the changes in vertical velocity throughout its flight. They also reflect on the complexity of calculating real-world projectile motion, acknowledging the simplifications made in the ideal physics world presented in the video. The speaker's analysis underscores the importance of understanding these principles for various applications, not limited to military scenarios.

Mindmap

Keywords

💡Projectile Motion

Projectile motion refers to the movement of an object that is launched into the air and is influenced only by gravity. In the video, this concept is central to understanding how direct and indirect fire weapons, such as rifles and artillery, operate. The script discusses how a bullet or projectile moves initially horizontally and then becomes more vertically influenced as it travels, illustrating the parabolic trajectory of projectile motion.

💡Direct Fire

Direct fire refers to a type of weapon engagement where the weapon is aimed and fired directly at the target. In the context of the video, this is exemplified by the use of rifles and certain armored vehicles like tanks, where the weapon is used to engage the enemy directly in line-of-sight engagements.

💡Indirect Fire

Indirect fire is a military term for firing a weapon in a high arc, targeting an area that is not directly visible to the gun or launcher. This is different from direct fire, as it does not require line-of-sight and is typically used for longer distances. In the video, indirect fire is associated with artillery, which is designed to fire at a high angle over long distances.

💡Artillery

Artillery refers to large-caliber weapons such as cannons, howitzers, and mortars, which are typically used for indirect fire. These weapons are designed to fire projectiles over long distances and at high angles to reach targets that are not in direct line-of-sight. In the video, artillery is contrasted with direct fire weapons and is associated with the use of complex calculations to account for various factors like wind, air temperature, and projectile weight.

💡Trajectory

Trajectory is the path followed by an object in motion through space. In the context of the video, it specifically refers to the parabolic path that a projectile follows due to the influence of gravity. The trajectory is divided into two halves for the purpose of simplifying calculations and understanding the motion of the projectile.

💡Acceleration

Acceleration is the rate of change of velocity of an object with respect to time. In the video, it is used to describe the constant acceleration due to gravity that affects the projectile's vertical motion. Horizontal acceleration is considered negligible in an ideal physics world without air resistance.

💡Air Resistance

Air resistance, also known as drag, is the force that opposes the motion of an object through the air. In the video, it is mentioned as a factor that is ignored in the ideal physics world scenario but is crucial in real-world projectile motion calculations. Air resistance affects the trajectory, range, and speed of a projectile.

💡Range

Range in the context of the video refers to the horizontal distance that a projectile can travel from the point of firing to the point of impact. It is a critical factor in determining the effectiveness of a weapon system, as it indicates the maximum distance a weapon can engage targets.

💡Time of Flight

Time of flight is the total time a projectile is in the air from the moment it is launched until it hits the ground or the target. It is an important parameter in projectile motion analysis, as it affects the planning and execution of fire missions in military contexts.

💡SUVA Equations

SUVA equations are a set of kinematic equations used to describe the motion of an object under constant acceleration. In the video, these equations are used to calculate the horizontal and vertical components of the projectile's motion, assuming no air resistance. The equations help in determining the distance traveled, the height reached, and the time of flight.

💡Parabolic Trajectory

A parabolic trajectory is the path followed by a projectile under the influence of gravity when launched into a uniform gravitational field. It is characterized by a symmetrical arc shape. In the video, the parabolic trajectory is the result of the projectile's motion, which initially moves horizontally and then is increasingly affected by gravity, causing it to follow a curved path to the ground.

Highlights

Projectile motion is explored in the context of direct fire weapons, such as those used in armored vehicles and rifles.

The video focuses on indirect fire, a technique utilized by artillery guns which are not designed for direct confrontation with the enemy.

Artillery guns are designed to fire at a high angle over long distances, utilizing the projectile's initial upward movement.

The video uses the example of a light gun, which has been used in various conflicts, including the Falkland Islands, Iraq, and Afghanistan.

In an ideal physics world without air resistance, Sievert equations can be applied to understand projectile motion.

Real-life artillery must account for factors like wind, air temperature, and the rotation of the Earth.

The projectile's initial velocity is calculated to be 259.81 m/s when fired at an angle of 30 degrees with a speed of 300 m/s.

The maximum height reached by the projectile is determined to be approximately 1146.79 meters.

The time taken for the projectile to reach its maximum height is about 15.29 seconds.

The first half of the projectile's journey covers a distance of approximately 3772.6 meters.

Since the projectile's time to ascend and descend are equal, the total distance covered in the second half of the journey is the same as the first.

The total range of the projectile is roughly 8 kilometers, with a total time of flight of about 31 seconds.

The principles of projectile motion apply to various scenarios, not just military, such as balls being thrown or water flowing from a hose.

The video breaks down the projectile's journey into two halves, analyzing the motion both horizontally and vertically.

The importance of considering the projectile's maximum height for deconflicting airspace on the battlefield is discussed.

Transcripts

Browse More Related Video

Time of Flight in Projectile Motion | Physics

Trajectory of a projectile without drag

Projectile at an angle | Two-dimensional motion | Physics | Khan Academy

Consolidation: Projectile Motion Calculations

PROJECTILE MOTION RELATED DERIVATIONS | TIME OF FLIGHT | HORIZONTAL RANGE | MAXIMUM HEIGHT | KANNADA

Projectile Motion Maximum Height Formula Derivation

Projectile Motion (of an Object Fired at an Angle) - A Level Physics

Takeaways

Q & A

What type of projectile motion is discussed in the video?

How does the video differentiate between direct fire and indirect fire weapons?

What factors are ignored in the ideal physics world example provided in the video?

What is the significance of the projectile's angle and initial velocity in determining its trajectory?

How does the absence of air resistance affect the projectile motion calculations?

What are the key elements of the projectile motion equation used in the video?

What is the maximum height reached by the projectile in the example given?

How does the time it takes for the projectile to reach its maximum height relate to the total time of flight?

What is the total range of the projectile in the ideal physics world scenario?

Why is the height reached by the projectile significant in a battlefield context?

How does the video's explanation of projectile motion apply to non-military scenarios?