How To Find The Derivative of a Fraction - Calculus

The Organic Chemistry Tutor
27 Jun 202014:37
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on calculating the derivatives of fractions, emphasizing the importance of understanding basic rules like the power rule. It explains the process with clear examples, demonstrating how to handle variables in both numerators and denominators, and how to deal with constants. The video also covers special cases, such as derivatives involving square roots, and introduces the quotient rule for more complex fractions. By walking through each step methodically, the script aims to solidify the viewer's grasp on differential calculus.

Takeaways
  • 📚 The power rule states that the derivative of x raised to a constant n is n times x to the power of n-1.
  • 🌟 Examples: The derivative of x^2 is 2x, x^3 is 3x^2, and x^4 is 4x^3, following the pattern of moving the exponent and subtracting one.
  • 🔢 Constants in front of variables can be pulled out, such as the derivative of 8x^9 is 8 times the derivative of x^9, which is 9x^8.
  • 📈 The derivative of a constant is zero, so the derivative of -6 would be 0.
  • 🤔 When finding the derivative of a fraction, it's important to rewrite the fraction if necessary to make the process clearer.
  • 🔄 For fractions where the variable is in the denominator, rewrite the expression to isolate the variable using the power rule.
  • ⚙️ Negative exponents can be converted to positive by moving the variable to the denominator and changing the sign.
  • 🛠️ The quotient rule is used for more complex fractions, defined as (g * f' - f * g') / g^2, where f and g are the numerator and denominator expressions, respectively.
  • 📊 Practice problems are essential for understanding the process and applying the rules to find derivatives of various functions.
  • 📝 Simplification of the final result may involve combining like terms and checking for factorability, but not all expressions can be factored.
  • 🎓 Understanding the derivative of radicals, such as the square root of x (x^(1/2)), is crucial for working with more advanced functions.
Q & A

  • What is the power rule for finding the derivative of a variable raised to a constant?

    -The power rule states that the derivative of a variable x raised to a constant n is n times x raised to the power of n minus 1, or in formula terms, (d/dx)[x^n] = n * x^{n-1}.

  • How do you find the derivative of x squared?

    -The derivative of x squared (x^2) is found by applying the power rule. You move the exponent (2) to the front and subtract it by one, resulting in 2 times x raised to the first power, or (d/dx)[x^2] = 2x.

  • What is the derivative of x cubed?

    -Using the power rule, the derivative of x cubed (x^3) is 3 times x squared (x^2), which is expressed as (d/dx)[x^3] = 3x^2.

  • How do you find the derivative of a constant multiplied by a variable?

    -The derivative of a constant multiplied by a variable is the constant multiplied by the derivative of the variable. For example, the derivative of 8x^9 is 72x^8, as 8 times the derivative of x^9 (which is 9x^8) equals 72x^8.

  • What is the derivative of a constant?

    -The derivative of a constant is zero. This is because a constant does not change with respect to the variable, so its rate of change is zero.

  • How do you find the derivative of a fraction where the variable is in the numerator?

    -To find the derivative of a fraction with the variable in the numerator, you multiply the constant in the denominator by the derivative of the numerator. For example, the derivative of 4x/7 is 4/7 times the derivative of x, which is 1, resulting in 4/7.

  • What is the process for finding the derivative of a fraction where the variable is in the denominator?

    -When the variable is in the denominator, you first rewrite the expression by inverting the fraction and changing the exponent of the variable using the power rule. Then, you multiply the result by the negative coefficient of the exponent. Finally, you move the variable back to the bottom to get the final answer in the form of a fraction, such as in the example where the derivative of 8/x became -8/x^2.

  • How do you find the derivative of a square root in the numerator of a fraction?

    -To find the derivative of a square root in the numerator, you first rewrite the square root as the variable to the power of one-half. Then, apply the power rule by moving the exponent to the front and subtracting it by one. After that, you multiply by the coefficient of the new exponent and simplify the result, such as in the example where the derivative of √x/5 became 1/(10√x).

  • What is the quotient rule for finding the derivative of a fraction?

    -The quotient rule states that the derivative of a fraction f/g is equal to (g * f' - f * g') / g^2, where f' and g' are the derivatives of the numerator and denominator, respectively.

  • How do you simplify the result of applying the quotient rule?

    -After applying the quotient rule, you distribute and combine like terms, and if possible, factor the result to simplify it further. However, if the expression is not factorable, you leave it in its most simplified form, as demonstrated in the example where the derivative of (5 + 3x) / (x^2 + 7) resulted in -3x^2 - 10x + 21 / (x^2 + 7)^2.

  • What is the final answer for the derivative of the fraction (5 + 3x) / (x^2 + 7)?

    -The final answer for the derivative of the fraction (5 + 3x) / (x^2 + 7) is -3x^2 - 10x + 21 / (x^2 + 7)^2, after applying the quotient rule and simplifying the result.

Outlines
00:00
📚 Understanding Basic Derivative Rules

This paragraph introduces the foundational rules for calculating derivatives, emphasizing the power rule. It explains how to find the derivative of a variable raised to a constant, using the formula n × x^{n-1}, where x is the variable and n is the constant. The explanation is supported by examples, such as finding the derivatives of x^2, x^3, and x^4, and extends to include the impact of constants on the derivative. It also touches on the derivative of a constant being zero and sets the stage for finding derivatives of fractions.

05:00
📈 Deriving Fractions with Variable in Numerator

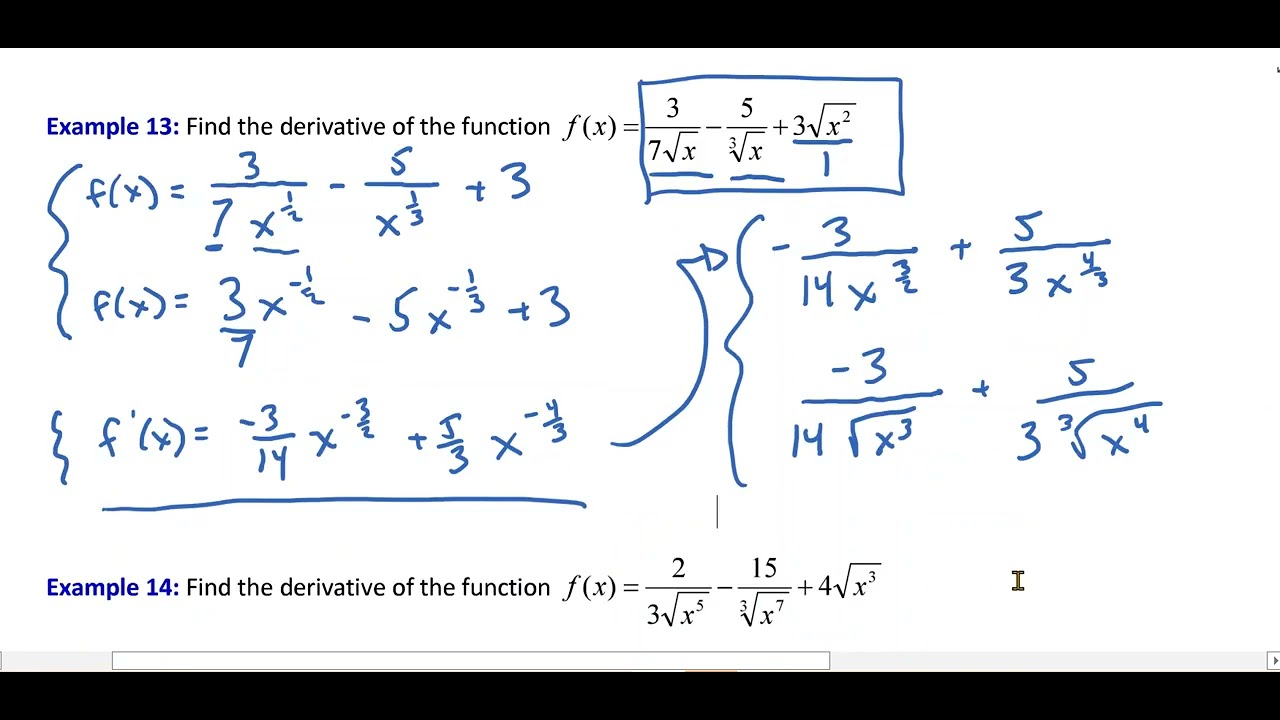
This section focuses on finding the derivative of fractions where the variable is in the numerator. It demonstrates the process of rewriting the fraction and applying the power rule to find the derivative. The examples provided cover a range of scenarios, including fractions with constants in the numerator and variable in the denominator, such as ¾7/x^2¾ and ¾8/x^3¾, and their derivatives are calculated accordingly. The explanation also addresses the concept of negative exponents and how to convert them into positive exponents by moving the variable to the denominator.

10:02
🌟 Advanced Derivative Calculations

This paragraph delves into more complex derivative calculations, including fractions with the variable in the denominator and irrational expressions in the numerator. It explains how to handle square roots and other radicals by rewriting expressions and applying the power rule. The examples given illustrate the process of finding derivatives for expressions like ¾sqrt(x)¾ and ¾7/sqrt(x)¾, emphasizing the conversion of negative exponents and the use of rationalizing techniques. The paragraph concludes with the application of the quotient rule for a more complicated fraction, providing a step-by-step breakdown of the process.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the video, it is used to describe how to calculate the slope of a curve at any given point, which is essential for understanding the behavior of functions. For instance, the derivative of x squared is 2x, indicating that the rate of change of x squared with respect to x is twice the value of x.
💡power rule
The power rule is a basic formula in calculus that allows us to find the derivative of a function where the variable is raised to a constant power. It states that the derivative of x^n, where n is a constant, is n*x^(n-1). This rule is crucial for differentiating polynomial functions and is a key concept covered in the video.
💡constant
In the context of calculus, a constant is a value that does not change. The derivative of a constant is always zero because there is no rate of change associated with a non-changing value. This concept is fundamental in the video as it simplifies the process of differentiating functions by allowing us to ignore constants when calculating derivatives.
💡fraction
A fraction in mathematics is a way of representing a number as the ratio of two integers. In the context of the video, fractions are used to express the division of two functions, and finding their derivatives involves specific rules and techniques, such as the quotient rule.
💡quotient rule
The quotient rule is a mathematical technique used to find the derivative of a quotient of two functions. It states that the derivative of a function f(x) divided by g(x) is equal to (g*f' - f*g') / (g^2), where f' and g' are the derivatives of f and g, respectively. This rule is essential for more complex differentiation problems involving fractions and is thoroughly explained in the video.
💡variable
In mathematics and calculus, a variable is a symbol, often a letter like x or y, that represents an unknown quantity or a value that can change. Variables are the core of functions and equations, and understanding their behavior is crucial for solving mathematical problems.
💡exponent
An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. In the context of the video, the exponent is used to define the power to which a variable is raised, and understanding exponents is essential for applying the power rule to find derivatives.
💡simplify
Simplification in mathematics refers to the process of making a mathematical expression or equation easier to understand or calculate by reducing it to a more straightforward form. In the context of the video, simplification is important for making the derived expressions more comprehensible and manageable.
💡product rule
The product rule is a fundamental calculus rule used to find the derivative of a product of two functions. It states that the derivative of f(x)g(x) is f'(x)g(x) + f(x)g'(x). Although not explicitly used in the video, it is a related concept to the quotient rule and is essential for more complex differentiation problems.
💡rationalize
Rationalization is the process of eliminating a radical, usually a square root, from a denominator or a numerator in a fraction. This is done to simplify expressions and make them easier to work with, especially when dealing with square roots.
Highlights

Introduction to finding the derivative of a fraction.

Review of basic rules such as the power rule for derivatives.

Derivative of a variable raised to a constant power, expressed as n times x to the power n-1.

Example of finding the derivative of x squared, which results in 2x.

Derivative of x cubed is 3x squared, demonstrating the pattern of moving the exponent.

Explanation of how to find the derivative of a constant multiplied by a variable, such as 8x to the 9th power.

Derivative of a constant is zero, as shown by the example of the derivative of negative six.

Process for finding the derivative of a fraction by rewriting the expression.

Derivative of x over nine is one over nine, using the power rule for the numerator.

Derivative of a fraction with x in the denominator, such as 8 over x, results in negative eight over x squared.

Derivative of a fraction with a square root in the numerator, like the square root of x over five.

Derivative of 7 divided by the square root of x, using the power rule and converting to radical form.

Explanation of the quotient rule for finding the derivative of a complex fraction, such as (5 + 3x) / (x squared + 7).

Step-by-step calculation of the derivative of the complex fraction, resulting in -3x squared - 10x + 21 over x squared + 7 squared.

Conclusion of the video, summarizing the process of finding the derivative of a fraction.

Transcripts

Browse More Related Video

Implicit Differentiation Second Derivative Trig Functions & Examples- Calculus

CALCULUS 1: DERIVATIVES

Derivatives of Rational Functions

The Constant Multiple Rule For Derivatives

Partial Derivatives - Multivariable Calculus

Basic Derivative Rules - Part 2: Combining the Rules

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativeCalculusFractionDerivativesPowerRuleQuotientRuleMathematicsEducationOnlineLearningClearExamplesBasicRulesConstantDerivativeVariableExponents