Limits and Derivatives
TLDRThe video script discusses the concept of limits and derivatives in calculus. It explains how to evaluate expressions that represent the definition of a derivative by recognizing the function and applying the rules of limits. The script provides examples of finding derivatives of natural logarithm, sine, and tangent functions, demonstrating the process of evaluating limits using the concept of derivatives. The examples are worked out step by step, showing how to arrive at the derivative of each function, which is the value of the limit in each case.
Takeaways
- 📚 The concept of limits is introduced as a fundamental part of calculus, specifically in finding derivatives.
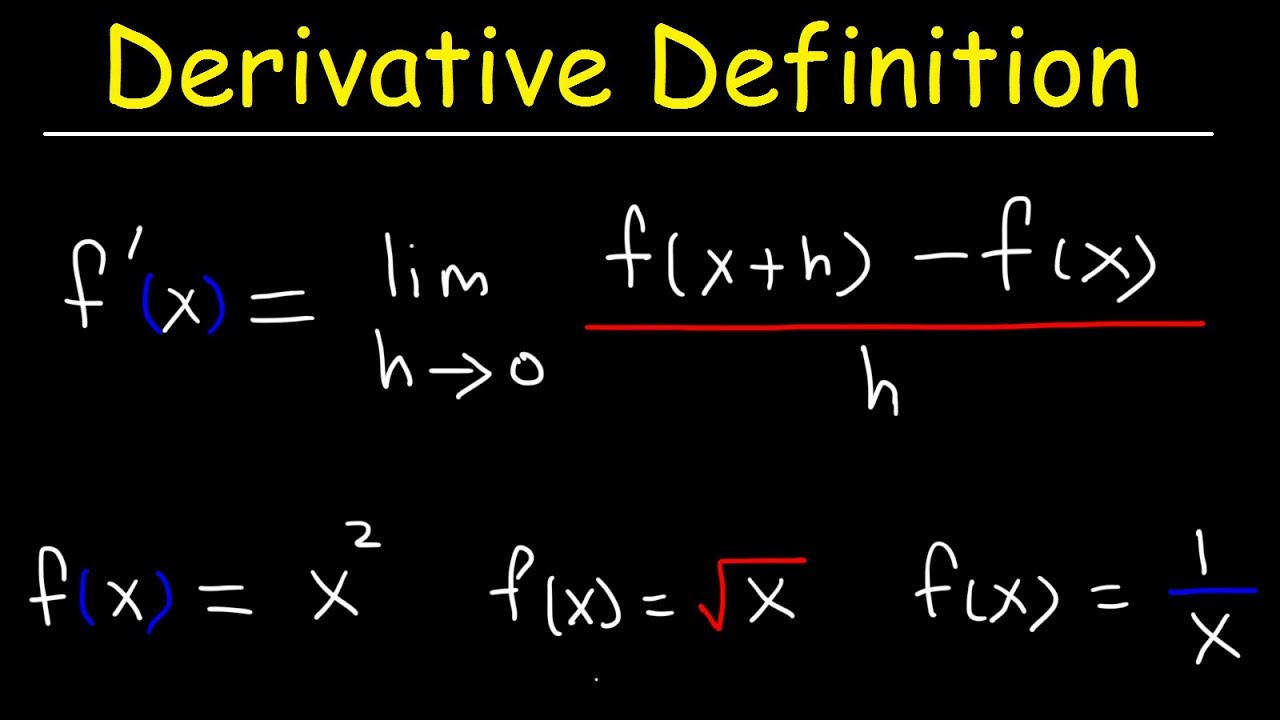
- 🔍 The formula for the derivative is explained as the limit of (f(x+h) - f(x))/h as h approaches 0.
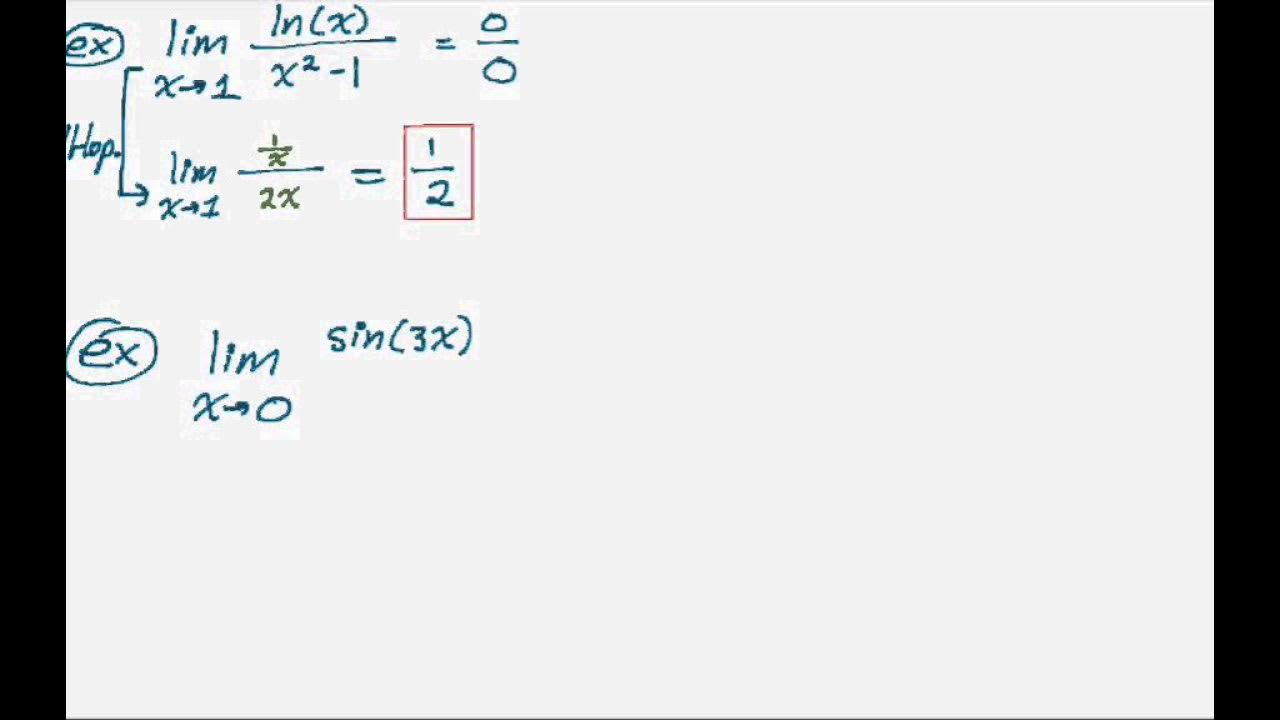
- 🌟 The first example demonstrates evaluating the derivative of the natural logarithm function, ln(x), which is 1/x.
- 📈 The second example covers the derivative of the sine function, showing that the derivative is cosine.
- 🤔 The third problem involves the alternative definition of the derivative, focusing on the limit as x approaches a specific value (a).
- 👉 The derivative of the tangent function is identified as secant squared, leading to the solution of the third problem.
- 📌 The importance of recognizing the structure of expressions in relation to the definition of derivatives is emphasized.
- 🧮 The script provides a step-by-step approach to solving limit problems, starting with identifying the function (f(x)) and the point (a).
- 🌐 The application of trigonometric identities, such as the cosine of 30 degrees, is demonstrated in the process of solving the problems.
- 📊 The process illustrates the practical use of derivatives in analyzing functions and their behavior near specific points.
- 🎓 The script serves as an educational resource for understanding and applying the concept of limits in calculus.
Q & A
What is the limit expression given in the transcript for the first problem?
-The limit expression is the limit as h approaches 0, of (ln x + h) - ln x divided by h.
How is this expression related to the concept of a derivative?
-The expression is related to the derivative because it represents the definition of the derivative of a function f at a point x, which is f'(x) = lim(h->0) [(f(x+h) - f(x))/h].
What function is being differentiated in the first problem of the transcript?
-The function being differentiated is ln x, as indicated by the expression ln x + h - ln x over h.
What is the derivative of ln x?
-The derivative of ln x is 1/x.
In the second problem, what is the limit expression for the sine function?
-The limit expression for the sine function is the limit as h approaches 0, of (sin(x+h) - sin x) divided by h.
What is the derivative of the sine function?
-The derivative of the sine function is the cosine function, so the limit expression evaluates to cos x.
What is the alternative definition of the derivative mentioned in the transcript?
-The alternative definition of the derivative is f'(a) = lim(x->a) [f(x) - f(a)] / (x - a).
What function and point are being considered in the third problem?
-The third problem considers the function f(x) = tan x and the point a = pi/6.
What is the derivative of the tangent function?
-The derivative of the tangent function is the secant squared function, denoted as (sec x)^2.
What is the value of the secant squared at pi/6?
-The secant squared at pi/6 is 4/3, since secant is 1/cosine and cos(pi/6) is the square root of 3 over 2.
What is the final answer to the third problem?
-The final answer to the third problem is 4/3, which represents the value of the limit as x approaches pi/6 for the expression (tan x - tan(pi/6)) / (x - pi/6).
Outlines
📚 Introduction to Evaluating Limits and Derivatives
This paragraph introduces the concept of limits and derivatives in calculus. It explains how to evaluate the limit of a function as a variable approaches a specific value by associating the expression with the definition of the derivative. The paragraph provides an example of finding the derivative of the natural logarithm function (ln x) and demonstrates that the derivative is equal to 1/x. It also explains the process of evaluating limits for trigonometric functions, such as the sine function, by identifying their derivatives. The paragraph emphasizes the importance of recognizing the formula for the expression and applying the definition of the derivative to find the value of the limit.
🔢 Solving Limits of Trigonometric Functions
This paragraph focuses on solving limits involving trigonometric functions, specifically the sine and tangent functions. It begins by calculating the derivative of the sine function and applying it to evaluate a limit as h approaches zero. The paragraph then moves on to a more complex problem involving the tangent function and the alternative definition of the derivative. It explains the need to find the derivative of the tangent function, which is the secant squared, and applies this to evaluate a limit as x approaches pi/6. The paragraph concludes with the calculation of the secant squared of pi/6, which is found to be 4/3, by using the cosine of 30 degrees.
Mindmap
Keywords
💡Limit
💡Derivative
💡Function
💡ln(x)
💡Sine(x)
💡Tangent(x)
💡Cosine(x)
💡Secant squared (x)
💡Rate of change
💡Trigonometric functions
Highlights
The limit as h approaches 0 of (ln x + h - ln x) / h is essentially the definition of the derivative.
The function f(x) in this context is ln x, which is crucial to identify to find the limit.
The derivative of ln x is 1/x, which is the value the original expression simplifies to.
The second example involves the limit as h approaches 0 of (sin(x + h) - sin x) / h, which is another application of the derivative definition.
The function f(x) in the second example is sin x, which is needed to determine the limit.
The derivative of sin x is cos x, which is the value the second expression evaluates to.
The third problem involves the limit as x approaches pi/6 of (tan x - tan(pi/6)) / (x - pi/6), which is different from the previous examples.
This problem uses the alternative definition of the derivative, which is key to solving it.
The function f(x) in the third problem is tan x, which is essential for finding the derivative.
The derivative of tan x is sec(x)^2, which is needed to find the value of the limit.
The value of sec(pi/6) is 1 / cos^2(pi/6), which is important for calculating the final answer.
Cosine of pi/6 or 30 degrees is equal to the square root of three over 2.
The final answer is 4/3, which is derived from the squared value of the cosine of pi/6.
This transcript provides a clear and detailed explanation of how to evaluate limits using the definition of derivatives.
The process of identifying the function f(x) and its derivative is crucial for solving these types of limits.
The transcript demonstrates the application of the derivative definition in various scenarios, enhancing understanding of calculus concepts.
The use of trigonometric function derivatives, such as cosine for sin x, is well-explained and applied.
The transcript is a valuable resource for learning how to evaluate limits, especially those related to derivatives.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: