AP Physics Workbook 2.H Force on inclined Planes

Mr.S ClassRoom
23 Apr 202007:20
EducationalLearning
32 Likes 10 Comments

TLDRIn this segment of the AP Physics Workbook Solution, the focus is on determining the relationship between the normal force on a box and the angle of incline. The video explains how to draw a Freebody diagram and correctly identify the forces at play, including gravity, normal force, and friction. It then applies Newton's second law to derive an equation relating the normal force to the incline's angle. A linearization graph is introduced to visually represent the data, with the slope representing the force of gravity experienced by the box. The explanation is clear, engaging, and educational, providing a solid understanding of the physics concepts involved.

Takeaways
  • πŸ“š The topic is Dynamics, focusing on the forces acting on an object resting on an inclined plane.
  • 🎨 A Freebody diagram is essential for visualizing the forces involved, such as gravity, normal force, and friction.
  • πŸ”„ The force of gravity can be decomposed into two components: one parallel (x-axis) and one perpendicular (y-axis) to the incline.
  • βœ… The normal force is always perpendicular to the surface of the incline, not necessarily opposite to gravity.
  • πŸ“ Newton's second law is applied to derive the relationship between the normal force and the incline angle.
  • 🌐 The axes are chosen with the x-axis parallel to the incline and the y-axis perpendicular to it.
  • πŸ”’ The vertical component of gravity is given by \( mg \cos \theta \) and the horizontal component by \( mg \sin \theta \).
  • πŸ“‰ The graph of normal force vs. angle initially shows a curve, which needs to be linearized for analysis.
  • πŸ“ˆ Linearization is achieved by plotting the normal force against the cosine of the angle of inclination.
  • πŸ”„ The slope of the linearized graph represents the change in the cosine of the angle (Y) over the change in the normal force (X).
  • 🌍 The physical meaning of the slope is the force of gravity experienced by the box, reflecting the Earth's gravitational pull on it.
Q & A

  • What is the scenario described in the video script?

    -The scenario involves Angelica and Carlos determining the relationship between the normal force on a box of mass m and the angle of incline ΞΈ while the box is at rest on an inclined plane.

  • What is the first task in the video script?

    -The first task is to draw the Freebody diagram for the scenario.

  • Why is the assumption that the normal force is the opposite of the force of gravity incorrect?

    -The normal force is always perpendicular to the surface, not necessarily the opposite of the force of gravity.

  • How is the force of friction related to the motion of the box?

    -The force of friction is opposite to the motion of the box. If the box were to slide down, the frictional force would act up the ramp.

  • What are the two components of the force of gravity according to the video script?

    -The two components of the force of gravity are the force of gravity in the X direction (mg sine ΞΈ) and the force of gravity in the Y direction (minus mg cosine ΞΈ).

  • How does Newton's second law apply to this scenario?

    -Newton's second law is used to derive an equation that relates the normal force with the angle of the incline, considering the box is not accelerating.

  • What does the normal force FN equal when the box is not accelerating?

    -When the box is not accelerating, the normal force FN equals the vertical component of the gravitational force, which is mg cosine ΞΈ.

  • How does the video script suggest graphing the relationship between normal force and the angle of incline?

    -The script suggests graphing the normal force on the x-axis and the angle on the y-axis, then linearizing the graph by plotting the normal force against the cosine of the incline angle.

  • What does the slope of the linearized graph represent?

    -The slope of the linearized graph represents the force of gravity experienced by the box, which is the weight the box feels as a result of the Earth pulling it down.

  • What is the significance of linearizing the graph in this context?

    -Linearizing the graph helps to clearly see the relationship between the normal force and the cosine of the angle of incline, making it easier to understand and analyze the data.

  • What is the final equation derived from the analysis in the video script?

    -The final equation derived is FN = mg cosine ΞΈ, which relates the normal force to the gravitational force experienced by the box at different angles of incline.

Outlines
00:00
πŸ“š Introduction to Dynamics: Force on an Inclined Plane

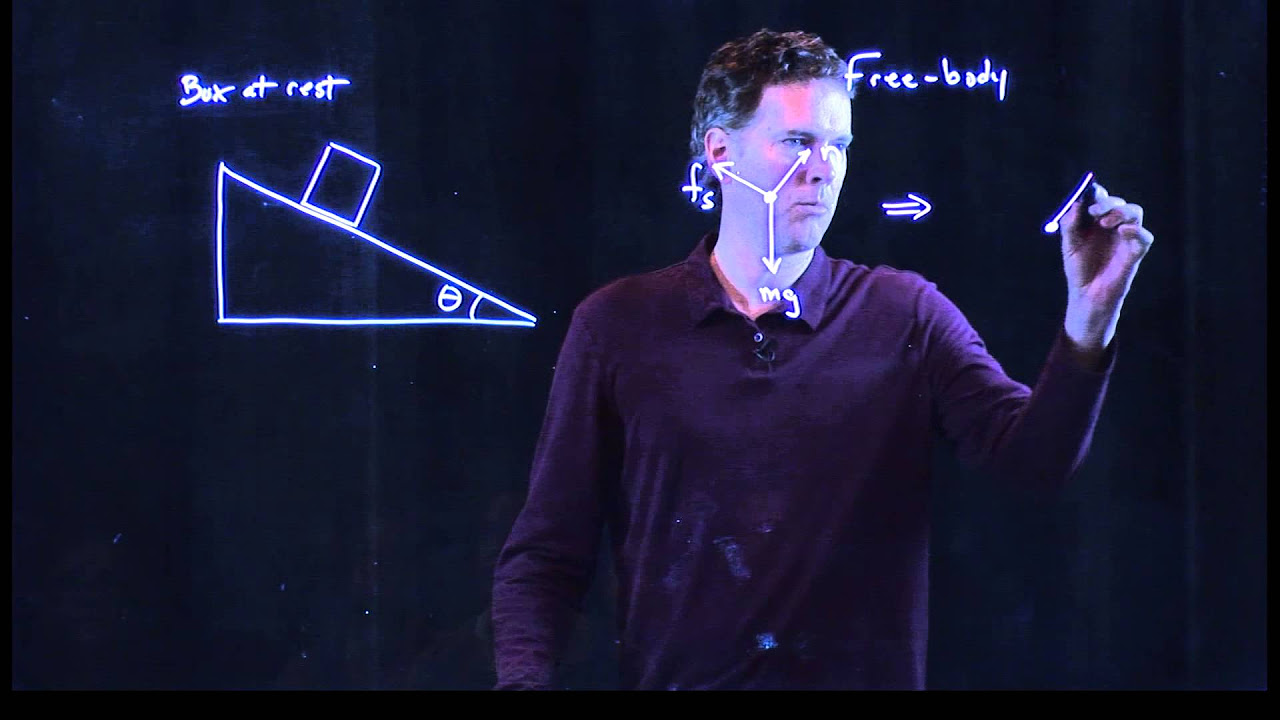
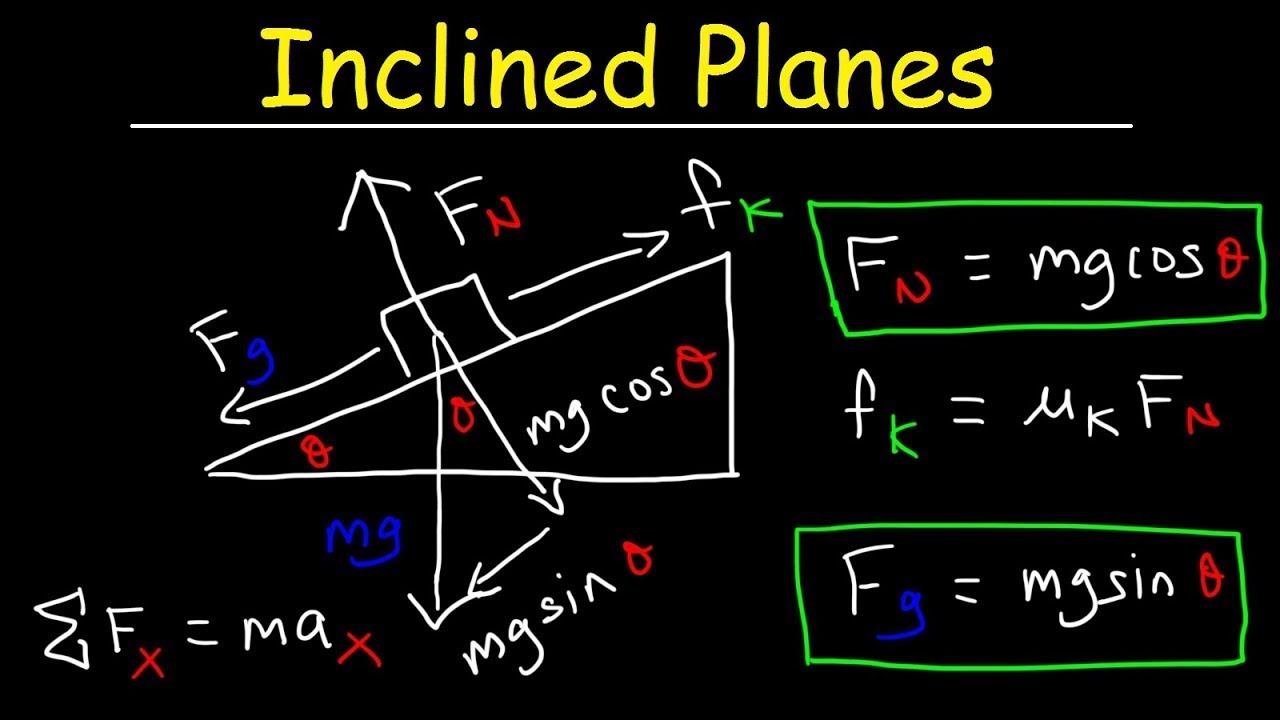
This paragraph introduces the topic of dynamics, specifically focusing on the force on an inclined plane (section 2.8). It presents a scenario where two characters, Angelica and Carlos, are tasked with understanding the relationship between the normal force on a box of mass 'm' and the angle of incline 'ΞΈ'. The discussion emphasizes the importance of correctly drawing a Freebody diagram and understanding the direction of the normal force and frictional force. It also introduces the concept of breaking down the force of gravity into components and applying Newton's second law to derive an equation relating the normal force to the incline angle. The paragraph concludes with a mention of creating a linearization graph to further analyze the relationship.

05:03
πŸ“ˆ Linearization and Interpretation of Forces

This paragraph delves into the process of linearization to better understand the relationship between the normal force and the angle of inclination. It explains how to graph the normal force against the cosine of the incline angle and emphasizes the importance of using degrees in the calculations. The paragraph highlights the physical meaning of the slope in the linearized graph, which represents the force of gravity experienced by the box. It clarifies that the slope is not a force produced by the box but rather the weight the box feels due to the Earth's gravitational pull. The summary concludes with a reiteration of the solutions for the given problem, reinforcing the understanding of the concepts discussed.

Mindmap
Keywords
πŸ’‘Freebody diagram
A freebody diagram is a graphical representation that shows all the forces acting on an object when it is in equilibrium or in motion. In the context of the video, it is used to analyze the forces acting on a box resting on an inclined plane, such as gravity, normal force, and friction. The diagram helps visualize the components of the gravitational force parallel and perpendicular to the ramp's surface.
πŸ’‘Normal force
The normal force is the force exerted by a surface as it supports the weight of an object resting on it. It is always perpendicular to the surface. In the video, the normal force acts on the box to counteract the component of gravitational force perpendicular to the inclined plane, ensuring the box remains in place and does not sink into the surface.
πŸ’‘Inclined plane
An inclined plane is a flat surface that is tilted at an angle to the horizontal. In physics problems, it is often used to study the effects of gravity and other forces on objects as they rest on or move along the slope. The angle of the incline, denoted by theta in the video, is a crucial parameter that affects the forces acting on the object.
πŸ’‘Friction
Friction is a force that opposes the relative motion or tendency of such motion of two surfaces in contact. In the context of the video, friction acts on the box to prevent it from sliding down the inclined plane. The direction of the frictional force is opposite to the potential motion of the box, which would be sliding down the ramp if not for the frictional force.
πŸ’‘Gravity
Gravity is the force that attracts two bodies towards each other, typically the force of attraction between the Earth and objects on or near its surface. In the video, the force of gravity acts vertically downward and is responsible for the weight of the box. The gravitational force is decomposed into two components: one parallel to the inclined plane (x-direction) and one perpendicular to it (y-direction).
πŸ’‘Newton's second law
Newton's second law of motion states that the acceleration of an object is directly proportional to the net force acting upon it and inversely proportional to its mass. It is often written as F = ma, where F is the net force, m is the mass, and a is the acceleration. In the video, this law is applied to derive the relationship between the normal force and the angle of the incline.
πŸ’‘Component of force
A component of a force is a part of a larger force that acts in a specific direction. In physics, forces are often broken down into their components to analyze their effects more easily along different axes. In the video, the force of gravity is decomposed into two components: one along the incline and one perpendicular to it.
πŸ’‘Equilibrium
Equilibrium in physics refers to a state where all forces acting on an object cancel each other out, resulting in no acceleration. In the video, the box is in equilibrium on the inclined plane, meaning the net force acting on it is zero, and thus the forces (normal force, gravitational force, and frictional force) are balanced.
πŸ’‘Linearization
Linearization is the process of converting a non-linear relationship into a linear one for easier analysis or interpretation. In the context of the video, the speaker linearizes the relationship between the normal force and the cosine of the incline's angle by plotting the cosine values against the normal force.
πŸ’‘Slope
In the context of a graph, the slope represents the rate of change between two variables, typically calculated as the change in the dependent variable (Y) divided by the change in the independent variable (X). In the video, the slope of the linearized graph represents the change in the force of gravity experienced by the box (Y) with respect to the change in the normal force (X).
πŸ’‘Cosine
The cosine is a trigonometric function that relates the ratio of the adjacent side to the hypotenuse in a right-angled triangle. It is used in physics to describe angles in relation to a reference direction, often the horizontal or vertical axis. In the video, the cosine of the incline's angle is used to calculate the components of the gravitational force acting on the box.
Highlights

The scenario involves determining the relationship between the normal force on a box and the angle of incline.

The box is at rest on an inclined plane, which is a common problem in physics involving forces.

A Freebody diagram is used to visualize the forces acting on the box, including gravity, normal force, and friction.

The force of gravity is decomposed into two components: one parallel and one perpendicular to the inclined plane.

The normal force is always perpendicular to the surface on which the object rests, contrary to common misconceptions.

Newton's second law is applied to derive an equation relating the normal force with the angle of the incline.

The axes are chosen to align with the ramp for simplicity in applying Newton's second law.

The vertical component of gravity is balanced by the normal force when the box is at rest.

The normal force is isolated to find its relationship with the gravitational force and the incline angle.

A graph of normal force versus the angle of incline is suggested to visualize the relationship.

The graph initially shows a curvilinear relationship between normal force and the angle of incline.

Linearization of the graph is achieved by plotting the normal force against the cosine of the incline angle.

The slope of the linearized graph has a physical meaning representing the force of gravity experienced by the box.

The slope is not indicative of the force of gravity by the box, but rather the force experienced by the box due to Earth's gravity.

The problem-solving process integrates theoretical physics concepts with practical graphical analysis.

The solution provides a comprehensive understanding of forces at work on an object at rest on an inclined plane.

The method can be applied to a variety of physics problems involving inclined planes and static equilibrium.

The explanation corrects common misunderstandings about the direction and nature of the normal force.

Transcripts

Browse More Related Video

Free body diagram sine and cosine components

AP Physics Workbook 2.F Direction of Friction

Net Force Physics Problems With Frictional Force and Acceleration

Banked Turn with Friction - Physics of Speed Limits on Banked Curves

Introduction to Inclined Planes

How Many Different Types of Forces Are There In Physics?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsWorkbookInclinedPlaneForceAnalysisNormalForceGravityComponentsNewtonsLawFrictionForceLinearizationCosineGraphForceOfGravity