Z-Statistics vs. T-Statistics EXPLAINED in 4 Minutes

Ace Tutors
8 Apr 202104:08
EducationalLearning
32 Likes 10 Comments

TLDRThe video script by Mark from Ace Tutors elucidates the distinction between z-statistics and t-statistics, and their appropriate applications. It explains that z-scores are used for individual sample values when the population standard deviation is known, while t-statistics are employed when this standard deviation is unknown and replaced with the sample's standard deviation. The script emphasizes the increased uncertainty with smaller sample sizes, leading to more dispersed distributions, and how larger samples improve the accuracy of the t-statistic, approximating a normal distribution.

Takeaways
  • πŸ“š The main topic is the difference between z-statistics and t-statistics and their appropriate usage.
  • πŸ”’ A z-score (x - ΞΌ) / Οƒ is used for a single value in a sample to compare it to the sample size.
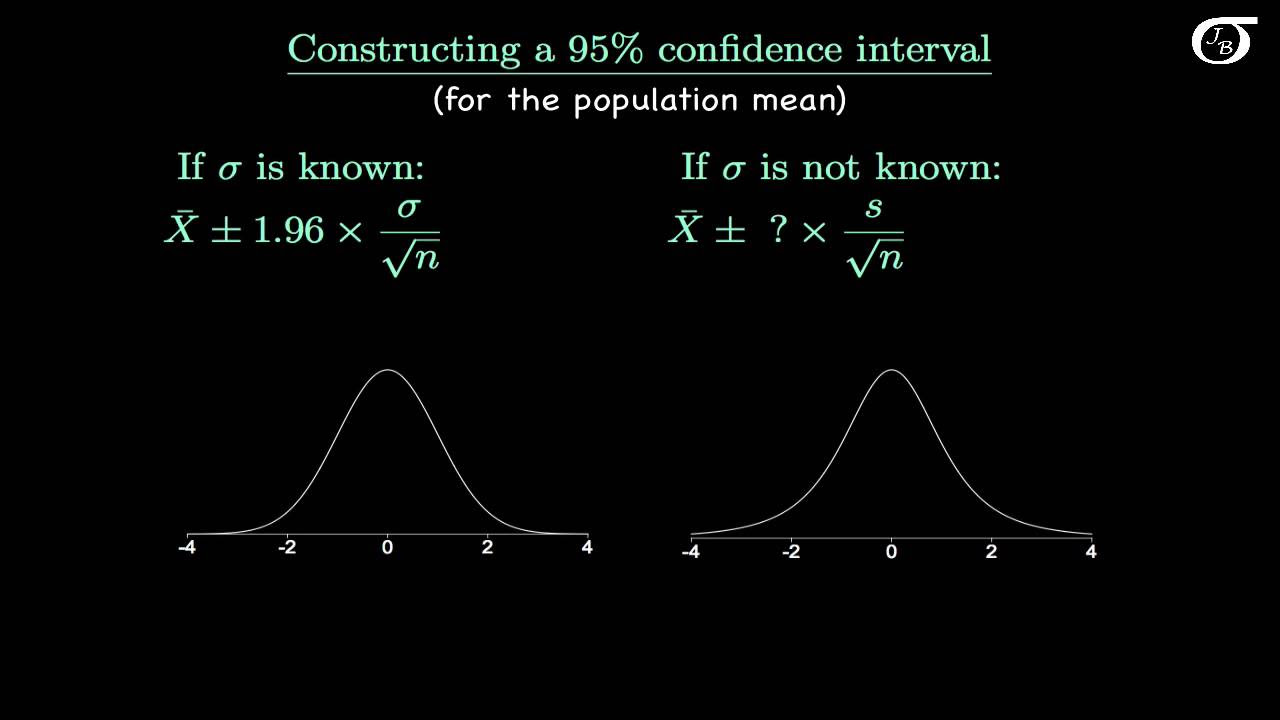
  • πŸ“ˆ A z-statistic (xΜ„ - ΞΌ) / (Οƒ / √n) is used for comparing an entire sample mean to the population mean when the population standard deviation is known.
  • πŸ€” The z-statistic formula requires knowledge of the population mean, sample size, and population standard deviation.
  • πŸ” When the population standard deviation is unknown, the t-statistic is used with the sample standard deviation as a replacement.
  • 🧐 The t-statistic accounts for the increased uncertainty from using a sample standard deviation instead of the population standard deviation.
  • πŸ“Š Smaller sample sizes lead to less confidence in the normal distribution and a more spread out distribution (higher t-value).
  • πŸ”„ As sample size increases, the sample standard deviation becomes a better estimate, and the distribution approaches a normal distribution.
  • πŸ“ The rule of thumb is to use z-statistics when the population standard deviation is known, and t-statistics when it is unknown.
  • 🌟 The video aims to clarify a common statistical concept to help students better understand and apply these tests.
  • πŸ’‘ Understanding the difference between z and t statistics is crucial for accurate statistical analysis and interpretation.
Q & A

  • What is the main difference between a z-score and a z-statistic?

    -A z-score is used for a single value in a sample (like a single grade) compared to the mean and standard deviation of that sample. A z-statistic, on the other hand, is used when comparing the mean of a sample to the mean of a larger population, taking into account the population standard deviation and the sample size.

  • Why might you use a t-statistic instead of a z-statistic?

    -You would use a t-statistic when the population standard deviation is unknown. Instead of using the population standard deviation, you replace it with the standard deviation of your sample in the calculation.

  • How does the sample size affect the accuracy of the t-statistic?

    -A smaller sample size means that the sample standard deviation is a less accurate estimate of the population standard deviation, leading to a distribution that is more spread out. As the sample size increases, the distribution becomes more like a normal distribution, increasing the confidence in the accuracy of the t-statistic.

  • What is the main rule of thumb for deciding between using z-statistics and t-statistics?

    -Use z-statistics when the population standard deviation is known, and t-statistics when it is unknown.

  • How does the normal distribution relate to the use of z-statistics?

    -Z-statistics are used with the assumption that the distribution of the data follows a normal distribution. This is why having the population standard deviation is crucial, as it defines the shape of the normal distribution.

  • What is the population standard deviation and why is it important in statistics?

    -The population standard deviation is a measure of the variability or spread of the entire population's data. It is important because it helps define the normal distribution and is a key component in calculating z-statistics.

  • What does the mean of a sample represent?

    -The mean of a sample (x-bar) represents the average value of the data points within that sample. It is used in calculations to compare the sample to the larger population.

  • Why is it impractical to find the population standard deviation in some cases?

    -It is impractical because it would require data from every single member of the population, which is often impossible or extremely difficult to obtain, such as in the case of all stat students in the world.

  • How does the accuracy of the sample standard deviation affect the shape of the distribution?

    -The less accurate the sample standard deviation, the more spread out the distribution will be, with more weight in the tails. This reflects a lower confidence in the normal distribution shape.

  • What is the significance of the sample size (n) in statistical analysis?

    -The sample size is significant because it affects the precision of estimates like the mean and standard deviation. A larger sample size generally leads to more reliable and generalizable results.

  • How can you increase confidence in your statistical analysis?

    -You can increase confidence by using larger sample sizes, which provide more accurate estimates of population parameters, and by using appropriate statistical methods for the data you have, such as using t-statistics when the population standard deviation is unknown.

Outlines
00:00
πŸ“š Introduction to Z and T Statistics

This paragraph introduces the topic of the video, which is the difference between Z and T statistics and their respective use cases. Mark from Ace Tutors explains that many students struggle with understanding these concepts. The paragraph sets the stage for a deeper dive into the distinction between a Z score, used for a single value in a sample, and a Z statistic, used for an entire sample in relation to a larger population. It also mentions the prerequisites for understanding Z statistics, including knowledge of the normal distribution.

Mindmap
Keywords
πŸ’‘Z statistics
Z statistics refer to a set of calculations used when comparing a sample mean to a population mean, assuming the population standard deviation is known. In the context of the video, Z statistics are used to analyze data from a sample in relation to a larger population, such as comparing the average grade of a statistics class to the global average for all statistics classes. The formula for Z statistics is Z = (x - ΞΌ) / (Οƒ / √n), where x is the sample mean, ΞΌ is the population mean, Οƒ is the population standard deviation, and n is the sample size.
πŸ’‘T statistics
T statistics are an alternative to Z statistics when the population standard deviation is unknown, and instead, the sample standard deviation is used. As explained in the video, this approach introduces a degree of uncertainty due to the estimation nature of the sample standard deviation. T statistics account for this uncertainty by adjusting the distribution to be less centralized and more spread out, particularly with smaller sample sizes. The video illustrates this by discussing how a sample of only two values would not provide a reliable estimate of the population standard deviation, thus affecting the accuracy of the T statistic.
πŸ’‘Normal distribution
The normal distribution is a probability distribution that is symmetric and bell-shaped, with the mean, median, and mode all being equal. In the video, it is mentioned that Z statistics rely on the assumption that the data follows a normal distribution. This is important because it allows for the use of Z scores and Z statistics to make inferences about the population from sample data. The video also touches on how the normal distribution's shape changes with sample size, becoming more accurate as the sample size increases.
πŸ’‘Sample mean (xΜ„)
The sample mean, denoted as xΜ„, is the average value of a specific set of data points within a sample. In the video, the sample mean is used to represent the average grade of a statistics class. It is compared to the population mean using Z and T statistics to determine how the sample data compares to the larger population context. The sample mean is a crucial component in statistical analysis as it provides a basis for making inferences about the population.
πŸ’‘Population mean (ΞΌ)
The population mean, symbolized by ΞΌ, is the average value of an entire population's data set. In the context of the video, the population mean is the average grade for all statistics classes across the world. It serves as a benchmark to compare against the sample mean. Understanding the population mean is essential for making accurate statistical inferences and is a fundamental concept in Z and T statistics.
πŸ’‘Standard deviation (Οƒ)
The standard deviation, represented by Οƒ, is a measure of the amount of variation or dispersion in a set of values. In the video, it is discussed that the population standard deviation is required for Z statistics, while the sample standard deviation is used for T statistics. The standard deviation is a key element in understanding the spread of data and is integral to both Z and T statistical calculations.
πŸ’‘Sample size (n)
The sample size, denoted as n, refers to the number of observations or individuals in a sample. In the video, the sample size is the number of students in a statistics class. It plays a significant role in statistical analysis as it affects the precision of the sample mean and the reliability of both Z and T statistics. A larger sample size generally leads to more accurate estimates and a distribution that better approximates the normal distribution.
πŸ’‘Z score
A Z score is a value that represents the number of standard deviations a given data point is from the mean of its distribution. In the video, the Z score is used to compare an individual grade to the average grade of the class. It is a crucial concept in understanding how individual data points relate to the overall distribution and is used in conjunction with Z statistics to analyze data.
πŸ’‘Estimation
Estimation in statistics refers to the process of using sample data to make inferences about the characteristics of a population. In the video, the use of T statistics involves estimation, as the population standard deviation is unknown and the sample standard deviation is used as an estimate. This introduces a level of uncertainty, which is accounted for in the calculation of T statistics, making them particularly useful when dealing with smaller sample sizes and unknown population parameters.
πŸ’‘Statistical inference
Statistical inference is the process of drawing conclusions about a population using data from a sample. The video discusses Z and T statistics as tools for making such inferences. By comparing the sample mean to the population mean, one can make predictions or estimations about the larger population. This is a fundamental concept in many areas of statistics and is the main goal of the analysis presented in the video.
πŸ’‘Distribution shape
The shape of a distribution refers to the pattern that data points form when graphed, such as the bell-shaped curve of a normal distribution. In the video, it is explained that the shape of the distribution changes depending on the sample size. With smaller sample sizes, the distribution is more spread out and less centralized, while larger sample sizes result in a distribution that more closely resembles the normal distribution. Understanding distribution shape is important for interpreting statistical results and making accurate inferences.
Highlights

The main topic is the difference between z-statistics and t-statistics and their usage.

Z-score is used for a single value in a sample compared to the sample size.

Z-statistic is used when examining an entire sample in relation to a larger population.

The formula for z-score is z = (x - mean) / standard deviation.

To use z-statistic, the population mean, sample mean, sample size, and population standard deviation are required.

T-statistic is used when the population standard deviation is unknown.

In place of the population standard deviation, the sample standard deviation can be used for calculating t-statistic.

Sample standard deviation is an estimate and introduces some margin of error.

Smaller sample sizes result in less confidence in the normal distribution of the data.

Larger sample sizes increase the confidence in the sample standard deviation and its resemblance to a normal distribution.

Use z-statistics when the population standard deviation is known; otherwise, use t-statistics.

The video aims to clarify a common point of confusion among students.

Understanding the difference between z and t statistics is crucial for accurate statistical analysis.

The video provides practical applications for understanding when to use each type of statistic.

The presenter encourages viewers to subscribe for more helpful content.

The video concludes with a motivational message about not letting a class get in the way of one's dreams.

Transcripts

Browse More Related Video

Z-statistics vs. T-statistics | Inferential statistics | Probability and Statistics | Khan Academy

Introduction to the t Distribution (non-technical)

t-distribution in Statistics and Probability | Statistics Tutorial #9 | MarinStatsLectures

Student's T Distribution

Confidence Intervals | Population Mean: Οƒ Unknown

Elementary Statistics - Chapter 7 - Estimating Parameters and Determining Sample Sizes Part 2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
StatisticsEducationZandTStatisticsMarkFromAceTutorsVideoTutorialStatisticalAnalysisNormalDistributionSampleSizePopulationStandardDeviationStatisticGradesEducationalContent