Trapezoidal sums | Accumulation and Riemann sums | AP Calculus AB | Khan Academy

Khan Academy
24 Jan 201308:27
EducationalLearning
32 Likes 10 Comments

TLDRThe video script outlines a method for approximating the area under the curve of the function y = sqrt(x-1) between x=1 and x=6 using five trapezoids of equal width. The process involves calculating the area of each trapezoid (which simplifies to triangles in this case), summing them up, and multiplying by the width (delta x), which is determined by the range of x divided by the number of trapezoids. The final calculation yields an approximate area of 7.26, demonstrating a practical application of the trapezoidal rule in integral calculus.

Takeaways
  • ๐Ÿ“ˆ The script discusses the method of approximating the area under a curve using trapezoids.
  • ๐Ÿ”ข The curve in question is y = โˆš(x - 1) between x = 1 and x = 6.
  • ๐ŸŸซ The approach involves dividing the area into five equal sections along the x-axis, each representing a trapezoid.
  • ๐Ÿ“ The width (delta x) of each trapezoid is determined to be 1, as the total range (6 - 1) is divided by 5.
  • ๐Ÿข The area of each trapezoid is calculated as the average of the heights (function values at the endpoints) times the width.
  • ๐Ÿ“Š The first and last sections are effectively triangles due to their single endpoint height being zero.
  • ๐Ÿ” The script emphasizes visualizing the trapezoids to better understand the approximation process.
  • ๐Ÿงฎ The final approximation formula includes one function value at the first endpoint, two at each subsequent endpoint, and two at the penultimate endpoint.
  • ๐Ÿคน The process simplifies to 0.5 * (f(1) + 2*f(2) + 2*f(3) + 2*f(4) + 2*f(5) + f(6)).
  • ๐Ÿ“ฑ The calculation yields an approximate area of 7.26 square units under the curve.
  • ๐Ÿ‘จโ€๐Ÿซ The script serves as a tutorial on approximating areas under curves, demonstrating the practical application of trapezoidal rules.
Q & A

  • What is the function being integrated in the given problem?

    -The function being integrated is y = โˆš(x - 1).

  • What is the interval over which the integration is performed?

    -The integration is performed over the interval from x = 1 to x = 6.

  • How many trapezoids are used to approximate the area under the curve?

    -Five trapezoids of equal width are used to approximate the area under the curve.

  • What is the width of each trapezoid?

    -The width of each trapezoid is 1 unit.

  • How is the area of a trapezoid calculated?

    -The area of a trapezoid is calculated as the average of the heights of the two parallel sides, multiplied by the base.

  • What is the formula used to approximate the area under the curve using trapezoids?

    -The formula used is approximately (1/2) * (f(1) + 2*f(2) + 2*f(3) + 2*f(4) + 2*f(5) + f(6)) * delta x.

  • What is the result of the area approximation?

    -The approximated area under the curve is 7.26 square units.

  • Why might the trapezoid method provide an underestimate of the actual area?

    -The trapezoid method might provide an underestimate because it does not account for the entire shape under the curve, missing out on some areas between the trapezoids.

  • What is the significance of using trapezoids to approximate the area under a curve?

    -Using trapezoids to approximate the area under a curve is a practical application of integration in calculus, allowing for numerical estimation when an analytical solution may not be feasible.

  • How does the approximation become more accurate with more trapezoids?

    -As the number of trapezoids increases, the width of each trapezoid decreases, leading to a better fit to the curve and a more accurate approximation of the area.

  • What mathematical concept does this problem illustrate?

    -This problem illustrates the concept of Riemann sums, which is a fundamental idea in the development of the integral calculus.

Outlines
00:00
๐Ÿ“Š Approximating Area Under a Curve with Trapezoids

The paragraph discusses the process of approximating the area under the curve of the function y = โˆš(x - 1) between x = 1 and x = 6 using trapezoids. The method involves dividing the area into five equal sections and calculating the area of each trapezoid (which actually turns out to be a triangle in the first and last cases). The key points include setting up the trapezoids, calculating the average height of each trapezoid, multiplying by the width (delta x), and summing the areas to get an approximation of the total area. The paragraph emphasizes the simplicity of the math and the visual aspect of the trapezoid method, which helps in understanding the concept better than a formulaic approach.

05:00
๐Ÿ“š Evaluating the Area Approximation with Trapezoids

This paragraph continues the discussion on approximating the area under the curve of y = โˆš(x - 1) using the trapezoid method. It explains how the formula for the approximation is derived from summing the areas of the trapezoids and how it can be simplified. The paragraph then proceeds to evaluate the approximation by plugging in the function values for each interval, using the square root of the respective x values minus one. The calculations are performed step by step, leading to a final approximate area of 7.26 square units under the curve. The paragraph highlights the practicality of this method and its usefulness in providing a clear, visual, and straightforward way to approximate areas under curves.

Mindmap
Keywords
๐Ÿ’กApproximation
Approximation in mathematics refers to the process of estimating a value or quantity that is not exactly known. In the context of the video, the speaker is using a method to approximate the area under a curve, which is a common application of approximation. The speaker aims to estimate this area by dividing the region into smaller, simpler shapes (trapezoids) whose areas can be more easily calculated, thus providing an approximation of the total area.
๐Ÿ’กCurve
A curve is a continuous, smooth shape in a two-dimensional space that represents the graph of a function. In the video, the curve is defined by the function y = sqrt(x - 1), and the speaker is interested in finding the area under this curve. The curve is the central object of study, as the area under it is the ultimate quantity to be approximated.
๐Ÿ’กTrapezoids
A trapezoid is a quadrilateral with at least one pair of parallel sides. In the video, the speaker divides the area under the curve into trapezoids to approximate the total area. This method is part of numerical integration techniques, where complex areas are estimated by breaking them down into simpler shapes, in this case, trapezoids.
๐Ÿ’กArea
Area refers to the amount of space inside a two-dimensional shape. In mathematics, calculating the area of a shape is a fundamental concept. In the video, the speaker's goal is to approximate the area under the curve y = sqrt(x - 1), which is a specific application of area calculation in the context of integration.
๐Ÿ’กIntegration
Integration is a fundamental concept in calculus that involves finding the area under a curve or the accumulated quantity associated with a variable. In the video, the speaker is performing a basic form of numerical integration by approximating the area under the curve using trapezoids.
๐Ÿ’กSquare Root
The square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the symbol โˆš. In the video, the function y = sqrt(x - 1) involves taking the square root of (x minus 1), which defines the shape of the curve under consideration.
๐Ÿ’กFunction
A function is a mathematical relation that pairs each element from a set (called the domain) to a unique element in another set (called the range). In the video, the function y = sqrt(x - 1) defines the relationship between x and y, which is used to create the curve and subsequently calculate the area under it.
๐Ÿ’กNumerical Methods
Numerical methods are techniques used in mathematics and science to find approximate solutions to problems that are difficult or impossible to solve exactly. In the video, the speaker uses a numerical method to approximate the area under a curve, which is a practical application of these methods.
๐Ÿ’กDelta X
Delta X, often denoted as ฮ”x, represents a small change or difference in the x-direction, typically used in calculus and physics to describe the interval over which a variable is being analyzed or integrated. In the video, the speaker uses the term 'delta x' to refer to the width of each trapezoid, which is set to be 1 for the purpose of approximation.
๐Ÿ’กCalculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. It includes two main branches: differential calculus, which deals with instantaneous rates of change, and integral calculus, which deals with accumulation. In the video, the speaker is applying concepts from integral calculus to approximate the area under a curve.
๐Ÿ’กEstimate
An estimate is a rough calculation or judgment of the value, number, quantity, or extent of something, often based on incomplete information. In the video, the speaker is providing an estimate of the area under the curve by using a numerical approximation method.
Highlights

The problem involves approximating the area under the curve of y = sqrt(x - 1) from x = 1 to x = 6.

The method used for approximation is by dividing the area into five equal trapezoids.

The width (delta x) of each trapezoid is determined to be 1, as the total range is 6 - 1.

The first trapezoid is actually a triangle due to the specific function and range.

The area of a trapezoid is calculated as the average of the two parallel sides' heights multiplied by the base.

The area of the first trapezoid (triangle) is calculated using the formula (f(2) * base * 1/2), resulting in an area of 0.5 * sqrt(2).

The second trapezoid's area is calculated using the formula ((f(2) + f(3)) / 2 * delta x), with f(3) being sqrt(2).

The third trapezoid's area is calculated similarly, with f(3) and f(4) being the heights, resulting in 2 * f(3) + 2 * f(4).

The fourth trapezoid's area calculation includes the heights f(4) and f(5), following the same pattern.

The fifth trapezoid's area includes the heights f(5) and f(6), with the final formula being 2 * f(5) + f(6).

The general formula for the area approximation using trapezoids is derived, including one f at the first endpoint, one at the last, and two of each in between.

The actual evaluation of the function at each endpoint and in-between points is performed, with f(x) being sqrt(x - 1).

The final calculation is done using a calculator, resulting in an approximate area of 7.26 under the curve.

The use of trapezoids provides a clear and understandable approximation method, as opposed to the more abstract formulas often seen in textbooks.

The process demonstrates the practical application of calculus in approximating areas under curves.

The method can be generalized for any similar problems, showcasing its versatility.

Transcripts

Browse More Related Video

The Trapezoid Rule for Approximating Integrals

Estimating areas using trapezoidal rule [IB Maths AI SL/HL]

Approximating Area Under the Curve, Using Rectangles

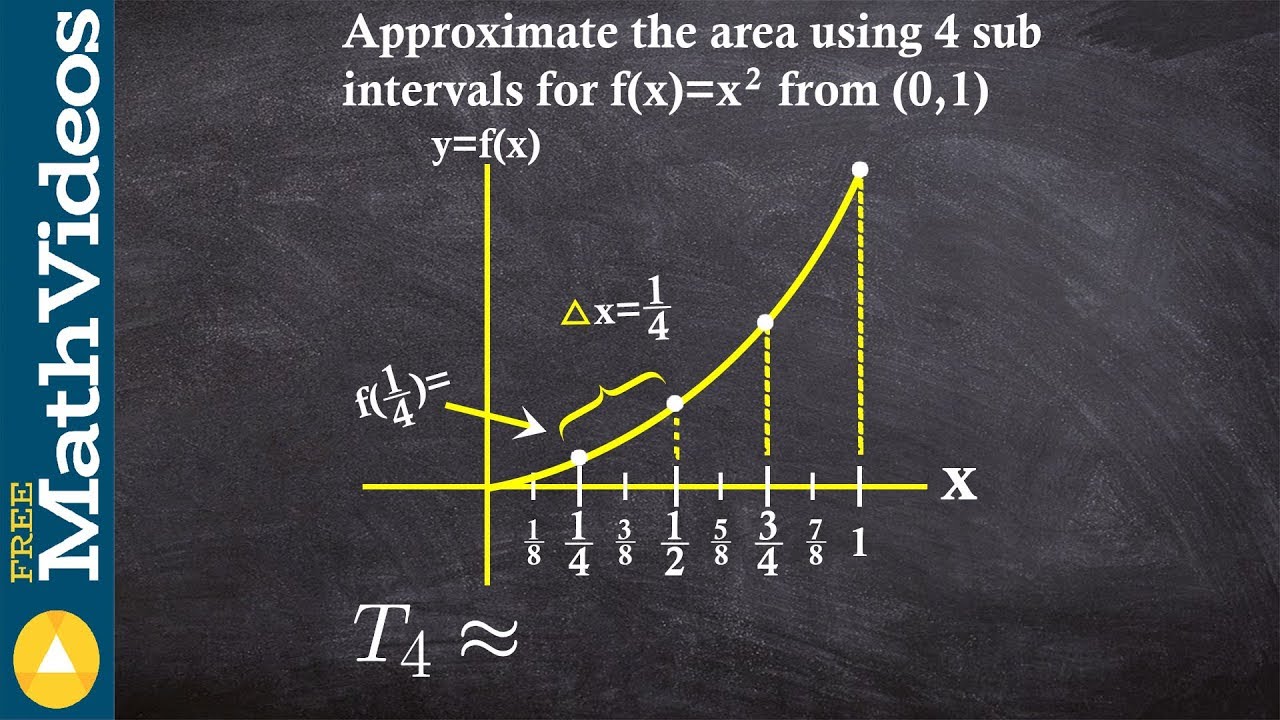
Learn how to use the trapezoidal rule with 4 sub intervals

Riemann sums in summation notation | Accumulation and Riemann sums | AP Calculus AB | Khan Academy

Fundamental Theorem of Calculus Explained | Outlier.org

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusArea ApproximationTrapezoidsRoot FunctionsIntegral CalculusEducationalInteractive LearningMath VisualizationNumerical Analysis