Limits of composite functions | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of limits involving composite functions, emphasizing the importance of understanding how to evaluate them step by step. It illustrates this with examples, showing how to find the limit of composite functions as the input approaches specific values. The examples demonstrate how to determine if a limit exists, whether it's finite or undefined, and how to proceed when dealing with discontinuities and unbounded behavior. The script effectively simplifies complex mathematical ideas, making it accessible for viewers to grasp the fundamentals of limits in calculus.
Takeaways
- 📌 The concept of limits involving composite functions is discussed, emphasizing the importance of understanding how to evaluate them step by step.
- 🔍 The process of finding a limit involves substituting the input function's limit into the outer function, which is demonstrated through the example of evaluating the limit of g(h(x)) as x approaches 3.
- 🌟 When approaching a certain point from the left and right, the function's behavior can differ, as seen with h(x) staying constant at 2 when approaching 3 from the left, but being undefined at 3 itself.
- 🚫 The limit of a function may not exist if the input function's limit is not defined or if the output function is undefined at the input function's limit, as illustrated with the example of h(x) at x = -1.
- ∞ The behavior of functions approaching discontinuities or unbounded values can lead to undefined limits, as the example with g(x) at x = -1 shows, where the function approaches negative infinity from the left and positive infinity from the right.
- 📈 The concept of one-sided limits is introduced, showing that even if the limit from both sides seems to be the same value, it might not hold if the function is not continuous at that point.
- 🔄 The process of evaluating composite functions involves a step-by-step substitution of limits, as demonstrated with the example of h(f(x)) as x approaches -3.
- 📊 The graph of a function can provide visual cues to help determine the limit, as seen with the evaluation of f(x) as x approaches -3, where the graph indicates the limit is 1.
- 🛑 Even if the limit of the inner function is well-defined, the composite function's limit may not exist if the outer function is undefined at the inner function's limit, as shown with h(f(x)) at x = -3.
- 🔑 The script underscores the importance of a systematic approach to evaluating limits, especially with composite functions, and the need to consider the behavior of functions around points of discontinuity or unbounded values.
Q & A
What is the limit of g(h(x)) as x approaches 3?
-The limit of g(h(x)) as x approaches 3 is equivalent to g(h(3)). Since h(x) approaches 2 from both the left and the right side as x approaches 3, the limit is g(2), which is 0 based on the given function values.
How does the limit of h(x) as x approaches 3 from the left differ from the right?
-The limit of h(x) as x approaches 3 does not differ from the left or the right. It consistently approaches 2, as h(x) stays at a constant value of 2 for values just less than 3, regardless of whether x approaches from the left or the right.
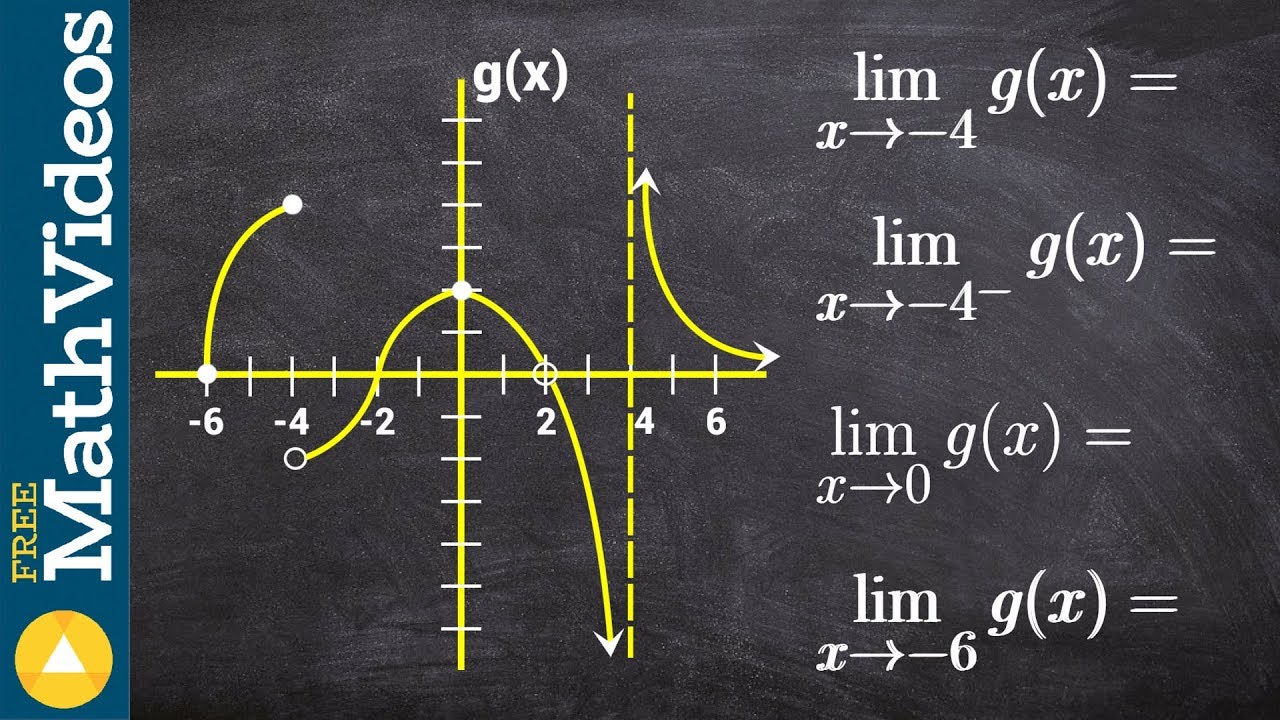
What is the significance of the discontinuity in the g(x) function at x equals negative one?
-The discontinuity in the g(x) function at x equals negative one indicates that the function is not defined at that point. This affects limit calculations, as the limit of g(x) as x approaches negative one is undefined, with the function approaching negative infinity from the left and positive infinity from the right.
How does the behavior of the function as it approaches infinity affect the limit calculation?
-When a function approaches infinity from both the left and right sides at a certain point, it can influence the limit of composite functions. However, if the function approaches positive and negative infinity from different sides, as in the case of g(x) at x equals negative one, the overall limit is undefined.
What is the limit of h(f(x)) as x approaches negative three?
-The limit of h(f(x)) as x approaches negative three is equivalent to h(f(negative three)). Since the limit of f(x) as x approaches negative three is 1, the expression simplifies to h(1). However, since h(1) is undefined, the overall limit does not exist.
Why does the limit of h(3.0000001) and h(3.01) matter in the calculation?
-The limit of h(3.0000001) and h(3.01) matters because it demonstrates the behavior of the function h(x) as x gets very close to 3 from the right side. Since both of these limits are equal to 2, it confirms that the limit of h(x) as x approaches 3 is indeed 2.
What is the role of limit properties in solving composite function limits?
-Limit properties are crucial in solving composite function limits as they allow us to break down the expression into simpler parts. By leveraging these properties, we can evaluate the limit of the outer function at the limit of the inner function, simplifying the process of finding the overall limit.
How does the limit of a function at a certain point affect the evaluation of composite functions?
-The limit of a function at a certain point is essential in evaluating composite functions because it becomes the input for the outer function. If the limit of the inner function does not exist or is undefined, it can render the overall limit of the composite function undefined, as seen with g(x) at x equals negative one.
What is the significance of evaluating the function at specific values near a limit point?
-Evaluating the function at specific values near a limit point, such as h(2.999999999) or f(negative 3.01), provides evidence of the function's behavior as it approaches the limit point. This helps in confirming whether the limit exists and determining its value.
What happens when the output of the inner function is undefined for the input of the outer function?
-When the output of the inner function is undefined for the input of the outer function, as was the case with h(1), the overall limit of the composite function does not exist. This is because the outer function cannot be evaluated at the given input.
How does the concept of limits approaching from the left and right influence the understanding of a function's behavior?
-Understanding the limits of a function as it approaches a point from the left and right provides insight into the function's behavior near that point. If the limits from both sides are equal, the function is consistent in its approach to the limit point. If they differ or if one does not exist, the function may have discontinuities or undefined behavior at that point.
Outlines
📚 Understanding Limits with Composite Functions
This paragraph delves into the concept of limits involving composite functions. It begins with a voiceover introducing the limit of g(h(x)) as x approaches 3, encouraging viewers to attempt solving it independently. The explanation unfolds by breaking down the problem using limit properties, demonstrating that the limit can be expressed as g(limit(h(x))) or equivalently, g(h(3)). The focus then shifts to finding the limit of h(x) as x approaches 3, highlighting the behavior of the function from both the left and the right, which leads to a limit of 2. The segment concludes by evaluating g(2), which is found to be 0, thus solving the initial limit problem. The paragraph also addresses the scenario where the limit of a function does not exist, as seen when approaching x equals negative one from both directions results in different infinities, rendering the overall limit undefined.
🔍 Dealing with Undefined Outputs in Limits
This paragraph continues the discussion on limits, specifically addressing situations where the output of a function is undefined when used as an input for another function. The example given involves finding the limit as x approaches negative one for h(g(x)). Initially, the limit of g(x) as x approaches negative one is determined, which is found to be unbounded, leading to the conclusion that the limit does not exist. Consequently, since the input for h(x) is undefined, the entire limit expression is deemed non-existent. The paragraph further illustrates this concept with another example, examining the limit of h(f(x)) as x approaches negative three. Despite a straightforward limit for f(x), the final result is an undefined output for h(x) at the value of one, rendering the overall limit non-existent.
Mindmap
Keywords
💡limits
💡composite functions
💡discontinuity
💡undefined
💡approaching from the left/right
💡one-sided limits
💡evaluation
💡convergence
💡infinity
💡limit properties
💡function graphs
Highlights
The concept of limits involving composite functions is discussed.
The limit of g(h(x)) as x approaches 3 is equivalent to g of the limit of h(x) as x approaches 3.
When approaching 3 from the left, h(x) consistently stays at a constant value of 2.
The limit of h(x) as x approaches 3 from both directions is 2.
g(2) is equal to 0, which simplifies the composite limit to 0.
The limit as x approaches -1 of h(g(x)) is explored next.
As x approaches -1 from the left, g(x) goes to negative infinity.
As x approaches -1 from the right, g(x) goes to positive infinity.
The limit of h(g(x)) as x approaches -1 does not exist due to g(x) approaching infinity in opposite directions.
The limit of h(f(x)) as x approaches -3 is examined.
The limit of f(x) as x approaches -3 from both directions is 1.
h(1) is undefined, which means the composite limit h(f(x)) as x approaches -3 does not exist.
The transcript demonstrates the importance of evaluating the limits of composite functions step by step.
The process of finding limits involves understanding the behavior of functions as they approach certain values.
The concept of limits is crucial in calculus for determining the behavior of functions at specific points.
The transcript provides a clear and methodical approach to finding limits of composite functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: