Position vector valued functions | Multivariable Calculus | Khan Academy

Khan Academy
26 Feb 201007:44
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the concept of vector-valued functions and their application to line integrals. It begins by differentiating between scalar and vector-valued functions, then explains how curves can be described using parametric equations. The script further illustrates how to represent a curve using position vectors, emphasizing the role of the origin and the unique specification of points in space. The discussion sets the stage for understanding the derivative of vector-valued functions, which will be explored in the subsequent video.

Takeaways
  • πŸ“Œ The discussion focuses on transitioning from scalar functions to vector-valued functions in the context of line integrals.
  • πŸ”„ The video script introduces the concept of parameterizing curves, initially using scalar functions and then moving to vector-valued functions.
  • πŸ“ˆ The parameterization of a curve is described using functions x(t) and y(t), where t is a parameter that varies between a and b.
  • πŸ€” The script emphasizes the difference between scalar values (a single number) and vector values (which have both magnitude and direction).
  • πŸ›€οΈ A curve in the plane is represented by a position vector-valued function r(t), which starts at the origin and ends at a point (x(t), y(t))
  • πŸ“ The position vector is composed of x(t) times the unit vector in the x-direction and y(t) times the unit vector in the y-direction.
  • πŸŒ€ The script explains how to evaluate the position vector at different values of the parameter t, illustrating how this traces out the curve.
  • πŸ”’ The concept of a unit vector is introduced, with the notation i (for the x-direction) and j (for the y-direction), and their role in constructing position vectors.
  • πŸš€ The script provides a visual description of how the position vector changes as the parameter t increases, moving along the curve.
  • πŸ“š The purpose of reviewing these concepts is to prepare for understanding the derivative of vector-valued functions, which will be discussed in the next video.
  • πŸŽ₯ The explanation is given in an abstract and general form, without specific functions or curves, to emphasize the concepts over particular examples.
Q & A

  • What is the main focus of the video script?

    -The main focus of the video script is to introduce the concept of line integrals with vector-valued functions and provide an understanding of how to describe curves using parametric equations and position vector-valued functions.

  • What is the difference between scalar functions and vector-valued functions?

    -Scalar functions are functions that, given an input, produce a single numerical value, whereas vector-valued functions produce a vector as their output, which has both magnitude and direction.

  • How is a curve described using parametric equations?

    -A curve is described using parametric equations by defining functions x(t) and y(t) for the x and y coordinates, respectively, where 't' is a parameter that varies between two values 'a' and 'b'.

  • What is a position vector?

    -A position vector is a vector that starts at the origin and points to a specific position in space, representing a unique location. It is used to describe the coordinates of a point in terms of its x, y, and (if applicable) z components.

  • How can a curve be represented using a vector-valued function?

    -A curve can be represented using a vector-valued function by defining a function r(t) that depends on the parameter 't', where r(t) is a position vector with components x(t) and y(t) for a two-dimensional space.

  • What is the significance of unit vectors in the context of position vectors?

    -Unit vectors are used in the context of position vectors to scale the x and y components of the vectors according to the values of the functions x(t) and y(t), which helps in constructing the position vector at any point 't' along the curve.

  • How does the vector r(t) change as 't' increases from 'a' to 'b'?

    -As 't' increases from 'a' to 'b', the vector r(t) changes by incrementally moving along the curve, with its components x(t) and y(t) representing the position on the curve at each value of 't'.

  • What is the purpose of reviewing parametric equations and position vector-valued functions in the script?

    -The purpose of reviewing parametric equations and position vector-valued functions is to provide a foundation for understanding how to take a derivative of a vector-valued function, which will be discussed in the next video.

  • How does the script relate the concept of position vectors to the description of a curve?

    -The script relates the concept of position vectors to the description of a curve by showing that both the parametric equations and the position vector-valued function can represent the same curve, with the position vectors specifying the points along the curve as 't' varies.

  • What is the significance of understanding the relationship between parametric equations and vector-valued functions?

    -Understanding the relationship between parametric equations and vector-valued functions is significant because it allows for a more versatile approach to describing and analyzing curves and their properties, such as taking derivatives and integrals in various mathematical and engineering applications.

Outlines
00:00
πŸ“Œ Introduction to Vector-Valued Functions and Line Integrals

This paragraph introduces the concept of vector-valued functions and their application to line integrals. It begins by discussing scalar functions and emphasizes the transition from working with scalar functions to vector-valued functions. The speaker then describes a curve 'c' that can be parameterized by a function of 't', with 'x' and 'y' being functions of 't' within the interval 'a' to 'b'. The aim is to understand how to take a line integral with vector-valued functions. The speaker abstractly describes a curve in the Cartesian coordinate system and explains how to represent it using a vector-valued function 'r(t)'. The concept of position vectors is introduced, which are distinct from general vectors in that they start at the origin and specify a unique position in space. The speaker then demonstrates how to represent the curve 'c' using a position vector-valued function 'r(t)', which is a sum of the product of the function's components and the corresponding unit vectors.

05:05
πŸ“ˆ Visualizing Position Vectors and their Derivatives

In this paragraph, the speaker delves into the visualization of position vectors and their derivatives. It starts by explaining how to represent the position vector 'r(a)' at the starting point 'a' of the curve 'c'. The speaker illustrates how the position vector is obtained by scaling the unit vectors in the x and y directions by the components 'x(a)' and 'y(a)', respectively. The concept of incrementing 't' to 'a + h' is introduced to show how the position vector changes, resulting in a new vector 'r(a + h)' that lies further along the curve. The speaker emphasizes that these position vectors specify the same points on the curve as the original parametric equations. The paragraph concludes with a mention of the upcoming topic of taking the derivative of the vector-valued function, which will be covered in the next video.

Mindmap
Keywords
πŸ’‘line integrals
Line integrals are a mathematical concept used to calculate the accumulated effect of a vector field along a curve. In the context of the video, line integrals are introduced as a way to understand the behavior of scalar functions along a path, and the video is transitioning towards discussing line integrals with vector-valued functions.
πŸ’‘scalar functions
Scalar functions are mathematical functions that assign a single value, or scalar, to each point in their domain. Unlike vector functions, which output vectors, scalar functions output single numbers. In the video, the concept of scalar functions is used as a starting point to introduce vector-valued functions.
πŸ’‘vectors
Vectors are mathematical objects that have both magnitude and direction. They are different from scalars, which only have magnitude. In the context of the video, vectors are introduced as a way to describe the position in space and to prepare for understanding line integrals with vector-valued functions.
πŸ’‘vector-valued functions
Vector-valued functions are functions that map inputs to outputs in a way that each output is a vector. These functions are contrasted with scalar-valued functions, which output only a single number. In the video, vector-valued functions are introduced as a means to describe curves in a different way, using position vectors.
πŸ’‘parameterized curves
Parameterized curves are mathematical descriptions of curves using a parameter, typically denoted as 't', to define the coordinates of points on the curve. The parameter varies within a specified range, and for each value of the parameter, a unique point on the curve is determined. In the video, parameterized curves are used to describe a curve 'c' in terms of x(t) and y(t) functions.
πŸ’‘position vectors
Position vectors are vectors that represent the displacement from the origin to a specific point in space. They have a magnitude and a direction, and theirθ΅·η‚Ή is always the origin. Position vectors are used to describe the location of points in space and are central to the concept of vector-valued functions in the video.
πŸ’‘unit vectors
Unit vectors are vectors with a magnitude of one, used to specify direction. They are often denoted with a hat or caret (^) above the vector symbol. In the context of the video, unit vectors are used to construct position vectors by scaling them with the coordinates of points on a curve.
πŸ’‘Cartesian coordinates
Cartesian coordinates are a coordinate system that specifies each point in a plane with an ordered pair of numbers, typically the x and y coordinates. In the video, Cartesian coordinates are used to describe the location of points on a curve and to relate the parametric form of a curve to its position vector representation.
πŸ’‘derivatives
Derivatives are a fundamental concept in calculus that describe the rate of change of a function at a particular point. In the context of the video, the concept of derivatives is introduced as a next step after understanding vector-valued functions, suggesting that the video will explore how to find the derivative of a vector-valued function.
πŸ’‘tangent vectors
Tangent vectors are vectors that are parallel to the curve at a given point and have a magnitude of one. They are used to describe the local behavior of a curve at a particular point. In the video, the concept of tangent vectors is implied when discussing the change in position vectors as the parameter 't' changes.
πŸ’‘n-dimensional space
n-dimensional space refers to a mathematical space with 'n' degrees of freedom, where 'n' can be any positive integer. In such a space, each point is specified by 'n' coordinates. The video mentions n-dimensional space to generalize the concept of position vectors beyond the two-dimensional example being discussed.
Highlights

The introduction of the concept of line integrals with scalar functions.

The transition from scalar functions to vector-valued functions in the context of line integrals.

The description of a curve using parametric equations, with x and y as functions of a parameter t.

The validity of the parameter t in the interval [a, b] for the parametric representation of the curve.

The visualization of the curve in the Cartesian coordinate system.

The introduction of vector-valued functions and their notation, with a vector arrow on top of the function symbol.

The definition of position vectors and their significance in specifying unique positions in space.

The representation of the curve as a position vector-valued function in two-dimensional space.

The explanation of unit vectors and their role in constructing position vectors.

The process of evaluating the position vector-valued function at specific points along the curve.

The illustration of how the position vector changes as the parameter t increases.

The comparison between the original parametric representation of the curve and the vector-valued function representation.

The anticipation of discussing the derivative of the vector-valued function in the subsequent video.

The emphasis on the importance of understanding vectors for comprehending line integrals with vector-valued functions.

Transcripts

Browse More Related Video

Derivative of a position vector valued function | Multivariable Calculus | Khan Academy

Calculus 3: Vector Functions and Space Curves (Video #7) | Math with Professor V

Calculus 3: Derivatives & Integrals of Vector Functions (Video #8) | Math with Professor V

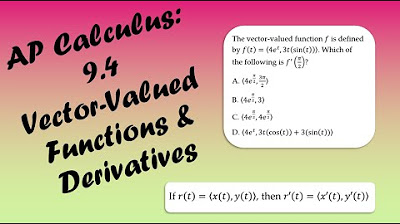
AP Calculus BC Lesson 9.4

Derivatives and Tangent Vectors (Vector-Valued Functions)

Calculus 3: Arc Length and Curvature (Video #9) | Math with Professor V

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Vector CalculusLine IntegralsParametric EquationsCurve AnalysisScalar FunctionsVector-Valued FunctionsMathematical ConceptsEducational ContentAdvanced MathGeometry