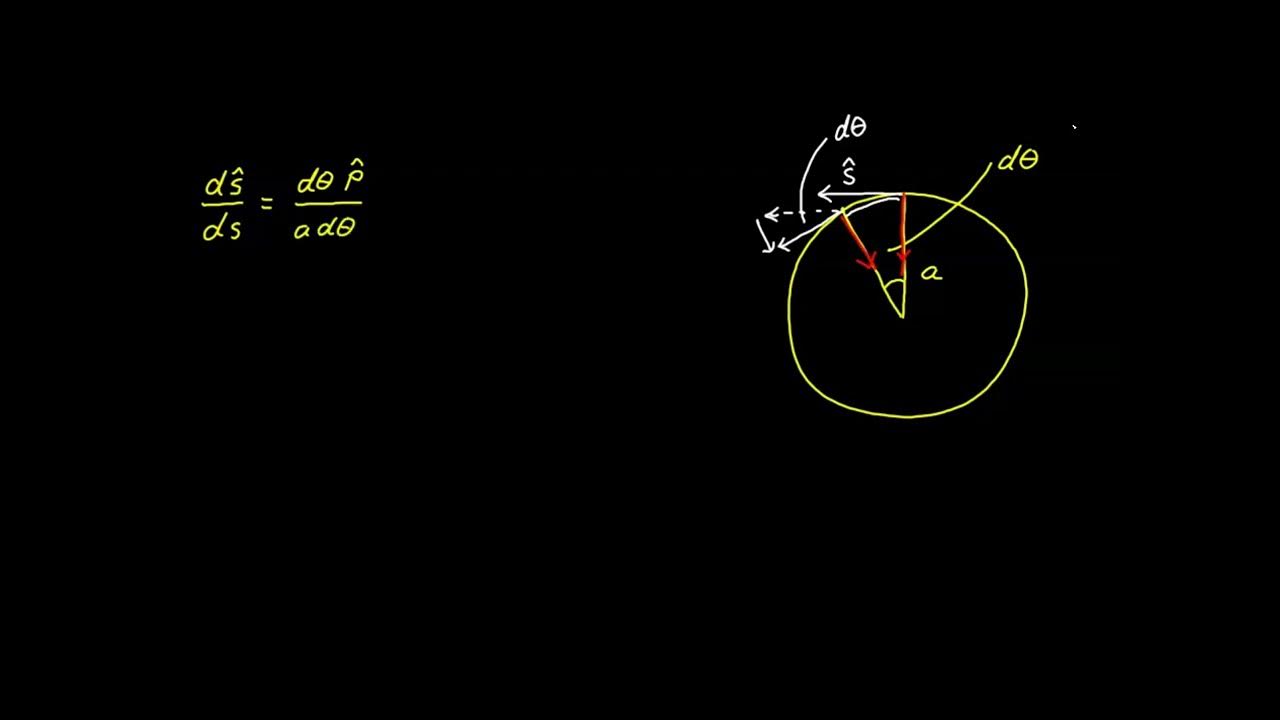Calculus 3: Arc Length and Curvature (Video #9) | Math with Professor V
TLDRThis video lecture delves into the concepts of arc length and curvature for vector-valued functions, essential in multivariable calculus. It explains how to calculate arc length using definite integrals of the magnitude of the derivative of the vector function. The lecture further explores the unit tangent vector, the principal unit normal vector, and the binormal vector, illustrating their geometric interpretations and relationships. Examples are provided to compute these quantities for specific functions, emphasizing the importance of these concepts in understanding the behavior of curves in three-dimensional space.
Takeaways
- 📚 The arc length of a curve in a plane can be calculated using the definite integral of the square root of \((dx/dt)^2 + (dy/dt)^2\) from 'a' to 'b' for a parametrically represented curve.
- 📐 The arc length for a vector-valued function \(\vec{r}(t)\) is given by the integral of the magnitude of \(d\vec{r}/dt\) from 'a' to 'b', which is the integral of \(\sqrt{(dx/dt)^2 + (dy/dt)^2 + (dz/dt)^2} dt\).
- 🔍 The integrand for arc length represents the magnitude of the derivative of the vector function, which is a measure of how much the function changes over an infinitesimally small segment of the curve.
- 📈 The concept of arc length extends naturally to three dimensions and is independent of the chosen parametrization as long as it does not impose different restrictions.
- 🌐 An example demonstrates how to find the arc length of a curve with components \(12t\), \(8t^{3/2}\), and \(3t^2\) for \(t\) between 0 and 1 by computing the integral of \(6(t+2)\).
- 🦄 The script discusses the concept of parametrization of curves and how different parametrizations can be used to represent the same curve, with the arc length remaining unchanged.
- 👣 The arc length parameterization of a curve uses the actual length of the curve as the parameter, which can be useful for finding the curvature of the curve.
- 💫 Curvature (\(\kappa\)) is defined as the magnitude of the rate of change of the unit tangent vector with respect to arc length, indicating how quickly the curve changes direction.
- 🌀 Curvature can also be calculated using the formula \(\kappa = \frac{| \vec{r'} \times \vec{r''} |}{|\vec{r'}|^3}\), which involves the cross product of the first and second derivatives of the vector function.
- 📏 The script provides an example of finding the curvature of a circle using its radius, showing that the curvature is inversely related to the radius (\(\kappa = \frac{1}{a}\) for a circle of radius \(a\)).
- 🧭 The principal unit normal vector (\(\vec{n}(t)\)) and the binormal vector (\(\vec{b}(t)\)) are introduced as part of the t-b frame, which are orthogonal to the unit tangent vector and each other, and are used to describe the motion of a curve in 3D space.
Q & A
What is the formula for calculating the arc length of a curve in a plane using parametric representation?
-The arc length of a curve in a plane with parametric representation (x(t), y(t)) is given by the definite integral from 'a' to 'b' of the square root of (dx/dt)^2 + (dy/dt)^2 dt.
How does the arc length formula extend to three dimensions for vector valued functions?
-In three dimensions, for a vector valued function r(t) = <f(t), g(t), h(t)>, the arc length is given by the definite integral from 'a' to 'b' of the square root of (dx/dt)^2 + (dy/dt)^2 + (dz/dt)^2 dt, which is equivalent to the integral of the magnitude of r'(t) dt.
What is the incremental change in arc length denoted as?
-The small incremental change in arc length is denoted as ds, and it is equal to the magnitude of r'(t) dt.
How can you find the arc length of a curve defined by a vector valued function r(t) with components 12t, 8t^(3/2), and 3t^2 for t between 0 and 1?
-First, find r'(t) = <12, 12t^(1/2), 6t>, then compute its magnitude, and integrate from 0 to 1. The result is the arc length.
What does the magnitude of r'(t) represent in the context of arc length?
-The magnitude of r'(t) represents the speed of the moving point along the curve at any instant 't', and when integrated, it gives the total arc length.
How can you parametrize a curve if you only have a function, like a parabola y = x^2?
-You can parametrize a curve like y = x^2 by letting x = t and y = t^2 for the right half of the parabola, with the domain restriction t ≥ 0.
Why is it not necessary to specify t ≥ 0 when parametrizing the parabola y = x^2 with x = sqrt(t) and y = t?
-It is not necessary because the domain of sqrt(t) inherently includes only values of t that are greater than or equal to zero.
What is the relationship between the arc length parameterization and the original parameter t for a circle x^2 + y^2 = k^2?
-For a circle, the relationship is s = kt, where 's' is the arc length, 'k' is the radius, and 't' is the angle in radians. The parameter 't' is then s/k.
How can you find the arc length parametrization for a less familiar curve without a direct formula?
-Define s(t) as the definite integral from 'a' to 't' of the magnitude of r'(u) du, which gives the arc length from the starting point to an arbitrary point on the curve. Then relate 's' and 't' and switch to a parametric representation in terms of 's'.
What is the curvature of a curve and how is it defined?
-Curvature is a measure of how quickly a curve changes direction at any point. It is defined as the magnitude of the rate of change of the unit tangent vector with respect to arc length, denoted as |dT/ds| where T is the unit tangent vector and s is the arc length.
How can you find the curvature of a circle of radius 'a'?
-The curvature of a circle is given by 1/a, as the unit tangent vector changes direction more quickly for smaller radii, indicating greater curvature.
What is the formula for curvature in terms of the vector-valued function r(t) and its derivatives?
-Curvature kappa can be found using the formula |r'(t) x r''(t)| / |r'(t)|^3, where x denotes the cross product.
What are the three fundamental vectors associated with a smooth curve, and what are their properties?
-The three fundamental vectors are the unit tangent vector T(t), the principal unit normal vector N(t), and the binormal vector B(t). T(t) is tangent to the curve, N(t) is orthogonal to T(t) and points in the direction of the curve's turning, and B(t) is orthogonal to both T(t) and N(t) and is already a unit vector.
How can you find the equation of the oscillating circle for a given curve at a specific point?
-Find the curvature at the point, which gives the radius of the oscillating circle. The center of the circle is at the point on the curve, and the equation is (x - x_center)^2 + (y - y_center)^2 = radius^2.
Outlines
📚 Introduction to Arc Length and Curvature
This paragraph introduces the concepts of arc length and curvature in calculus, specifically for vector-valued functions. It explains how the arc length of a plane curve can be calculated using a definite integral involving the square root of the sum of the squares of the derivatives of the parametric components. The concept is then extended to three dimensions, with the arc length formula adjusted to account for the vector nature of the functions. The importance of the magnitude of the derivative of the vector function, r'(t), in calculating arc length is highlighted. The paragraph also presents an example calculation of arc length for a given vector function.
🔍 Exploring Parametrization and Arc Length
The second paragraph delves deeper into the topic of parametrization, discussing how curves can be represented in various ways without changing the curve's length or shape. It uses the example of a parabola and a circle to illustrate different parametrizations and their implications. The concept of arc length parametrization is introduced, where the arc length 's' becomes the parameter, and a formula for the arc length of a circle is derived. The paragraph emphasizes that the choice of parametrization does not affect the computed arc length, as long as it does not impose different restrictions.
🌐 Transitioning to Arc Length Parametrization
This paragraph focuses on the process of transitioning to arc length parametrization for a curve. It explains how to define 's(t)', the arc length as a function of the parameter 't', and how to establish a relationship between 's' and 't'. An example is provided to demonstrate how to find the arc length parametrization for a less familiar curve, where a direct formula for arc length is not readily available. The process involves integrating the magnitude of the derivative of the vector function and solving for the relationship between 's' and 't'.
🔄 Deriving Curvature from Arc Length Parametrization
Curvature, as a measure of how quickly a curve changes direction, is the main topic of this paragraph. It introduces the concept of curvature, denoted by the Greek letter 'kappa', and explains it as the magnitude of the rate of change of the unit tangent vector with respect to arc length. The paragraph provides a formula for curvature using the arc length parameter and discusses the importance of correctly applying the chain rule and understanding the relationship between the unit tangent vector and its derivative.
🌀 Understanding Curvature and Its Computations
The paragraph discusses the computation of curvature using a direct method that does not require an arc length parametrization. It presents an alternative formula for curvature, kappa, which involves the magnitude of the cross product of the first and second derivatives of the vector function, divided by the cube of the magnitude of the first derivative. An example of finding the curvature of a circle using this formula is provided, highlighting the inverse relationship between curvature and radius.
📐 Curvature and Its Alternate Forms
This paragraph presents an alternate form of the curvature formula, which involves the cross product of the first and second derivatives of a vector function. It explains the derivation of this formula and how it relates to the unit tangent vector and its derivative. The paragraph also introduces another form of curvature applicable when a function y = f(x) is given without a parametric representation, using the first and second derivatives of the function.
🧭 The Role of Unit Tangent, Normal, and Binormal Vectors
The concept of the unit tangent, principal unit normal, and binormal vectors in the context of smooth curves is explored in this paragraph. It explains how these vectors are derived from the first and second derivatives of a vector function and how they are orthogonal to each other. The paragraph also discusses the significance of these vectors in determining the normal plane and the oscillating plane, and introduces the concept of the t and b frame.
📉 Vector Analysis for a Given Function
This paragraph provides a detailed example of how to find the unit tangent, unit normal, and binormal vectors for a given vector function at a specific point. It demonstrates the process of taking derivatives, normalizing them to create unit vectors, and using cross products to find the binormal vector. The example illustrates the application of these concepts in vector analysis.
🌀 The Oscillating Circle and Curvature
The final paragraph concludes the unit on vector-valued functions by discussing the oscillating circle associated with a curve at a given point. It explains how to find the equation of the oscillating circle using the curvature at a specific point on the curve. An example is provided to demonstrate how to calculate the curvature of a parabola at the origin and derive the equation of the corresponding oscillating circle.
Mindmap
Keywords
💡Arc Length
💡Parametric Representation
💡Vector Valued Function
💡Derivative
💡Magnitude
💡Unit Tangent Vector
💡Curvature
💡Parametrization
💡Cross Product
💡Binormal Vector
Highlights
Introduction to the concept of arc length for vector-valued functions in three dimensions.
Explanation of how to compute arc length using the integral of the magnitude of the derivative of the vector function.
Discussion on the relationship between the integrand and the magnitude of the derivative vector.
Application of the arc length formula using a specific example with components 12t, 8t^(3/2), and 3t^2.
Differentiation of vector components to find the derivative vector and its magnitude.
Integration of the magnitude of the derivative vector to find the arc length between two points.
Parametrization of curves and its impact on arc length calculations.
Illustration of multiple ways to parametrize a curve, such as a parabola, and the implications for arc length.
Introduction to the concept of arc length parameterization for curves.
Example of finding arc length parametrization for a circle using s = rθ.
General method for deriving arc length parameterization for any smooth curve.
Explanation of curvature as a measure of how quickly a curve changes direction.
Definition and computation of curvature using the rate of change of the unit tangent vector.
Derivation of an alternate formula for curvature involving the cross product of first and second derivatives of the vector function.
Application of the curvature formula to find the curvature of a circle with a given radius.
Introduction to the unit tangent, principal unit normal, and binormal vectors and their geometric interpretations.
Procedure to find the unit tangent, unit normal, and binormal vectors for a given vector function.
Explanation of the normal plane, oscillating plane, and the T and B frame formed by the unit vectors.
Example of finding the equation of the oscillating circle for a given parabola at a specific point.
Summary of the key concepts covered in the lecture on vector-valued functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: