Calculus 3: Derivatives & Integrals of Vector Functions (Video #8) | Math with Professor V
TLDRThis calculus lecture introduces derivatives and integrals of vector functions, explaining their geometric interpretation and significance in calculus. The instructor defines the derivative of a vector-valued function and illustrates it geometrically, showing how it represents the tangent vector to a curve traced by the function. The lesson covers finding the derivative of a given vector function, determining the parametric equations of a tangent line, and differentiating vector functions with examples. It also touches on the properties of differentiation rules for vector functions and concludes with an exploration of integrating vector functions, including finding the original vector function given its derivative and calculating the angle of intersection between two curves.
Takeaways
- 📚 The lesson covers derivatives and integrals of vector functions, building upon the understanding of vector valued functions from the previous lesson.
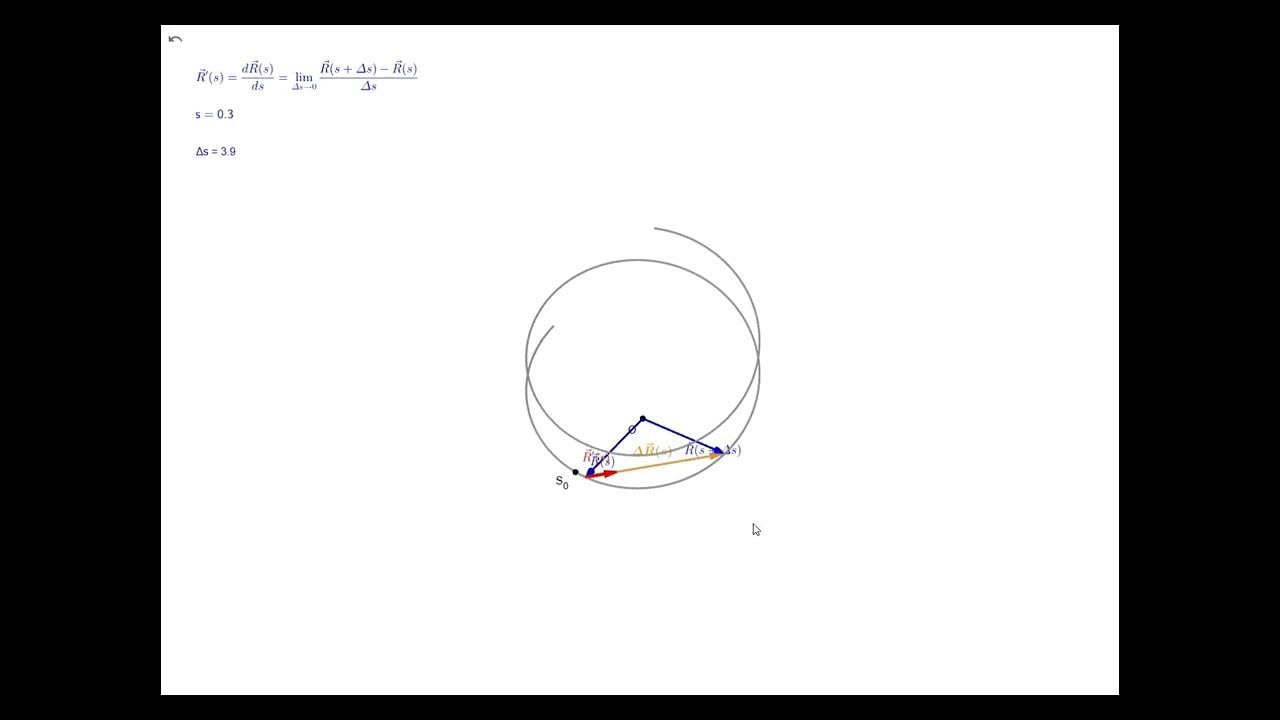
- 🔍 The definition of the derivative of a vector valued function parallels that of a real-valued function, with the limit process used to find the derivative geometrically represented as the tangent vector to a space curve.
- 📈 The process of differentiating a vector-valued function involves differentiating each component of the vector.
- 📝 An example provided involves finding the derivative and the parametric equations of the tangent line to a curve defined by a vector-valued function at a specific point.
- 📉 The script discusses the representation of plane curves with vector functions and the method to sketch curves and tangent vectors using components like sine and cosine, leveraging the Pythagorean identity.
- 📌 The importance of differentiating to find tangent vectors at specific points on a curve is emphasized, with an example of a curve defined by an ellipse.
- 📚 Differentiation rules for vector-valued functions are outlined, including distribution over addition, scalar multiplication, product rule, and the application of the chain rule.
- 🧩 A proof is provided to show that if the magnitude of a vector-valued function is constant, its derivative is orthogonal to the function itself, highlighting a property of circles.
- 📐 The concept of the unit tangent vector is introduced, which is a tangent vector with a magnitude of 1, found by dividing the derivative of a vector function by its magnitude.
- ✏️ Integration of vector functions is also component-wise, similar to differentiation, with an example provided to demonstrate the process.
- 🔄 The script concludes with an example of finding the angle of intersection between two curves by using the dot product of their tangent vectors at the point of intersection.
Q & A
What is the definition of the derivative of a vector-valued function?
-The derivative of a vector-valued function R(t), denoted as R'(t), is defined as the limit as h approaches 0 of (R(t + h) - R(t)) divided by h, which is similar to the definition of a derivative for a real-valued function.
How is the derivative of a vector function visualized geometrically?
-Geometrically, the derivative of a vector function can be visualized as the tangent vector to the space curve traced by the vector function. As h approaches zero, the vector (R(t + h) - R(t)) shrinks to the tangent vector at the point of tangency.
What is the process to find the parametric equations of the tangent line to a curve at a given point?
-To find the parametric equations of the tangent line, first evaluate the derivative of the vector function at the point of tangency to get the direction vector. Then, use the point and the direction vector to write the parametric equations in the form x = x₀ + at, y = y₀ + bt, z = z₀ + ct, where (x₀, y₀, z₀) is the point and a, b, c are the components of the direction vector.
How do you differentiate each component of a vector-valued function?
-When differentiating a vector-valued function, you differentiate each component of the vector with respect to the parameter. For example, if R(t) = f(t)i + g(t)j + h(t)k, then R'(t) = f'(t)i + g'(t)j + h'(t)k.
What is the significance of the dot product in proving that if the magnitude of a vector-valued function is constant, then its derivative is orthogonal to the function?
-The dot product is used to show that the derivative is orthogonal to the vector function by demonstrating that their dot product is zero. This is because the dot product of two orthogonal vectors is zero, and the magnitude squared of a vector is equal to the dot product of the vector with itself.
What is the unit tangent vector and how is it found?
-The unit tangent vector is a tangent vector with a magnitude of 1. It is found by dividing the derivative of the vector function by its magnitude, normalizing it to have a unit length.
How is integration of vector functions performed?
-Integration of vector functions is performed component-wise, just like with real-valued functions. Each component of the vector function is integrated separately, and the results are combined to form the integrated vector function.
What is the method to find the point of intersection between two vector functions?
-To find the point of intersection, set the corresponding components of the two vector functions equal to each other and solve the resulting system of equations for the parameters. The solution gives the values of the parameters at the point of intersection, which can then be substituted back into either vector function to find the coordinates of the intersection point.
How can the angle of intersection between two curves be found?
-The angle of intersection between two curves can be found by calculating the angle between the tangent vectors to the curves at their point of intersection. This is done using the dot product and the magnitudes of the tangent vectors, and then taking the inverse cosine (arccos) of the result.
What is the application of the tangent vector in the context of this script?
-The tangent vector is used to describe the direction of a curve at a specific point. It is also used to find the angle of intersection between two curves by comparing the tangent vectors at their point of intersection.
Outlines
📚 Introduction to Derivatives and Integrals of Vector Functions
This paragraph introduces the topic of derivatives and integrals of vector functions, following the study of vector valued functions in the previous lesson. The definition of the derivative of a vector function is presented, which is similar to that of a real-valued function. The geometric interpretation of the derivative is discussed, including the concept of a tangent vector to a curve traced by the vector function. An example of differentiating a specific vector function is given, along with finding the parametric equations of the tangent line to a curve at a given point.
🔍 Deriving Parametric Equations for Tangent Lines
The paragraph delves into the process of computing the derivative of a vector function and using it to find the parametric equations of a tangent line at a specific point. It explains the method of differentiating each component of the vector function and then using the derivative to determine the direction vector of the tangent line. An example is provided where the vector function's components are differentiated, and the point yielding the tangent line is identified by inspection. The parametric equations for the tangent line are then formulated based on the direction vector and the given point.
📐 Sketching Curves and Tangent Vectors from Vector Functions
This section discusses the representation of plane curves using vector functions and the process of sketching these curves along with their tangent vectors. The paragraph begins with an example where the components of a vector function are sine and cosine functions, leading to the conclusion that the graph is an ellipse. The orientation of the ellipse is determined, and the tangent vector at a specific parameter value is computed. The importance of drawing tangent vectors from the point of tangency rather than as position vectors is emphasized.
📝 Differentiation Rules and Orthogonality in Vector Functions
The paragraph presents the differentiation rules for vector-valued functions, which are straightforward and involve applying the same rules as for real-valued functions. It also introduces a proof for a specific property: if the magnitude of a vector-valued function is constant, then its derivative is orthogonal to the function itself for all parameters. This is illustrated with the example of a circle, where the radius has a constant magnitude, and the tangent is always perpendicular to the radius at the point of tangency.
🧭 Unit Tangent Vectors and Integration of Vector Functions
This section introduces the concept of unit tangent vectors, which are tangent vectors with a magnitude of 1, obtained by dividing a tangent vector by its magnitude. An example is provided to demonstrate how to find the unit tangent vector for a given vector function. The paragraph then transitions to the integration of vector functions, which is performed component-wise, similar to integration in calculus for real-valued functions. An example of integrating a vector function is given, showcasing the process of integrating each component separately.
🔍 Finding Original Vector Functions and Points of Intersection
The paragraph discusses the process of finding the original vector function given its derivative, including handling initial conditions to solve for constants of integration. It also presents a method to find the point of intersection between two vector functions by equating their components and solving the resulting system of equations. The intersection point is then used to determine the ordered triple that represents the common point on both curves.
📐 Angle of Intersection Between Vector Functions
This final paragraph focuses on determining the angle of intersection between two vector functions at their point of intersection. It explains that the angle is found by considering the tangent vectors to the curves at the intersection point and using the dot product to calculate the cosine of the angle between them. The process involves evaluating the derivatives of the vector functions at the intersection point, finding the tangent vectors, and then using these vectors in the dot product formula to find the angle, which is then approximated to the nearest degree.
Mindmap
Keywords
💡Derivative
💡Integral
💡Vector-Valued Function
💡Tangent Vector
💡Parametric Equations
💡Magnitude
💡Orthogonal
💡Unit Tangent Vector
💡Dot Product
💡Angle of Intersection
Highlights
Introduction to derivatives and integrals of vector functions in calculus.
Definition of the derivative of a vector-valued function and its geometric interpretation.
Visual representation of the derivative as a tangent vector to a space curve.
Differentiation of vector-valued functions by differentiating each component.
Example calculation of the derivative of a given vector-valued function.
Finding the parametric equations of the tangent line to a curve at a specific point.
Differentiation rules for vector-valued functions including the product and chain rules.
Proof that if the magnitude of a vector-valued function is constant, its derivative is orthogonal to the function.
Introduction and calculation of the unit tangent vector.
Integration of vector functions done component-wise similar to real-valued functions.
Example of integrating a vector-valued function using integration by parts.
Finding the original vector-valued function given its derivative and an initial condition.
Determining the point of intersection between two vector functions.
Calculating the angle of intersection between two curves using the dot product of their tangent vectors.
Practical application of finding the angle between curves and its significance in understanding geometric relationships.
Upcoming topics on arc length and curvature in future lessons.
Transcripts
Browse More Related Video
Calculus 3: Vector Functions and Space Curves (Video #7) | Math with Professor V

Derivative of a position vector valued function | Multivariable Calculus | Khan Academy
AP Calculus BC Lesson 9.4

Derivatives and Tangent Vectors (Vector-Valued Functions)

Calculus 3: Arc Length and Curvature (Video #9) | Math with Professor V
Video 08 - Vector Differentiation
5.0 / 5 (0 votes)
Thanks for rating: