Applications with Separable Equations (Differential Equations 14)
TLDRThe video delves into the application of differential equations, focusing on natural growth and decay modeled by exponential functions. It explains how these concepts apply to real-life scenarios, such as population growth, radioactive decay, and Newton's law of cooling. The video demonstrates how to set up and solve problems involving initial values and factors, as well as how to estimate unknowns like the time of death in a forensic context. The content is engaging and informative, offering a deep dive into the practical uses of differential equations.
Takeaways
- 📚 Differential equations are crucial for understanding natural growth and decay processes, such as population dynamics and radioactive decay.
- 🔢 The technique of separable equations is fundamental for solving basic types of differential equations and understanding their applications.
- 📈 Exponential growth and decay models are often used to represent natural phenomena where the rate of change is proportional to the current amount.
- 🕒 The concept of half-life is introduced as a key parameter in radioactive decay problems, where it represents the time it takes for half of the radioactive material to decay.
- 🧠 The importance of understanding the setup and wording of word problems is emphasized, as it is often the most challenging part of solving differential equation problems.
- 🎯 The video script provides a structured approach to solving problems by first identifying the type of problem (initial value or factor-based) and then applying the appropriate mathematical techniques.
- 🤔 The script highlights the need to differentiate between problems where an initial population or amount is given and those where a factor of change after a certain time is provided.
- 📊 Newton's law of cooling is discussed as an example of a differential equation that describes the rate of temperature change in an object relative to its surroundings.
- 🏞️ The concept of barometric pressure and how it changes with altitude is explored, demonstrating the application of differential equations in real-world scenarios.
- 🕵️♂️ A practical application of differential equations in estimating the time of death based on body cooling is presented, showing how these mathematical tools can aid in forensic investigations.
- 👨🏫 The video emphasizes the educational value of working through numerous examples to build confidence and competence in solving differential equation problems.
Q & A
What is the main focus of the video?
-The main focus of the video is to discuss the applications of differential equations, specifically separable equations, in real-life scenarios such as natural growth and decay, including exponential growth and decay.
Why are applications of differential equations important?
-Applications of differential equations are important because they demonstrate how these mathematical concepts can be used to model and solve real-world problems, thereby showing their practical utility and relevance.
What is the basic technique discussed for solving differential equations in the video?
-The basic technique discussed for solving differential equations in the video is by separating the variables, which is used for solving certain types of first-order differential equations.
How does the video demonstrate the setup of a differential equation for a real-world problem?
-The video demonstrates the setup of a differential equation for a real-world problem by using the example of population growth. It shows how the rate of change of the population is proportional to the current population size and leads to an exponential growth model.
What is the significance of the constant 'e' in the context of natural growth and decay models?
-The constant 'e' is significant in the context of natural growth and decay models because it is the base of the exponential functions that naturally arise when solving these types of problems. It represents the natural growth rate in these models.
How does the video address the challenge of setting up word problems in differential equations?
-The video addresses the challenge of setting up word problems in differential equations by emphasizing the importance of understanding the problem statement and identifying the key variables and their relationships. It provides a step-by-step approach to translating the problem into a mathematical model.
What is the role of integrals in solving separable differential equations?
-Integrals play a crucial role in solving separable differential equations by allowing the separation of variables and the simplification of the equation into a form that can be solved for the unknown function. The process involves integrating both sides of the equation after separating variables.
How does the video handle the concept of exponential growth and decay in the context of population dynamics?
-The video handles the concept of exponential growth and decay in the context of population dynamics by showing that the rate of change in population is proportional to the current population size. This leads to an exponential model where the population grows or decays at a rate that increases or decreases exponentially over time.
What is the significance of the 'K' constant in the differential equation for population growth?
-The 'K' constant in the differential equation for population growth represents the rate of change or the growth factor. A positive 'K' indicates exponential growth, while a negative 'K' indicates exponential decay. The value of 'K' is determined by the specific growth dynamics of the population being modeled.
How does the video approach the solution of a differential equation for a town's population growth?
-The video approaches the solution of a differential equation for a town's population growth by first setting up the equation based on the given initial population and the population after a certain number of years. It then solves for the 'K' constant using the given data points and finally uses the 'K' constant to predict the population at a future time point.
Outlines
📚 Introduction to Differential Equations and Applications
The video begins with an introduction to differential equations, emphasizing their importance in various real-world applications. The speaker highlights the technique of solving first-order differential equations by separating variables, particularly useful for modeling natural growth and decay processes such as exponential growth and decay. The focus is on understanding how these mathematical concepts apply to real-life scenarios, with an emphasis on the practicality of mathematics in solving such problems.
📈 Exponential Growth and Decay: A Town's Population
The speaker delves into the concept of exponential growth and decay using the example of a town's population. They explain how the population growth can be modeled as an exponential function, where the rate of change is proportional to the current population. The video demonstrates how to set up and solve a differential equation to predict the population in the year 2010, given the growth rate and initial population size. The process involves separating variables, integrating, and solving for the constant of variation.
🧬 Population Growth and the Natural Logarithm
This section further explores the mathematical modeling of population growth through differential equations. The speaker explains how the natural logarithm plays a crucial role in these models, leading to the exponential form of growth or decay. They illustrate this with a step-by-step walkthrough of solving a differential equation, highlighting the importance of understanding the setup and the thought process behind solving such problems.
📊 Solving for Population Growth: A Step-by-Step Guide
The speaker provides a detailed guide on solving for population growth using differential equations. They explain the process of separating variables, integrating, and solving for the constant of variation, emphasizing the importance of understanding the problem structure. The video shows how to use given information, such as initial population and population after a certain time, to find the growth constant and predict future population sizes.
🔄 Understanding Exponential Growth and Decay in Applications
The speaker discusses the two main types of problems encountered when dealing with exponential growth and decay: those with a specific initial amount and those with a certain amount after a certain time. They explain how to approach each type, emphasizing the need to solve for the constant of variation and use it to answer questions about future or past amounts. The section also touches on the concept of half-life and its application in decay problems.
🌡️ Newton's Law of Cooling and Its Differential Equation
The speaker introduces Newton's Law of Cooling, explaining how the rate of temperature change is proportional to the difference between the object's temperature and the ambient temperature. They derive the differential equation for Newton's Law of Cooling and discuss how it can be separated into variables for easier solving. The video provides a foundation for understanding how temperature changes over time in various scenarios, such as cooling objects or heating/cooling processes.
🍺 Newton's Law of Cooling: Solving for Time and Temperature
The speaker applies Newton's Law of Cooling to a real-world scenario of cooling a soda can and explains how to solve for the time it takes to reach a desired temperature. They walk through the process of solving the differential equation, including finding the constant of variation and using initial and final temperature values. The section demonstrates how to use the derived equation to predict the cooling process and answer questions about temperature changes over time.
💉 Drug Dosage and Half-Life: A Mathematical Approach
The speaker discusses the mathematical aspects of drug dosage and half-life, explaining how the amount of a drug in the body decreases over time due to its half-life. They describe how to calculate the initial dosage required to ensure the drug remains effective for a specific duration, using the concept of exponential decay. The video provides a practical example of applying mathematical models to real-world medical situations, highlighting the importance of precision in drug administration.
🌬️ Barometric Pressure and Differential Equations
The speaker explores how barometric pressure changes with altitude using a differential equation. They explain that as altitude increases, barometric pressure decreases, and provide a formula to calculate the pressure at different altitudes. The video demonstrates how to use calculus to separate variables and solve for the constant of variation, allowing for the prediction of barometric pressure at various heights above sea level.
🗣️ Rumor Spreading as a Differential Equation
The speaker presents a unique application of differential equations to model the spread of information in a town, using the example of a treasure discovery. They explain how the rate of increase in the number of people who know about the treasure is proportional to the number of people who do not yet know. The video shows how to set up and solve the differential equation, using initial and subsequent data points to find the constant of variation and predict the spread of knowledge over time.
🕵️♂️ Estimating Time of Death Using Newton's Law of Cooling
The speaker discusses how Newton's Law of Cooling can be used to estimate the time of death in forensic investigations. They describe a scenario where a body's temperature is measured at different times post-mortem and explain how to use these measurements to calculate the time since death. The video demonstrates the practical application of differential equations in real-world situations, highlighting the importance of accurate data and mathematical modeling in such cases.
Mindmap
Keywords
💡Differential Equations
💡Separable Equations
💡Exponential Growth and Decay
💡Newton's Law of Cooling
💡Half-Life
💡Initial Value Problems
💡Barometric Pressure
💡Word Problems
💡Applications of Mathematics
💡Natural Growth and Decay
Highlights
Introduction to differential equations and their applications in natural growth and decay.
Discussion on the basic technique of solving differential equations by separating variables.
Explanation of exponential growth and decay in the context of natural phenomena.
Importance of understanding applications of differential equations in real-life situations.
Presentation of a mathematical model for population growth based on the principle that the growth rate is proportional to the current population size.
Illustration of how to set up and solve a differential equation for a town's population growth.
Introduction to the concept of half-life and its application in problems involving radioactive decay.
Explanation of how to use the half-life to determine the age of an object based on the remaining amount of a radioactive isotope.
Discussion on Newton's law of cooling and its relevance in determining the time it takes for an object to cool down to a certain temperature.
Presentation of a method to estimate the time of death using Newton's law of cooling and the initial and final body temperatures.
Explanation of how the rate of change in barometric pressure decreases with altitude due to the weight of the atmosphere.
Demonstration of calculating the altitude at which the barometric pressure drops to a specific value.
Discussion on the spread of information in a town as a differential equation problem.
Illustration of how to model the spread of knowledge about a treasure discovery in a town using a differential equation.
Explanation of the structure and setup of differential equation problems involving initial values and factors.
Presentation of a method to solve for the initial amount in differential equation problems where the amount after a certain time is given.
Discussion on the three main types of differential equation problems and how to approach them.
Explanation of how to use the concept of half-life to solve for the time it takes for a drug to become ineffective based on its decay rate.
Presentation of a method to calculate the initial dosage of a drug needed to ensure its effectiveness for a specific duration.
Transcripts
Browse More Related Video
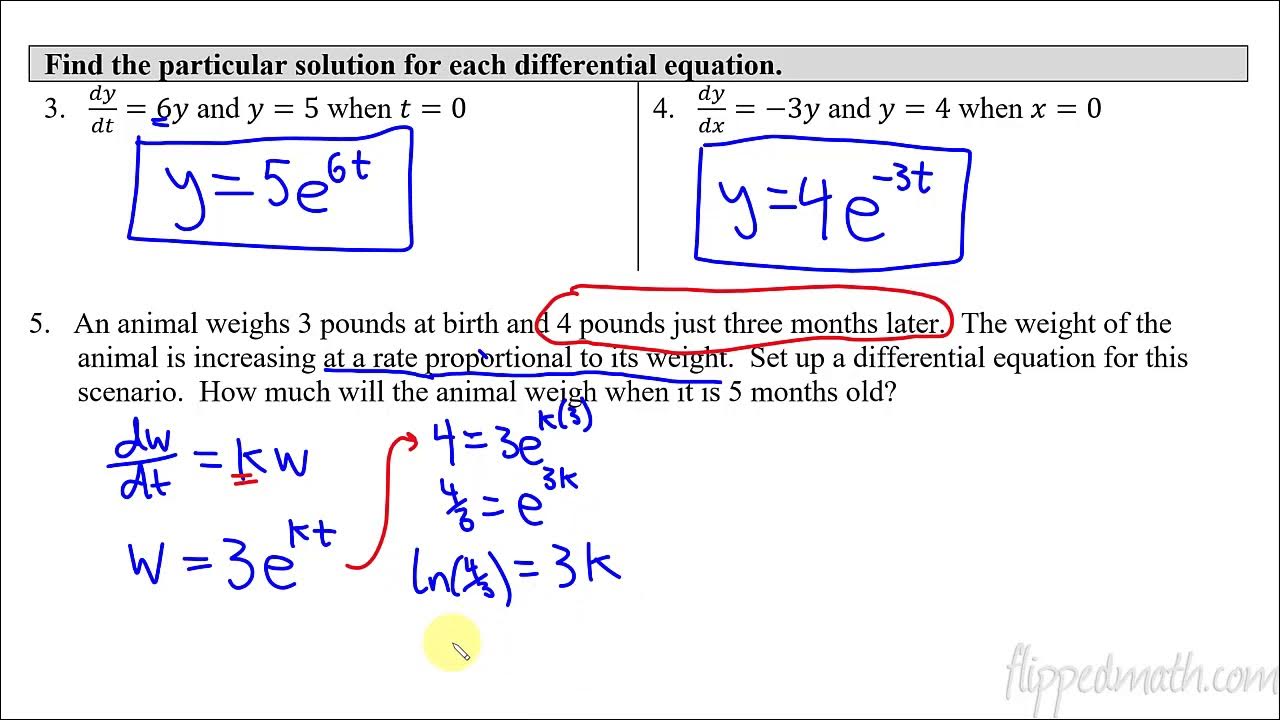
Calculus AB/BC – 7.8 Exponential Models with Differential Equations
Calculus AB Homework 7.1 Differential Equations

Calculus 2 Lecture 8.1: Solving First Order Differential Equations By Separation of Variables

AP Calculus AB - 7.8 Exponential Models With Differential Equations

Exponential Growth and Decay (Precalculus - College Algebra 66)

Exponential Growth and Decay BC Calc
5.0 / 5 (0 votes)
Thanks for rating: