Exponential Growth and Decay (Precalculus - College Algebra 66)

Professor Leonard

12 Nov 202048:26

EducationalLearning

32 Likes 10 Comments

TLDRThe video script delves into the concept of exponential growth and decay, a fundamental topic in mathematics with applications in various fields such as finance, population modeling, and physics. It begins by explaining the basics of exponential functions and their relation to continuously compounded interest. The presenter then explores three main examples: bacterial population growth, radioactive decay including the concept of half-life, and Newton's law of cooling. Each example is meticulously explained, with a focus on understanding the underlying principles rather than just applying formulas. The script emphasizes the importance of identifying the rate of change (k) in exponential models and uses logarithms to solve for variables within these models. The examples provided are designed to illustrate the practical applications of exponential growth and decay, such as determining the time it takes for bacteria to reach a certain population size or for an object to cool down to a specific temperature. The video aims to equip viewers with a solid understanding of exponential processes and their real-world implications.

Takeaways

📈 Exponential growth and decay are modeled using differential equations, which are used to describe phenomena like population changes and radioactive decay.
🧮 The formula for exponential growth is strongly related to continuously compounded interest in finance, where the initial amount grows at a rate over time.
⏱️ The variable 't' in the exponential model represents the time period, which can be measured in various units such as years, hours, or minutes.
🔄 The rate 'k' determines the direction of the exponential function; a positive 'k' indicates growth, while a negative 'k' indicates decay.
🌱 In the context of population growth, the initial amount (a sub 0) represents the starting population, and 'a' is the future population size after a certain time period 't'.
⏳ The concept of half-life is used in exponential decay to describe the time it takes for a substance to reduce to half of its initial amount.
📉 Exponential decay has a horizontal asymptote, meaning that a substance never completely disappears but gets infinitely close to zero.
🔢 The formula for exponential growth or decay can be manipulated using logarithms to solve for variables such as the time 't' it takes to reach a certain population size.
🍗 Newton's law of cooling is a specific application of exponential decay that describes how the temperature of an object changes over time as it approaches the ambient temperature.
🕒 The rate of cooling or heating can be determined by analyzing the change in temperature over a known time period, which helps to predict future temperatures.
🔧 Logarithms are essential tools for solving exponential equations, allowing us to isolate variables and find the time it takes for an exponential process to reach a certain value.

Q & A

What is the main topic of the video?
-The video discusses exponential growth and decay, including applications in population modeling, finance, and physics.
What is the mathematical model used for exponential growth?
-The mathematical model for exponential growth is A = P * e^(k*t), where A is the future value, P is the present value, e is the base of the natural logarithm, k is the growth rate, and t is the time period.
How is exponential decay different from exponential growth?
-Exponential decay involves a negative rate (k), which results in a decreasing function over time, unlike exponential growth which has a positive rate and increases over time.
What is the concept of continuously compounded interest?
-Continuously compounded interest is a method of calculating interest where the interest is added to the principal continuously, and it is closely related to exponential growth functions.
What is Newton's Law of Cooling?
-Newton's Law of Cooling is a principle that describes how the temperature of an object changes over time as it approaches the ambient temperature of its surroundings.
What is the significance of the rate (k) in exponential models?
-The rate (k) determines the direction and speed of growth or decay. A positive k indicates growth, while a negative k indicates decay.
How does the concept of half-life relate to exponential decay?
-Half-life is the time required for a quantity to reduce to half its initial value. It is a specific application of exponential decay, particularly used in the context of radioactive decay.
What is the formula used to calculate the time it takes to reach a certain population size in an exponential growth model?
-The formula is A = P * e^(k*t), where A is the desired future population size, P is the initial population size, k is the growth rate, and t is the time in question.
What is the role of logarithms in solving exponential equations?
-Logarithms are used to isolate and solve for the variable t (time) in exponential equations, by using the property that log_b(a) = c is equivalent to b^c = a.
How does the video script illustrate the concept of doubling time in an exponential growth context?
-The script uses the example of a bacterial population that doubles every three hours to explain doubling time, showing how to calculate the time it takes for an initial population to double in size.
What is the importance of matching units of time when working with exponential models?
-Matching units of time is crucial to ensure the accuracy of the model. It ensures that the rate of growth or decay is applied consistently over the correct time period.

Outlines

00:00

📈 Introduction to Exponential Growth and Decay

The video begins by introducing the topic of exponential growth and decay, also known as natural growth and decay or uninhibited growth and decay. It explains that these concepts involve differential equations and can be applied to model population changes and financial growth, such as continuously compounded interest. The presenter emphasizes the importance of understanding the underlying principles before delving into more complex models like logistic growth.

05:02

🧮 Understanding Exponential Functions and Rates

The presenter discusses the mathematical representation of exponential growth and decay, highlighting the role of the base 'e' and the rate 'k'. It is explained that a positive 'k' results in growth, while a negative 'k' leads to decay. The concept of continuously compounded interest is compared to population growth, with the initial amount, rate, and time period being key variables. The video also clarifies that the time period 't' can be measured in various units, not just years.

10:08

🌱 Exponential Growth in Bacterial Population

An example is given to illustrate exponential growth, using a bacterial population that starts with 100 units and grows at a rate of 4.5% per hour. The presenter shows how to calculate the population size after a given time period and how to determine the time required to reach a specific population size. The process involves using the exponential growth formula and applying logarithms to solve for the unknown time variable.

15:09

📊 Creating an Exponential Growth Model from Scratch

The video demonstrates how to create an exponential growth model when given information about a population that doubles every three hours. The presenter guides the viewer through finding the rate 'k' by using the doubling time and the initial population size. Once the rate is determined, the presenter shows how to use the exponential growth formula to answer various questions about the population's growth over time.

20:10

📉 Exponential Decay and Half-Life

The concept of exponential decay is introduced, with a focus on half-life as an example. The presenter explains that half-life is the time it takes for a substance to reduce to half of its initial amount. Using the half-life of radium as an example, the video shows how to calculate the remaining amount of a substance after a given time period and how to solve for the decay rate 'k'.

25:10

🕰️ Dating with Carbon-14 and Half-Life

The video explores the application of half-life in dating ancient objects, such as a dead tree, using the example of carbon-14. The presenter explains how to calculate the time it takes for the carbon-14 in the tree to decrease to a certain percentage of its original amount, which can be used to estimate the tree's age. The process involves solving for the decay rate 'k' and then using it to find the time variable 't'.

30:13

🌡️ Newton's Law of Cooling

The final topic covered is Newton's law of cooling, which describes the rate at which an object's temperature changes to match the ambient temperature. An example involving a turkey cooling down after being taken out of the oven is used to illustrate the concept. The presenter shows how to calculate the time it takes for the turkey to cool to a safe temperature for handling, using the initial temperature, the ambient temperature, and the future temperature as variables in the formula.

35:14

🔢 Moving On to Sequences and Series

The video concludes with a brief mention of the next topics to be covered: sequences and series, arithmetic and geometric sequences, and proofs of induction. The presenter expresses hope that the viewers found the discussion on exponential growth and decay interesting and that it provided a solid foundation for understanding related mathematical concepts.

Mindmap

Keywords

💡Exponential Growth

Exponential growth refers to a process where a quantity increases at a rate proportional to its current value. In the video, it is discussed in the context of population growth and finance, where the base e (Euler's number) is used to model continuous growth. An example given is bacterial growth, which increases by 4.5% every hour, demonstrating the concept of growth over time.

💡Exponential Decay

Exponential decay is the inverse of exponential growth, where a quantity decreases at a rate proportional to its current value. The video script explains this concept using the example of half-life, where substances like radium lose half of their mass over a specific period. It is characterized by a negative rate (k), which results in a decreasing exponential function.

💡Differential Equations

Differential equations are mathematical equations that involve rates of change and are essential for modeling phenomena in fields such as physics, biology, and economics. The video touches on differential equations as the mathematical foundation for exponential growth and decay models, although it does not delve deeply into the topic.

💡Continuously Compounded Interest

Continuously compounded interest is a method of calculating interest where the interest is added to the principal continuously, rather than at discrete intervals. The video script draws a parallel between this financial concept and exponential growth, highlighting that both involve the base e and demonstrate how population growth models can be analogous to financial growth models.

💡Half-Life

Half-life is a concept used to describe the time it takes for a substance to decay to half of its initial amount. It is a key concept in nuclear physics and is used in the video to illustrate exponential decay. The script explains how to calculate the remaining amount of a substance like radium after a given number of years using its half-life.

💡Newton's Law of Cooling

Newton's Law of Cooling is a formula that describes how the temperature of an object changes over time as it approaches the ambient temperature. The video uses the example of a turkey cooling after being taken out of the oven to explain how the law can be applied to real-life scenarios. It involves an exponential approach to the ambient temperature, which is a horizontal asymptote in the model.

💡Logistic Modeling

Logistic modeling is a mathematical model used to describe growth that has an upper limit, such as population growth in an environment with limited resources. Although not the main focus of the video, it is mentioned as a more complex topic that builds upon the understanding of exponential growth and decay.

💡Rate (k)

In the context of the video, the rate (k) is a constant that determines the speed of exponential growth or decay. A positive k indicates growth, while a negative k indicates decay. The rate is crucial for solving for the future value in exponential models and is derived from the initial and future conditions of the modeled phenomenon.

💡Base of Exponential Function

The base of an exponential function is the number that is raised to the power of an exponent in the function. In the video, the base e is used for modeling growth and decay continuously over time. The base affects the shape of the exponential curve, with a base greater than 1 leading to growth and a base less than 1 leading to decay.

💡Horizontal Asymptote

A horizontal asymptote is a horizontal line that an exponential function approaches but never actually reaches. In the context of the video, it is shown that exponential decay models have a horizontal asymptote at y=0, indicating that the quantity being modeled never truly reaches zero but gets infinitely close as time progresses.

💡Time Period (t)

The time period (t) is a variable in the exponential growth or decay model that represents the elapsed time. In the video, it is used to calculate future values based on the initial amount and the rate of growth or decay. The time period can be measured in various units such as minutes, hours, or years, depending on the context of the problem.

Highlights

Introduction to exponential growth and decay, and their applications in modeling population dynamics and financial interests.

Explaining the concept of differential equations and their fundamental role in understanding exponential growth and decay models.

Connection between exponential functions and continuously compounded interest, highlighting the mathematical similarities.

Explanation of how positive and negative rates (k) affect growth and decay, respectively, and the underlying mathematical reasoning.

Practical example of bacterial population growth, illustrating how to apply exponential growth formulas to real-world situations.

Detailed walkthrough of calculating future population sizes and the time required to reach specific growth milestones.

Discussion on the concept of half-life and its application in understanding radioactive decay and other decay processes.

Methodology for determining the rate of decay (k) using half-life information and its implications.

Application of exponential decay in dating ancient artifacts using carbon-14, demonstrating the practical use of half-life in archaeology.

Introduction to Newton's law of cooling, explaining how it models the rate at which an object's temperature changes over time.

Real-world example of Newton's law of cooling applied to a turkey cooling down after being removed from the oven, illustrating the law's practical use.

Explanation of how Newton's law of cooling can be used to calculate the time it takes for an object to reach a desired temperature.

Discussion on the theoretical aspects of Newton's law of cooling, including its limitations and the concept of an asymptote in temperature change models.

Transition from exponential growth and decay to sequences and series, setting the stage for further mathematical exploration.

Emphasis on the importance of understanding the underlying concepts in mathematics to apply formulas effectively and accurately.

Transcripts

Browse More Related Video

AP Calculus AB - 7.8 Exponential Models With Differential Equations

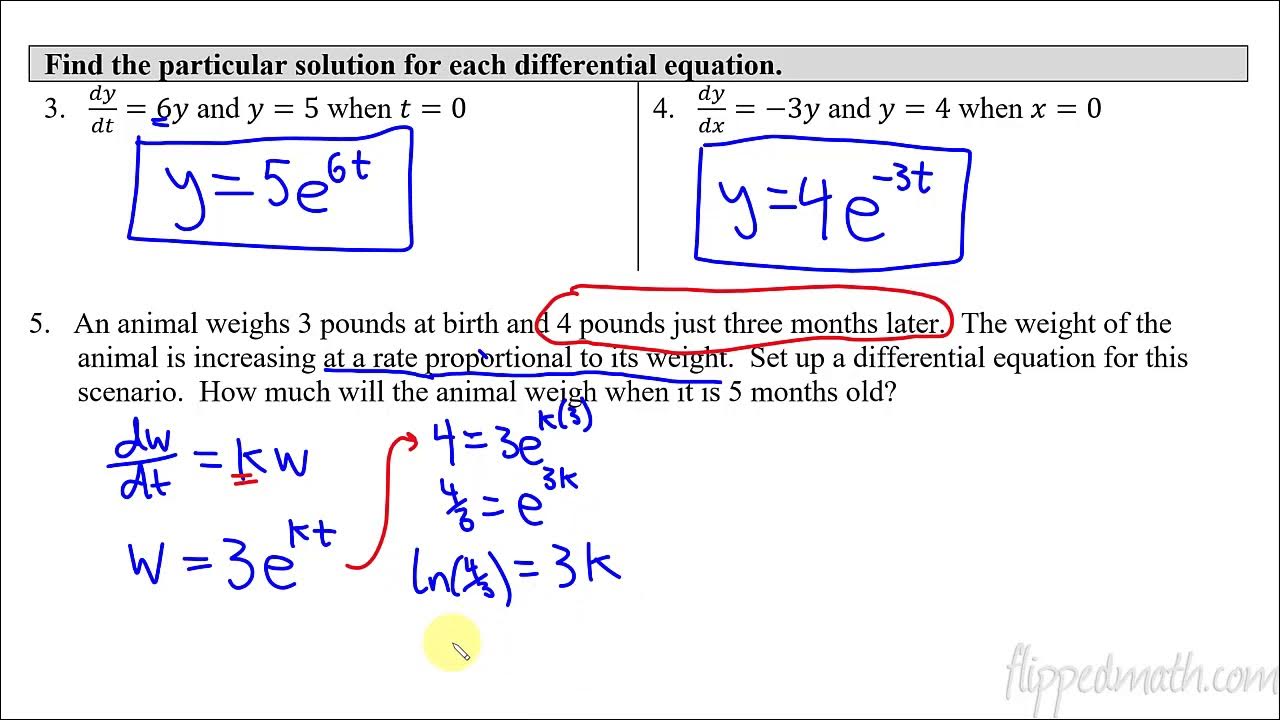
Calculus AB/BC – 7.8 Exponential Models with Differential Equations

Calculus Chapter 3 Lecture 18 A Simple ODE

Exponential Growth and Decay Word Problems & Functions - Algebra & Precalculus

Business Calculus - Math 1329 - Section 4.1 - Exponential Functions and Continuous Compounding

Applications with Separable Equations (Differential Equations 14)

Related Tags

Exponential Growth Exponential Decay Population Modeling Finance Math Newton's Law Differential Equations Continuous Compounding Half-Life Carbon Dating Mathematical Modeling Educational Content