Differential equation from slope field | First order differential equations | Khan Academy
TLDRIn this video, the presenter reverse-engineers a differential equation from a given slope field. By selecting points with simple arithmetic, such as (1,1), and calculating the slope at those points, the presenter eliminates options until identifying the correct equation: dy/dx = x + y. The video illustrates how the slope increases with x and y, leading to potential solution curves and an asymptote at y = -x - 1, providing a clear and engaging explanation of the process.
Takeaways
- 🔍 The video discusses the process of identifying a differential equation from a given slope field.
- 🧐 The viewer is encouraged to pause the video and attempt to identify the differential equation independently.
- 📐 The method involves selecting points with simple arithmetic, such as (x=1, y=1), to test against the slope field.
- ✅ The first equation is ruled out because the calculated slope (-1) does not match the positive slope shown in the field.
- ✅ The second equation is also ruled out as the calculated slope (0) does not match the observed positive slope.
- 🤔 The third equation is considered a potential match when x and y are both 1, giving a slope of 2, which aligns with the slope field.
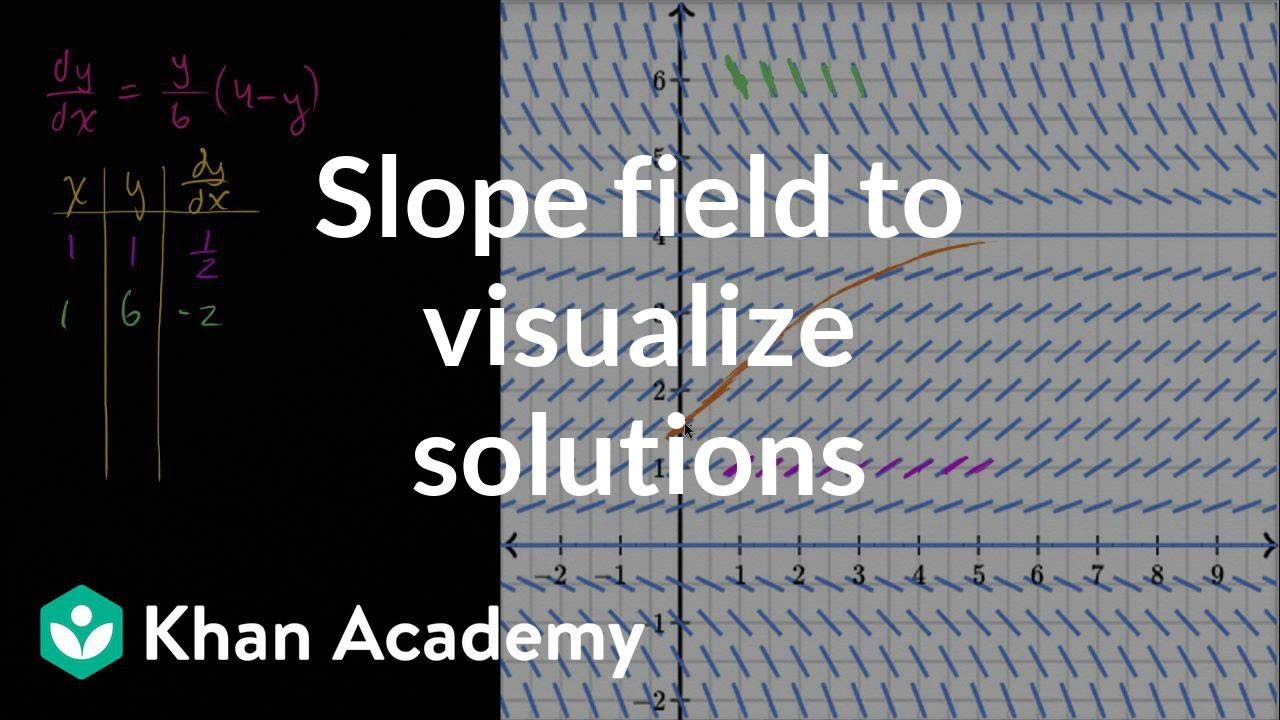
- 📈 The slope field illustrates how the slope increases with higher x and y values, and decreases towards the bottom left.
- 💡 The video suggests that the slope field is consistent with the differential equation dy/dx = x + y.
- 🌐 The solutions to the differential equation are discussed, with potential curves and asymptotes being analyzed.
- 🔗 The line y = -x - 1 is identified as an asymptote for the solutions of the differential equation dy/dx = x + y.
Q & A
What is the main goal of the exercise in the video?
-The main goal of the exercise is to start with a given slope field and determine the differential equation that the slope field is describing.
How does the speaker suggest approaching the problem?
-The speaker suggests choosing points with simple arithmetic, such as x=1 and y=1, to check if the slope from the differential equation matches the slope in the slope field.
What is the first differential equation the speaker tests?
-The first differential equation tested is dy/dx = -1/y, and it is ruled out because the calculated slope at x=1, y=1 does not match the slope field.
What is the second differential equation considered in the video?
-The second one is dy/dx = 1 - y/x, which is also ruled out because the calculated slope at x=1, y=1 does not match the slope field.
Which differential equation does the speaker find to be a good candidate?
-The speaker finds dy/dx = x + y to be a good candidate because the calculated slope at x=1, y=1 matches the slope field.
How does the slope behave in the slope field for the differential equation dy/dx = x + y?
-The slope increases as x increases for a given y and also increases as y increases for a given x, with slopes becoming more negative towards the bottom left.
What does the speaker conclude about the differential equation after testing several options?
-The speaker concludes that the differential equation dy/dx = x + y is the one being described by the slope field.
What is the potential solution path for the differential equation dy/dx = x + y?
-The potential solution paths depend on the starting points, and they might asymptote towards the line y = -x - 1.
How can the line y = -x - 1 be verified as a solution to the differential equation?
-If y = -x - 1, then differentiating both sides with respect to x gives dy/dx = -1, which matches the given differential equation.
What is the significance of the slope field in understanding differential equations?
-A slope field visually represents the slopes of solutions to a differential equation, which helps in understanding the behavior of the solutions and in finding the actual equations.
What method does the speaker use to validate the chosen differential equation?
-The speaker validates the chosen differential equation by checking multiple points and observing the behavior of the slopes in the slope field, ensuring they match the calculated slopes.
Outlines
📊 Deductive Analysis of a Slope Field
This paragraph introduces a methodical approach to deducing the underlying differential equation from a given slope field. The voiceover guides the viewer through a process of elimination, using specific points (x=1, y=1) to test against various differential equations. The key points include the use of arithmetic to find the derivative at chosen points and comparing these values to the slopes shown in the slope field. The paragraph emphasizes the importance of understanding how the slope changes with respect to x and y, and how this can help identify the correct differential equation. The process leads to the identification of the equation dy/dx = x + y as the likely candidate describing the slope field, with a brief mention of the solutions' behavior and asymptotic properties.
🔍 Further Exploration of the Differential Equation's Solutions
This paragraph continues the analysis by exploring the implications of the identified differential equation, dy/dx = x + y, on the solutions' paths. It discusses the asymptotic behavior of the solutions, suggesting that they approach the line y = -x - 1, which is derived from the differential equation itself. The paragraph also touches on the verification of this line as an integral part of the solution set, highlighting the importance of understanding the relationship between the differential equation and its solutions. The summary ends with a reflection on the interesting nature of the problem and an invitation for the viewer to engage with the content.
Mindmap
Keywords
💡differential equation
💡slope field
💡solutions
💡arithmetic
💡derivative
💡visualize
💡increase
💡asymptote
💡negative
💡cancel out
Highlights
The video discusses the process of deriving a differential equation from a given slope field.
The method involves selecting points with simple arithmetic to check the consistency of the slopes.
The first differential equation tested results in a negative slope at the point (x=1, y=1), which does not match the slope field.
The second equation yields a slope of zero at the same point, which also does not align with the slope field.
The third equation, where dy/dx equals one minus y, results in a slope of zero at (x=1, y=1), again not matching the slope field.
The fourth equation, dy/dx = x + y, gives a slope of two at (x=1, y=1), which is consistent with the slope field.
As x increases with a constant y, the slope increases, which is reflected in the slope field.
Similarly, increasing y with a constant x also increases the slope, as observed in the slope field.
The slope field indicates an increase in slope towards the top right and a decrease towards the bottom left.
The equation dy/dx = x + y is identified as the most likely differential equation describing the slope field.
The video also explores the implications of the derived equation on the shape of its solutions.
Potential solutions are discussed, with their behavior depending on the starting points.
The solutions appear to asymptote towards the line y = -x - 1, based on the derived differential equation.
The line y = -x - 1 is confirmed to be a solution to the differential equation y = -x - 1.
The process demonstrated in the video is a practical application of understanding and working with differential equations.
The video encourages viewers to pause and attempt the exercise independently before revealing the solution.
The method used in the video can be applied to other similar exercises involving slope fields and differential equations.
The video provides a clear and detailed explanation of how to match a slope field to its corresponding differential equation.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: