1. Periodic Oscillations, Harmonic Oscillators

MIT OpenCourseWare

18 Apr 201857:07

EducationalLearning

32 Likes 10 Comments

TLDRIn this engaging lecture, Professor Yen-Jie Lee introduces the fundamental concepts of vibrations and waves, emphasizing their importance in understanding the universe. By exploring the relationship between physical phenomena and mathematical representations, Lee demonstrates how harmonic oscillators can be analyzed using Newton's laws and Hooke's law. The lecture delves into the solutions of a mass-spring system, highlighting the applicability of Hooke's law for small oscillations and the elegance of complex exponential functions in representing harmonic motion. Lee also touches on the limitations of linear approximations and the need to consider nonlinear effects for larger amplitudes, providing a foundation for future discussions on damping forces and more complex systems.

Takeaways

🌟 Understanding vibrations and waves is crucial as they are fundamental to comprehending the universe, including sound and light waves, and even gravitational waves.
📚 The course 8.03 is closely related to classical mechanics, optics, quantum mechanics, and has numerous practical applications.
🔢 Mathematics is highlighted as a powerful tool in solving physical problems, with the ability to translate physical situations into mathematical models.
🏋️‍♂️ The simple harmonic oscillator, such as a mass attached to a spring, is used as a starting point to illustrate the principles of vibrations and waves.
📈 Newton's law and Hooke's law are applied to derive the equation of motion for the spring-mass system, leading to a second-order differential equation.
🦄 The solution to the equation of motion is a cosine function, representing a harmonic oscillation with amplitude and angular frequency determined by initial conditions.
🔄 The principle of superposition and time translation invariance are discussed as properties of the linear equation of motion.
🔁 The concept of Taylor expansion is introduced to explain the applicability of Hooke's law for small oscillations around an equilibrium position.
📊 The lecture demonstrates the power of mathematics by showing how the theoretical predictions match the actual physical behavior of the spring-mass system.
🌀 Nonlinear effects are introduced, showing that for large oscillations, the simple harmonic motion approximation may not hold, and higher-order terms become significant.
🔮 The complex exponential function is introduced as an alternative representation of the solution, highlighting its mathematical advantages and the concept of rotation in the complex plane.

Q & A

Why is it important to study vibrations and waves in physics?
-Studying vibrations and waves is crucial because it helps us understand various phenomena in the universe, including the propagation of sound and light, quantum physics, and even recent discoveries like gravitational waves. These concepts are foundational to many areas of physics and have practical applications in technology and our understanding of the natural world.
What is the significance of probability density in the context of 8.03?
-Probability density is significant in 8.03 as it is closely connected to the study of waves. It is a fundamental concept in quantum physics, which is a part of the curriculum that builds upon the understanding of waves gained in 8.03.
How does the concept of a harmonic oscillator relate to the study of vibrations and waves?
-A harmonic oscillator is a key concept in the study of vibrations and waves. It is a system that oscillates at a frequency that is independent of the amplitude of oscillation. The analysis of a single harmonic oscillator, as well as the interaction between multiple oscillators, provides a foundation for understanding wave behavior and the mathematical models that describe it.
What is the role of mathematics in the study of physical systems?
-Mathematics is a powerful tool in the study of physical systems. It allows us to translate real-world situations into mathematical models, which can then be used to make predictions and solve problems. This is particularly important in understanding the behavior of oscillations and waves.
How does the spring-mass system demonstrate Hooke's Law?
-The spring-mass system demonstrates Hooke's Law through the relationship between the force exerted by the spring and the displacement of the mass. The force is proportional to the displacement (F = -kx), where 'k' is the spring constant and 'x' is the displacement from the equilibrium position. This linear relationship is what allows for simple harmonic motion.
What is the significance of the angular frequency (omega) in the equation of motion for a harmonic oscillator?
-The angular frequency (omega) in the equation of motion for a harmonic oscillator represents the rate of change of the oscillation's phase per unit time. It determines the frequency of the oscillation and is a crucial parameter in describing the system's periodic motion.
How does the concept of time translation invariance apply to the equation of motion for a harmonic oscillator?
-Time translation invariance means that the equation of motion for a harmonic oscillator remains unchanged if the time variable is shifted. This implies that the system's behavior at any point in time is identical to its behavior at any other point, assuming no external forces or damping effects are present.
What is the physical interpretation of the complex exponential function in the context of the harmonic oscillator?
-In the context of the harmonic oscillator, the complex exponential function is used to represent the solution to the equation of motion in a form that reveals the oscillator's behavior as a rotation in the complex plane. The real part of this function corresponds to the actual position of the oscillator as it oscillates over time.
How does the Taylor expansion help in understanding the applicability of Hooke's Law?
-Taylor expansion is used to approximate the potential energy function of a system near its equilibrium position. By expanding the potential function and considering only the leading terms (when the displacement is small), Hooke's Law can be derived, which states that the force is proportional to the displacement. This approximation is valid for small oscillations around the equilibrium point.
What are the implications of ignoring damping forces in the analysis of a harmonic oscillator?
-Ignoring damping forces can lead to an incomplete understanding of a system's behavior, especially over time. Damping forces, such as friction or air resistance, can cause the amplitude of oscillations to decrease over time, leading to a change in the system's energy and eventual cessation of motion. In a real-world scenario, these forces are often significant and must be considered for an accurate analysis.
How does the Euler's formula relate to the solutions of the harmonic oscillator's equation of motion?
-Euler's formula allows the representation of complex exponentials in terms of sine and cosine functions, which is useful in solving the differential equation of motion for a harmonic oscillator. This formula simplifies the mathematical analysis and provides a convenient way to express periodic motion, as it relates the complex exponential to its equivalent in polar form (magnitude and phase).

Outlines

00:00

📚 Introduction to Vibrations and Waves

The paragraph introduces the topic of vibrations and waves, emphasizing their importance in understanding the universe. It explains how sound and electromagnetic waves enable communication and perception. The instructor, Yen-Jie Lee, highlights the relevance of these concepts to other fields such as classical mechanics, quantum physics, and the recent discovery of gravitational waves. The goal is to use mathematics as a tool to solve physical problems and predict outcomes in experiments.

05:04

🧪 Experimenting with a Spring-Mass System

This section delves into a practical example of a spring-mass system to illustrate the concepts of vibrations and waves. The instructor sets up a scenario where a mass is attached to a spring and released, prompting a discussion on the forces acting on the mass and how to predict its motion. The use of mathematics, specifically Newton's law, is emphasized to solve the resulting physical question.

10:05

🔢 Applying Mathematics to Physical Laws

The paragraph focuses on the application of mathematics to physical laws, particularly Newton's law, to derive the equation of motion for the spring-mass system. It explains how defining a coordinate system and understanding the forces involved allows for the formulation of a second-order differential equation. The process of solving this equation using mathematical techniques is outlined, highlighting the power of mathematics in physics.

15:06

🌀 Understanding Harmonic Oscillation

This section discusses the solution to the differential equation for the spring-mass system, revealing it as a harmonic oscillation. The solution involves determining unknown coefficients using initial conditions. The concept of angular frequency and its relation to the motion of the mass is introduced, along with the idea of a harmonic oscillation as a universal solution for small perturbations around an equilibrium point.

20:09

🔄 Exploring the Implications of Hooke's Law

The paragraph examines the broader implications of Hooke's Law, noting its applicability to various systems with well-behaved potentials. It discusses the conditions under which Hooke's Law breaks down, particularly when the potential is not proportional to x squared or when the amplitude of oscillation is large. The concept of Taylor expansion is introduced to explain the近似 of Hooke's Law for small oscillations.

25:16

🌟 The Beauty of Complex Exponential Functions

This section introduces the complex exponential function as a elegant way to represent the solution to the equation of motion. It explains the mathematical properties of these functions, including their resilience to differentiation and their ability to represent time translation invariance. The paragraph also touches on the concept of Euler's formula and its utility in simplifying differential equations.

30:19

🚫 Acknowledging Real-World Complications

The final paragraph acknowledges the limitations of the idealized spring-mass system model presented in the lecture. It introduces the concept of damping forces, such as air resistance and friction, which affect the motion of the mass in real-world scenarios. The instructor sets the stage for future lectures where these complications will be incorporated into the analysis, promising a deeper understanding of how to solve such problems.

Mindmap

Keywords

💡Vibrations and Waves

Vibrations and waves are fundamental concepts in physics that describe the propagation of energy through space. In the context of the video, they are essential for understanding how sound travels through air as sound waves, allowing us to hear and communicate. Additionally, electromagnetic waves, such as light, are also discussed as they relate to our perception of the world around us.

💡Harmonic Oscillator

A harmonic oscillator is a system that oscillates at a constant frequency and can be modeled by a simple spring-mass system. In the video, the concept is used to demonstrate how physical systems can be described and predicted using mathematical equations, specifically Newton's laws of motion and Hooke's law.

💡Newton's Law

Newton's laws of motion are a set of three fundamental principles in classical mechanics that describe the relationship between the motion of an object and the forces acting upon it. In the video, Newton's second law, which states that force equals mass times acceleration (F=ma), is used to derive the equation of motion for a harmonic oscillator.

💡Hooke's Law

Hooke's law states that the force exerted by a spring is proportional to the displacement from its equilibrium position, both mathematically expressed as F = -kx, where k is the spring constant and x is the displacement. This law is crucial in understanding the behavior of harmonic oscillators and is discussed in the video as a fundamental principle that allows for the prediction of small oscillations around an equilibrium point.

💡Gravitational Waves

Gravitational waves are ripples in the fabric of spacetime caused by the acceleration of massive objects. They were predicted by Einstein's theory of general relativity and were first directly observed in 2015. In the video, gravitational waves are mentioned as an example of how the principles of vibrations and waves are relevant to understanding the universe at large scales.

💡Quantum Physics

Quantum physics, also known as quantum mechanics, is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, where classical mechanics no longer applies. The video touches on quantum physics as a field where wave-like phenomena, such as probability density waves, are central to understanding the behavior of particles.

💡Complex Exponential Function

A complex exponential function is a mathematical function that involves Euler's formula and is used to represent oscillatory behaviors in a more convenient form, especially in the context of differential equations. In the video, the complex exponential function is introduced as a way to elegantly express the solutions to the equations of motion for harmonic oscillators.

💡Euler's Formula

Euler's formula is a fundamental identity in mathematics that establishes a deep connection between trigonometric and exponential functions. It states that e^(ix) = cos(x) + i*sin(x), where e is the base of the natural logarithm, i is the imaginary unit, and x is any real number. This formula is crucial in the context of the video for understanding the complex exponential representation of harmonic oscillations.

💡Boundary Conditions

Boundary conditions are specifications of a function's values at the edges of its domain, which are essential in solving differential equations. In the context of the video, boundary conditions are mentioned as a critical aspect of understanding waves and their behavior in physical systems, particularly when dealing with the Fourier decomposition of waves.

💡Fourier Decomposition

Fourier decomposition is a mathematical method that breaks down a complex wave into a sum of simpler sinusoidal waves, each with a specific frequency, amplitude, and phase. This technique is fundamental in signal processing and the analysis of wave phenomena. In the video, Fourier decomposition is mentioned as a tool to understand the composition of waves arising from an infinite number of oscillating particles.

💡Nonlinear Effects

Nonlinear effects refer to changes in a system's behavior that do not follow a linear cause-and-effect relationship. These effects become significant when the system is subjected to large inputs or experiences extreme conditions, deviating from the predictable, small-scale linear behavior. In the video, nonlinear effects are discussed in the context of pendulum oscillations, where increasing the amplitude leads to changes in the oscillation frequency.

Highlights

The importance of understanding vibrations and waves is emphasized, as they are fundamental to recognizing the universe.

Sound waves and electromagnetic waves are discussed as essential for communication and perception.

The connection between 8.03 (vibrations and waves) and other fields such as classical mechanics, optics, and quantum mechanics is highlighted.

The role of mathematics as a powerful tool in solving physical problems is underscored.

The process of translating physical situations into mathematical equations is outlined.

The example of a spring-mass system is used to demonstrate the application of Newton's law and the concept of forces.

The three forces acting on the mass in the spring-mass system are identified: spring force, gravitational force, and normal force.

The equation of motion for the spring-mass system is derived and explained.

The solution to the differential equation for the spring-mass system is presented, involving cosine and sine functions.

The uniqueness theorem is mentioned, ensuring the single solution to the equation of motion.

Initial conditions are used to determine the unknown coefficients in the solution.

The concept of harmonic oscillation and angular frequency is introduced.

The experiment with the spring-mass system is conducted to verify the theoretical predictions.

The universality of Hooke's law for small oscillations around an equilibrium point is discussed.

The potential application of the spring-mass system equation to other systems with well-behaved potentials is explored.

The properties of the linear equation of motion, including superposition and time translation invariance, are explained.

The concept of a complex exponential function as a solution to the equation of motion is introduced.

Euler's formula is discussed as a fundamental mathematical tool for understanding exponential functions in the context of the course.

The implications of ignoring damping forces and the real-world complexities in the harmonic oscillator model are acknowledged.

The lecture concludes with a summary of the key points and an invitation for questions, emphasizing the practical applications and theoretical significance of the material covered.

Transcripts

Browse More Related Video

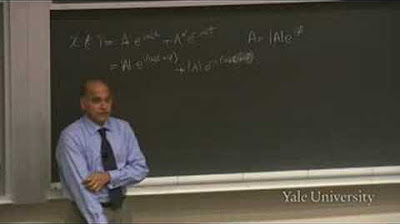
17. Simple Harmonic Motion

Simple Harmonic Motion, Mass Spring System - Amplitude, Frequency, Velocity - Physics Problems

01 - Oscillations And Simple Harmonic Motion, Part 1 (Physics Tutor)

Undamped Mechanical Vibrations & Hooke's Law // Simple Harmonic Motion

Simple Harmonic Motion: Hooke's Law

8.01x - Lect 10 - Hooke's Law, Springs, Pendulums, Simple Harmonic Motion

Related Tags

PhysicsEducation HarmonicOscillators HookesLaw MathematicalApplications MITLecture YenJieLee VibrationsAndWaves EducationalResources NewtonsLaw ComplexExponentials