Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (79 of 92) Simplified Transmission Coeff.

Michel van Biezen
8 May 201804:36
EducationalLearning
32 Likes 10 Comments

TLDRThis lecture demonstrates how to simplify the transmission coefficient equation for quantum tunneling by assuming that the barrier width is significantly large. The key insight is that when alpha times L (the barrier width) is much greater than one, the hyperbolic sine function simplifies to an exponential decay, allowing for a more manageable expression. This approximation is valid when alpha times L is considerably larger than one, facilitating easier calculations of particle amplitudes after passing through the barrier.

Takeaways
  • 📚 The lecture focuses on simplifying the transmission coefficient equation for cases where the barrier is wide.
  • 🔢 The simplification assumes that alpha times L (αL) is much greater than one.
  • 📈 The hyperbolic sine function is central to the equation, and its behavior changes significantly when αL is large.
  • 🌐 As αL increases, e to the minus αL approaches zero, and the hyperbolic sine function simplifies to e to the αL.
  • 💡 The simplification leads to the conclusion that the transmission coefficient can be approximated under certain conditions.
  • 🎯 The new form of the transmission coefficient is derived, relating to the energy of the particle and the potential of the barrier.
  • 📉 An exponential decay function, e to the minus 2αL, represents the decrease in wave function amplitude as it encounters the barrier.
  • 🌟 The simplification is valid for αL values much larger than 1, ensuring a high degree of accuracy.
  • 🔍 The simplified equation is useful for calculating the amplitude of oscillations after passing through the barrier.
  • 📌 The process involves making approximations, which may not be exact but are relevant depending on the size of αL.
  • 🔧 The method is demonstrated step by step, showing how the original complex equation can be transformed into a simpler form.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is simplifying the transmission coefficient equation for a quantum system with a wide barrier.

  • What assumption is made to simplify the transmission coefficient equation?

    -The assumption made is that alpha times L is much larger than one, indicating a wide barrier.

  • How does the hyperbolic sine function change when alpha times L is large?

    -When alpha times L is large, e to the minus alpha times L approaches zero, and the hyperbolic sine of alpha times L becomes very large, simplifying the equation.

  • What happens to the transmission coefficient when the barrier is very wide?

    -When the barrier is very wide, the transmission coefficient simplifies to an exponential decay function, e to the minus 2 alpha L, which represents the decay of the wave function amplitude.

  • What does the term 'e to the X' represent in the context of the hyperbolic sine function?

    -'e to the X' represents the exponential function with X as the exponent, which is part of the definition of the hyperbolic sine function.

  • Why does the term '1' in the hyperbolic sine function become negligible when alpha times L is large?

    -Because the term '1' is much smaller compared to the large exponential term 'e to the alpha times L', it becomes negligible and can be dropped from the equation.

  • How does the transmission coefficient equation change when the hyperbolic sine term is simplified?

    -The transmission coefficient equation becomes 16 times the ratio of the particle's energy to the barrier's potential, multiplied by '1 minus the same ratio', with an exponential decay factor.

  • What is the significance of the exponential decay function in the simplified equation?

    -The exponential decay function indicates the decrease in wave function amplitude as it encounters the barrier, which helps in determining the probability of a particle's transmission through the barrier.

  • Under what condition is the simplified equation considered accurate?

    -The simplified equation is considered accurate when alpha times L is significantly larger than 1, such as 3, 4, or 5 times larger, ensuring a sufficient decay for the approximation to be valid.

  • How can the simplified equation be used in practice?

    -The simplified equation can be used to find the amplitude of oscillations of a particle on the other side of the barrier with respect to its initial amplitude.

  • What is the role of the potential barrier in the transmission coefficient equation?

    -The potential barrier affects the transmission coefficient by determining the probability of a particle passing through it, which is reflected in the energy and potential ratio in the simplified equation.

Outlines
00:00
📚 Simplifying the Transmission Coefficient Equation

This paragraph delves into the process of simplifying the transmission coefficient equation, which is initially complex. The speaker explains how assuming alpha times L (αL) is significantly larger than one allows for a simplification. The hyperbolic sine of αL, when squared, becomes a large term that dominates the equation. The speaker illustrates that as X (representing αL) becomes large, e^(-X) approaches zero and e^X grows large, leading to the simplification of the hyperbolic sine function. The transmission coefficient is then reformulated to be equal to 1 divided by (1 - e^(-2αL)), highlighting the decay function e^(-2αL) that represents the amplitude decrease of the wave function as it encounters a barrier. The speaker emphasizes that this approximation is valid when αL is much larger than 1, and it can be used to calculate the amplitude of oscillations on the other side of the barrier relative to the initial amplitude.

Mindmap
Keywords
💡Transmission Coefficient
The transmission coefficient is a fundamental concept in quantum mechanics that describes the probability of a particle passing through a potential barrier. In the context of the video, it is initially represented by a complex equation which is then simplified under certain conditions. The simplification process is central to the video's educational message, demonstrating how complex concepts can be made more manageable for practical applications.
💡Hyperbolic Sine
Hyperbolic sine is a mathematical function that is the inverse of the hyperbolic cosine function. In the video, it is used to describe the behavior of a wave function when encountering a potential barrier. The simplification of the hyperbolic sine function is crucial in transforming the complex transmission coefficient equation into a more manageable form.
💡Barrier
In the context of the video, a barrier refers to a region in space where a particle's wave function encounters a potential energy that is higher than its kinetic energy. The interaction of the particle with the barrier is what the transmission coefficient describes. The width and potential of the barrier are key factors in determining the particle's transmission and reflection probabilities.
💡Alpha
Alpha, in the script, represents a proportionality constant related to the properties of the barrier and the particle. It is a factor in the equation for the transmission coefficient and plays a significant role in determining the simplification of the equation when the barrier is wide.
💡Potential Energy
Potential energy is the energy a body possesses due to its position in a force field, such as a gravitational or electric field. In the video, it refers to the energy associated with the barrier that the particle encounters. The potential energy of the barrier is a critical factor in determining the transmission coefficient and the probability of the particle passing through the barrier.
💡Wave Function
The wave function is a mathematical description in quantum mechanics that provides information about the probability amplitude of a particle's location. It is central to understanding quantum states and behaviors. In the video, the decay of the amplitude of the wave function as it encounters the barrier is described by the exponential term e to the minus 2 alpha L.
💡Amplitude
Amplitude in the context of waves refers to the maximum displacement of the wave from its equilibrium position. In the video, it is used to describe the strength or intensity of the particle's wave function as it interacts with the barrier. The simplification of the transmission coefficient equation helps in predicting the amplitude of the wave function on the other side of the barrier.
💡Exponential Decay
Exponential decay is a mathematical model that describes a quantity approaching zero at a rate proportional to its current value. In the video, it is used to describe the decrease in the amplitude of the wave function as it encounters the barrier. The term e to the minus 2 alpha L represents this decay function in the context of the particle's wave function interacting with the barrier.
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. The video's content is rooted in quantum mechanics principles, particularly the behavior of particles encountering potential barriers and the calculation of transmission coefficients.
💡Simplification
Simplification in this context refers to the process of making a complex equation or concept more straightforward and easier to understand or calculate. The video focuses on simplifying the transmission coefficient equation under certain conditions, which is essential for practical applications in quantum mechanics.
💡Equation
An equation in mathematics is a statement that asserts the equality of two expressions. In the video, equations are used to model the physical phenomena of quantum mechanics, specifically the transmission of particles through potential barriers. The simplification of these equations is the main focus of the lecture.
Highlights

The lecture introduces a method to simplify the transmission coefficient equation.

Assumption made is that alpha times L is much larger than one, implying a wide barrier.

Hyperbolic sine of alpha times L becomes a large term squared, leading to simplification.

The equation for hyperbolic sine is given as (e^X + e^-X)/2.

When X is large, e^-X approaches zero and e^X becomes large, simplifying the hyperbolic sine function.

The transmission coefficient is redefined based on the simplified hyperbolic sine term.

The new form of the transmission coefficient is 1 divided by (1 - e^(-alpha*L))

Further simplification leads to the transmission coefficient being 16e/V₀ times (1 - E/V₀P).

The denominator simplifies to e^(2*alpha*L), leading to an exponential decay function.

The decay function e^(-2*alpha*L) represents the decrease in wave function amplitude as it encounters the barrier.

The simplified equation is valid for alpha*L much larger than 1, making it easier to work with.

The simplification allows for finding the amplitude of oscillations on the barrier's other side.

The approximation is accurate when alpha*L is significantly larger than 1, such as 3, 4, or 5.

The process demonstrates a practical approach to handling complex quantum mechanics equations.

The lecture provides a clear explanation of how to simplify the transmission coefficient for wider barriers.

The final simplified equation is useful for quantum mechanics problems involving potential barriers.

Transcripts

Browse More Related Video

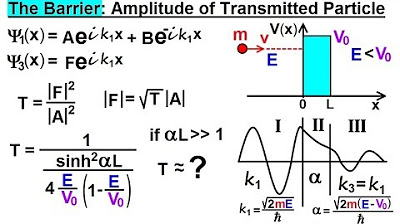
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (78 of 92) The Barrier: Amplitude

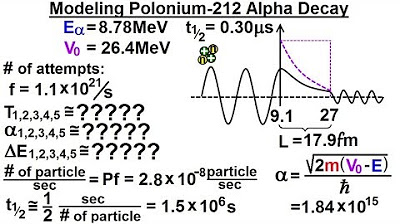
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (92 of 92) Modeling Polonium-212 Decay

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (76 of 92) Penetration Depth V0, E: Ex.

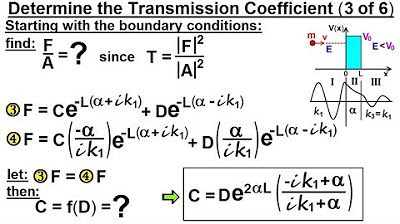
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (85 of 92) Transmission Coeff=? (3 of 6)

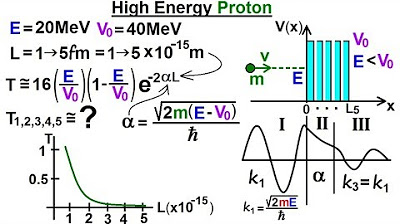
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (90 of 92) A High Energy Proton

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (91 of 92) Modeling Polonium-212 Decay

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsTransmissionCoefficientBarrierWidthHyperbolicSineEquationSimplificationWaveFunctionAmplitudeDecayMathematicalPhysicsLectureSeriesEducationalContent