Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (24 of 92) Prob. of Finding Particle 4

Michel van Biezen
25 Feb 201704:14
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the concept of finding the probability of a particle's location is explored using a one-dimensional model. The video demonstrates how, as the interval Delta X becomes infinitesimally small, the probability at a specific point converges to a precise value. By squaring the wave function and multiplying it by a small Delta X, the probability at any given point is determined. This method simplifies the process of calculating probabilities along a particle's path and is applicable to more complex models, offering a clear and efficient approach to understanding quantum behavior.

Takeaways
  • 📈 The probability of finding a particle between L/4 and L/4 + ΔX was calculated to be 0.01032 with ΔX趋向于0.
  • 🔄 As ΔX becomes smaller, the 3/2 term in the probability expression diminishes, approaching zero in the limit.
  • 🎯 The script introduces an alternative method for finding the probability of a particle's location when ΔX is sufficiently small.
  • 🌟 The alternative method involves using the probability function (wave function squared) multiplied by a very small ΔX to find the exact probability.
  • 📌 For a one-dimensional model, the probability function is derived from the wave function squared, specifically 2/L * sin^2(nπx/L) with n=1 for the lowest energy level.
  • 🔢 The example given uses ΔX = 0.01L and finds the probability at location L/4, resulting in a probability P = 0.01.
  • 🥢 The script demonstrates that the new method yields the same result as integrating the probability function over specific limits.
  • 🌐 This approach can be generalized for more complex models beyond the simple one-dimensional case presented.
  • 📊 The method provides a slick and efficient way to determine the probability of finding a particle at any point along its path.
  • 🚀 As X approaches 0, the 3/2 term becomes negligible, confirming the consistency of the method with previous results.
  • 📋 The script serves as an educational tool for understanding quantum mechanics and the calculation of probabilities in particle physics.
Q & A

  • What was the probability found in the previous video for a particle being between L/4 and L/4 + ΔX?

    -The probability found was 0.01032, with ΔX being a small value.

  • How does the probability change as ΔX approaches zero?

    -As ΔX approaches zero, the probability tends to zero, following the 3/2 term in the expression.

  • What is the alternative method introduced for finding the probability of finding a particle at a particular location?

    -The alternative method involves using the probability function (wave function squared) multiplied by a very small ΔX to find the probability at a specific location.

  • What is the significance of using the wave function squared in this context?

    -The wave function squared represents the probability density, which when multiplied by a small interval ΔX, gives the probability of finding the particle within that interval.

  • What is the role of the constant 2/L in the probability calculation?

    -The constant 2/L normalizes the probability function, ensuring that the total probability over the entire range equals 1 for a normalized wave function.

  • Why is the value of N set to 1 in this calculation?

    -N is set to 1 because the calculation is focused on the innermost energy level, or the lowest energy level, where the quantum number n is equal to 1.

  • What is the value of ΔX used in the example calculation?

    -In the example, ΔX is set to 0.01L, representing a very small interval along the particle's path.

  • What is the location X chosen for the probability calculation in the example?

    -The location X chosen is L/4, which corresponds to the midpoint of the interval between L/4 and L/4 + ΔX.

  • What is the result of the probability calculation at X = L/4?

    -The probability at X = L/4 is 0.005 (or 0.01 times 0.5), which is the chance of finding the particle at that specific location.

  • How does this method simplify the process of finding probabilities along a path?

    -This method simplifies the process by directly using the probability function and a small interval ΔX, eliminating the need to calculate integrals over specific limits.

  • What happens to the 3/2 term in the probability expression as ΔX becomes infinitesimally small?

    -As ΔX becomes infinitesimally small, the 3/2 term disappears, leading to the same result obtained from integrating the probability function over the interval.

Outlines
00:00
📊 Quantum Probability and the Electron Line

This paragraph introduces the concept of finding the probability of a particle's location in a quantum context. It discusses the previous video's findings where the probability of finding a particle between L and L + ΔX was calculated as 0.01032. The speaker explains that as ΔX approaches zero, the probability tends to a fixed value of 0.01. The paragraph then transitions to a new method for determining the probability of finding a particle at a specific location by using the probability function and multiplying it by a very small ΔX. The example given uses a one-dimensional model and a sine squared function to illustrate how the probability is calculated for the lowest energy level. The key takeaway is that this method provides the same result as integrating the probability function over a range, offering a simpler approach to finding probabilities at any point along the particle's path.

Mindmap
Keywords
💡probability
In the context of the video, probability refers to the likelihood of finding a particle within a certain range or at a specific location. It is a fundamental concept in quantum mechanics, representing the chance of a particular outcome. The video discusses calculating this probability by integrating the probability function over a small interval (Delta X) and also by using the wave function squared for a discrete set of points.
💡integral
An integral in mathematics is a concept that represents the area under a curve, which in physics often corresponds to quantities such as work or probability. In the video, the integral is used to calculate the probability of finding a particle over a certain range by summing up infinitesimally small probabilities across that range. The process involves evaluating the integral between two limits to get the total probability.
💡wave function
A wave function in quantum mechanics is a mathematical description of the quantum state of a system. It contains all the information about a physical system and is used to calculate probabilities of finding a particle in a particular state. The video specifically discusses the wave function squared, which is used to determine the probability density of a particle being at a certain location.
💡Delta X
Delta X, often denoted as Δx, represents a small change or interval in the position variable x. In the context of the video, it is used to define the range over which the probability of finding a particle is being calculated. As Delta X becomes smaller, the probability approaches a definite value, illustrating the concept of limits in calculus and its application in physics.
💡quantum mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. The video's discussion of probability, wave functions, and energy levels are all concepts rooted in quantum mechanics, which is essential for understanding the behavior of particles at the quantum level.
💡energy levels
In quantum mechanics, energy levels are the specific, quantized amounts of energy that a particle can have within a system. The video mentions the innermost energy level or the lowest energy level, which is the state with the least amount of energy a particle can have in a given system, such as an electron in a one-dimensional well.
💡sine squared
The sine squared function, often denoted as sin^2(θ), is a trigonometric function that represents the square of the sine of an angle. In the context of the video, it is used in the calculation of the probability density function for a particle in a one-dimensional well, where the angle is determined by the position x and the length L of the well.
💡one-dimensional well
A one-dimensional well, also known as an infinite potential well or particle in a box, is a theoretical model in quantum mechanics where a particle is confined to move within a one-dimensional space with impenetrable walls. This model is used to illustrate and understand the quantization of energy levels and the behavior of wave functions in confined spaces.
💡quantization
Quantization is the process of restricting the possible values of a physical quantity to discrete values or levels. In quantum mechanics, this concept is crucial as it explains how energy, momentum, and other properties can only take on certain discrete values. The video's discussion of energy levels in a one-dimensional well is an example of quantization.
💡limits
In mathematics, limits are a fundamental concept used to describe the behavior of a function as its input approaches a certain value. In the context of the video, limits are used to understand how the probability approaches a specific value as Delta X becomes infinitesimally small, which is essential for calculating probabilities in quantum mechanics.
💡calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. In the video, calculus is applied to integrate the probability function over a range to find the total probability of finding a particle within that range. It is also used to understand the behavior of functions as variables approach certain values, such as the limit as Delta X approaches zero.
Highlights

The probability of finding a particle between L/4 and L is calculated as 0.01032.

As Delta X approaches zero, the probability tends towards a specific value.

An alternative method for finding the probability of a particle's location is introduced when Delta X is sufficiently small.

The probability function is replaced by the wave function squared to find the probability.

For a one-dimensional well, the wave function squared is used to calculate the probability.

The probability is given by (2/L) * sine squared(n PI x/L) * Delta X.

N equals 1 is used for the lowest energy level in the calculations.

The probability at L/4 is calculated to be 0.01.

The method simplifies the process of finding the probability at any point along the path.

The result is consistent with the integral method when Delta X approaches zero.

This approach can be applied to more complex models in the future.

The sine squared function is used to determine the probability at a specific location.

The probability calculation is demonstrated with Delta X set to 0.01 L.

The final probability value is 0.005, illustrating the effectiveness of the method.

This method provides a slick way to find the particle's probability at any point.

The process is explained step by step, making it easy to understand and replicate.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

How Derivatives Arise from Limits – Topic 50 of Machine Learning Foundations

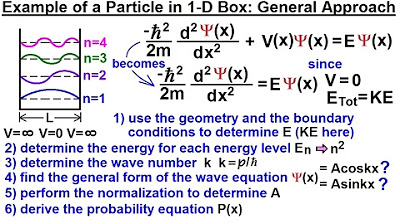
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

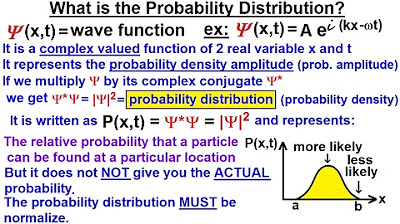
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (27 of 92) Expectation Value=? 1-D Box n=1

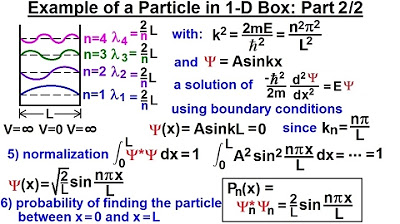
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsProbabilityCalculationWaveFunctionsParticlePhysicsSchrodingerEquationEducationalContentPhysicsTutorialMathematicalAnalysisQuantumBehaviorEducation