Calculus AB/BC – 6.9 Integrating Using Substitution
TLDRIn this comprehensive lesson, Mr. Bean explains the concept of u-substitution in calculus, particularly for integration. He begins by relating it to the chain rule for derivatives, then demonstrates how to identify when to use u-substitution by examining the integrand. Through a series of examples, he illustrates the process of substituting variables, simplifying integrals, and re-substituting to find the final answer. He emphasizes the importance of thinking ahead to ensure the correct choice of u and the cancellation of terms for a successful integration. The lesson also touches on the application of u-substitution in definite integrals, showing how to adjust boundaries accordingly. The examples cover a range of scenarios, including when to avoid u-substitution and the concept of double u-substitution.
Takeaways
- 📚 The concept of substitution, specifically u-substitution, is introduced as a technique for solving integrals of composite functions, analogous to the chain rule for derivatives.
- 🔄 U-substitution is used when the integrand suggests a composite function that would require the chain rule if taking the derivative, indicated by the presence of an inner and outer function.
- 📈 The choice of u in u-substitution is typically the innermost function, and the process involves replacing the inner function with u and solving for du/dx or du.
- 🌟 A key step in u-substitution is to rewrite the integral in terms of u, replacing dx with du, and then integrating with respect to u to find the anti-derivative.
- 🔄 After finding the anti-derivative with respect to u, the original function (in terms of x) is re-substituted to obtain the final result of the integral.
- 📊 Examples provided in the script demonstrate the process of u-substitution, including the cancellation of terms to simplify the integral, and the importance of choosing the correct u for successful simplification.
- 🚫 The script also highlights cases where u-substitution is not applicable, such as when the chosen u does not lead to simplification or cancellation of terms.
- 🔢 The process of u-substitution is shown to be iterative, with the possibility of performing double u-substitution (or more) in complex cases.
- 📌 The script emphasizes the importance of recognizing when not to use u-substitution, such as with inverse trigonometric functions that require a different approach.
- 🔍 The method of changing boundaries is introduced for definite integrals, where the limits of integration are recalculated based on the u-substitution.
- 📋 The script concludes with a reminder to practice and verify answers, reinforcing the practical application of the concepts taught.
Q & A
What is the main topic of the video?
-The main topic of the video is the concept of substitution, specifically u-substitution, in the context of integration in calculus.
How does the video relate u-substitution to the chain rule?
-The video explains that u-substitution is to integrals what the chain rule is to derivatives. Both are used when dealing with composite functions, with the chain rule applying to derivatives and u-substitution to integrals.
What is the general process for u-substitution?
-The general process for u-substitution involves identifying a suitable u (often the inside function), finding its derivative (du/dx), replacing the inside function with u, and then solving for du/dx or dx in terms of du. This allows the integral to be rewritten with u and du, simplifying the process of finding the integral.
How can you determine if u-substitution is necessary for an integral?
-You can determine if u-substitution is necessary by examining the integrand and considering whether taking the derivative would require the chain rule. If the chain rule would be needed for the derivative, then u-substitution will likely be required for the integral.
What is the significance of choosing the right u in u-substitution?
-Choosing the right u is crucial because it should allow for the cancellation of terms when taking the derivative, simplifying the integral process. The chosen u should be a function of the variable that will result in a derivative that can cancel out terms within the integral.
How does the video illustrate the concept of u-substitution with examples?
-The video provides several examples where different functions are integrated using u-substitution. It walks through the process step by step, showing how to choose u, find the derivative, and then simplify the integral by canceling out terms and evaluating the anti-derivative.
What is the role of the derivative in u-substitution?
-The derivative plays a key role in u-substitution as it is used to express dx in terms of du. This allows the integral to be rewritten in terms of u and du, which often simplifies the process of finding the integral.
What is the concept of double u-substitution mentioned in the video?
-Double u-substitution, or double q-substitution, is a technique used when a single u-substitution does not directly lead to a recognizable integral form. It involves performing u-substitution twice to find the integral, which can be more complex but is necessary for certain types of integrals.
How does the video address the issue of not being able to find an integral for a function?
-The video acknowledges that sometimes, after u-substitution, the resulting function may not have a straightforward integral. In such cases, further manipulation or a different approach may be required to solve the integral, as demonstrated with the cotangent function example.
What is the difference between indefinite and definite integrals as discussed in the video?
-Indefinite integrals provide the antiderivative of a function without specific numerical values, often resulting in an expression plus a constant (C). Definite integrals, on the other hand, involve specific numerical limits and provide a numerical value by evaluating the antiderivative at these limits and finding the difference.
How does the video explain changing boundaries in definite integrals with u-substitution?
-The video explains that when performing u-substitution for definite integrals, the boundaries must also be changed in accordance with the substitution. The new boundaries are found by substituting the original limits of the integral into the u expression, which allows the evaluation of the definite integral using the new limits.
Outlines
📚 Introduction to U-Substitution in Integration
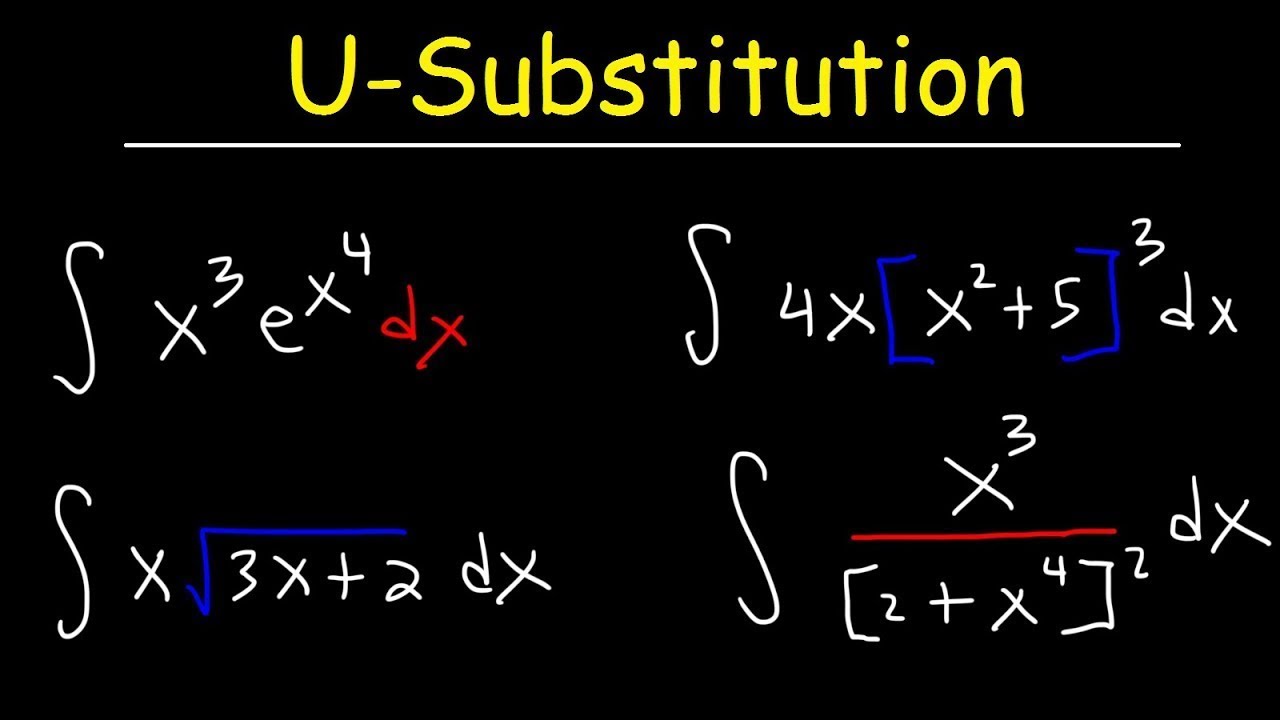
This paragraph introduces the concept of U-substitution in the context of integration, specifically for composite functions. It emphasizes the importance of recognizing when to apply U-substitution by comparing it to the chain rule used in differentiation. The explanation includes a step-by-step guide on how to perform U-substitution, starting with identifying the integrand and deciding on a suitable U variable. The paragraph also provides a clear example of integrating a function involving u to the fifth power, demonstrating the process of substituting, integrating, and then re-substituting to find the final answer.
🧠 Choosing the Right U and Additional Examples
This paragraph delves deeper into the selection process of the U variable for U-substitution, highlighting that it should be the inside function of the integrand. It provides additional examples, including integrals of functions with square roots, exponentials, and trigonometric functions. The explanation covers the thought process behind choosing U, the cancellation mechanism during integration, and the importance of thinking ahead to ensure a smooth calculation process. The paragraph also touches on the concept of double U-substitution and its application in more complex integrals.
🔍 Dealing with Tricky Cases and Inverse Trigonometric Functions
This paragraph addresses more challenging cases in U-substitution, such as when the integrand does not lend itself easily to the standard substitution process. It introduces strategies for dealing with such cases, like solving for x in terms of u when necessary. The paragraph also clarifies the misconception around U-substitution with inverse trigonometric functions, explaining why it's not always applicable and how to recognize when to use other methods instead.
📈 Defining Definite Integrals and Boundary Adjustments
The final paragraph shifts focus to definite integrals, explaining the process of changing boundaries when applying U-substitution. It provides a clear example of integrating a function involving t cubed plus one, showing how to adjust the boundaries from the original limits to new ones based on the U-substitution. The paragraph concludes with a worked-out example of a definite integral involving trigonometric functions, emphasizing the importance of understanding the process and the significance of boundary adjustments in obtaining the correct numerical answer.
Mindmap
Keywords
💡Substitution
💡Integration
💡Chain Rule
💡Integral
💡Derivative
💡Composite Function
💡Anti-derivative
💡u-substitution
💡Definite Integral
💡Inverse Trigonometric Functions
Highlights
Introduction to the concept of substitution in calculus, specifically in relation to integration.
Explanation of the chain rule as it relates to derivatives of composite functions.
The letter 'u' is commonly used for u-substitution, but it can be any variable.
Guidelines on when to use u-substitution: if the integrand requires the chain rule for its derivative, u-substitution is likely needed.
Step-by-step demonstration of u-substitution using the function 3x - 4.
Illustration of how to determine the choice of 'u' by looking at the integrand and anticipating the derivative.
Example of u-substitution with the integrand 6x^2 * x^3 + 4, emphasizing the importance of cancellation.
Explanation of how to check if the choice of 'u' is correct by thinking ahead to the derivative and cancellation.
Demonstration of u-substitution with a more complex example involving square roots and x terms.
Clarification on when u-substitution might not be the best choice, using sine and cosine functions as an example.
Introduction to double u-substitution with an example involving trigonometric functions.
Explanation of how to handle u-substitution with inverse trigonometric functions.
Transition from indefinite integrals to definite integrals and the adjustments needed for boundaries.
Example of solving a definite integral using u-substitution and boundary adjustments.
Final example given for practice, involving the integrand sine x and a discussion on the correct choice of 'u'.
Summary of the lesson's content and encouragement for students to practice and master the material.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: