Calculus 1: U-Substitution Examples
TLDRThis video script introduces the concept of u-substitution, a fundamental technique in calculus for solving integrals. The speaker illustrates how u-substitution can simplify complex integrals by reversing the chain rule process. Through several examples, the script demonstrates the process of choosing an appropriate substitution variable, transforming the integral into a more manageable form, and then applying the power rule or other known integral forms. The examples cover various scenarios, including exponential functions, trigonometric functions, and more, with a focus on identifying the right substitution to simplify the problem. The script concludes with an invitation to visit the speaker's website for additional calculus resources and examples.
Takeaways
- 📚 The video discusses the u-substitution technique, a crucial method in calculus for solving integrals.
- 🔍 U-substitution is used to reverse the chain rule operations and simplify complex integrals into more manageable forms.
- 📐 The process involves setting u equal to a function of x and then finding du/dx to replace terms in the integral.
- 🎯 The goal of u-substitution is to transform the integral into a form that can be solved using known antiderivatives like exponentials, power rules, or trigonometric functions.
- 🔑 An example given is integrating x^2 dx, where u is set to x^3 - 5, and du is found to be 3x^2 dx, simplifying the integral.
- 📉 The video demonstrates that u-substitution can be tricky and may require trying different substitutions to find the correct one.
- 📝 Differentials are used in the process, such as du = 2x dx for u = x^2 + 1, to facilitate the substitution.
- 📈 The technique can handle integrals with complex functions, such as when the integrand involves square roots or exponentials.
- 🧩 After integrating with respect to u, the final step is to substitute x back in to express the answer in terms of the original variable.
- 🔄 The video provides several examples, including cases where the differential du is not directly present in the integral, requiring manipulation before substitution.
- 📚 The presenter offers additional resources on their website for further practice with calculus problems and u-substitution.
Q & A
What is the primary purpose of u-substitution in calculus?
-The primary purpose of u-substitution in calculus is to reverse chain rule operations and simplify the process of finding antiderivatives for complex integrals that cannot be easily solved by direct application of known forms like power rule, exponentials, or trigonometric functions.
How does u-substitution help in evaluating integrals that involve the product or quotient rule?
-U-substitution helps in evaluating such integrals by converting them into a form where the antiderivative can be directly applied without getting entangled in a web of product or quotient rules, thus simplifying the process.
What is the general approach when applying u-substitution to an integral?
-The general approach is to identify a part of the integral that can be replaced by a new variable, 'u', such that the integral can be transformed into a known form or something simpler to integrate. This involves setting u equal to an expression involving x, finding du (the differential of u), and then replacing all instances of x with u in the integral.
Can you provide an example of a u-substitution where the integral is transformed into a form involving exponential functions?
-Yes, in the script, the example where u is set to be the square root of x transforms the integral into a form involving exponential functions, specifically e to the power of u, which is a known form that can be easily integrated.
What is the significance of differentials in the context of u-substitution?
-Differentials are crucial in u-substitution as they allow us to express dx in terms of du, which simplifies the integral by replacing dx with du/du or 1, making the integral easier to solve.
How do you determine the correct form of 'u' to use in a u-substitution problem?
-The correct form of 'u' is typically determined by identifying a part of the integral that can be simplified or transformed into a known form. It often involves looking for expressions that can be exponentiated or integrated directly once the substitution is made.
What is the final step in the u-substitution process?
-The final step in the u-substitution process is to resubstitute x back into the integral after integrating with respect to 'u'. This gives the final answer in terms of the original variable x.
Why is it necessary to include absolute values when integrating functions involving 1/u?
-Absolute values are necessary to account for the fact that u can be positive or negative, which affects the sign of the antiderivative. Including absolute values ensures the solution is valid for all values of u.
Can you provide an example from the script where the choice of 'u' is not straightforward?
-Yes, in the script, the integral involving the square root of (x + 3) is an example where the choice of 'u' is not straightforward. Initially, one might try u = sqrt(x + 3), but the differential would be 1/(2*sqrt(x + 3)) dx, which complicates the integral. Instead, the script suggests u = x + 3, which simplifies the process.
How does the script demonstrate the process of u-substitution with a specific integral?
-The script demonstrates the process by first setting u equal to an expression, finding the differential du, and then replacing all instances of x with u. It then integrates with respect to u, applies the power rule, and finally resubstitutes x to get the answer in terms of the original variable.
Outlines
📚 Introduction to U-Substitution in Calculus
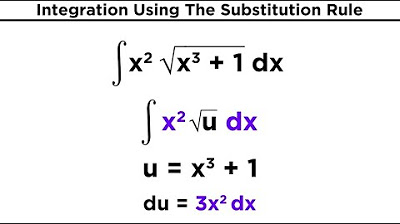
The first paragraph introduces the concept of U-substitution, a fundamental technique in calculus for solving integrals that don't fit into standard forms. The speaker explains that instead of applying the product or quotient rule directly, U-substitution is used to reverse the chain rule operations. An example is provided where U is set to X cubed minus 5, and the integral is transformed into a more manageable form by using differentials (Du = 3x^2 dx). The integral is then solved using the power rule after replacing all instances of X with U, and the final answer is obtained by substituting X back into the solution. The importance of recognizing when to use U-substitution and the process of identifying the correct substitution are also discussed.
🔍 Advanced U-Substitution Techniques and Examples
The second paragraph delves into more complex scenarios where U-substitution is applied. The speaker discusses the importance of choosing the right U to simplify the integral and provides several examples to illustrate the process. The first example involves substituting U with x squared plus 1, and adjusting the integral by dividing by two to fit the differential (Du = 2x dx). The second example shows a tricky case with a square root, where U is chosen as x plus 3 to simplify the expression, and algebraic manipulation is used to split the integral into a form that can be solved using power rules. The third example features a substitution where U is the square root of x, and the integral is transformed to involve the exponential function, which is then solved using the natural logarithm. The speaker emphasizes the need for precision, such as including absolute value signs when necessary, and concludes by inviting viewers to their website for more calculus examples and solutions.
Mindmap
Keywords
💡u-substitution
💡antiderivative
💡product rule
💡quotient rule
💡chain rule
💡differential
💡power rule
💡trigonometry
💡inverse trig functions
💡resubstitution
Highlights
Introduction to u-substitution as an integral technique in calculus.
Explanation of the limitations of direct antiderivatives and the product rule.
The concept of reversing chain rule operations using u-substitution.
Conversion of integrals into known forms like exponentials, power rule, trigonometry, and inverse trigonometric functions.
Use of differentials in u-substitution with the example of U = x^3 - 5.
Process of replacing x terms with u terms in integrals.
Application of power rule after substitution to simplify integration.
The importance of resubstituting x to get the final answer in terms of x.
Demonstration of u-substitution with the integral of (x^2 + 1)^8 * x dx.
Compensation for missing terms by multiplying through by constants.
Dealing with integrals involving the reciprocal of the differential.
Integration of 1/u using natural logarithm as the antiderivative.
The necessity of absolute value in the antiderivative of 1/u.
Approach to square root integrals with u = x + 3 and handling of x terms.
Algebraic manipulation to simplify the integral of (u - 3) / (sqrt(u)) du.
Integration involving exponential functions with u = sqrt(x).
Final substitution back to x to complete the integral.
Invitation to the instructor's website for more calculus examples and resources.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: