AP Calculus BC Lesson 10.11 Part 2
TLDRThis video tutorial delves into the application of Taylor and Maclaurin polynomials, particularly their relevance in the AP Calculus BC exam. It emphasizes the importance of understanding the general structure and formulas of these polynomials, and demonstrates how to approximate functions using polynomials of varying degrees. The video uses examples and visual aids to clarify the process of constructing and evaluating Taylor and Maclaurin polynomials, making it an informative resource for students preparing for calculus exams.
Takeaways
- 📚 Taylor and Maclaurin polynomials are frequently tested topics in the AP Calculus BC exam.
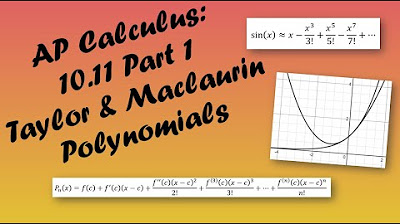
- 🔢 The nth degree Taylor polynomial formula and the nth degree Maclaurin polynomial formula are essential to understand and apply.
- 🎯 Maclaurin polynomials are a special case of Taylor polynomials centered at x=0, simplifying calculations by substituting c=0.
- 📈 Memorizing the Maclaurin polynomials for sine(x), cosine(x), and e^x simplifies problem-solving, especially in multiple-choice exams.
- 🧩 To construct a Taylor polynomial, you need to know the function's value and its derivatives at the center point (c).
- 🔍 The general formula for a Taylor polynomial can be used to approximate function values by plugging in the appropriate values for x.
- 🌐 The script provides examples of constructing Taylor polynomials for functions like e^(3x) and x*sin(x), demonstrating the process step by step.
- 📊 The process of finding the Taylor polynomial involves evaluating derivatives at the center point and simplifying the expression.
- 🛠️ The derivative of a Taylor polynomial at a certain point can be found by taking the derivative of the original function at that point.
- 📝 The script also covers how to approximate values of trigonometric functions using Taylor polynomials, which is a useful technique in calculus.
- 📌 The visual representation of a function's graph can help deduce the second degree Maclaurin polynomial by analyzing the function's behavior and concavity.
Q & A
What is the main topic of the video?
-The main topic of the video is Taylor and Maclaurin polynomials, specifically their application in multiple-choice questions for the AP Calculus BC exam.
What are Taylor polynomials?
-Taylor polynomials are polynomials that approximate a given function by taking its derivatives at a certain point and building a polynomial around that point.
What is the difference between a Taylor polynomial and a Maclaurin polynomial?
-A Maclaurin polynomial is a special case of a Taylor polynomial where the point of expansion (center) is set at x equals zero.
What is the general formula for the nth degree Taylor polynomial?
-The general formula for the nth degree Taylor polynomial is: F(c) + F'(c)(x - c) + F''(c)(x - c)^2/2! + F'''(c)(x - c)^3/3! + ... + F^n(c)(x - c)^n/n!
How do you find the Maclaurin polynomial for a function?
-To find the Maclaurin polynomial for a function, you evaluate the function and its derivatives at x = 0 and then plug these values into the Taylor polynomial formula centered at x = 0.
What are the Maclaurin polynomials for sine(x), cosine(x), and e^x?
-The Maclaurin polynomials for sine(x), cosine(x), and e^x can be found by taking their derivatives at x = 0 and plugging these into the Maclaurin polynomial formula. For sine(x), it's x - x^3/3! + x^5/5! - ...; for cosine(x), it's 1 - x^2/2! + x^4/4! - ...; and for e^x, it's 1 + x + x^2/2! + x^3/3! + ... (which is the same as the Taylor series for e^x).
How do you approximate a function using a third degree Taylor polynomial?
-To approximate a function using a third degree Taylor polynomial, you find the function's value and its first three derivatives at the center point, plug these into the third degree Taylor polynomial formula, and then evaluate the polynomial at the point of interest.
What is the process for finding the nth term in a Taylor polynomial?
-The nth term in a Taylor polynomial is found by taking the nth derivative of the function at the center point, multiplying it by the (n-1)th power of (x - center) divided by n!, and then adding it to the polynomial formed by the previous terms.
How does the video demonstrate the use of Taylor polynomials in solving multiple-choice questions?
-The video demonstrates the use of Taylor polynomials in solving multiple-choice questions by walking through the process of setting up the polynomial, plugging in given values, and evaluating the polynomial at specific points to find approximations for functions.
What is the significance of memorizing the Maclaurin polynomials for basic functions like sine(x), cosine(x), and e^x?
-Memorizing the Maclaurin polynomials for basic functions like sine(x), cosine(x), and e^x is significant because it allows for quick approximations without having to calculate the derivatives at x=0 each time, thus saving time and simplifying the problem-solving process.
How does the video use the concept of Taylor and Maclaurin polynomials to approximate values of functions?
-The video uses the concept of Taylor and Maclaurin polynomials to approximate values of functions by constructing the polynomials based on the function's derivatives at a certain point (center), and then evaluating the polynomial at the point of interest to find an approximation of the function's value.
Outlines
📚 Introduction to Taylor and Maclaurin Polynomials
This paragraph introduces the topic of Taylor and Maclaurin polynomials, emphasizing their frequent appearance in the multiple-choice section of the AP Calculus BC exam. It mentions the existence of a separate video explaining the general structure and function of these polynomials. The paragraph outlines the key formulas needed for understanding Taylor and Maclaurin polynomials, including the nth degree Taylor and Maclaurin polynomial formulas and the structure of their terms. It also highlights the importance of memorizing the Maclaurin polynomials for sine, cosine, and e to the power of x for quick problem-solving.
📈 Solving Problems with Taylor Polynomials
The paragraph demonstrates how to apply Taylor polynomials to solve problems, using the third-degree Taylor polynomial as an example. It explains the process of using given values of a function and its derivatives to construct the polynomial and approximate the function's value at a specific point. The paragraph provides a step-by-step solution to a multiple-choice question involving the approximation of a function using the third-degree Taylor polynomial centered at x equals 4. It also discusses the construction of a third-degree Taylor polynomial for a function centered at x equals 2 and how to simplify the polynomial using given derivative values.
🌐 Special Cases of Taylor Polynomials
This paragraph explores special cases of Taylor polynomials, such as the fourth-degree Taylor polynomial for the cosine function centered at x equals Pi and the sixth-degree Taylor polynomial for the product of x and the sine function centered at x equals zero. It explains the process of finding the derivatives of the given functions at the center point and plugging them into the Taylor polynomial formula. The paragraph also discusses the use of Maclaurin polynomials as a shortcut for certain functions and provides the correct answer choices for the given examples.
🔄 Derivatives and Tangent Lines of Taylor Polynomials
The paragraph focuses on the concept of derivatives and tangent lines in the context of Taylor polynomials. It explains how the derivative of a Taylor polynomial at a certain point can be used to find the slope of the tangent line to the graph of the polynomial. The paragraph provides examples of finding the third-degree Taylor polynomial for the exponential function e to the power of 3x centered at x equals 6 and how to determine the slope of the tangent line at that point. It also discusses the construction of the third-degree Taylor polynomial for the function x minus 1 to the fourth, centered at x equals zero, and its relation to the Maclaurin polynomial.
📊 Approximating Functions with Maclaurin Polynomials
This paragraph delves into the use of Maclaurin polynomials for approximating the values of functions, specifically focusing on the sine function. It explains how to construct the fifth-degree Maclaurin polynomial for sine and use it to approximate the value of sine of one-third. The paragraph also presents a multiple-choice question involving the third-degree Taylor polynomial for a function centered at x equals eight, providing the correct answer choice based on the given derivative values. Additionally, it describes a method for visually identifying the second-degree Maclaurin polynomial of a function based on its graph and the behavior of the function at x equals zero.
Mindmap
Keywords
💡Taylor Polynomials
💡Maclaurin Polynomials
💡AP Calculus BC Exam
💡Derivatives
💡Center of Polynomial
💡Multiple Choice Questions
💡Factorials
💡Trigonometric Functions
💡Exponential Functions
💡Chain Rule
Highlights
The video covers multiple choice questions related to Taylor and Maclaurin polynomials, a frequent topic in the AP Calculus BC exam.
Taylor polynomials are essential for understanding the structure and application in approximating functions.
Maclaurin polynomials are a specific case of Taylor polynomials centered at x=0.
The general formula for the nth degree Taylor polynomial and Maclaurin polynomial is introduced, which is crucial for solving problems.
The video provides a method for calculating the Taylor polynomial for a function using given derivatives at a specific point.
An example is worked out to approximate a function using a third degree Taylor polynomial, demonstrating the application of the formula.
The importance of memorizing Maclaurin polynomials for sine, cosine, and exponential functions is emphasized for efficiency in problem-solving.
A step-by-step approach to finding the Taylor polynomial approximation for a function is shown, including plugging in values and simplifying the expression.
The concept of the derivative of a Taylor polynomial is discussed, which is equivalent to the derivative of the original function.
The video illustrates how to find the slope of the tangent line to the graph of a Taylor polynomial, which is the first derivative of the polynomial.
A shortcut for finding the Taylor polynomial for the sine function is presented, simplifying the process of taking multiple derivatives.
The video demonstrates how to approximate the value of a function using a Taylor polynomial, showing the practical application in solving calculus problems.
The process of finding the Taylor polynomial for a function like e to the power of 3x is explained, including evaluating derivatives at a specific point.
The general formula for a third degree Taylor polynomial is used to find the polynomial for a function x minus 1 to the fourth, centered at x=0.
An example of approximating the value of sine for a specific angle using a Taylor polynomial is provided, showcasing the use of the polynomial in trigonometric functions.
The video concludes with a problem involving finding the third degree Taylor polynomial for a function given certain derivatives at x=8, illustrating the application of the formula in various scenarios.
A method for determining the second degree Maclaurin polynomial for a function based on its graph and given derivatives is discussed.
The video demonstrates how to use the graph of a function to infer the coefficients of its Maclaurin polynomial, applying visual analysis to mathematical problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: