Let’s Learn a “Little” Calculus - step-by-step…
TLDRIn this engaging video, John, the founder of Tablet Class Math, demystifies calculus by introducing the concept of integration through a simple problem: finding the area under a curve defined by a given function. He emphasizes that despite calculus's reputation for complexity, understanding its fundamentals is accessible with basic problems. John also shares his experience as a math teacher and the importance of good note-taking for academic success. He offers a range of online math courses and resources, including comprehensive math notes, to support learners at various levels, from middle school to college and beyond.
Takeaways
- 📚 The video aims to demystify calculus and encourage viewers to understand its essence through basic problems.
- 🌟 Calculus is an advanced branch of mathematics that can seem intimidating, but its core concepts can be grasped with practice.
- 📈 The main problem discussed is finding the area of a shape defined by a function, which is a fundamental application of integration.
- 🤔 The video emphasizes that there's no simple formula for calculating the area of complex shapes, which is where calculus comes in.
- 👨🏫 The speaker, John, introduces himself as a math teacher and founder of Tablet Class Math, offering online math help programs.
- 📊 The video demonstrates the process of evaluating an integral to find the area under a curve, using the function y = 2x^2 - 3x + 2.
- 📚 The importance of taking good math notes and having a strong foundation in prerequisite subjects is highlighted for success in calculus.
- 🎓 The prerequisites for taking calculus include courses in algebra, geometry, and pre-calculus, which prepare students for more advanced mathematics.
- 🚀 John also discusses his work in area test preparation and support for independent learners, such as homeschoolers.
- 💪 The video encourages viewers not to be discouraged by math and to take personal responsibility for their learning journey.
- 👍 The speaker invites viewers to like the video and subscribe to his YouTube channel for more math education content.
Q & A
What is the main topic of the video?
-The main topic of the video is to provide an introduction to calculus, specifically focusing on the concept of integration and its application in finding the area under a curve.
How does the speaker describe the general perception of calculus?
-The speaker describes the general perception of calculus as something that is often intimidating and considered difficult by most people. It is sometimes viewed as a form of 'math torture' due to its complexity.
What is the problem the speaker aims to solve in the video?
-The speaker aims to solve the problem of finding the area of a yellow shape defined by a specific function, which cannot be calculated using traditional geometric formulas.
What is the function that defines the curve in the problem?
-The function that defines the curve is y = 2x^2 - 3x + 2.
What is integration in calculus?
-Integration in calculus is a key concept used to find the area under a curve, which is demonstrated in the video by calculating the area of the yellow shape between the x-axis and the curve defined by the given function.
What are the prerequisites for taking calculus according to the speaker?
-The prerequisites for taking calculus, as mentioned by the speaker, include having a solid foundation in pre-algebra, algebra, geometry, and algebra 2. Additionally, a pre-calculus course that covers advanced algebra and trigonometry is typically required.
What advice does the speaker give to those struggling with calculus?
-The speaker advises that struggling with calculus could be due to a weak foundation, not working hard enough, or being placed in the wrong course level. He encourages viewers to strengthen their foundation, work diligently, and seek additional help if necessary.
How does the speaker demonstrate the integration process?
-The speaker demonstrates the integration process by first introducing the function that defines the curve, then setting up the integral with the 'elongated S' notation, and finally evaluating the integral by applying the power rule and substituting the bounds of the interval (0 to 2) into the antiderivative of the function.
What is the result of the area calculation?
-The result of the area calculation is 10/3 or approximately 3.33 square units.
What is the speaker's role and what does he offer to help viewers?
-The speaker is John, the founder of Tablet Class Math and a middle and high school math teacher. He offers over a hundred different math courses, ranging from pre-algebra to advanced mathematics, as well as test preparation and homeschooling programs.
What is the speaker's final message to viewers?
-The speaker's final message is to encourage viewers not to be intimidated by math, especially calculus, and to take personal responsibility for their learning. He invites viewers to explore their options for taking calculus and to use his resources if they find themselves interested in learning more.
Outlines
📚 Introduction to Calculus
The speaker introduces the topic of calculus, acknowledging that many people find it intimidating. They aim to demystify calculus by explaining that despite its complexity, the essence of the subject can be understood through basic problems. The speaker sets up a problem involving a yellow shape defined by a function and aims to find its area using calculus, specifically integration. The speaker also introduces himself as John, the founder of Tablet Class Math and a math teacher with years of experience, and mentions his online math help program.
📈 Prerequisites for Calculus
The speaker discusses the typical math courses one needs to take before being ready for calculus, including algebra, geometry, and pre-calculus. He emphasizes that calculus is often taken in college, but advanced high school students may take AP calculus. The speaker also mentions his own pre-calculus course that will be launching soon and reassures viewers that understanding calculus is possible with a solid foundation in prerequisite subjects.
🧮 Solving a Calculus Problem
The speaker walks through the process of solving a calculus problem involving finding the area under a curve defined by the function y = 2x^2 - 3x + 2 between x=0 and x=2. He explains the concept of integration and the use of the integral symbol, demonstrating how to set up and evaluate the integral. The speaker simplifies the integral and evaluates it at the bounds of the interval, ultimately finding the area of the shape to be 10/3 or 3 and 1/3 square units.
🌟 Encouragement and Advice for Learning Calculus
The speaker encourages viewers not to be discouraged by calculus, emphasizing that with effort and a strong foundation, anyone can learn it. He advises viewers to strengthen their math foundation if they struggle with calculus and to work hard, seeking additional help if necessary. The speaker also suggests that viewers might be placed in the wrong course level and advises discussing this with their teachers. He concludes by promoting his YouTube channel and math help program, encouraging viewers to take responsibility for their learning and to seek out resources to improve their math skills.
Mindmap
Keywords
💡calculus
💡integration
💡function
💡area
💡intimidating
💡prerequisites
💡notes
💡foundation
💡online math help
💡homeschooling
Highlights
Introduction to calculus and its intimidating reputation.
The essence of calculus can be understood with basic problems.
Demonstration of calculating the area of a shape using integration.
Explanation of the function y = 2x^2 - 3x + 2 defining the curve.
Integration as a key concept in calculus for finding areas.
The process of evaluating an integral by applying calculus rules.
Breaking down the integration process into simpler steps.
The importance of taking good math notes for academic success.
The prerequisites for taking calculus, including algebra and geometry courses.
The structure of a basic first-year calculus course, divided into derivatives and integration.
Advice for students struggling with calculus, emphasizing the need for a strong foundation and hard work.
The founder's introduction and his online math help program, Tablet Class Math.
The significance of area test preparation and how the speaker's program can assist.
The availability of various math courses, from pre-algebra to advanced mathematics.
The upcoming launch of a pre-calculus course within the speaker's program.
The practical application of calculus in solving real-world problems.
The encouragement for viewers to explore their options for taking calculus.
Transcripts
Browse More Related Video

Algebra AGE WORD PROBLEM – Let’s solve it step-by-step...

Algebra SYSTEM WORD PROBLEM – Let’s solve it step-by-step...
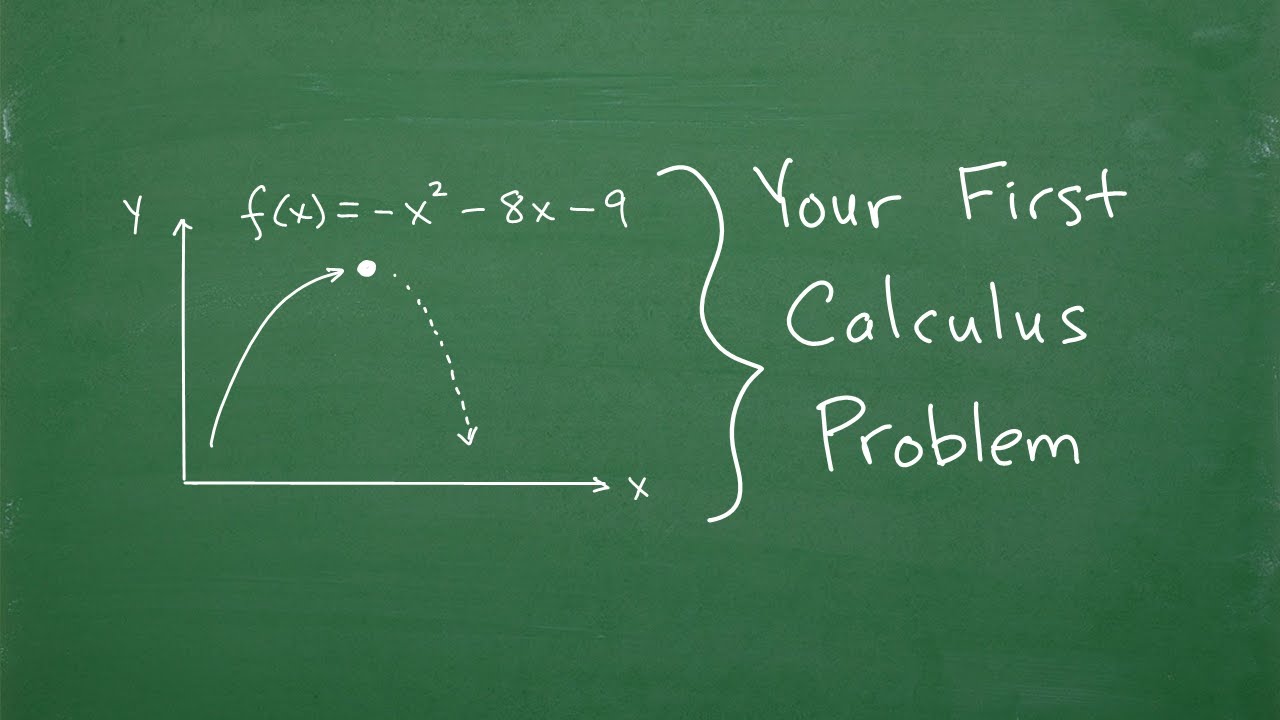
Your First Basic CALCULUS Problem Let’s Do It Together….
Get The Help You Need To Succeed In Math!
100% Certainty you will see this in ALGEBRA
Want to PASS College Algebra? Absolutely, better understand this…
5.0 / 5 (0 votes)
Thanks for rating: