AP Physics 1 Simple Harmonic Motion, Mechanical Waves, and Sound Review
TLDRThis video script delves into the concepts of simple harmonic motion and sound, particularly within the context of AP Physics 1. It explains the principles of oscillation, restoring forces, and the period of a mass-spring system and a simple pendulum. The script further explores the work done by forces on a pendulum and the conservation of energy, highlighting the effects of damping due to resistive forces. It also discusses the characteristics of mechanical waves, including longitudinal and transverse waves, and the equations governing wave behavior. The concept of standing waves, the properties of sound, and the Doppler effect are also covered, providing a comprehensive understanding of wave mechanics and sound phenomena.
Takeaways
- 📈 Simple harmonic motion occurs when an object oscillates back and forth due to a restoring force proportional to its displacement from equilibrium.
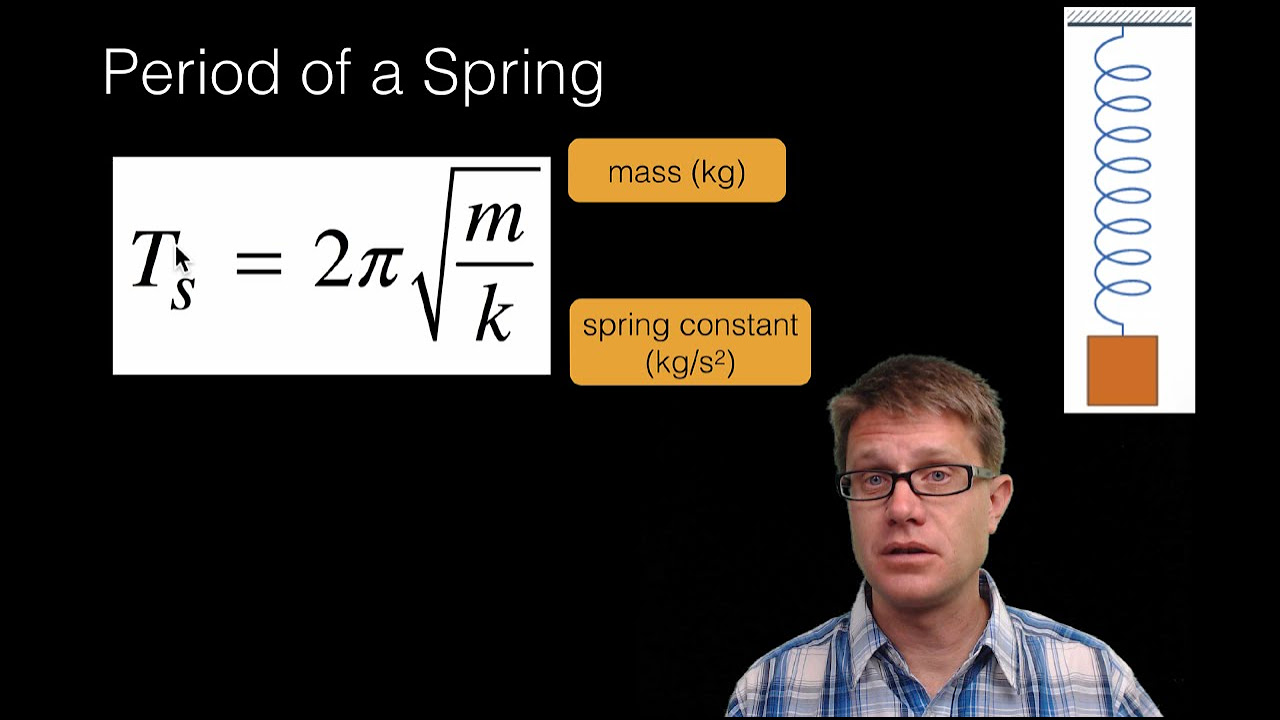
- 🌀 The period of a mass-spring system is given by T = 2π√(M/K), where M is the mass and K is the spring constant.
- 🔄 The period of a simple pendulum depends on the length of the string and the acceleration due to gravity, independent of the mass of the pendulum bob.
- 💤 Tension forces in a pendulum and spring systems do no work, conserving the system's energy in the absence of resistive forces like friction or air resistance.
- 📊 The velocity of a mass-spring system or pendulum is maximum at the equilibrium position and zero at the amplitudes.
- 📈 The acceleration of a mass-spring system or pendulum is zero at the equilibrium position and maximum (in magnitude) at the amplitudes.
- 🌊 Waves are disturbances that carry energy from one place to another, with longitudinal waves having particles oscillating parallel to the wave travel and transverse waves perpendicular.
- 🔄 The speed of waves is determined by the medium's properties, the frequency by the oscillator, and the wavelength is the ratio of these two.
- 🌐 Standing waves are formed by the superposition of waves traveling in opposite directions, resulting in nodes and antinodes.
- 🚗 The Doppler effect describes the change in observed frequency due to the relative motion between a source of sound and an observer.
- 🎵 Sound waves travel at different speeds through different media, with the speed of sound in air being approximately 343 meters per second.
Q & A
What is simple harmonic motion?
-Simple harmonic motion occurs when an object oscillates back and forth about an equilibrium position due to a restoring force that is proportional to the object's displacement from equilibrium.
What are the conditions for an object to exhibit simple harmonic motion?
-For an object to exhibit simple harmonic motion, there must be a well-defined equilibrium position and a restoring force that always points toward this equilibrium position.
How does the period of a mass-spring system depend on its mass and spring constant?
-The period of a mass-spring system is given by the formula T = 2π * √(M/K), where M is the mass and K is the spring constant. The period changes if either the mass or the spring constant is altered.
What is the restoring force for a mass-spring system?
-The restoring force for a mass-spring system is the spring force, which obeys Hooke's law (F = -kx), where F is the force exerted by the spring, k is the spring constant, and x is the displacement from the equilibrium position.
How does the period of a simple pendulum depend on its length and the acceleration due to gravity?
-The period of a simple pendulum depends on the length of the string (L) and the acceleration due to gravity (g), and it is given by the formula T = 2π * √(L/g).
Why does the work done by the tension force in a pendulum system equal zero?
-The work done by the tension force in a pendulum system equals zero because the tension force is always perpendicular to the direction of motion of the pendulum. Since work is calculated as F * d * cos(θ), and cos(90°) = 0, no work is done by the tension force.
What is the significance of nodes and antinodes in standing waves?
-Nodes are points in a standing wave where there is zero displacement, and antinodes are points of maximum displacement. These features are important for understanding the pattern and behavior of standing waves.
How does the speed of sound vary with different media?
-The speed of sound depends on the physical properties of the medium, such as temperature, density, and elasticity. It travels faster in media with greater density and elasticity, like metals, compared to air.
What is the Doppler effect and how does it affect the observed frequency?
-The Doppler effect is the change in the observed frequency of a wave due to the relative motion between the source of the wave and the observer. If the source and observer are moving closer together, the observed frequency increases; if they are moving apart, the observed frequency decreases.
How can the speed of sound be used to determine distance?
-Since sound travels at a constant speed in a given medium, the time it takes for a sound wave to travel to an object and back can be used to calculate the distance between the source and the object using the formula distance = speed of sound * time.
What are the differences between open-open, open-closed, and closed-closed systems in terms of standing waves?
-Open-open systems, like open pipes or strings, require antinodes at both ends and can produce waves with any harmonic number (n). Open-closed systems, such as a pipe with one end closed, require a node at the closed end and can produce odd harmonics only (n = 1, 3, 5, ...). Closed-closed systems, like a string with both ends fixed, also require nodes at both ends and produce standing waves similar to open-open systems.
Outlines
📚 Introduction to Simple Harmonic Motion and Waves
The video begins with an introduction to simple harmonic motion and mechanical waves, focusing on the concept of an object oscillating due to a restoring force. It explains that for simple harmonic motion to occur, the restoring force must be proportional to the object's displacement from equilibrium. The video introduces the mass-spring system as an example, detailing how the spring force, governed by Hooke's Law, creates the restoring force. It also discusses the period of oscillation for both mass-spring systems and pendulums, highlighting how they depend on different variables but are independent of amplitude.
💡 Work Done by Forces in Oscillatory Systems
This paragraph delves into the work done by different forces in a pendulum system. It explains that the work done by the tension force is zero because it is always perpendicular to the direction of motion. The gravitational force, being a conservative force, does no net work over a complete cycle, leading to the conservation of energy in an ideal pendulum system. However, in practical scenarios, resistive forces like friction and air resistance cause damping, leading to a gradual decrease in amplitude over time. The paragraph also compares these concepts to the mass-spring system, noting similarities in energy conservation and damping effects.
📈 Analysis of Position, Velocity, and Acceleration
The video script provides an in-depth analysis of the position, velocity, and acceleration of an object in simple harmonic motion at different points in its cycle. It explains that at the equilibrium position, the object's velocity is at its maximum and acceleration is zero due to the net force being zero. At the amplitude positions, the object momentarily stops (zero velocity) and experiences maximum acceleration in opposite directions. The paragraph also introduces a table to summarize these dynamics and discusses the implications for the net force and energy of the system.
🌊 Understanding Waves: Types and Characteristics
This section introduces the broader concept of waves as disturbances that transmit energy. It differentiates between longitudinal and transverse waves, explaining how particle motion differs in each type. Longitudinal waves, like sound waves, see particles oscillating parallel to the wave direction, while transverse waves, such as those on a string, have particles moving perpendicularly. The video also presents the wave equation, V = fλ, highlighting the relationship between wave velocity, frequency, and wavelength. It emphasizes that the speed of a wave is determined by the medium's properties, while frequency is determined by the source.
🌀 Superposition and Wave Interference
The paragraph discusses the principles of superposition and wave interference, explaining how waves can constructively or destructively interfere when they meet. Constructive interference results in larger amplitudes when waves align in the same direction, while destructive interference leads to cancellation when waves oppose each other. The concept of beat frequencies is introduced, where two close but unequal frequencies create a pulsing effect due to alternating constructive and destructive interference. The paragraph also touches on standing waves, which result from the superposition of waves traveling in opposite directions and have fixed nodes and antinodes.
🎵 Standing Waves in Strings and Pipes
This section explores standing waves, particularly in strings and pipes. It explains how standing waves are formed by the superposition of waves traveling in opposite directions, leading to nodes and antinodes. The video outlines the conditions for standing waves in open-open, open-closed, and closed-closed systems. For open-open pipes, antinodes are required at both ends, while open-closed pipes need a node at the closed end. The video provides equations for calculating the wavelength of standing waves in these systems and discusses the harmonics that can be supported, including the odd harmonics in open-closed pipes and the necessity of both nodes and antinodes in closed-closed pipes.
🚗 The Doppler Effect and Sound
The final paragraph discusses the Doppler effect, which is the change in observed frequency due to the relative motion between a source of sound and an observer. It explains how an approaching object will observe a higher frequency as the sound waves bunch up, while a receding object will perceive a lower frequency as the sound waves spread out. The paragraph clarifies that the Doppler effect is about the change in frequency, not the change in loudness. It also briefly touches on the practical applications of the speed of sound, such as in motion detectors, and how it can be used to measure distances based on the time it takes for sound waves to travel and return.
Mindmap
Keywords
💡Simple Harmonic Motion
💡Restoring Force
💡Period
💡Damping
💡Work
💡Conservative Force
💡Wave
💡Wavelength
💡Standing Wave
💡Doppler Effect
💡Beat Frequency
Highlights
Simple harmonic motion occurs when an object oscillates back and forth due to a restoring force proportional to its displacement from equilibrium.
The period of a mass-spring system is given by TS = 2π * sqrt(M/K), where M is the mass and K is the spring constant.
In a simple pendulum, the period depends on the length of the string and the acceleration due to gravity.
The work done by the tension force in a pendulum is zero because it is always perpendicular to the direction of motion.
The gravitational force on a pendulum is conservative, meaning the net work done over a complete oscillation is zero.
Damping in oscillations occurs due to resistive forces like friction and air resistance, causing the amplitude to decrease over time.
At equilibrium, the velocity of a mass-spring system or pendulum is at its maximum, and the acceleration is zero.
The acceleration at the maximum amplitude of a mass-spring system is at its maximum (negative or positive) due to the maximum spring force.
Waves are disturbances that carry energy from one place to another, with longitudinal and transverse waves being the two main types.
Longitudinal waves, like sound waves, have particles oscillating parallel to the direction of wave travel.
Transverse waves have particles oscillating perpendicular to the direction of wave travel, with peaks (crests) and troughs as the high and low points.
The wave equation V = fλ relates the wave velocity (V), frequency (f), and wavelength (λ).
The period and frequency of a wave are inversely related; as frequency increases, the period decreases.
Constructive interference occurs when wave amplitudes combine in the same direction, resulting in a larger amplitude.
Destructive interference happens when wave amplitudes oppose each other, leading to a smaller or zero amplitude.
Standing waves are formed by the superposition of two waves traveling in opposite directions, creating nodes and antinodes.
The wavelength of a standing wave can be determined by the number of antinodes and the length of the medium (e.g., string or pipe).
In open-closed pipes, only odd harmonics are possible, with the closed end always being a node.
The speed of sound in a medium is constant and depends on the medium's physical properties, such as temperature and density.
The Doppler effect describes the change in observed frequency due to the relative motion between the source and observer of the sound.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: