5 counterexamples every calculus student should know
TLDRThis video script explores the concept of counterexamples in calculus, which serve to disprove seemingly reasonable theorems based on intuition. The video begins by challenging the notion that discontinuities of a function are always isolated, using the example of the function x^2 - 1 / (x - 1), which has an isolated discontinuity at x = 1. However, it introduces the Dirichlet function, which is discontinuous everywhere, as a counterexample. The script then refutes the claim that differentiable functions have continuous derivatives, using the function x^2 * sin(1/x) as an example. It also discusses the local to global property of derivatives and increasing functions, and provides a counterexample showing that a function can be differentiable at a point with a positive derivative without being increasing in any neighborhood of that point. The video further illustrates that the derivative of a function may not share the asymptotic behavior of the original function, as demonstrated by the function sin(x^2)/x. Lastly, it shows that a sequence of continuous functions can converge to a discontinuous limit function, using the sequence x^n as an example. These examples highlight the importance of critical thinking in calculus and the need to test conjectures with specific cases.
Takeaways
- 📉 The first counterexample challenges the intuition that discontinuities of a function are always isolated. The script introduces a function that is discontinuous everywhere, disproving this notion.
- 🤔 The example of the Dirichlet function demonstrates that a function can be defined in a way that it takes the value of one for rational numbers and zero for irrational numbers, showing a function that is discontinuous across the entire real line.
- 📈 The script refutes the claim that if a function is differentiable, then its derivative must be continuous. An example is given where the derivative exists but is not continuous.
- 🔍 The concept of an increasing function on an interval is discussed, and it is shown that a function can have a positive derivative at a point without being increasing on any interval around that point.
- 🧵 The behavior of a function and its derivative as x approaches infinity is explored. It is shown that even if a function approaches zero as x goes to infinity, its derivative may not.
- 🌊 The script provides an example where the original function has a nice asymptotic behavior, but its derivative does not. This highlights that derivatives can be more complex than the original functions.
- 🔁 A sequence of functions that are all continuous (x to the power of n) is shown to converge to a discontinuous function, contradicting the idea that a sequence of continuous functions must converge to a continuous function.
- 📌 The importance of local versus global properties in calculus is emphasized. For example, a positive derivative at a single point does not guarantee that the function is increasing in any neighborhood around that point.
- 📙 The use of the squeeze theorem is demonstrated to evaluate limits, particularly in the context of the derivative of a function at a specific point.
- 📈 The graphical representation of functions and their derivatives is used to illustrate complex mathematical concepts, such as the behavior of functions and their derivatives.
- 🔢 Rational and irrational numbers are used to construct examples that challenge common assumptions about the continuity and differentiability of functions.
- 📘 The script concludes by emphasizing that there are many counterexamples in calculus, encouraging a deeper understanding and critical thinking when dealing with mathematical theorems and properties.
Q & A
What is a counterexample in calculus?
-A counterexample in calculus is a specific example that disproves a general claim or theorem that might seem reasonable based on intuition or common cases. It demonstrates that a proposed statement is not universally true.
Why might one initially think that discontinuities of a function are isolated?
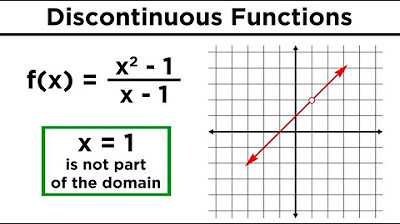
-One might think discontinuities are isolated because many common examples in calculus, such as the function x^2 - 1/(x - 1), exhibit isolated discontinuities. This function has a hole at x = 1, which is the point of discontinuity.
What is the Darboux function and why is it significant in calculus?
-The Darboux function is a function that takes the value of one for rational numbers and zero for irrational numbers. It is significant because it is discontinuous everywhere on the real line, serving as a counterexample to the claim that discontinuities must be isolated.
How does the function x^2 * sin(1/x) when x is not zero illustrate the claim that differentiability does not imply continuity of the derivative?
-The function x^2 * sin(1/x) is differentiable everywhere except at x = 0, but its derivative exhibits oscillatory behavior and does not settle to a constant value or zero as x approaches zero. This shows that even though the original function is differentiable at a point, its derivative is not necessarily continuous at that point.
What is the Squeeze theorem and how is it used in evaluating the limit of H * sin(1/H) as H approaches zero?
-The Squeeze theorem is a method for determining the limit of a function by 'sandwiching' it between two other functions with known limits. In this case, it is used to show that H * sin(1/H) oscillates between -H and H, both of which go to zero as H approaches zero, thus the whole expression also goes to zero.
What is the connection between a function's derivative and its increasing behavior on an interval?
-If a function's derivative is positive throughout an interval, it means the function is increasing on that interval. This is because the derivative represents the slope of the function at a point, and a positive slope indicates an increasing function.
How does the function x + x^2 * sin(1/x) serve as a counterexample to the claim that a positive derivative at a point implies the function is increasing on some interval around that point?
-The function x + x^2 * sin(1/x) has a positive derivative at x = 0, but it is not increasing on any interval around zero. The oscillations of the sine term ensure that there are points arbitrarily close to zero where the derivative is negative, thus the function does not maintain a consistent increasing behavior in any neighborhood of zero.
What might one intuitively expect about the asymptotic behavior of a derivative compared to the original function?
-One might expect that if the original function has a nice asymptotic behavior as x approaches infinity, then the derivative would as well. However, the example of the function sin(x^2)/x shows that the derivative can exhibit different behavior, such as not approaching zero.
What is a sequence of functions and how can it provide a counterexample to the claim that a limit of a sequence of functions with a property is also endowed with that property?
-A sequence of functions is an ordered list of functions, typically indexed by a natural number. The sequence x^n (where n is the index) converges to a limit function that is discontinuous, even though each function in the sequence (x^n for each natural number n) is continuous. This serves as a counterexample to the claim.
Why is it important to consider counterexamples when studying calculus?
-Counterexamples are important in calculus because they challenge intuition and common assumptions, ensuring a deeper and more accurate understanding of mathematical concepts. They highlight the nuances and exceptions to general rules or theorems.
How does the video script use technology to illustrate mathematical concepts?
-The video script uses Maple Learn, a mathematical software, to graph functions and their derivatives, illustrating the behavior of these functions visually. This aids in understanding complex concepts like differentiability, continuity, and the behavior of functions and their derivatives.
Outlines
🧮 Disproving Intuitions in Calculus
The first paragraph discusses the common misconception that discontinuities of a function are isolated, using the example of the function x^2 - 1 / (x - 1) which has an isolated discontinuity at x = 1. It then introduces the Dirichlet function as a counterexample, which is discontinuous everywhere because it takes the value of 1 for rational numbers and 0 for irrational numbers. The Dirichlet function serves to show that not all functions have isolated discontinuities and challenges the notion of continuity, as it is not continuous anywhere on the real line.
📈 Differentiability vs. Continuity of Derivatives
The second paragraph challenges the belief that if a function is differentiable, then its derivative must be continuous. It presents the function x^2 * sin(1/x) as an example, which is differentiable everywhere except at x = 0, but its derivative is not continuous. The explanation involves computing the derivative at x = 0 using the definition of the derivative and the squeeze theorem, showing that the derivative exists but does not imply continuity. The paragraph also touches on the concept of an increasing function and how it relates to the derivative being positive, providing a counterexample to the idea that a positive derivative at a point implies the function is increasing in a neighborhood around that point.
📉 Asymptotic Behavior and Sequences of Functions
The third paragraph explores the idea that a function's derivative may not share the same asymptotic behavior as the function itself. It uses the function sin(x^2) / x as an example, which approaches zero as x goes to infinity, but its derivative does not have a limit as x approaches infinity. The paragraph also discusses the misconception that a sequence of functions with a certain property, such as continuity, will necessarily converge to a function with that same property. A sequence of functions defined by x^N is given as a counterexample, where the sequence converges to a discontinuous function on the interval [0, 1].
📚 Conclusion and Invitation for Further Exploration
The final paragraph summarizes the video's content, highlighting five counterexamples that disprove common conjectures in calculus. It emphasizes the importance of understanding these counterexamples to gain a deeper insight into the subject. The speaker encourages viewers to like the video to support the content and to leave comments with any questions, promising more mathematical discussions in the next video.
Mindmap
Keywords
💡Counterexample
💡Discontinuity
💡Diraclis Function
💡Differentiable
💡Derivative
💡Increasing Function
💡Continuity
💡Asymptotic Behavior
💡Sequence of Functions
💡Squeeze Theorem
💡Limit
Highlights
Discontinuities of a function are not always isolated, which counters the intuition that they are, based on common examples in calculus.
The function x^2 - 1 / (x - 1) has an isolated discontinuity at x = 1, creating a hole in its graph.
There exist functions, like the Dirichlet function, that are discontinuous everywhere, contradicting the idea that discontinuities are isolated.
The Dirichlet function takes the value of 1 for rational numbers and 0 for irrational numbers, making it discontinuous across the entire real line.
The concept of continuity is based on the ability to zoom in on the domain without any gaps between the function's values and the limiting value.
A function being differentiable does not guarantee that its derivative is continuous, as demonstrated by the function x^2 * sin(1/x).
The derivative of x^2 * sin(1/x) exists at x = 0 but is not continuous, showing a discrepancy between differentiability and continuity of the derivative.
An increasing function on an interval is defined by the property that if x1 < x2, then f(x1) < f(x2) for all points in the interval.
A theorem connects derivatives to increasing functions, stating that if the derivative is positive throughout an interval, the function is increasing.
The derivative being positive at a single point does not guarantee that the function is increasing in any neighborhood around that point, as shown by the modified x^2 * sin(1/x) function.
The function x + x^2 * sin(1/x) has a positive derivative at x = 0 but is not increasing on any interval around zero.
The asymptotic behavior of a function as x approaches infinity does not necessarily apply to its derivative, as seen with sin(x^2)/x.
Even if a function has a limit as x approaches infinity, its derivative may not, as demonstrated by the non-converging limit of the derivative of sin(x^2)/x.
A sequence of functions with a nice property like continuity does not ensure that the limit of the sequence will also have that property, as shown by the sequence x^n.
The sequence x^n converges to a piecewise function that is discontinuous on the interval (0,1), despite each function in the sequence being continuous.
Counterexamples in calculus are crucial for understanding the limitations of theorems and the behavior of functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: