Tensor Calculus for Physics Ep. 9 | Derivatives of Tensors, and the Affine Connection
TLDRThis video script delves into the complexities of tensor calculus, particularly the challenges of differentiating tensors and the resulting non-tensorial terms. It introduces the concept of the affine connection, or Christoffel symbols, which are pivotal in addressing the issue of tensor transformation under general coordinate changes. The script uses an analogy of a falling 'Flat Earth' book to illustrate the problem and sets the stage for upcoming discussions on covariant differentiation, aiming to resolve the dilemma of non-commutative derivatives in curved spaces.
Takeaways
- 📚 The video discusses the concept of tensor calculus, specifically the problem of taking derivatives of tensors and how they may not always transform as tensors.
- 🔍 The script establishes the foundation of tensor transformations, including covariant and contravariant tensors, and the role of the metric tensor.
- 🤔 It introduces the main objective of the episode, which is to explore the implications of taking the derivative of a tensor and the resulting issues.
- 🔑 The concept of the affine connection is introduced to address the problem of derivatives not transforming as tensors.
- 📉 The script uses the example of a vector's transformation coefficients and the application of the product rule to illustrate the problem with derivatives.
- 🌐 An analogy is made comparing the issue to living on the surface of a sphere, where the curvature of space affects the transformation of vectors.
- 📚 The video script also discusses the implications of second derivatives, which often indicate curvature, and how this relates to the problem of tensor derivatives.
- 🌌 The idea of 'free fall' in a gravitational field is used to further explain the concept of the affine connection and its relation to acceleration.
- 🔄 The script mentions that in general, derivatives commute, but when they don't, the theory is said to have torsion, which is not the focus for the immediate future.
- 📝 The affine connection is defined with the introduction of the Christoffel symbols, which will be explored in more detail in future videos.
- 🔮 The video concludes by emphasizing the problem of tensor derivatives and hints at future discussions on how to resolve this issue within the framework of tensor calculus.
Q & A
What is the main objective of the video on tensor calculus for physics?
-The main objective of the video is to explore the problem that arises when taking derivatives of tensors and to introduce the concept of the affine connection, which is crucial for understanding how to properly differentiate tensors in a way that respects their transformation properties.
What is the issue with taking the derivative of a tensor?
-The issue is that the derivative of a tensor does not necessarily transform as a tensor should. This problem arises due to the presence of second derivative terms in the transformation equations, which can indicate curvature in the space being considered.
What is the transformation rule for a vector?
-The transformation rule for a vector states that the components of a vector in one frame transform to another frame using the transformation coefficients, which relate the components of the vector in the new frame to those in the old frame.
Why is the product rule necessary when differentiating the transformation of a vector with respect to time?
-The product rule is necessary because the transformation coefficients are not constants; they are functions of the coordinates, which themselves are functions of time. This requires the use of the product rule to account for the derivative of the coefficients themselves.
What is an example of a transformation where the second derivative terms in the transformation rule for a vector are zero?
-An example is a rotation or a Galilean transformation, where the transformation coefficients are constants, and thus their second derivatives with respect to any coordinate are zero.
What does the term 'curvature' imply in the context of the video?
-In the context of the video, 'curvature' implies that the space being considered may not be flat, and this curvature affects how tensors, such as vectors, behave when their derivatives are taken and compared at different points in the space.
What is the affine connection and why is it introduced?
-The affine connection, represented by the Christoffel symbols, is introduced to address the problem of derivatives of tensors not transforming as tensors. It provides a way to adjust the derivative so that it respects the transformation properties of tensors, even in curved spaces.
What is the significance of the 'chain rule' in the context of taking derivatives of tensor components?
-The chain rule is significant because it is used to take the derivative of tensor components that are expressed in terms of other variables, which are themselves functions of time or other coordinates. It allows for the proper calculation of derivatives in a coordinate-transformed space.
What assumption is made about the order of derivatives in the video?
-The assumption made is that derivatives commute, meaning that the order in which they are taken does not affect the result. This is a common assumption in most physical theories, and theories where this does not hold are said to have torsion.
How does the video relate the concept of the affine connection to the gravitational field?
-The video suggests that the affine connection, through the Christoffel symbols, is somehow related to the gravitational field. This relationship is further explored in future videos, where the connection between the affine connection and Newtonian physics is discussed.
What is the next step after introducing the affine connection in the video?
-The next step is to define the Christoffel symbols, which are the coefficients of the affine connection, and to explore how they transform, which will be discussed in the following videos.
Outlines
📚 Introduction to Tensor Derivatives and the Affine Connection
This paragraph introduces the concept of taking derivatives of tensors in the context of physics, highlighting the foundational knowledge required such as tensor transformation rules, covariant and contravariant tensors, and the metric tensor. The main issue discussed is the non-commutative nature of derivatives of tensors, leading to the introduction of the affine connection. The paragraph sets the stage for further exploration of the affine connection and its properties in subsequent videos.
🔍 Exploring the Transformation of Vector Components and the Product Rule
The speaker delves into the specifics of how vector components transform between different frames of reference, using the product rule to take the derivative of these components with respect to time. This is akin to calculating velocity. The paragraph discusses the complications that arise when the transformation coefficients are not constants, necessitating the use of the chain rule. The Einstein summation convention is adopted, and the importance of the order of derivatives is highlighted, with remarks on the implications if this assumption cannot be made.
🌐 The Impact of Curvature on Tensor Derivatives and the Concept of Affine Connection
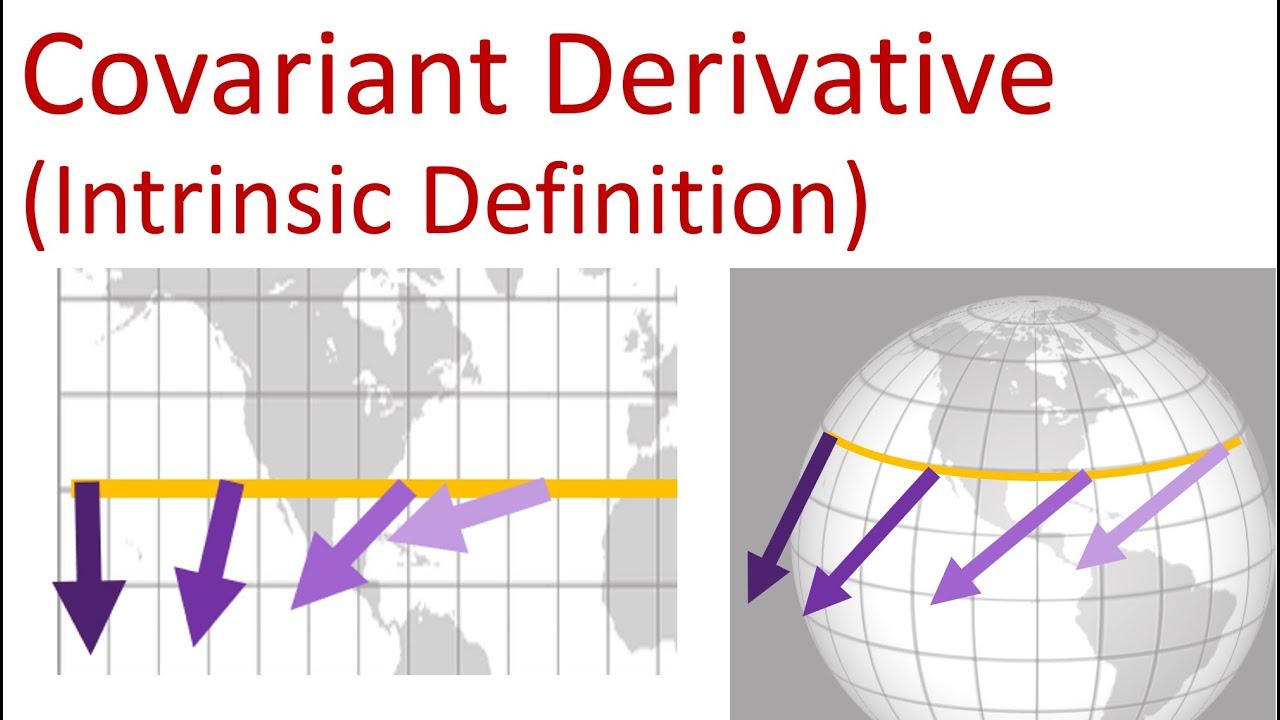
The paragraph explores the implications of space curvature on the transformation of vectors and the concept of the affine connection. It uses the analogy of an ant on a sphere to illustrate the challenges of comparing vectors in different tangent spaces. The discussion leads to the realization that the problem of non-commutative derivatives of tensors may be related to the curvature of space. The paragraph also introduces the idea of free-falling objects and how their acceleration is related to the gravitational field, setting the stage for the definition of the affine connection.
🛰️ The Affine Connection and Its Relation to Gravitational Fields
This paragraph focuses on the definition of the affine connection, which is introduced as a solution to the problem of non-commutative derivatives of tensors. The speaker explains how the affine connection, represented by the Christoffel symbols, is related to the acceleration of objects in free fall and, by extension, to gravitational fields. The paragraph also discusses the assumption of derivative commutativity and the implications of its violation, such as the presence of torsion. The summary concludes with a preview of upcoming topics, including the transformation properties of the affine connection and its connection to Newtonian physics.
Mindmap
Keywords
💡Tensor Calculus
💡Covariant and Contravariant
💡Metric
💡Derivative of a Tensor
💡Affine Connection
💡Christoffel Symbols
💡Covariant Derivative
💡Transformation Coefficients
💡Chain Rule
💡Curvature
💡Free Fall
Highlights
Introduction to the concept of taking derivatives of tensors and the associated problem that arises.
Exploration of tensor transformation, including covariant and contravariant transformations, and the role of the metric.
The problem of derivatives not transforming as tensors when taking derivatives of tensor components.
Introduction of the affine connection as a solution to the problem of non-transforming derivatives.
Explanation of how the transformation coefficients are not necessarily constants, leading to the need for the product rule in differentiation.
Use of the chain rule to derive the time derivative of the derivative of new coordinates with respect to old coordinates.
Adoption of Einstein summation convention to simplify the expression of derivatives and transformation rules.
Discussion on the implications of second derivatives in the context of curvature and the analogy of living on a sphere's surface.
Illustration of how the velocity vector is tangent to the position vector on a curved space.
The dilemma of comparing vectors in different points in a curved space and the need to modify the notion of a derivative.
Example of Galilean transformations and rotations where second derivative terms vanish, simplifying the transformation rule for vectors.
Introduction of the concept of the affine connection, denoted by gamma, and its relation to acceleration in a gravitational field.
Explanation of the symmetry of the affine connection coefficients and their implication in the absence of torsion.
The connection between the affine connection and the gravitational field in the context of free fall.
Preview of upcoming videos focusing on the affine connection, Christoffel symbols, and their transformation properties.
Discussion on the broader implications of the problem of derivatives of tensors for divergence and curl, not just time derivatives.
Conclusion emphasizing the problem of non-transforming derivatives of tensors and the upcoming solutions in future videos.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: