Tensor Calculus 9: Integration with Differential Forms
TLDRThis video script delves into the concept of integration with differential forms, illustrating how it connects to traditional integration and the calculation of areas under curves. It revisits the D operator as a covector field and explains the integral computation by counting contour lines crossed by a path. The script also covers the fundamental theorems of calculus, introduces gravitational potential, and demonstrates how work can be visualized and calculated using scalar fields and their level sets, emphasizing the path-independence of integral results and the elegance of differential forms in various coordinate systems.
Takeaways
- 📚 The video explains the concept of integration with differential forms and its relation to traditional integration of areas under curves.
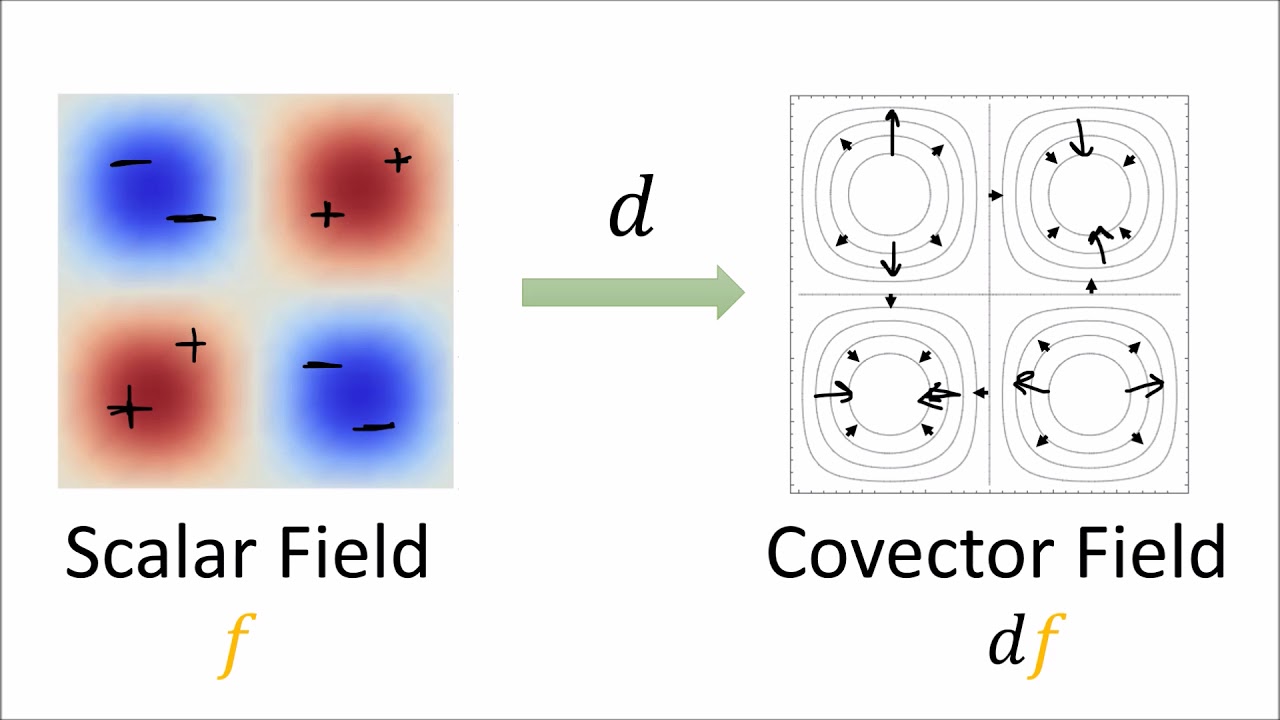
- 🔍 It assumes viewers have seen previous videos on differential forms, which reinterpret the 'D' operator to produce covector fields from scalar fields.
- 📈 The script discusses how covector fields, or differential forms, can act on vectors by counting the number of lines a vector pierces through the covector field at a point.
- 🌐 The video connects the 'D' operator in integrals to differential forms, explaining that integrals are computed by counting how many contour lines the path crosses in the differential form.
- 📚 Two important theorems are recalled: the fundamental theorem of calculus for single integrals and the gradient theorem for line integrals, both emphasizing the importance of endpoint values in computing integrals.
- 🔧 The concept of work in physics is used as an example to illustrate the application of differential forms in calculating the work done by a force field on an object.
- 🌗 The script uses the Earth's gravitational force field as an example to demonstrate how work is computed along a path, emphasizing that the work done is independent of the path taken.
- 📉 The video explains that when calculating work, the integral of the dot product of force vectors and tangent vectors simplifies to the integral of 'dPhi', where Phi is the gravitational potential.
- 🌟 The concept of gravitational potential is introduced as a scalar field, which simplifies the visualization and calculation of work done by a gravitational field.
- 📊 The video concludes by emphasizing that every integral involves a path and a covector field, and the result of an integral is the number of covector stacks pierced by the path, considering orientation.
- 🌍 The final takeaway is that the interpretation of integration with differential forms does not depend on the coordinate system used, making it a universal method for computing integrals.
Q & A
What is the main topic of the video?
-The main topic of the video is the explanation of how integration is done with differential forms and how it relates back to the traditional understanding of integration, particularly areas under curves.
What is the significance of the D operator in the context of the video?
-In the video, the D operator is reinterpreted as an operator that takes a scalar field F and produces a covector field DF, which represents the level sets of F oriented towards positive values.
How does the covector field DF behave like a function?
-The covector field DF behaves like a function by acting on vectors. To determine how DF acts on a vector V, one zooms in on the cofactor at that point and counts how many lines the vector pierces to get the output.
What is the relationship between the differential form and the traditional DX in integrals?
-The relationship is that every single integral involves a differential form and a path. The integral is computed by counting how many contour lines in the differential form that the path crosses.
What are the two important theorems from calculus mentioned in the video?
-The two important theorems mentioned are the Fundamental Theorem of Calculus for single integrals and the Gradient Theorem, which is also known as the Fundamental Theorem of Calculus for line integrals.
How does the video explain the concept of work in physics?
-The video explains work as the quantity obtained by applying a force on an object over some distance, calculated as the dot product of the force vector with the displacement vector.
What is the role of the gradient theorem in the video?
-The gradient theorem is used to compute the line integral involving the gradient of a function along a path, showing that the integral can be computed by knowing the value of the function at the endpoints.
How does the video illustrate the computation of work done by a gravitational field?
-The video uses examples of paths in a gravitational field, showing how to compute the work done by integrating the dot product of the force vector and the tangent vector along the path.
What is the concept of gravitational potential and how does it simplify the computation of work?
-Gravitational potential is a scalar field that represents the gravitational field. It simplifies the computation of work by allowing the use of the scalar field's level sets to determine the work done along a path by simply counting the contour lines pierced.
How does the video demonstrate that the choice of path does not affect the result of an integral?
-The video demonstrates this by showing that regardless of the path taken between two points, the work done (integral result) only depends on the endpoints, as the path's choice does not change the number of contour curves pierced.
What is the final takeaway from the video regarding the interpretation of integration with differential forms?
-The final takeaway is that every integral involves a path and a covector field (differential form), and the result of an integral is the number of covector stacks pierced by the path, considering the orientation.
Outlines
📚 Introduction to Differential Forms in Integration
This paragraph introduces the concept of integrating with differential forms and relates it to the traditional understanding of integration as areas under curves. The speaker assumes viewers have seen previous videos on differential forms and reminds them of the reinterpretation of the D operator to create covector fields, which are also known as differential forms. The paragraph also touches on how these fields can act on vectors and how integrals are computed by counting the number of contour lines crossed by a path in a differential form. The speaker sets the stage for the video by referencing two important theorems from calculus: the fundamental theorem of calculus and the gradient theorem, which are essential for understanding the integration process with differential forms.
🔧 Work as an Example of Line Integrals with Differential Forms
In this paragraph, the concept of work in physics is used to illustrate line integrals with differential forms. Work is defined as the dot product of force and displacement vectors, and the speaker uses examples of force fields, including gravity, to explain how work is calculated along a path. The paragraph delves into the complexities of calculating work when the force field varies and the path is curved, necessitating an integral approach. The speaker provides step-by-step examples, including a semicircle path where the gravitational field does no work due to perpendicularity of force and displacement vectors, and a straight-line path where gravity does negative work as the object moves against the field. These examples highlight the importance of the path and the endpoints in calculating work using differential forms.
🌐 Gravitational Potential and the Visualization of Work
The speaker introduces gravitational potential as a means to simplify the visualization of gravitational force and work done by it. Gravitational potential is described as a scalar field, Phi, which can be used to express the gravitational force as the negative gradient of this field. The paragraph explains how work can be recalculated using this potential field, emphasizing the simplification it brings to the computation. The speaker contrasts the complexity of using gradient vectors with the ease of using the scalar field itself, where work can be visualized as the change in potential between the start and end points of a path. The concept of level sets of the scalar field is introduced as an intuitive way to calculate work by counting the number of contour lines crossed by a path, independent of the path's specific shape.
📉 The Independence of Integrals from Path Choice in Differential Forms
The final paragraph emphasizes the main point of the video: the result of an integral involving a differential form is independent of the path taken between two endpoints. The speaker explains that the integral's outcome is determined solely by the number of covector field lines (or contour curves) crossed by the path, considering the orientation of these lines. This interpretation is shown to be coordinate-system-independent, making it a universal method for computing integrals. The speaker concludes by highlighting the upcoming video's promise to further explore this concept and its implications, especially in relation to coordinate system independence.
Mindmap
Keywords
💡Integration
💡Differential Forms
💡Covector Field
💡Fundamental Theorem of Calculus
💡Gradient Theorem
💡Work
💡Gravitational Field
💡Gravitational Potential
💡Contour Lines
💡Path Independence
Highlights
The video explains the integration of differential forms and its relation to traditional integration of areas under curves.
Reinterpretation of the D operator as a covector field, which acts on vectors by counting the number of lines pierced.
Every single integral involves a differential form and a path, computed by counting contour lines crossed by the path.
Fundamental theorem of calculus and the gradient theorem are key to understanding integration with differential forms.
The gradient theorem for line integrals is proven to provide insight into integrals with differential forms.
Work in physics is related to the dot product of force and displacement vectors.
Examples of work done by forces, such as gravity, are used to illustrate the concept of integration with differential forms.
Work done by a force field can be computed using an integral that considers the dot product of force and tangent vectors along a path.
The work done by gravity on an object traveling a semicircle path is shown to be zero due to perpendicular force and motion directions.
In contrast, straight-line paths against a gravitational field result in negative work, indicating the force opposes motion.
Gravitational potential is introduced as a scalar field that simplifies the visualization and computation of work.
The work done by a gravitational field can be seen as the change in gravitational potential between endpoints of a path.
Level sets of the scalar field are used to visually compute work by counting contour lines pierced by a path.
The choice of path does not affect the work done, as only the endpoints and the pierced contour lines matter.
Integration with differential forms is visualized as a path through a covector field, counting pierced contour curves.
The interpretation of integrals using differential forms is independent of coordinate systems, offering a universal approach.
The video promises further exploration of differential forms in the next installment, focusing on their coordinate independence.
Transcripts
Browse More Related Video
Tensor Calculus 10: Integration with Differential Forms Examples

Conservative Fields & Path Independence (Vector Fields)
The Fundamental Theorem of Calculus: Redefining Integration
Calculus Made EASY! Learning Calculus
Tensor Calculus 8: Covector Field Transformation Rules (Covariance)
Tensor Calculus 6: Differential Forms are Covectors
5.0 / 5 (0 votes)
Thanks for rating: