Calculating the Magnetic Flux for a Non-Uniform Magnetic Field
TLDRThe video script provides a detailed explanation on calculating magnetic flux through a hoop in a non-uniform magnetic field created by a wire. It begins by applying Ampère's law to determine the magnetic field at a point and then uses this to calculate the magnetic field for an infinitely long wire. The script introduces a method to find the flux through a thin strip of the hoop, assuming uniformity in the magnetic field over the strip. By integrating the magnetic field over the entire hoop, the final formula for the total magnetic flux is derived, showcasing the use of natural logarithms to account for the non-uniformity of the field.
Takeaways
- 🧲 The script discusses how to calculate magnetic flux for a non-uniform magnetic field.
- 📏 It uses the example of a hoop and a wire to illustrate the concept of magnetic field variation with distance.
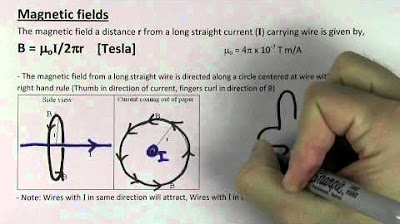
- 🔍 Ampère's law is applied to determine the magnetic field at a point, using an Ampèren loop.
- 📐 The magnetic field lines are visualized wrapping around the current-carrying wire, indicating field direction.
- ⚙️ The magnetic field B is found to be perpendicular to the differential length element (DL) in the loop.
- 🌀 The magnetic field B for an infinitely long wire is given by the formula: \( \mu I / (2\pi R) \).
- 🔄 The magnetic flux through a small area element is considered to be uniform due to the thinness of the strip.
- 📏 The magnetic flux through the hoop is calculated by integrating the product of B and the area element over the hoop's width.
- ∫ The total flux is obtained by summing the flux through all the thin strips from radius 'a' to 'a + w'.
- 📚 The final formula for the total flux through the hoop is derived as: \( \mu I h / (2\pi) \) times the natural logarithm of \( (a + w) / a \).
- 📝 The importance of considering the uniformity of the magnetic field in small areas for flux calculation is emphasized.
Q & A
What is the main topic discussed in the script?
-The script discusses how to calculate the magnetic flux for a non-uniform magnetic field, specifically through a hoop due to the magnetic field of a wire.
Why is the magnetic field considered non-uniform in this context?
-The magnetic field is considered non-uniform because its strength varies with distance from the wire, diminishing as one moves away from it.
What is the significance of Ampère's Law in this context?
-Ampère's Law is used to determine the magnetic field at any point along the wire, which is essential for calculating the magnetic flux through the hoop.
How does the script suggest applying Ampère's Law to find the magnetic field at a point?
-The script suggests drawing an Amperian loop around the point of interest and using Ampère's Law to calculate the magnetic field, considering the current and the geometry of the loop.
What is the relationship between the magnetic field B and the distance R from the wire?
-For an infinitely long wire, the magnetic field B at a distance R is given by B = (μ * I) / (2π * R), where μ is the permeability and I is the current.
Why can't the magnetic field B be pulled out of the integral when calculating the flux through the hoop?
-The magnetic field B cannot be pulled out of the integral because it is not uniform across the hoop; its strength varies with distance from the wire.
What is the approach taken to calculate the flux through a thin strip of the hoop?
-The approach is to consider the magnetic field B to be uniform across the thin strip due to its infinitesimal thickness and then calculate the flux as the product of B and the area of the strip.
How is the total magnetic flux through the hoop calculated?
-The total magnetic flux is calculated by summing the flux through all the thin strips from the inner radius a to the outer radius a + w, and then integrating with respect to the radius R.
What is the final formula for the total magnetic flux through the hoop?
-The final formula for the total magnetic flux is Φ = (μ * I * H) / (2π) * ln((a + w) / a), where H is the height of the hoop.
What assumptions are made in the script to simplify the calculation of magnetic flux?
-The script assumes that the magnetic field B is uniform across each thin strip of the hoop due to its infinitesimal thickness and that the hoop is symmetrical around the wire.
Outlines
🧲 Calculating Magnetic Flux in a Nonuniform Field
This paragraph explains the process of calculating magnetic flux through a hoop in a nonuniform magnetic field created by a wire. The speaker begins by setting the context of a decreasing magnetic field as one moves away from the wire, emphasizing the need to understand the magnetic field's strength at various points. Using Ampère's Law, the magnetic field (B) at a point is determined by considering an Ampèrean loop around the wire. The field's uniformity along the loop allows for simplification, leading to the formula B = (μ * I) / (2π * R) for an infinitely long wire. The discussion then shifts to the relationship between B and the area element (da), highlighting that B is perpendicular to da, allowing for the dot product to be eliminated and the magnetic flux to be calculated as an integral over the hoop's area.
📏 Integrating Magnetic Flux Across a Hoop
The second paragraph delves into the specifics of calculating the total magnetic flux through a hoop of width w, height h, and distance a from the wire. The speaker introduces the concept of breaking down the hoop into infinitesimally thin strips, each with a uniform magnetic field strength due to their proximity to the wire. The magnetic flux through each strip is then the product of the magnetic field strength (B) and the strip's area (da). By summing these fluxes across the entire hoop, an integral expression is formed. After simplifying the expression, the total magnetic flux is given by μ * I * h / (2π) times the natural logarithm of the ratio (a + w) / a, where a is the distance from the wire to the hoop's left side, and w is the hoop's width. This formula accounts for the nonuniformity of the magnetic field and provides a method to calculate the flux through the hoop as a function of its dimensions and position relative to the wire.
Mindmap
Keywords
💡Magnetic Flux
💡Non-uniform Magnetic Field
💡Ampère's Law
💡Magnetic Field
💡Flux Calculation
💡Permeability of Free Space (μ0)
💡Integral
💡Dot Product
💡Infinitesimally Thin Strip
💡Natural Logarithm
💡Magnetic Field Perpendicularity
Highlights
Calculation of magnetic flux for a non-uniform magnetic field is discussed.
The concept of a non-uniform magnetic field is introduced with an example involving a hoop and a wire.
Ampère's law is applied to determine the magnetic field at a specific point.
An empirical loop is drawn to visualize the magnetic field lines around the wire.
The magnetic field B is found to be parallel to the differential length element DL in the loop.
The integral of B dot DL is simplified using the symmetry of the magnetic field around the wire.
The formula for B in the case of an infinitely long wire is derived.
The magnetic flux is calculated by considering the perpendicularity of B to the area element DA.
A strip is introduced to break down the hoop into infinitesimal areas for flux calculation.
The magnetic field B is assumed to be uniform across the thin strip for simplification.
The flux through the entire hoop is found by summing the flux through each thin strip.
The total flux is expressed as an integral from a to a + w.
Constants are factored out of the integral to simplify the calculation.
The natural logarithm function appears in the final expression for the total flux.
The final formula for the magnetic flux through the hoop is presented.
The significance of the uniformity of B in the calculation process is emphasized.
The method concludes with a practical application of the derived formula for magnetic flux.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: