Flux and magnetic flux
TLDRThis video script offers an intuitive introduction to the concept of flux, starting with a tangible example of air particles moving through a net to illustrate how flux is influenced by density, velocity, and orientation. It then transitions to magnetic flux, explaining how it relates to the strength and direction of magnetic field vectors relative to a surface. The analogy between physical and magnetic flux is used to emphasize the importance of perpendicularity and field strength in determining the amount of flux.
Takeaways
- 🌀 The concept of flux generally refers to the amount of something flowing through a surface in a given time.
- 💨 Flux is influenced by the density and velocity of the substance in question, as well as the orientation of the surface relative to the flow.
- 🌬️ In the example of air, the flux increases with higher density and velocity of the air particles, and when the flow is perpendicular to the surface.
- 🔄 The orientation of the net (or surface) affects flux; parallel orientation to the flow results in zero flux, while perpendicular orientation maximizes it.
- 📏 The size of the surface also impacts flux; a larger surface area allows for more substance to flow through, thus increasing the flux.
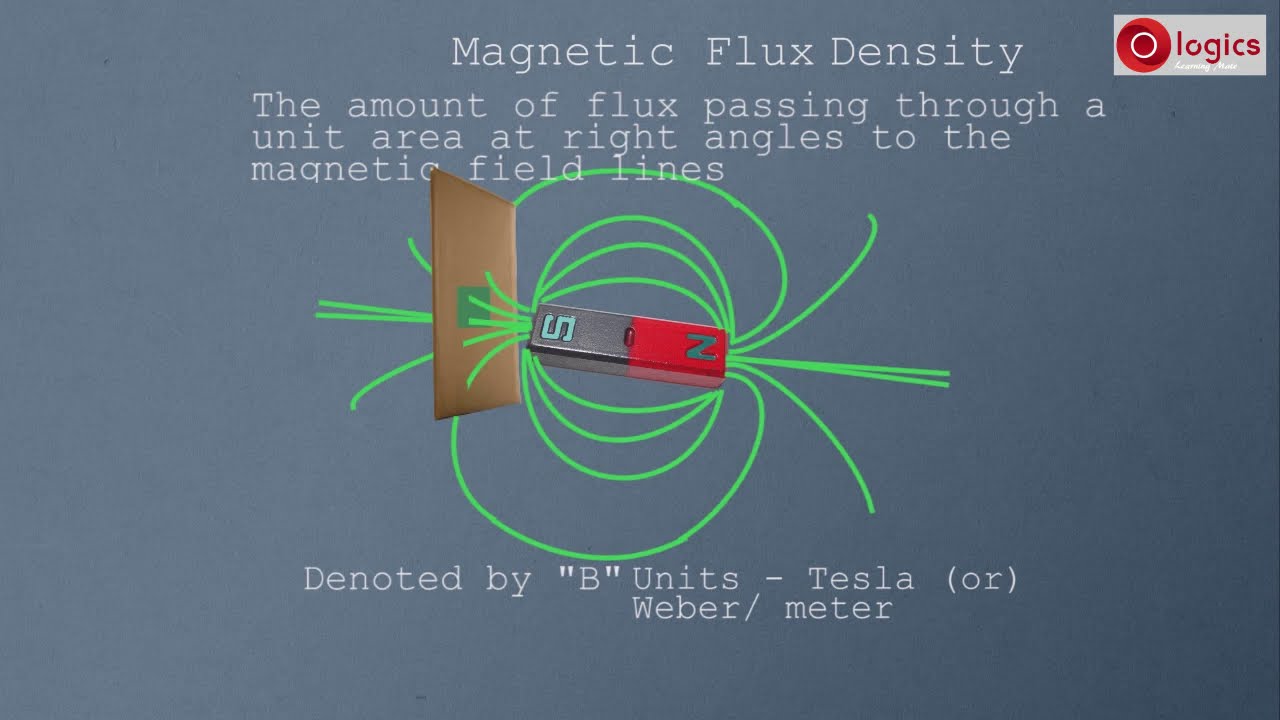
- 🧲 Magnetic flux is an application of the concept of flux, but instead of physical particles, it concerns the lines of magnetic force.
- 🌐 The strength and orientation of the magnetic field vectors in relation to the surface determine the magnetic flux.
- 💠 Magnetic flux is maximized when the field lines are perpendicular to the surface and minimized or zero when they are parallel.
- 🔗 Just as with physical flux, the density of the magnetic field (its strength) affects the amount of magnetic flux through a surface.
- 🛰️ Moving the surface further from the source of the magnetic field, where the field is weaker, results in decreased magnetic flux.
- 🔄 The analogy between physical flux and magnetic flux lies in the relationship between the direction of flow (or field lines) and the orientation of the surface.
Q & A
What is the general concept of 'flux' as introduced in the video?
-Flux is a measure of how much of something is flowing through a surface in a given amount of time. It is often used to describe the flow of a substance, like air or water, across a defined area.
How does air density affect the concept of flux in the video example?
-In the video, air density is used to illustrate how a denser area (with more particles) near the bottom of the volume will have a higher flux through a surface compared to a less dense area (with fewer particles) at higher altitudes.
What role do velocity vectors play in determining flux?
-Velocity vectors represent the speed and direction of the flow. The magnitude and direction of these vectors influence the flux, with greater velocities leading to a higher flux through a surface, assuming the density is constant.
How does the orientation of a surface affect the flux of air through it?
-The orientation of a surface relative to the flow direction of the air significantly affects the flux. If the surface is perpendicular to the flow, it will have a higher flux. If the surface is parallel to the flow, the flux will be minimal or zero.
What happens to the flux if the surface area through which the air is flowing is increased?
-Increasing the surface area will result in a higher flux, as there is more space for the air to flow through, assuming all other conditions remain constant.
Can you explain the analogy made between traditional flux and magnetic flux in the video?
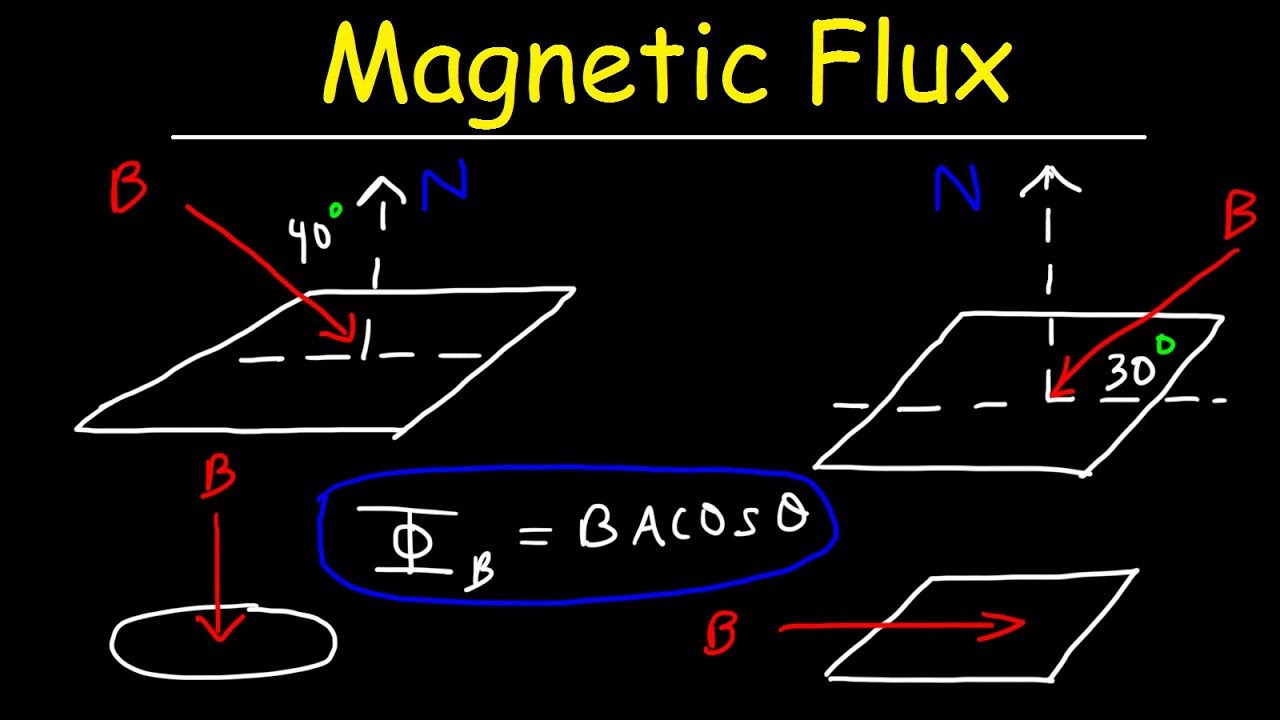
-The analogy between traditional flux and magnetic flux lies in the concept of 'flow through a surface.' While traditional flux deals with physical substances like air or water, magnetic flux considers the flow of the magnetic field lines through a surface.
What is the difference between physical flux and magnetic flux in terms of what is flowing?
-Physical flux involves the actual movement of particles or substances through a surface, whereas magnetic flux is concerned with the magnetic field lines and their interaction with a surface, without any physical movement of particles.
How does the strength of the magnetic field influence magnetic flux?
-The strength of the magnetic field directly influences the magnetic flux. A stronger magnetic field will result in a higher magnetic flux through a surface, provided the field lines are perpendicular to the surface.
What is the impact of the orientation of a surface relative to the magnetic field lines on the magnetic flux?
-If the surface is oriented perpendicular to the magnetic field lines, the magnetic flux will be higher. If the surface is parallel to the field lines, the magnetic flux will be minimal or zero.
How does the size of the surface area impact the magnetic flux?
-A larger surface area will allow for a greater amount of magnetic field lines to be perpendicular to the surface, resulting in a higher magnetic flux through that surface.
Can the concept of flux be applied to other fields or phenomena besides air and magnetic fields?
-Yes, the concept of flux can be applied to various fields and phenomena where there is a flow of something through a surface, such as electric fields, heat transfer, or even financial transactions.
Outlines
🌀 Introduction to Flux Concept
The video script introduces the concept of flux, explaining it as the measure of how much of something flows through a surface over a given time. Using air as an analogy, the narrator illustrates how air density and velocity affect the flux through a theoretical net. The net's position, orientation, and size relative to the flow of air are discussed to demonstrate how these factors influence the amount of air passing through it, which is analogous to flux. The summary emphasizes the importance of density, velocity magnitude, and direction in calculating flux.
🧲 Understanding Magnetic Flux
This paragraph delves into the concept of magnetic flux, drawing parallels with the previously discussed flux but focusing on the magnetic field instead of air particles. The narrator uses a bar magnet and field lines to explain how the magnetic field's strength and orientation to a surface determine the magnetic flux. The summary highlights that the component of the magnetic field perpendicular to the surface and the density of the field lines are crucial in calculating magnetic flux. It also touches on how changing the surface's orientation or moving it to an area with a weaker magnetic field can reduce the flux, and how increasing the surface area can increase the magnetic flux through it.
Mindmap
Keywords
💡Flux
💡Magnetic Flux
💡Surface
💡Density
💡Velocity Vectors
💡Air Flow
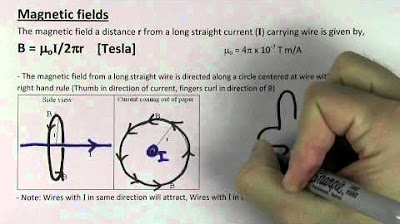
💡Magnetic Field
💡Field Lines
💡Component
💡Orientation
💡Strength of the Magnetic Field
Highlights
Introduction to the concept of flux as a measure of how much of something is flowing through a surface in a given time.
Flux conceptualized through the example of air density and movement, illustrating how air particles flow through a defined volume.
Explanation of how air density and velocity vectors affect the flux through a surface.
Visualization of flux through a net, demonstrating how air molecules pass through based on density and velocity.
The impact of surface orientation on flux, showing how perpendicular orientation maximizes flux.
Demonstration of how moving the net to an area of higher velocity increases flux.
Comparison of flux in areas of different air density and velocity, showing how flux varies.
The concept of zero flux when the direction of flow is parallel to the surface.
Illustration of how stretching a net (increasing surface area) results in increased flux.
Introduction to magnetic flux, drawing parallels with traditional flux but applied to magnetic fields.
Magnetic flux defined in terms of the component of the magnetic field normal to the surface.
The analogy between air molecule flow and magnetic field lines to explain magnetic flux.
How the orientation of a surface relative to magnetic field vectors affects magnetic flux.
The effect of moving a surface to an area of weaker magnetic field on magnetic flux.
The relationship between the strength of the magnetic field and the resulting magnetic flux.
The impact of surface size on the total magnetic flux through that surface.
Magnetic flux symmetry explanation, where flux through symmetrically positioned surfaces is the same.
Summary of the key properties of magnetic flux, drawing connections to physical flux concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: