Magnetic Flux | Physics with Professor Matt Anderson | M24-01
TLDRIn this engaging lecture, the instructor starts the session by discussing the weekend's Comic-Con event and the fun had with a young child dressed as a superhero. The focus then shifts to the topic of magnetic flux, explaining its relationship with the angle between magnetic field lines and a surface. The instructor uses the concept of 'flux' to illustrate how the number of magnetic field lines passing through a surface varies depending on the orientation of the surface relative to the field, providing examples with different angles to clarify the concept.
Takeaways
- 😀 The speaker starts with a casual greeting and mentions a common feeling of the 'Mondays', referencing the movie 'Office Space'.
- 🎉 The speaker shares a personal experience of attending Comic-Con with their young child, highlighting the fun and interesting atmosphere.
- 👶 The child's outfit, a mix of Superman, Batman, and a 'Shooter Man' cape, adds a humorous and relatable anecdote to the discussion.
- 📚 An update is given about a homework assignment, which has been postponed to the next day, eliciting a sigh of relief from the audience.
- 🕒 The session's structure is outlined, with a 45-minute discussion followed by a break and then a longer discussion, indicating a lengthy and comprehensive lesson.
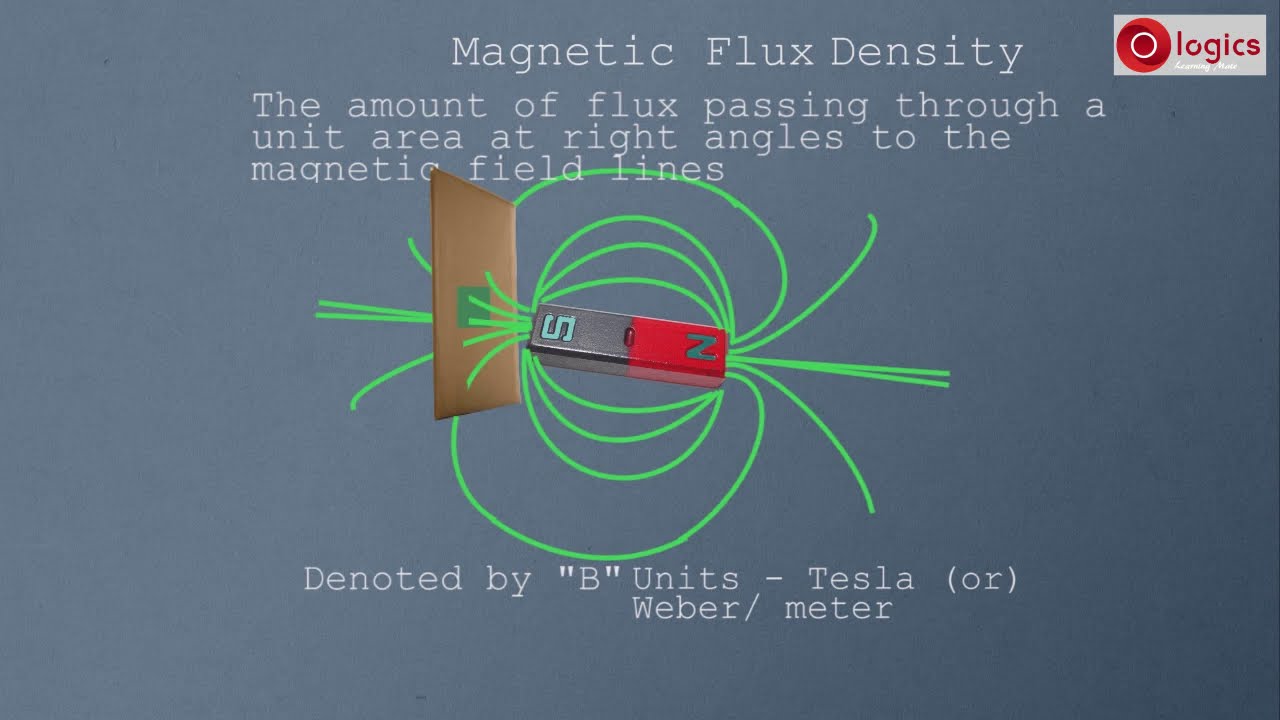
- 🧲 The concept of magnetic flux is introduced, explaining it as the number of magnetic field lines passing through a surface.
- 📏 The relationship between the orientation of the magnetic field (B) and the surface area is discussed, showing how it affects the flux.
- 📐 An example is given to illustrate how flux changes when the surface is tilted at different angles to the magnetic field lines.
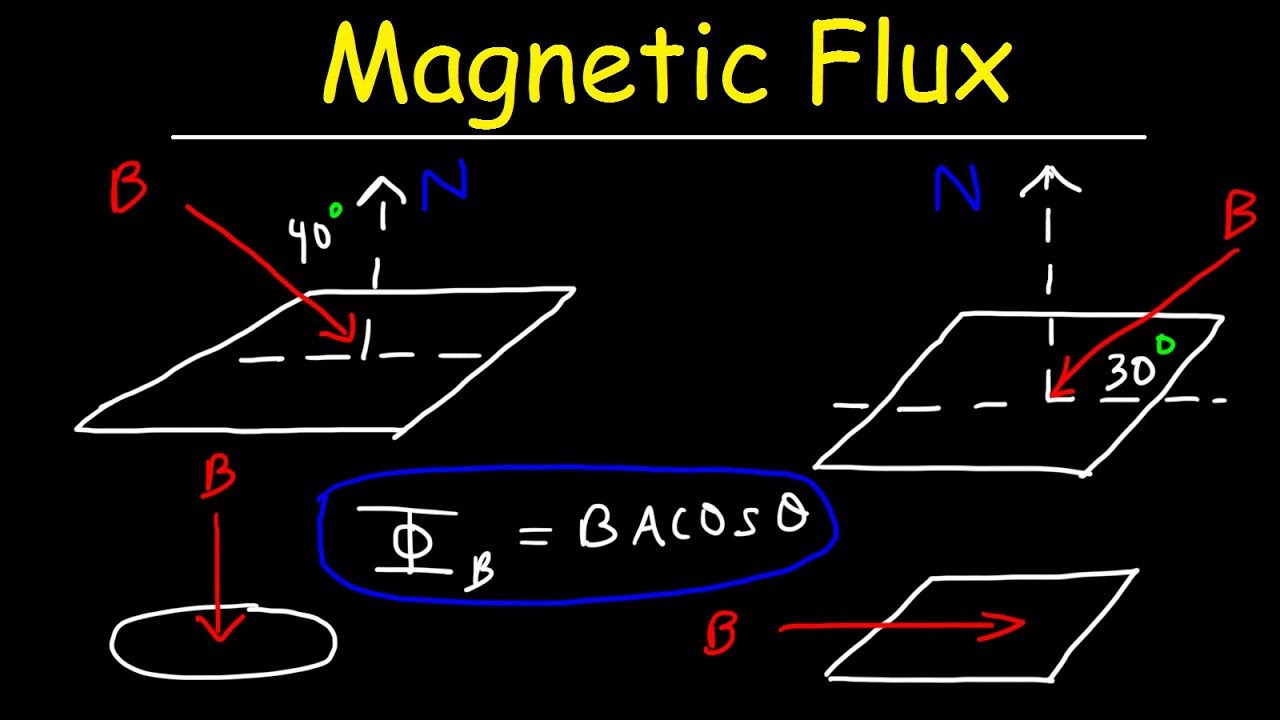
- 📚 The formula for calculating flux is presented: B times the area times the cosine of the angle between the field and the surface normal.
- 📝 A practical example is used to demonstrate the calculation of flux through different surfaces in an XYZ coordinate system with a magnetic field at an angle.
- 🔍 The importance of considering the angle between the surface normal and the magnetic field when calculating flux is emphasized.
Q & A
What is the speaker's initial mood or sentiment towards the start of the week?
-The speaker is trying to uplift the mood by asking how everyone is feeling, acknowledging the common feeling of the 'Mondays' and referencing the movie 'Office Space' to lighten the atmosphere.
What event did the speaker attend over the weekend?
-The speaker attended Comic-Con, where they walked around near the convention center and observed various interesting characters.
Why did the speaker's son dress up in a specific outfit?
-The speaker's son, who is three and a half years old, dressed up in a Superman hat, Batman shirt, and a cape that said 'Shooter Man' to fit in with the costumed attendees at Comic-Con.
What change was made to the homework due date mentioned in the script?
-The homework that was originally due on the night the script was given has been postponed to the following night, which brought a sigh of relief to several people.
What is the main topic of discussion for the session described in the script?
-The main topic of discussion is magnetic flux through a surface, including the concept of flux and how it is affected by the orientation of the surface relative to the magnetic field lines.
What is the definition of magnetic flux as described in the script?
-Magnetic flux, represented by the symbol 'Phi' (Φ), is defined as the number of magnetic field lines (B) passing through a given surface area (A), taking into account the angle between the field lines and the normal to the surface.
How does the speaker illustrate the difference in magnetic flux through surfaces oriented differently?
-The speaker uses the example of magnetic field lines pointing to the right and surfaces oriented perpendicularly and parallelly to these lines to demonstrate how the flux varies from three lines of B through a perpendicular surface to zero through a parallel surface.
What is the formula for calculating magnetic flux through a tilted surface?
-The formula for calculating magnetic flux through a tilted surface is Φ = B × A × cos(θ), where θ is the angle between the magnetic field lines and the normal to the surface.
What is the significance of the angle θ in the context of magnetic flux?
-The angle θ is significant because it determines the component of the magnetic field that contributes to the flux through the surface. When θ is 0 degrees, the flux is at its maximum, and when θ is 90 degrees, the flux is zero.
How does the speaker use the coordinate system to explain flux through different areas?
-The speaker introduces an x, y, z coordinate system with a magnetic field (B) pointing at an angle of 35 degrees in the y-z plane and then considers two areas, one in the x-z plane (Axz) and one in the x-y plane (Axy), to demonstrate how flux is calculated for each area based on the angle between the field and the surface normal.
What is the flux through the x-z plane (Axz) in the given example?
-The flux through the x-z plane (Axz) is calculated as B × A × cos(55 degrees), considering the angle between the surface normal and the magnetic field direction.
What is the flux through the x-y plane (Axy) in the given example?
-The flux through the x-y plane (Axy) is calculated as B × A × cos(35 degrees), since the surface normal is along the z-axis, which is the direction of the magnetic field.
Outlines
🎬 Comic-Con Recap and Magnetic Flux Introduction
The speaker starts by addressing the audience with a casual Monday morning greeting and mentions the feeling of the 'Mondays', referencing the movie 'Office Space'. They share a personal experience of attending Comic-Con with their three-and-a-half-year-old dressed in a superhero outfit, creating a fun and relatable atmosphere. The speaker then shifts to the main topic, which is magnetic flux, explaining its concept and how it is calculated through a surface. They illustrate the flux with examples of lines of magnetic field 'B' passing through different orientations of a surface, showing how the flux varies from three lines when perpendicular to zero when parallel, and something in between when at an angle.
📚 Magnetic Flux Calculation with Angle Variation
The speaker continues the discussion on magnetic flux, focusing on the relationship between the magnetic field 'B', the area 'A', and the angle 'theta' between them. They explain that the flux is maximized when the surface is perpendicular to the magnetic field lines and minimized when it's parallel. Using a detailed example with a coordinate system and a magnetic field at a 35-degree angle, they demonstrate how to calculate the flux through two different planes (xz and xy) by considering the cosine of the angle between the surface normal and the magnetic field. This explanation provides a clear understanding of how the orientation of a surface affects the magnetic flux through it.
🧲 Advanced Flux Calculations with Tilted Surfaces
In the final paragraph, the speaker emphasizes the complexity of flux calculations when the magnetic field and the surface are not at right angles or parallel to each other. They highlight the importance of considering the angle between the magnetic field and the surface normal when calculating flux, as this affects the number of magnetic field lines passing through the surface. The speaker's approach to explaining flux with varied angles and orientations provides a comprehensive understanding of the concept, preparing the audience for more advanced discussions or problems involving magnetic fields.
Mindmap
Keywords
💡Magnetic Flux
💡Office Space
💡Comic-Con
💡Homework
💡Flux
💡Surface Normal
💡Cosine
💡Coordinate System
💡Angle Theta
💡 Superman and Batman
💡Tilting
Highlights
Introduction to the concept of magnetic flux and its relation to lines of B (magnetic field) passing through a surface.
Discussion on the impact of surface orientation on magnetic flux, demonstrating how a perpendicular surface results in maximum flux.
Explanation of how tilting a surface affects the magnetic flux, with examples of horizontal and tilted planes.
Introduction of the formula for calculating magnetic flux: B * A * cos(theta), where theta is the angle between the magnetic field and the surface normal.
Illustration of how flux is zero when the surface is parallel to the magnetic field lines, as none of the lines penetrate the surface.
Example of calculating flux through different planes when the B field is at an angle, using the cosine of the angle between the field and the surface normal.
Use of a right-handed XYZ coordinate system to demonstrate the calculation of flux through areas in different planes.
Clarification on the importance of considering the angle between the B field and the surface normal, rather than the angle with the plane itself.
Detailed walkthrough of calculating flux through an area in the XZ plane with a B field at a 35-degree angle in the YZ plane.
Methodology for determining the relevant angle for flux calculation when the B field and surface normal are not aligned.
Application of the flux formula to calculate the magnetic flux through an area in the XY plane with the same B field conditions.
Emphasis on the practicality of understanding flux calculations for various orientations of B fields and surfaces.
Discussion on the implications of flux calculations for understanding magnetic fields in different spatial configurations.
Highlight of the importance of the cosine function in flux calculations and its role in determining the effective component of the magnetic field perpendicular to the surface.
Summary of the key principles for calculating magnetic flux through surfaces at various angles to a magnetic field.
Encouragement for students to engage with the material and apply the concepts to solve flux problems in different scenarios.
Note on the extension of the discussion to the next session due to the complexity and depth of the flux topic.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: