Product Rule | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRIn this educational segment, Christine Breiner explains the product rule for derivatives, initially reviewing the rule for two functions and then extending it to three functions. She uses the functions u, v, and w as examples, demonstrating how to find the derivative of their product by treating two of them as a single function and applying the rule iteratively. The lecture includes a practical example using powers of x and trigonometric functions, leading to a simplified expression of the derivative. The clear explanation and step-by-step process make the concept accessible and engaging for learners.
Takeaways
- 📚 The video script is a lesson on the product rule for derivatives, specifically extending it from two functions to three functions.
- 🔍 The product rule for two functions is first reviewed: (u*v)' = u'v + uv', where u and v are functions of x.
- 📝 The notation simplifies by dropping 'of x' for clarity, assuming u, v, and w are all functions of x.
- 📈 The product rule is extended to three functions, u*v*w, by treating v*w as a single entity and applying the product rule for two functions.
- 🔑 The derivative of u*v*w is expressed as u'vw + v'uw + w'uv, highlighting the pattern of taking the derivative of one function while leaving the others intact.
- 🧩 The pattern is further explained by breaking down the derivative into three terms, each representing the derivative of one function and the product of the others.
- 📐 The script suggests a pattern that can be generalized to the product of n functions, with n terms resulting from the derivative.
- 📚 An example is provided to apply the product rule to the function f(x) = x^2 * sin(x) * cos(x), encouraging the viewer to pause and attempt the problem.
- 📝 The example's solution is worked out step by step, applying the product rule to each of the three functions in the product.
- 📉 The final derivative of f(x) is given as 2x * sin(x) * cos(x) + x^2 * cos^2(x) - x^2 * sin^2(x), with a note on possible simplifications using trigonometric identities.
- 📝 The script concludes with the correct application of the product rule and an example that demonstrates the process clearly.
Q & A
What is the main topic discussed in the provided transcript?
-The main topic discussed in the transcript is the product rule for derivatives, specifically extending it to the product of three functions and then applying it through an example.
What is the product rule for two functions in calculus?
-The product rule for two functions states that if you have two functions u and v, the derivative of their product (u*v) is u' * v + u * v', where u' and v' denote the derivatives of u and v with respect to x.
How does the product rule for two functions apply to the product of three functions?
-The product rule for three functions is an extension of the rule for two functions. It involves treating two of the functions as a single entity and then applying the product rule for two functions, and then applying it again to the resulting expression.
What is the first step in finding the derivative of the product of three functions u, v, and w?
-The first step is to treat the product of two of the functions, say v*w, as a single function and then apply the product rule for two functions, considering u and (v*w) as the two functions.
What does the expression u'v*w + uv'*w + uw*v' represent in the context of the product rule for three functions?
-This expression represents the derivative of the product of three functions u, v, and w after applying the product rule for two functions, where u' is the derivative of u, v' is the derivative of v, and w' is the derivative of w.
How can you further simplify the expression obtained after applying the product rule for three functions?
-You can further simplify the expression by applying the product rule again to the term involving the derivative of the product of v and w, resulting in three terms: u'v*w, v'u*w, and w'u*v.
What is the example given in the transcript to illustrate the product rule for three functions?
-The example given is f(x) = x^2 * sin(x) * cos(x), and the task is to find the derivative f'(x).
What are the three terms obtained when applying the product rule to the example f(x) = x^2 * sin(x) * cos(x)?
-The three terms are 2x * sin(x) * cos(x), x^2 * cos(x) * cos(x), and -x^2 * sin(x) * sin(x).
How can the final expression of the derivative in the example be simplified further?
-The final expression can be simplified by factoring out common terms and using trigonometric identities, such as cos^2(x) - sin^2(x) which can be rewritten using the Pythagorean identity.
What is the significance of the product rule in calculus and why is it important to understand?
-The product rule is significant in calculus because it allows for the differentiation of products of functions, which is a common occurrence in various mathematical and real-world problems. Understanding the product rule is important for solving complex derivative problems and for applying calculus in fields such as physics and engineering.
Can the product rule be extended to more than three functions?
-Yes, the product rule can be extended to more than three functions. The pattern observed with three functions can be generalized to n functions, resulting in n terms when the derivative is taken.
What is the derivative of the fourth function in a product of four functions, if the product rule is applied?
-If the product rule is applied to a product of four functions, the derivative of the fourth function would be included in one of the terms, multiplied by the products of the derivatives of the other three functions.
Outlines
📚 Introduction to the Product Rule for Three Functions
Christine Breiner begins the segment by welcoming viewers back to the recitation and introducing the topic: the product rule for three functions. She starts by reviewing the product rule for two functions, which is essential for understanding the expanded rule for three functions. The rule is explained with the assumption that u, v, and w are all functions of x, and the notation is simplified for ease of writing. The segment aims to derive the product rule for the product of three functions, u*v*w, by treating v*w as a single entity and applying the known product rule for two functions. This leads to an expression involving the derivatives of u, v, and w, as well as the functions themselves.
🔍 Deriving the Product Rule for Three Functions
The paragraph continues with the derivation of the product rule for three functions. The process involves breaking down the product u*v*w into simpler components and applying the product rule for two functions iteratively. The result is an expression that includes the derivative of the first function times the product of the second and third, plus the derivative of the second function times the product of the first and third, and finally the derivative of the third function times the product of the first and second. The explanation is clear and methodical, ensuring that the viewer can follow the logic behind each step. The paragraph concludes with a hint at a pattern that could be extended to the product of more than three functions.
📘 Applying the Product Rule with Trigonometric Functions
In this paragraph, Christine provides an example to illustrate the application of the product rule for three functions. The example involves the function f(x) = x^2 * sin(x) * cos(x), and the task is to find its derivative, f'(x). The process is broken down into three terms, each corresponding to one of the functions in the product. The first term involves the derivative of x^2, which is 2x, multiplied by sin(x) and cos(x). The second term takes the derivative of sin(x), which is cos(x), and multiplies it by x^2 and cos(x). The third term involves the derivative of cos(x), which is -sin(x), and multiplies it by x^2 and sin(x). The final expression for f'(x) is simplified and presented in a clear format, demonstrating the application of the product rule with basic trigonometric functions.
Mindmap
Keywords
💡Product Rule
💡Derivative
💡Function
💡Trigonometric Functions
💡Powers of x
💡Recitation
💡Example
💡Simplification
💡Trig Identities
💡Coefficients
💡Summation
Highlights
Introduction to the product rule for three functions, building upon the product rule for two functions.
Assumption that u, v, and w are all functions of x, simplifying notation by dropping the 'of x'.
Review of the product rule for two functions, u*v, and its derivative.
Expansion of the product rule to three functions, u*v*w, by treating v*w as a single function.
Derivation of the product rule for three functions, resulting in u'vw + v'uw + w'uv.
Explanation of the pattern in the product rule, taking derivatives of the first, second, and third functions while leaving the others.
Anticipation of the pattern for the product rule with a fourth function, u*v*w*z.
Generalization of the product rule for the derivative of the product of n functions.
Computation of an example using the product rule with functions involving powers of x and trigonometric functions.
Example given: f(x) = x^2 * sin(x) * cos(x), with a challenge for viewers to find f'(x).
Step-by-step working out of the example, applying the product rule to find f'(x).
Identification of the three terms in the derivative of f(x): 2x*sin(x)*cos(x), x^2*cos(x), and -x^2*sin(x).
Simplification of the derivative expression by grouping polynomials and coefficients.
Final expression for f'(x): 2x*cos(x)*sin(x) - x^2*sin(x)^2.
Mention of alternative ways to rewrite the derivative using trigonometric identities.
Conclusion on the method to write the derivative of the given function f(x) in a clear and reader-friendly manner.
Transcripts
Browse More Related Video
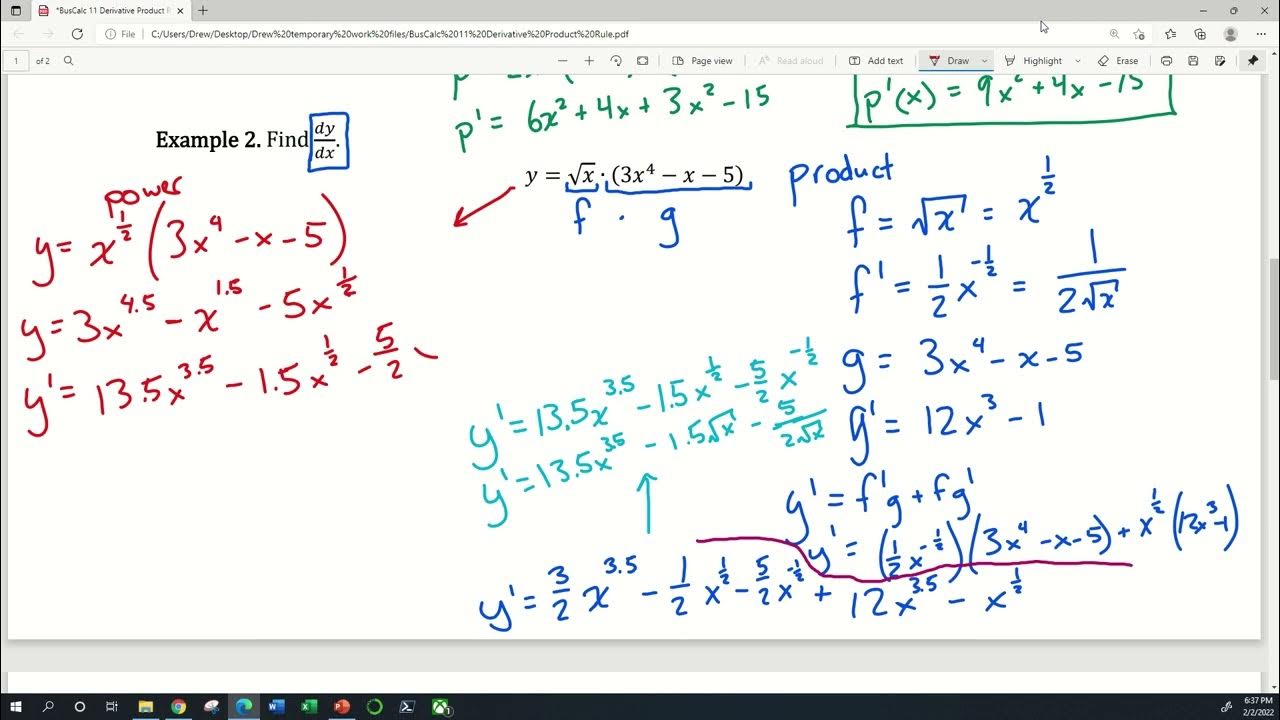
BusCalc 11 Derivative Product Rule
Rules of Logs | MIT 18.01SC Single Variable Calculus, Fall 2010

Derivative of e_____cos(e_) | Advanced derivatives | AP Calculus AB | Khan Academy

1.6 - The Product and Quotient Rules

The Product Rule for Derivatives — Topic 57 of Machine Learning Foundations

Chain rule with the power rule
5.0 / 5 (0 votes)
Thanks for rating: