Calculus Chapter 4 Lecture 38 Elements
TLDRIn this calculus lecture, Professor Greist explores various applications of differential elements beyond the traditional area, volume, and length, delving into physics, finance, and more. He explains how to calculate the mass of objects with variable density, the torque on a distributed force, and the force exerted by a fluid on a tank's side. Additionally, the lecture touches on financial concepts like present value, illustrating how integrals can solve complex real-world problems across different disciplines.
Takeaways
- 📚 The lecture introduces various differential elements like area, volume, surface area, length, and work, and discusses their integration to solve problems.
- 🔍 The process for computing definite integrals is outlined, emphasizing determining the differential element 'DU' and then integrating to find 'U'.
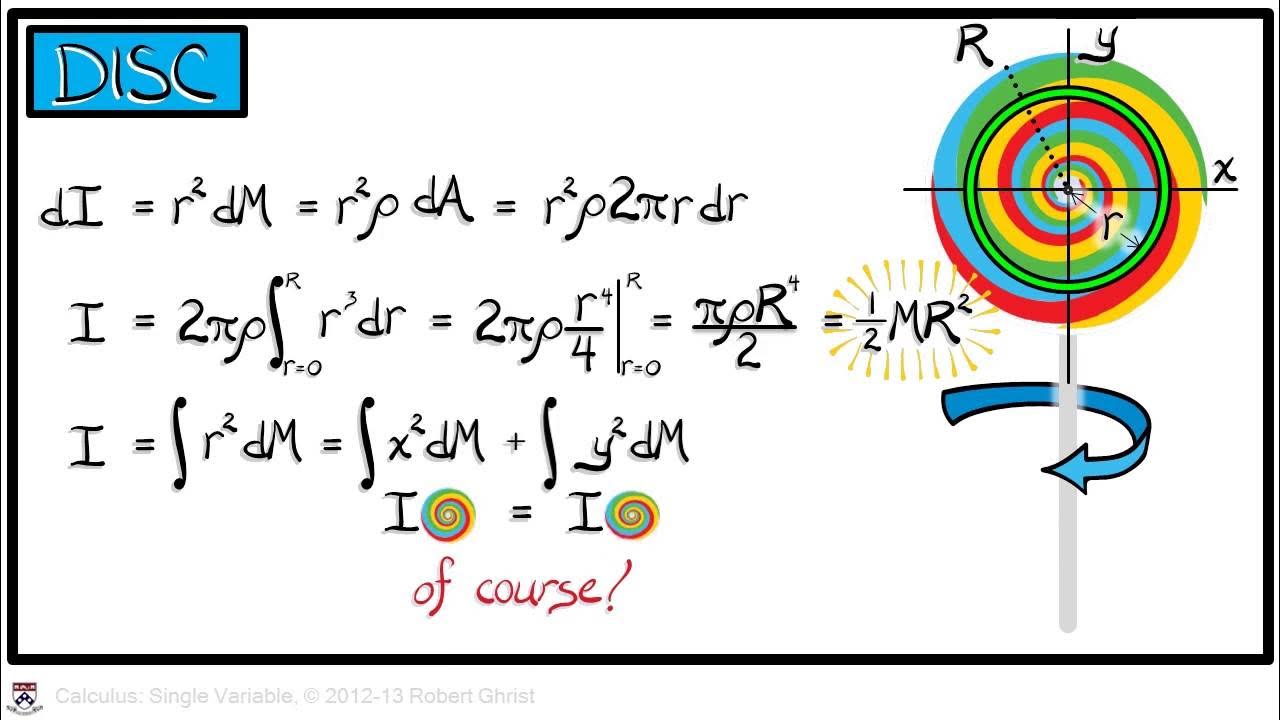
- 📏 The concept of linear density 'Rho' is explained as the rate of change of mass with respect to a coordinate, used to calculate mass elements in objects like a pen.
- 🌏 The mass of the Earth is considered in terms of volumetric density as a function of the radial coordinate 'R', leading to an integral expression for mass.
- 🔧 The torque experienced due to a force is discussed, with the torque element 'DT' being the product of the perpendicular force and the distance from the point of application.
- 🏗 The force exerted by a fluid on a tank is examined, using the differential force element 'DF' which is the product of pressure and the area element.
- 🚰 An example of calculating the net force on an end cap of a cylindrical tank filled with fluid is provided, using a coordinate transformation for simplification.
- 💰 The concept of present value in finance is introduced, explaining how future money is worth less today due to the time value of money and interest rates.
- 📈 The present value of an income stream is calculated using the present value element 'DPV', integrating over time to find the total present value.
- 🔬 The lecture emphasizes the importance of understanding the procedure for solving problems with integrals rather than memorizing every possible application.
- 🚀 The next lesson will focus on computing averages using integrals, indicating a continuation of the topic with a different application.
Q & A
What is the main focus of Professor Greist's lecture 38 on calculus?
-The main focus of the lecture is to explore various examples of differential elements that can be integrated to solve problems in different fields, ranging from physics to finance.
What is the procedure for computing the definite integral as discussed in the lecture?
-The procedure involves first determining the appropriate differential element, denoted as D U, and then integrating to compute the value of U.
How is the mass of an object like a pen computed using calculus?
-The mass of a pen can be computed by setting up a coordinate along the pen, slicing it into thin pieces, and integrating the product of the linear density function and the length element DX.
What is the difference between linear density and volumetric density?
-Linear density, often denoted as Rho, is the rate of change of mass with respect to a change in coordinate, while volumetric density is the mass per unit volume, often measured in grams per cubic centimeter.
How is the mass of the Earth computed considering the density as a function of the radial coordinate?
-The mass of the Earth is computed by integrating the product of the volumetric density function and the volume element, which is the surface area of a sphere (4 PI R squared) times the infinitesimal thickness dR, over the radial coordinate from 0 to 6400 kilometers.
What is torque and how is it related to force and distance?
-Torque is the measure of the force that can cause an object to rotate about an axis, and it depends on the magnitude of the force and the perpendicular distance from the point of force application to the axis of rotation.
How can the torque on an arm with a distributed weight be computed?
-The torque can be computed by integrating the product of the distance along the arm (X), the gravitational constant (G), and the linear density function (Rho of X) over the length of the arm.
What is the differential formulation for the force exerted by a fluid on the side of a tank?
-The force element (DF) is the product of the pressure (P), which depends on the fluid's density and depth, and the area element (Da) on the side of the tank.
How is the net force on an end cap of a cylindrical tank filled with fluid calculated?
-The net force is calculated by integrating the product of the pressure, which is the weight density (Rho) times the depth (X), and the area element over the surface of the end cap.
What is the concept of present value in finance and how is it related to future money?
-The present value is the amount of money today that is equivalent to a certain amount of money to be received in the future, considering a fixed interest rate and continuous compounding.
How can the present value of an income stream be computed?
-The present value is computed by integrating the product of the income stream function (I of T) at time T and the discount factor e to the negative RT over time.
Outlines
📚 Introduction to Differential Elements in Calculus
Professor Greist introduces the concept of differential elements in calculus, discussing the integration of area, volume, length, and work elements to solve various problems. The lecture emphasizes the procedure for computing definite integrals by determining the appropriate differential element 'DU' and integrating it. Examples range from physics, like mass computation of a pen and the Earth, to financial concepts such as present value. The importance of understanding the underlying procedure for solving problems using integrals is highlighted.
🔧 Applications of Differential Elements in Physics and Torque
This section delves into the application of differential elements in physics, specifically focusing on torque and the force exerted by fluids. The torque element 'DT' is defined as the product of distance 'X' and the force element 'DF', which is derived from mass, acceleration due to gravity, and linear density. The concept of weight density is introduced, and the process of calculating the force exerted by a fluid on the side of a tank is explained using differential force elements. An example of calculating the net force on an end cap of a cylindrical tank is provided, illustrating the integration process.
💰 Financial Application: Present Value Calculation
The final paragraph explores the financial application of differential elements, particularly the calculation of present value. It explains how future money is worth less today due to the time value of money and the effect of interest rates. The present value element 'DPV' is introduced, which is the product of the exponential decay factor 'e^(-RT)' and the income 'I(T)' at time 'T'. The process of integrating this element over time to find the present value of an income stream is outlined, providing a practical example of applying calculus in finance.
Mindmap
Keywords
💡Calculus
💡Differential Element
💡Definite Integral
💡Linear Density
💡Volumetric Density
💡Torque
💡Pressure
💡Force Element
💡Present Value
💡Continuously Compounded Interest
💡Integration
Highlights
Introduction to lecture 38 on differential elements in calculus.
Explanation of the procedure for computing definite integrals using differential elements.
Application of calculus to compute mass elements and linear density.
Mass of a pen example using calculus to determine mass through linear density.
Concept of volumetric density and its application to calculate the mass of the Earth.
Integration of mass elements to find the Earth's mass as a function of radial coordinate.
Introduction to torque, its definition, and physical intuition through everyday experiences.
Calculation of torque using differential elements and linear mass density functions.
Integration of torque elements to determine the total torque on an object.
Force exerted by a fluid on a tank, explained through pressure and weight density.
Differential formulation of force on the side of a tank using pressure and area elements.
Net force calculation on an end cap of a cylindrical tank filled with fluid.
Change of coordinates to simplify the integration of force elements on a cylindrical tank.
Integration of force elements to find the net force exerted by fluid on a tank's end cap.
Introduction to the financial concept of present value and its calculation.
Explanation of present value computation for an income stream using differential elements.
Emphasis on understanding the integral procedure for solving problems rather than memorizing formulas.
Anticipation of the next lesson focusing on computing averages through integrals.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: