Calculus Chapter 4 Lecture 30 Simple Areas
TLDRIn this calculus lecture, Professor Gist introduces the concept of using definite integrals for calculating areas, starting with simple geometric shapes like rectangles, parallelograms, and triangles. He explains how these areas can be derived from the integral of the area element, demonstrating through examples that traditional area formulas are essentially the result of integration. The lecture also touches on the application of these concepts to more complex shapes and introduces the Gini Index, an economic measure of income inequality, showcasing the practical applications of calculus in various fields.
Takeaways
- 📚 The lecture introduces the concept of using definite integrals to calculate areas, moving away from memorizing specific formulas for different shapes.
- 📐 The area of a parallelogram can be found by integrating the area element, which is the product of the base and the infinitesimally small change in height (dy).
- 🔄 Shearing a parallelogram and sliding the horizontal slices to form a rectangle preserves the area element, demonstrating the principle behind the area calculation.
- 📉 The area of a triangle can be derived by considering a vertical strip with height from the equation of the hypotenuse and width dx, leading to the formula for area as base times height.
- ⭕ The area of a circle is approached by considering an infinitesimally small wedge and approximating it as a triangle, leading to the integral of the area element.
- 🔄 The area of a circle can also be calculated by integrating the circumference times the infinitesimally small thickness (dt) of the annuli formed by a radial variable.
- 📈 The area between two curves is found by integrating the difference in their y-values (F(x) - G(x)) over the interval [A, B].
- 💰 The Gini Index, an economic measure of income inequality, is defined as the ratio of the area between the income distribution curve and the line of perfect equality to the area of the triangle formed by the line and the x-axis.
- 📊 The Gini Index can be calculated using calculus by integrating the difference between the income distribution and the line of perfect equality over the population.
- 📈 An example of calculating the Gini Index for a power law distribution of income is provided, showing how it relates to the polynomial degree of the distribution.
- 🌐 The script concludes by emphasizing the importance of revisiting classical area formulas to deepen understanding and prepare for tackling more complex planar shapes in future lessons.
Q & A
What is the main focus of the lecture 30 on calculus?
-The main focus of the lecture is on the applications of the definite integral, starting with simple area formulas and progressing to more complex topics.
What is the fundamental formula for calculating area according to the lecture?
-The fundamental formula for calculating area is the integral of the area element, denoted as 'da'.
How does the lecture explain the area of a parallelogram using calculus?
-The lecture explains the area of a parallelogram by slicing it into horizontal strips of infinitesimally small thickness 'Dy', and then integrating the product of the base 'B' and 'Dy' from 0 to the height 'H' of the parallelogram.
What rearrangement argument is used to understand the area of a parallelogram?
-The rearrangement argument used is that by cutting the parallelogram into pieces and reshuffling them, one can form a rectangle, whose area is well-known, and thus understand the area of the parallelogram.
How is the area of a triangle explained in the lecture using calculus?
-The area of a triangle is explained by considering a vertical strip of infinitesimally small thickness 'DX' and height given by the equation of the triangle, and then integrating the product of the height and 'DX' from 0 to the base 'B' of the triangle.
What is the equation used to describe the hypotenuse of the right triangle in the triangle area example?
-The equation used to describe the hypotenuse of the right triangle is 'y = H / B * x'.
How does the lecture use the concept of an area element to calculate the area of a circular disc?
-The lecture considers an infinitesimally small wedge defined by an angular variable 'Theta' and approximates it as a triangle, with the area element being half the base times the height, which is '1/2 * R * R * dTheta', and then integrates this from 0 to 2π to find the area.
What is the Gini Index and how is it related to the concepts discussed in the lecture?
-The Gini Index is an economic measure used to quantify income inequality in a population. It is related to the concepts discussed in the lecture as it involves calculating the area between two curves, which is a fundamental concept of integral calculus.
How is the Gini Index calculated according to the lecture?
-The Gini Index is calculated as the ratio of the area between the line representing the actual income distribution 'F(x)' and the line representing an even income distribution 'y = x', divided by the area of the triangle formed by 'y = x' and the axes.
What is an example of a power law distribution and how does it relate to the Gini Index?
-An example of a power law distribution is when income is distributed as a polynomial of 'x' to the power of 'n'. The Gini Index for such a distribution can be calculated by integrating 'x - F(x)' from 0 to 1, doubling the result, and then dividing by 1/2, yielding '(n+1) / (2n)'.
How does the lecture illustrate the concept of different area elements for calculating the area of a circular disc?
-The lecture illustrates the concept by considering different methods of dividing the disc into area elements, such as using angular variables to create thin wedges, radial variables to create thin annuli, and vertical slices to create strips, each with its own integral for calculating the area.
Outlines
📚 Introduction to Calculus and Area Calculation
Professor Gist begins Lecture 30 by transitioning from classical mathematical tools to applications of the definite integral, starting with simple area formulas. He emphasizes that instead of memorizing numerous formulas, there is a fundamental concept that area is the integral of the area element, 'da'. The lecture revisits classical area formulas for shapes like rectangles, parallelograms, triangles, and circles, explaining their validity through the integral of the corresponding area elements. The professor uses the parallelogram as an example to demonstrate how slicing it into horizontal strips and integrating these strips can lead to the area calculation. He also introduces the concept of shearing a parallelogram to form a rectangle, which preserves the area element, and applies a similar approach to the area of a triangle.
📐 Advanced Area Calculations and Economic Applications
Continuing the discussion on area calculation, the script delves into more complex shapes and introduces the concept of the Gini Index, an economic measure of income inequality. The area of a circular disc is revisited, this time using different methods to calculate it, such as considering angular variables and radial variables, leading to the integral of area elements. The script explains how the area can be determined by integrating half the product of the radius and the differential angle, or by considering the circumference times the thickness of an infinitesimally thin anulus. The Gini Index is introduced as the ratio of the area between the actual income distribution and an even distribution to the area of the triangle formed by an even distribution, which is a measure of economic disparity within a population.
📉 Calculating the Gini Index with Economic Data
The script provides a practical example of calculating the Gini Index using a power law distribution model for income. It explains how to integrate the difference between the polynomial distribution and the even distribution from 0 to 1, and then double the result to find the Gini Index. The example shows that if the income distribution followed a cubic monomial, the Gini Index would be calculated by a specific integral, resulting in a value that reflects the deviation from an equitable income distribution. The script also references real economic data, mentioning that in 2010, New York State had a Gini Index of approximately 1/2, suggesting a significant income disparity.
🔍 Reflecting on Classic Formulas and Preparing for Complex Shapes
In the final paragraph, the script reflects on the importance of revisiting classic area formulas to deepen understanding and prepare for tackling more complex planar shapes in future lessons. It emphasizes that while students may already be familiar with the formulas for areas of triangles and circles, the lecture serves to reinforce the foundational concept that these areas can be derived from the integral of area elements. The professor hints at the upcoming challenges of calculating areas of more intricate shapes, setting the stage for advanced topics in calculus.
Mindmap
Keywords
💡Calculus
💡Definite Integral
💡Area Element
💡Parallelogram
💡Triangle
💡Circular Disc
💡Gini Index
💡Shearing
💡Power Law Distribution
💡Trigonometric Substitution
Highlights
Introduction to Lecture 30 on simple areas using definite integrals.
Transition from classical mathematical tools to their applications in calculus.
Explanation of the fundamental formula for area as the integral of the area element.
Classical area formulas revisited through the lens of integral calculus.
Derivation of the area of a parallelogram using horizontal strips.
Shearing and sliding of a parallelogram to preserve the area element.
Alternative approach to the area of a triangle using horizontal shearing.
Calculation of the area of a triangle using the integral of a vertical strip.
Introduction to the area of a circular disc using an angular variable.
Different methods for calculating the area of a circle using various area elements.
Exploration of the area between two curves and its economic applications.
Definition and explanation of the Gini Index in economics.
Calculation of the Gini Index for a power law distribution of income.
Historical data on the Gini Index for the state of New York in 2010.
Theoretical implications of a cubic distribution of income based on the Gini Index.
Importance of revisiting classical formulas to deepen understanding and prepare for complex problems.
Anticipation of tackling more complex planar shapes in the next lesson.
Transcripts
Browse More Related Video
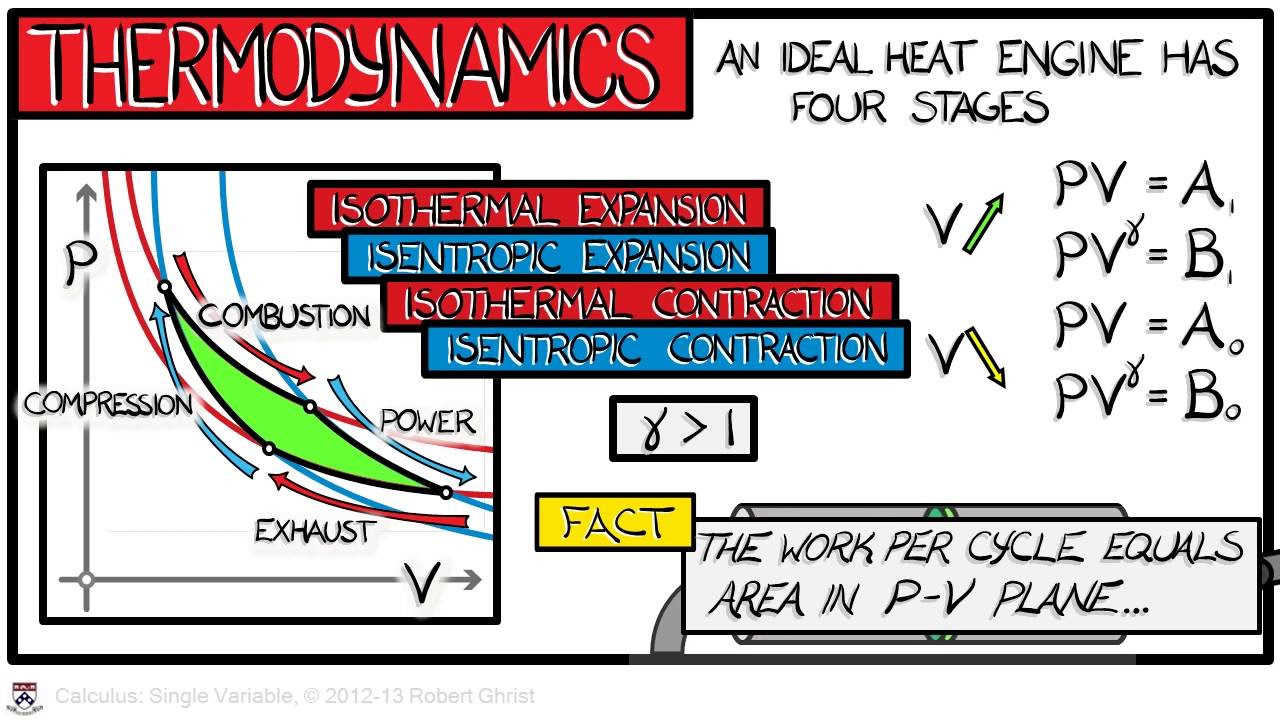
Calculus Chapter 4 Lecture 31 Complex Areas
Calculating the Area of Shapes

Lec 18 | MIT 18.01 Single Variable Calculus, Fall 2007

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Calculus 3: Green's Theorem (Video #30) | Math with Professor V

Definite Integrals from Graphs (Part 1 of 3)
5.0 / 5 (0 votes)
Thanks for rating: