20. Fluid Dynamics and Statics and Bernoulli's Equation
TLDRIn this comprehensive lecture, Professor Ramamurti Shankar delves into the principles of fluid dynamics and statics, starting with foundational concepts such as fluid density and pressure. He explains the formal definition of pressure as a scalar quantity, using the example of a submerged cube within a fluid to illustrate the concept. The lecture progresses to discuss the relationship between fluid pressure, depth, and the resulting force, leading into the hydrostatic equilibrium principle. Professor Shankar also covers the construction of a barometer, the dynamics of drinking through a straw, and the use of U-tubes to determine fluid densities. The lecture further explores Archimedes' principle, illustrating how the buoyant force allows objects to float and the conditions under which they do so. Finally, Bernoulli's equation is introduced, highlighting how fluid velocity and pressure are interrelated, and its implications for various real-world applications such as the lift of an airplane wing and the functioning of a Venturi meter. The lecture concludes with practical problems involving holes in a water tank and the behavior of fluids in different scenarios, emphasizing the utility of Bernoulli's principle in understanding fluid flow and its practical applications.
Takeaways
- 📚 The concept of fluid dynamics and statics involves understanding the properties of fluids like water or oil, including density (ρ) and pressure.
- 🧊 Density is defined as mass per unit volume, and for water, it's typically 1,000 kilograms per cubic meter.
- 📊 Pressure in a fluid is the force per unit area exerted by the fluid in all directions and is measured in Pascals (Newtons per square meter).
- 🌊 Pressure in a fluid increases with depth, meaning that at any given depth, all points within a fluid have the same pressure.
- 📈 The pressure at a point within a fluid can be calculated using the formula P = P_0 + ρgh, where P_0 is the pressure at the surface, ρ is the fluid's density, g is the acceleration due to gravity, and h is the depth.
- 🚗 In the context of a car tire, gauge pressure is the pressure above atmospheric pressure, which is necessary to maintain the tire's function.
- 🎓 The principle of Archimedes states that the upward buoyant force on a body immersed in a fluid is equal to the weight of the fluid displaced by the body.
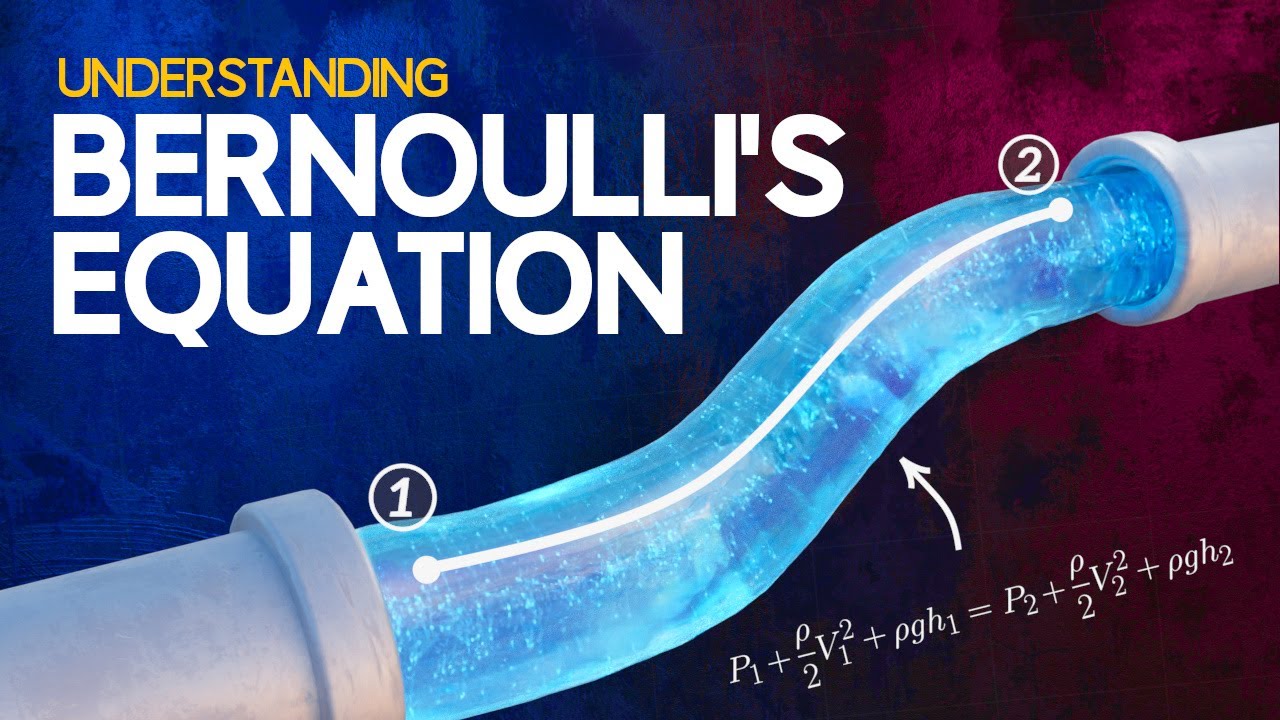
- ✈️ Bernoulli's Equation, P + ½ρV^2 + ρgh = constant, describes the conservation of energy for an incompressible fluid in motion, relating pressure, kinetic energy, and potential energy.
- 🚀 An airplane's lift is a result of the pressure difference created by the faster airflow over the top of the wing compared to underneath it, according to Bernoulli's principle.
- 💧 The speed at which water flows out of a hole in a tank can be determined using Bernoulli's equation, with the velocity of the water being proportional to the square root of the height of the water above the hole.
- 🌪 Viscosity, a property not considered in ideal fluid dynamics, causes a fluid to experience resistance when in contact with the walls of a pipe, leading to a decrease in speed near the walls and increased pressure drop.
Q & A
What is the primary topic discussed in the transcript?
-The primary topic discussed in the transcript is fluid dynamics and statics, including concepts such as fluid pressure, density, and the principles of Archimedes and Bernoulli.
What is the formal definition of pressure?
-Pressure is defined as the force exerted by a fluid on a unit area. It is an intensive property, meaning it does not depend on the size of the volume of the fluid.
What is the unit of pressure in the International System of Units (SI)?
-The unit of pressure in the SI system is the Pascal (Pa), which is equivalent to one Newton per square meter (N/m²).
How does the pressure within a fluid change with depth?
-The pressure within a fluid increases with depth. At any given depth, all points within the fluid experience the same pressure due to the fluid being in equilibrium.
What is the Archimedes' principle?
-Archimedes' principle states that the upward buoyant force that is exerted on a body immersed in a fluid is equal to the weight of the fluid that the body displaces.
What is the relationship between the atmospheric pressure and the height of a fluid column in a barometer?
-The atmospheric pressure is equal to the pressure at the top of the fluid column in a barometer, which is the sum of the pressure due to the fluid's weight (ρgh) and the vacuum pressure (zero pressure at the top of the column).
How does the shape of an object affect its buoyancy in a fluid?
-The shape of an object can affect how much of it is submerged in a fluid and thus its buoyancy. However, the key factor determining whether an object floats or sinks is its density relative to the fluid's density.
What is the principle behind a hydraulic press?
-The principle behind a hydraulic press is the transmission of force through a fluid, which allows for the amplification of force. This is due to the fact that the pressure in a fluid at a given height is the same in all directions, and thus the force exerted on a smaller area can be translated to a larger force on a larger area.
How does Bernoulli's equation relate to the speed and pressure of a fluid in motion?
-Bernoulli's equation states that for a fluid in motion, the sum of the pressure energy, kinetic energy, and potential energy per unit volume remains constant along a streamline. This means that an increase in the speed of the fluid (kinetic energy) results in a decrease in pressure if the potential energy and the elevation remain constant.
What is the equation of continuity in fluid dynamics?
-The equation of continuity in fluid dynamics states that for an incompressible fluid, the product of the cross-sectional area and the velocity at one point in the fluid is equal to the product of the cross-sectional area and the velocity at any other point in the fluid. Mathematically, this is expressed as A₁V₁ = A₂V₂.
How can one estimate the speed of an airplane using Bernoulli's principle?
-One can estimate the speed of an airplane using Bernoulli's principle by measuring the pressure difference between a point in the undisturbed airflow and a point in the airflow where the airplane's shape causes the airflow to speed up. The faster airflow over the wings of the airplane results in lower pressure, and this pressure difference can be related to the speed of the airplane.
Outlines
📚 Introduction to Fluid Dynamics and Statics
Professor Shankar begins by introducing the topic of fluid dynamics and statics, noting that it's a fundamental subject even for those with high school physics knowledge. He explains that fluids, like water or oil, can be characterized by properties such as density (ρ), and that pressure, which increases with depth in a fluid, is a key concept. The formal definition of pressure is discussed, highlighting that it's a scalar quantity, measured as force per unit area, and denoted in Pascals. The concept of gauge pressure and absolute pressure is also introduced, with examples provided to illustrate these principles.
🔍 The Equilibrium of Pressure in Fluids
The paragraph delves into the principle that pressure in a fluid at a given depth is the same in all directions, leading to equilibrium. Using the mental model of an imaginary cylindrical section within the fluid, it's demonstrated that any imbalance in pressure would result in net force, contradicting the equilibrium state. The pressure difference between two depths in a fluid is then calculated, showing that it depends on the density of the fluid, gravitational acceleration, and the height difference between the two points.
🌌 Atmospheric Pressure and its Effects
This section discusses the concept of atmospheric pressure, which is the pressure exerted by the weight of the air above us. It is explained that although we live at the bottom of a 100-mile-high 'pool' of air, we do not experience the full pressure due to the air passing through our bodies. The relationship between atmospheric pressure, density, and height is given as ρgh, with the standard atmospheric pressure being 10^5 Pascals. An experiment involving a heated and sealed can is used to illustrate changes in pressure.
🧪 Applications of Pressure Principles
The applications of the principles of pressure are explored, including the creation of a barometer to measure atmospheric pressure by using the height of a mercury column. The principle behind drinking through a straw is also explained, which involves creating a pressure differential to draw liquid up the straw. Additionally, the concept of a U-tube filled with different fluids is introduced to demonstrate how the difference in fluid heights can be used to determine the relative densities of the fluids.
🔧 Hydraulic Press and Archimedes' Principle
The hydraulic press, which uses the principle of pressure to amplify force, is described. It's shown that by applying force to a smaller piston in a hydraulic system, a larger force can be exerted on a larger piston due to the conservation of energy and the incompressible nature of the fluid. Archimedes' principle is then introduced, stating that an object submerged in a fluid is buoyed up by a force equal to the weight of the fluid displaced by the object. The principle is applied to explain why objects of different densities float or sink, and the concept of the fraction of an object's volume that will be submerged based on its density relative to the fluid is discussed.
🚢 Buoyancy and Floating Bodies
The principles of buoyancy are further elaborated through the example of a boat floating on water. It's explained that a boat made of steel can float due to its hollow design, which displaces a volume of water equal in weight to the boat itself. The critical loading point of a boat, where it is on the verge of sinking, is also discussed, highlighting the balance between the boat's weight, cargo, and the weight of the displaced water. The paragraph concludes with a mention of Bernoulli's Equation, which will be addressed in the next topic, and its significance in considering fluids in motion.
🚿 Equation of Continuity and Fluid Flow
The 'equation of continuity' is introduced, which relates the flow rate of an incompressible fluid through different sections of a pipe. It's shown that the product of the cross-sectional area and the velocity of the fluid at any point must be constant, leading to the conclusion that as the pipe's cross-sectional area decreases, the fluid's velocity must increase to maintain continuity. The implications of this principle for fluid dynamics, such as the behavior of water in pipes of varying sizes, are discussed.
🌟 Bernoulli's Equation and its Applications
Bernoulli's equation is derived and explained in the context of fluid dynamics. The equation, which states that the sum of pressure, kinetic energy, and potential energy per unit volume remains constant along a streamline, is applied to various scenarios. Examples include the behavior of a spinning baseball, the lift generated by an airplane wing, and the functioning of an atomizer. The principle that an increase in the speed of a fluid results in a decrease in pressure is highlighted, and the qualitative and quantitative aspects of Bernoulli's equation are discussed.
✈️ Measuring Velocity with Bernoulli's Principle
The final paragraph explores practical applications of Bernoulli's principle, such as measuring the speed of an airplane or the flow rate of a liquid in a pipe using a device called a Venturi meter. The principle that a decrease in pressure corresponds to an increase in fluid velocity is utilized to determine these velocities. The Venturi meter works by creating a constriction in the pipe, which increases the fluid velocity and decreases the pressure, measurable by the difference in height of a fluid in a secondary tube. This principle is also used to explain how the height of fluid in an open tube adjusts when the tube is opened or closed to the atmosphere.
Mindmap
Keywords
💡Fluid Dynamics
💡Pressure
💡Density
💡Archimedes' Principle
💡Bernoulli's Equation
💡Hydraulic Press
💡Atmospheric Pressure
💡Gauge Pressure
💡Equation of Continuity
💡Barometer
💡Venturi Effect
Highlights
Introduction to fluid dynamics and statics, emphasizing the importance of understanding fluid properties such as density and pressure.
Explanation of pressure as an intensive property, defining it as the force per unit area within a fluid.
Discussion on the units of pressure, including Newtons per square meter and Pascals.
Illustration of pressure differences in a gas within a cylinder and the effect of external forces on pressure.
Differentiation between absolute pressure and gauge pressure, with practical examples such as tire pressure.
Concept that pressure in a fluid at a given depth is the same at all points, demonstrated through a cylindrical section of fluid.
Calculation of pressure difference in a fluid column based on height and density, leading to the formula P = P_0 + ρgh.
Real-world application of the pressure formula in the construction of barometers to measure atmospheric pressure.
Use of the pressure formula to understand the limitations of drinking through a straw and the concept of vacuum.
Practical application of the density formula using a U-tube to compare the densities of two different fluids.
Explanation of the hydraulic press principle, where a small force can be amplified to lift heavier loads, adhering to the conservation of energy.
Introduction to Archimedes' principle, which states that the buoyant force on an object submerged in a fluid is equal to the weight of the fluid displaced.
Discussion on the conditions for an object's buoyancy based on the density of the object relative to the fluid it is in.
Explanation of why ice floats on water due to its lower density compared to liquid water.
Application of Archimedes' principle in the design of boats and ships, balancing the weight of the boat with the weight of the displaced water.
Introduction to Bernoulli's Equation for analyzing fluid flow, focusing on the conservation of energy in a fluid system.
Derivation of Bernoulli's Equation using the law of conservation of energy and its implications for fluid dynamics.
Practical use of Bernoulli's Equation in determining the speed of fluid exiting an orifice in a tank.
Application of Bernoulli's principle in the design of Venturi meters for measuring flow rates in pipes.
Transcripts
Browse More Related Video

8.01x - Lect 28 - Hydrostatics, Archimedes' Principle, Bernoulli's Equation

Fluids (AP Physics SuperCram Review)
Understanding Bernoulli's Equation

AP Physics 2 Fluids Review

IMPORTANT Derivations in Physics: Bernoulli's Equation

2022 Live Review 1 | AP Physics 2 | Working Fluids Multiple-Choice and Free-Response Questions
5.0 / 5 (0 votes)
Thanks for rating: