Fun Math Videos!
TLDRThis homeschool pop video script offers an engaging and fun exploration of various mathematical concepts. It covers fractions, teaching how they represent parts of a whole, using relatable examples like pizza sharing. Roman numerals are introduced as an ancient numerical system, with simple mnemonics to aid learning. Division is simplified through storytelling, emphasizing equal sharing, while multiplication is likened to solving mysteries with easy-to-follow examples. The script also delves into perimeter calculation for 2D shapes and introduces 3D shapes like the sphere, cone, pyramid, cylinder, cube, and rectangular prism, making learning about geometry interactive and enjoyable.
Takeaways
- 🍕 Fractions are introduced as parts of a whole, such as splitting a pizza into equal parts for multiple people.
- 🔢 The fraction is composed of a numerator (top number) representing the part of the whole and a denominator (bottom number) representing the total number of equal parts.
- 🏀 Fractions can be used to represent proportions, such as the fraction of sports balls that are basketballs in a collection.
- 🐱 Roman numerals are an ancient system of representing numbers using letters like I, V, and X, where I stands for 1, V for 5, and X for 10.
- 📖 Special Roman numerals include IV for 4, IX for 9, and they are combined to represent numbers up to 30.
- 🥕 Division is the process of splitting a number into equal parts, such as dividing carrots equally among friends.
- 🚫 Division by zero is undefined and represents an impossible operation in mathematics.
- ↔️ Division can be represented in three ways: using the division symbol, a slash, or a horizontal line.
- 🔁 Division has an inverse property where the quotient and the divisor can be flipped while maintaining the truth of the equation.
- 🔢 Even and odd numbers are distinguished by their divisibility by two; even numbers can be grouped into pairs, while odd numbers have a leftover when grouped by two.
- 🛣️ Perimeter is the total distance around a shape, calculated by adding up the lengths of all its sides.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is various mathematical concepts, including fractions, Roman numerals, division, even and odd numbers, multiplication, perimeter, and three-dimensional shapes.
What is a fraction according to the video script?
-A fraction, as described in the video script, is something that shows parts of a whole. It consists of a numerator, which indicates the number of parts being considered, and a denominator, which represents the total number of equal parts.
Can you explain the concept of Roman numerals as presented in the video script?
-The video script explains Roman numerals as an ancient system of writing numbers using letters from the Latin alphabet. It introduces the numerals I for one, V for five, and X for ten, and demonstrates how to combine them to represent other numbers.
What is the definition of division given in the script?
-In the script, division is defined as the process of splitting a number up by any given number. It is illustrated through the example of dividing a certain number of items equally among a group of people.
How does the script describe the difference between even and odd numbers?
-The script describes even numbers as those that can be grouped by two, meaning they have pairs, while odd numbers are those that cannot be evenly grouped by two, leaving at least one without a pair.
What is the basic principle of multiplication explained in the video script?
-The basic principle of multiplication explained in the script is that it involves taking the number in each group and multiplying it by the number of groups to get the total count.
How is the perimeter of a shape calculated according to the video script?
-According to the video script, the perimeter of a shape is calculated by adding all the sides of the shape, which gives the total distance around the shape.
What are the key characteristics of a sphere as a 3D shape?
-A sphere, as a 3D shape, is perfectly round with all points on its surface equidistant from its center. It is the three-dimensional version of a circle.
What is the significance of the rhyme '3D shapes are fat not flat' in the script?
-The rhyme '3D shapes are fat not flat' is used in the script to help distinguish between two-dimensional (flat) and three-dimensional (solid) shapes, emphasizing that 3D shapes have depth.
Can you provide an example of a 3D shape from the script and its characteristics?
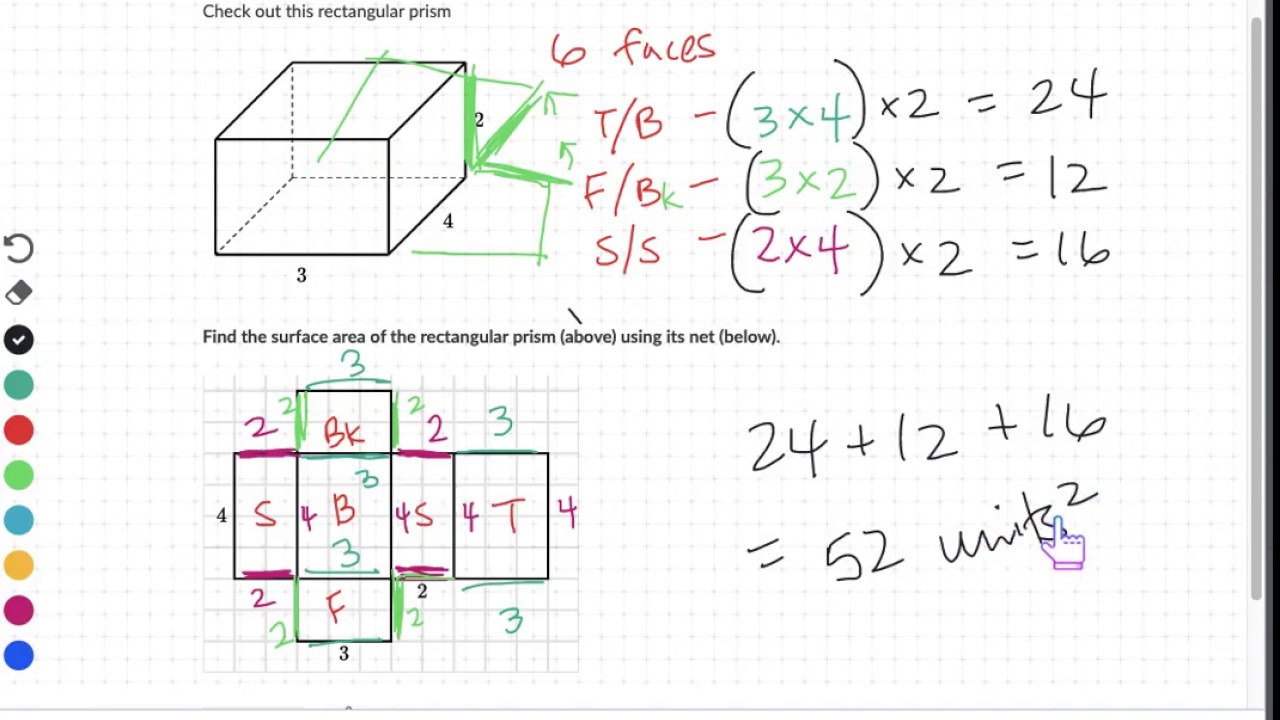
-An example of a 3D shape from the script is the cube. It is the three-dimensional version of a square and has six sides or faces, all of which are squares.
What are the two tricks for even and odd numbers mentioned in the script?
-The two tricks mentioned in the script for even and odd numbers are: 1) Numbers alternate from odd to even and back as you count up, and 2) For numbers bigger than 10, whether they are odd or even can be determined by the last digit (the units place).
Outlines
🍕 Fractions and Pizzas: Understanding the Basics
This paragraph introduces the concept of fractions using the relatable example of sharing a pizza. It explains that a fraction represents parts of a whole, using the scenario of Cindy and Chomsky dividing a pizza to illustrate one-half. The explanation includes the definition of numerators and denominators, emphasizing that the denominator shows the total parts and the numerator shows the parts being considered. The paragraph aims to demystify fractions and present them as a fundamental and commonly used mathematical concept.
🏀 Sports Balls and Fractions: Applying Fractions to Real-Life
Building upon the basic understanding of fractions, this paragraph explores how to determine what fraction of a group is represented by a subset, using sports balls as an example. It outlines the process of identifying the total number of items (the denominator) and the number of items in the subset of interest (the numerator). The paragraph reinforces the concept of fractions by showing how they can be used to describe parts of a collection, such as one-fifth being basketballs out of five sports balls.
🐱 Fractions of Pets and Roman Numerals: More Fractions and a Glimpse into History
This paragraph continues the theme of fractions with a different example, determining what fraction of a group of pets are cats. It also introduces Roman numerals as an ancient method of representing numbers, explaining the basic symbols for one (I), five (V), and ten (X). The paragraph serves to further familiarize viewers with fractions and offers an introduction to the historical context of Roman numerals, adding a cultural element to the mathematical lesson.
🥕 Division with Carrots: Learning the Concept of Division
Using the story of Ethan and his friends sharing carrots, this paragraph teaches the concept of division. It simplifies division as the act of splitting a number evenly among a certain quantity. The paragraph explains how to perform division with the example of three carrots shared among three kids, resulting in one carrot per child. It emphasizes the idea that division is about fairness and equal distribution, and introduces the division sign, highlighting that division by zero is undefined.
🔢 Division Facts and Multiple Representations: Exploring Division's Characteristics
This paragraph delves into interesting facts about division, such as the impossibility of dividing by zero and the various ways to represent the division operation, including the traditional division sign, a slash, and a horizontal line. It also introduces the concept that the order of the divisor and dividend can be switched without changing the outcome of the division, providing a deeper understanding of division's properties and its mathematical notation.
🦖 Even and Odd Numbers: Unraveling the Patterns
The paragraph introduces the distinction between even and odd numbers using a playful detective theme with dinosaurs. It explains that even numbers can be grouped into pairs, while odd numbers have one left over. The summary includes the identification of even and odd numbers through examples with dinosaurs and mentions two tricks: the alternation pattern of odd and even numbers, and the rule that the last digit of a number determines its parity, with even numbers ending in 0, 2, 4, 6, or 8.
🚴♂️ Multiplication as a Detective Game: Cracking the Code of 'Times'
This paragraph presents multiplication as a fun detective game where one must figure out the total number of items in several groups. It explains the basic concept of multiplication as adding the same number several times, using the examples of bicycles with tires and packs of trading cards. The paragraph simplifies multiplication as a process of adding a number to itself as many times as indicated by another number, emphasizing the importance of understanding the number of groups and the number within each group.
🎮 Multiplication Tricks and Tables: Simplifying the Process
Building on the multiplication concept, this paragraph shares tricks to simplify multiplication problems, such as the outcomes of multiplying by zero or one, and the use of multiplication tables. It emphasizes that multiplying by zero always results in zero, while multiplying by one leaves the number unchanged. The paragraph also highlights the utility of multiplication tables in quickly solving common multiplication problems, making the learning process more accessible and efficient.
🏎️ Perimeter as a Race Track: Measuring the Distance Around Shapes
This paragraph introduces the concept of perimeter through the analogy of a race car track, explaining that the perimeter is the distance around a shape. It provides examples of calculating the perimeter for different shapes, such as a triangle, a square, and a rectangle, by adding the lengths of their sides. The summary illustrates the process of finding the perimeter and emphasizes its importance in understanding the dimensions of two-dimensional spaces.
🎲 3D Shapes: Exploring the立体 World Beyond Flatland
The paragraph transitions from 2D to 3D shapes, starting with a review of familiar 2D shapes like the circle, oval, triangle, and square, and then introducing the concept of three-dimensional shapes. It explains that 3D shapes are 'fat not flat' and provides the sphere as the first example of a 3D shape, comparing it to its 2D counterpart, the circle. The summary sets the stage for a deeper exploration of 3D shapes and their characteristics, highlighting the transition from flat shapes to those with depth.
🏺 A Tour of 3D Shapes: From Spheres to Rectangular Prisms
This paragraph takes the viewer on a journey through various three-dimensional shapes, starting with the sphere and moving on to the cone, pyramid, cylinder, cube, and rectangular prism. Each shape is described with its distinctive features, such as the sphere being perfectly round with no flat surfaces, the cone having a circular base and a single vertex, and the rectangular prism being the 3D version of a rectangle with six rectangular faces. The summary provides examples of where these shapes can be found in everyday life, emphasizing their prevalence and utility.
🎉 A Celebration of 3D Shapes: Recognition and Review
The paragraph concludes the lesson on 3D shapes with a celebration of the learner's achievements and a review of the shapes covered. It uses visual aids to help identify and remember the characteristics of each 3D shape, from the sphere to the rectangular prism. The summary highlights the importance of recognizing and differentiating between these shapes, encouraging continued learning and exploration of the three-dimensional world.
Mindmap
Keywords
💡Fractions
💡Numerator
💡Denominator
💡Roman Numerals
💡Division
💡Even Numbers
💡Odd Numbers
💡Multiplication
💡Perimeter
💡3D Shapes
💡Cube
Highlights
Introduction to fractions as parts of a whole using the relatable example of sharing a pizza.
Explanation of fraction components: the numerator as the number of parts taken and the denominator as the total parts.
Engaging poll results showing the community's interest in learning about fractions.
Illustration of fractions using the scenario of dividing a pizza among friends.
Teaching Roman numerals as an ancient and intriguing way of writing numbers.
Simple mnemonics to remember the values of Roman numerals I, V, and X.
Creative approach to learning division through the story of children sharing carrots.
Clarification that division is the process of splitting a number into equal parts.
Emphasizing the impossibility of dividing by zero and the concept of undefined results.
Introduction of even and odd numbers with a fun and interactive exploration using dinosaurs.
Understanding even numbers as those that can be grouped into twos and odd as those that cannot.
Multiplication presented as a detective game to solve the number of items in groups.
The concept of multiplication tables as a learning tool for quick problem-solving.
Introduction to perimeter as the distance around a shape and its calculation.
Practical examples of finding the perimeter of various shapes like triangles, squares, and rectangles.
Introduction and exploration of 3D shapes starting with the sphere and its characteristics.
Learning about cones, pyramids, cylinders, cubes, and rectangular prisms as 3D counterparts to 2D shapes.
Interactive quiz to reinforce the understanding of 2D and 3D shapes.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: