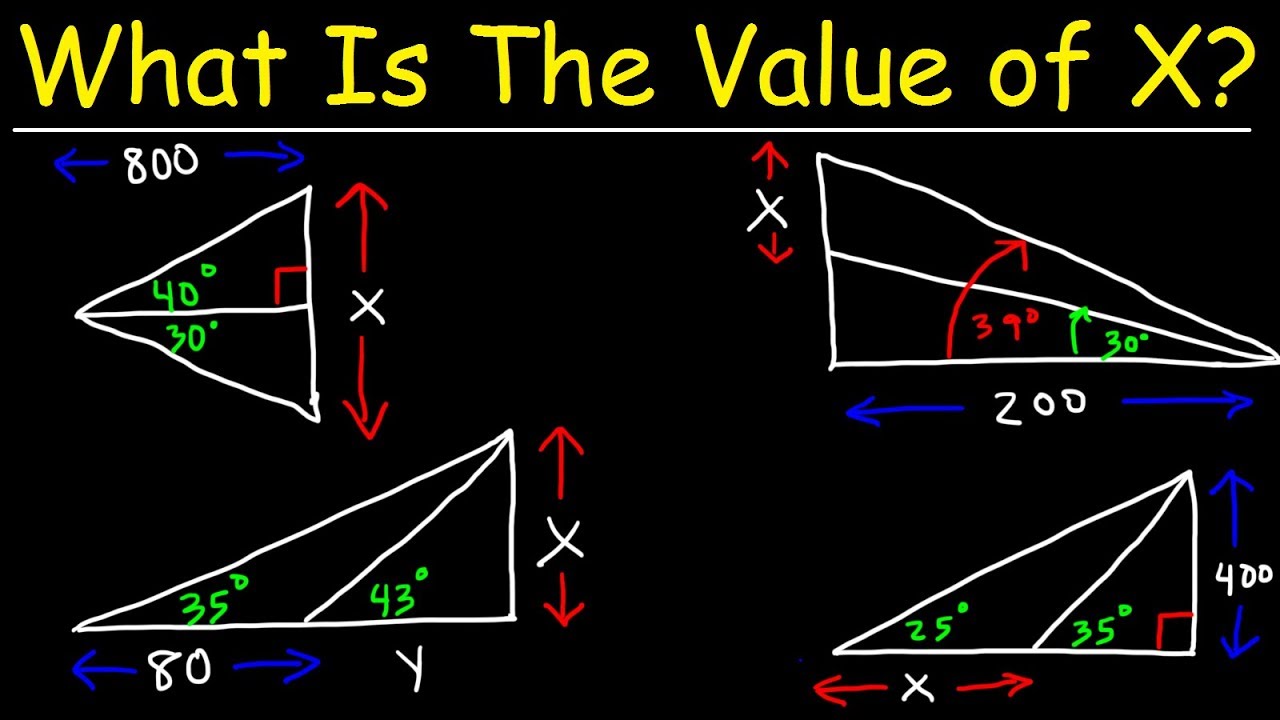
Math 8 4 2 Homework Help Morgan

In this segment, the focus is on understanding how to keep a balance hanger level by figuring out the unknown weights using the concept of balanced equations. The video introduces the idea with a practical example of socks on a hanger, explaining how additional weight from one sock causes imbalance. It then moves on to the 'hanging blocks' activity, where different shapes like squares and triangles are used to demonstrate balance and the effects of removing an unequal number of blocks from each side, leading to an imbalance. The segment concludes with a mathematical approach to determine the weight of a square when given the weight of a triangle, using algebraic equations to solve for the unknown.

This paragraph delves deeper into the concept of balance with hangers, introducing different shapes and their weights. It presents a scenario with triangles, circles, and squares, and uses these to create equations that represent the balance of the hangers. The video explains how to set up equations based on the given weights and solve for the unknown weights, such as the weight of a square in relation to triangles and circles. It also discusses a situation where the weight of a pentagon cannot be determined due to the equations being identical after simplification, highlighting the importance of having distinct equations to solve for unknowns.

The script presents a more complex scenario involving a hanger with multiple shapes and their respective weights. It challenges the viewer to determine if certain changes would keep the hanger balanced, using the concept of equal weights on both sides of the hanger. The video provides a step-by-step analysis of each change, explaining why some would maintain balance while others would not. It also touches on the idea of canceling out balanced weights to simplify the problem and solve for the weight of a square, emphasizing the importance of careful consideration in maintaining balance.

This part of the script introduces a homework assignment that involves determining which changes to a hanger would keep it balanced, given the weights of different shapes. It provides a detailed analysis of each possible change, explaining the reasoning behind whether the hanger would remain balanced or not. The video also includes a puzzle involving the ages of a family, where the sum of their ages equals 87, and challenges the viewer to determine each family member's age using the given relationships and a system of equations.

The script presents a family age puzzle where the relationships between family members' ages are given, and the sum of their ages is 87. The video guides the viewer through setting up a system of equations based on the relationships, such as Andre being three years younger than his brother and two years older than his sister. It then solves for Andre's age and subsequently finds the ages of his brother, sister, and mother, ensuring that the sum of the ages matches the given total of 87.

In this segment, the focus shifts to writing equations for two parallel lines, using the concept of slope. The video explains how to determine the slope from given points on the lines and emphasizes that parallel lines have the same slope. It demonstrates two methods for writing the equations: using point-slope form and y-intercept form. The video also shows how to simplify the equations and discusses the importance of knowing the slope and a point on the line to write its equation.

The final paragraph wraps up the lesson on writing equations for parallel lines. It summarizes the process of finding the slope from two points and using it to write the equations in different forms. The video also provides examples of how to simplify the equations and reiterates the key concept that parallel lines share the same slope, which is essential for writing their equations correctly.