Calculus Nth Term Test

Chad Gilliland
26 Jan 201210:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the instructor delves into the concepts of sequences and series, focusing on their convergence or divergence. They define a sequence as an ordered list and a series as the summation of a sequence. The video emphasizes the importance of the limit for determining convergence, introducing the nth term test for divergence. The instructor provides several examples to illustrate these concepts, including series with factorials, and cautions that the nth term test cannot confirm convergence, only divergence. The session encourages students to explore series further with calculators and anticipates a follow-up video for deeper exploration.

Takeaways
  • 📚 The lecture begins with an introduction to Chapter 9, focusing on sequences and series, specifically sections 9.1 and 9.2.
  • 🔍 A sequence is defined as an ordered list of terms, often denoted as \( a_n \), where 'n' represents the position in the sequence.
  • 🌟 The convergence of a sequence is determined by whether it has a limit, \( L \), as \( n \) approaches infinity.
  • 💥 A series is the summation of a sequence, and it diverges if the sum approaches infinity; otherwise, it converges if the sum remains finite.
  • 🤔 The chapter's main questions are to determine if a series converges or diverges, and if it converges, to what value.
  • 🌌 An example given is the series \( \sum_{1}^{\infty} 2n \), which clearly diverges as it approaches infinity.
  • 📉 Another example is the series \( \sum_{1}^{\infty} \frac{1}{n(n+1)} \), which converges as the sequence approaches a limit of 1/2.
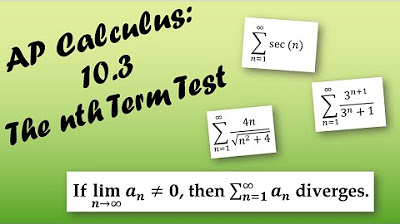
  • 🚫 The nth term test for divergence states that if the limit of \( a_n \) as \( n \) approaches infinity does not equal zero, the series diverges.
  • ⚠️ The nth term test cannot be used to prove convergence; it only indicates divergence if the limit is non-zero.
  • 📝 The instructor encourages students to use calculators to explore series and observe the behavior of partial sums.
  • 📅 A follow-up video is planned for the weekend, with a continuation of the topic on Monday.
Q & A

  • What is the main topic discussed in Chapter 9 of the book?

    -The main topic discussed in Chapter 9 is sequences and series, specifically focusing on the term test.

  • How does the instructor define a sequence?

    -A sequence is defined as a list of things where order matters, usually denoted as a_sub_N, where N represents the position in the sequence.

  • What is the condition for a sequence to converge?

    -A sequence converges if it has a limit as N approaches infinity, meaning a_sub_N settles down to a limit L.

  • How is a series defined in the context of sequences?

    -A series is defined as a summation of a sequence, starting at a specific point, usually at one, and continues adding the terms of the sequence.

  • What does it mean for a series to diverge?

    -A series diverges if the summation of its terms approaches infinity or does not stay finite.

  • What are the two main questions addressed in the chapter about series?

    -The two main questions are: 1) Does a series converge or diverge? 2) If it converges, to what value does it converge?

  • What is the 'nth term test for divergence'?

    -The nth term test for divergence states that if the limit of the sequence (a_sub_N) as N approaches infinity does not equal zero, then the series diverges.

  • Can the nth term test be used to prove convergence?

    -No, the nth term test can only be used to prove divergence, not convergence.

  • What is an example of a series that diverges?

    -An example of a diverging series is the summation from 1 to infinity of 2, which results in the sequence 2 + 4 + 6 + 8 + ... and diverges to infinity.

  • What happens to a series where the limit of the sequence as N approaches infinity is zero?

    -If the limit of the sequence as N approaches infinity is zero, the nth term test says nothing about whether the series converges; other tests are needed to determine convergence.

Outlines
00:00
📚 Introduction to Sequences and Series

The video begins with an introduction to Chapter 9, focusing on sequences and series. A sequence is defined as an ordered list of terms, often denoted as \( a_n \), and is said to converge if it has a limit as \( n \) approaches infinity. Conversely, a sequence diverges if it does not have a limit or if the limit is infinity. A series is the summation of a sequence, and it converges if the sum remains finite as \( n \) approaches infinity, otherwise, it diverges. The chapter will address two main questions: whether a series converges or diverges and, if so, to what value. The instructor provides an example of a series that clearly diverges, the sum of even numbers from 2 to infinity.

05:05
🔍 The nth Term Test for Divergence

This paragraph delves into the nth term test for divergence, a method to determine if a series diverges by examining the limit of its sequence as \( n \) approaches infinity. If the limit is not zero, the series is guaranteed to diverge. The instructor illustrates this with examples, including a series where the terms are \( \frac{1}{n} \), which converges because the limit of the sequence is zero, and another where the terms are \( \frac{n}{3^n} \), which diverges because the limit is not zero. The instructor also emphasizes that the nth term test cannot be used to prove convergence, only divergence.

10:06
⚠️ Caution on Using the nth Term Test

The final paragraph serves as a cautionary note about the limitations of the nth term test. It is a test strictly for divergence and should not be misconstrued as evidence for convergence. The instructor encourages students to experiment with series using calculators to observe the behavior of partial sums and to look forward to another video that will be released over the weekend. The session concludes with a reminder of the upcoming Monday's class.

Mindmap
Keywords
💡Sequence
A sequence is an ordered list of numbers or terms, where the position of each term is important and is usually denoted by a subscript, such as (a_n). In the video, sequences are discussed in the context of their convergence or divergence, which is determined by whether the terms of the sequence approach a limit as (n) approaches infinity. The script uses sequences to introduce the concept of series and their convergence properties.
💡Convergence
Convergence in the context of sequences and series refers to the property of approaching a certain value or behavior as the number of terms increases indefinitely. A sequence converges if it has a limit as (n) approaches infinity. In the video, the concept of convergence is used to determine whether a series will have a finite sum or not, with examples provided to illustrate sequences that converge to a limit.
💡Divergence
Divergence is the opposite of convergence and describes a situation where a sequence or series does not approach a finite limit as (n) approaches infinity. In the script, divergence is discussed in relation to sequences and series, with examples given to show series that continue to increase without bound, such as the series of 2 plus 4 plus 6 and so on.
💡Limit
The limit is a fundamental concept in calculus that describes the value that a function or sequence approaches as the input approaches some point. In the video, the limit is used to determine whether a sequence converges to a specific value or diverges to infinity. The script explains that if the limit of (a_n) as (n) approaches infinity exists and is finite, the sequence converges; otherwise, it diverges.
💡Series
A series is the summation of the terms of a sequence. It is represented by a sigma notation, which sums up the terms from a starting point to infinity. In the video, series are discussed in terms of their convergence or divergence, with the script providing examples of series that either have a finite sum or increase without bound.
💡Partial Sum
A partial sum is the sum of the first (n) terms of a series. It is used to analyze the behavior of a series and to determine its convergence. In the script, partial sums are calculated for various series to observe whether they approach a finite value or increase indefinitely, which helps in determining the convergence of the series.
💡nth Term Test for Divergence
The nth term test for divergence is a test used to determine whether a series diverges. It states that if the limit of the (n)th term of a series, (a_n), as (n) approaches infinity, is not zero, then the series diverges. The script explains this test and uses it to analyze several series, emphasizing that it cannot be used to prove convergence, only divergence.
💡Factorial
Factorial, denoted by an exclamation mark (e.g., (n!)), is the product of all positive integers up to a given number (n). In the video, factorials are used in the context of sequences and series, such as in the example where (n!) is divided by (3^n) to form a series that is analyzed for convergence.
💡Homework Assignment
A homework assignment is a task given to students to be completed outside of class, typically to reinforce or apply concepts learned during the lesson. In the script, the instructor mentions a homework assignment related to sequences and series that students are expected to complete and submit, indicating the practical application of the concepts discussed in the video.
💡Calculator
A calculator is a device or software used to perform mathematical calculations. In the video, the instructor encourages students to use a calculator to plug in numbers and observe the behavior of different series, which helps in understanding the convergence or divergence of series in a practical manner.
Highlights

Introduction to Chapter 9 focusing on sequences and series.

Definition of a sequence as an ordered list where order matters, denoted by sub N.

Explanation of sequence convergence based on having a limit as n approaches infinity.

Sequence divergence occurs if it does not have a limit or if the limit is infinity.

Definition of a series as the summation of a sequence, often starting at one.

Series convergence is determined by the summation staying finite as n approaches infinity.

The two main questions of the chapter: Does the series converge, and if so, to what value?

Example of a divergent series: the sum of 2 plus 4 plus 6 to infinity.

Illustration of the nth term test for divergence using the series 1/n.

The nth term test indicates divergence if the limit as n approaches infinity does not equal zero.

The nth term test cannot be used to argue for convergence, only divergence.

Example of a series that does not converge using the nth term test: 1/(3n).

Example of a series that diverges using the nth term test: n/(3n).

Discussion on factorials and their behavior in series convergence tests.

Final example involving factorials and the nth term test for divergence.

Encouragement to use calculators to explore series behavior and partial sums.

Announcement of a follow-up video to further discuss series.

Transcripts

Browse More Related Video

Infinite Series Multiple Choice Practice for Calc BC (Part 6)

Calculus BC – 10.3 The nth Term Test for Divergence

Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences

Geometric Series BC Calculus 1-31

AP Calculus BC Lesson 10.3

Calculus Chapter 5 Lecture 52 Convergence Tests 2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsSequencesSeriesConvergenceDivergenceLimit TestEducationalCalculusHomeworkTeachingTutorial