Power series of arctan(2x) | Series | AP Calculus BC | Khan Academy

Khan Academy
10 Oct 201411:05
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the presenter guides viewers through the process of finding the power series approximation of arctangent(2x) centered at zero, focusing on the first four nonzero terms. The key insight involves simplifying the problem by considering the derivative of arctangent(2x) and relating it to a more manageable function g(x), whose derivatives are easier to compute. By deriving the power series of g(x) and substituting appropriately, the presenter elegantly demonstrates how to obtain the desired terms for arctangent(2x), showcasing a clever approach to tackling complex calculus problems.

Takeaways
  • 📚 The video aims to find the power series representation of the arctangent of two x, specifically the first four nonzero terms of its Maclaurin series.
  • 🔍 The script suggests taking the derivative of arctangent of two x to start finding the series, which simplifies to two over one plus four x squared.
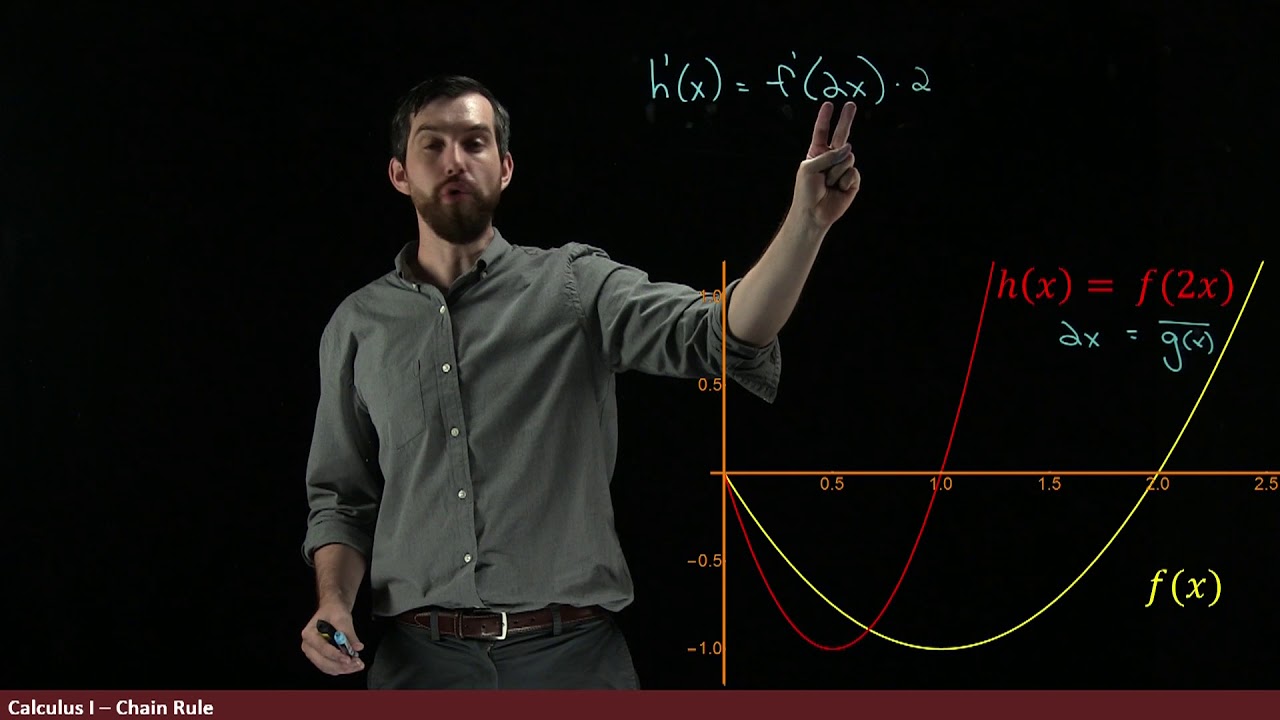
  • 💡 An insight is introduced to simplify the process by considering the derivative as a function f(x) and looking for a related function g(x) with easier derivatives.
  • 🔑 The function g(x) is defined as one over one plus x, which has a straightforward derivative that simplifies the process of finding the power series.
  • 📈 The derivatives of g(x) are calculated, showing a pattern that makes it easy to find the power series representation for g(x) by evaluating at zero.
  • 📝 The script demonstrates that f(x) can be expressed as two times g(four x squared), which allows leveraging the simpler power series of g(x) to find that of f(x).
  • 🔄 A substitution is made in the power series of g(x) to find the first four terms of f(x) by replacing x with four x squared and multiplying by two.
  • 📉 The resulting power series for f(x) is simplified to find the first four nonzero terms, which are then used to approximate the derivative of arctangent of two x.
  • ✏️ The antiderivative of the found power series for f(x) is taken to approximate the arctangent of two x, ensuring the constant term is adjusted to fit the Maclaurin series centered at zero.
  • 🎯 The final approximation for arctangent of two x is given as two x minus 8/3 x cubed plus 32/5 x to the fifth minus 128/7 x to the seventh, with the constant term set to zero.
  • 🚀 The video concludes by highlighting that the complex problem was made simpler through strategic function substitution and series manipulation.
Q & A

  • What is the main goal of the video?

    -The main goal of the video is to find the power series approximation, specifically the first four nonzero terms, of the arctangent of two x centered at zero.

  • What is the Maclaurin Series and why is it relevant to the video?

    -The Maclaurin Series is a power series representation of a function centered at zero. It is relevant because the video aims to find the Maclaurin Series of the arctangent of two x.

  • Why is the derivative of arctangent of two x with respect to x equal to two over one plus four x squared?

    -The derivative of arctangent of x is one over one plus x squared. For arctangent of two x, the derivative is the derivative of the original function multiplied by two (the coefficient of x), leading to two over one plus four x squared.

  • What is the key insight provided in the video to simplify finding the power series of arctangent of two x?

    -The key insight is to find the power series representation of the derivative of arctangent of two x and then integrate it to get the power series of arctangent of two x, ensuring the constant term is adjusted for the series to be centered at zero.

  • What function g(x) is introduced in the video to simplify the process of finding the power series?

    -The function g(x) is introduced as one over one plus x, which simplifies to one plus x to the negative one power. This function is chosen because its derivatives are easy to compute.

  • How does the video simplify the computation of derivatives for the function g(x)?

    -The video shows that the derivatives of g(x) can be computed easily by applying the chain rule and adjusting the powers of one plus x accordingly, resulting in a series of terms that are simple to evaluate at zero.

  • What is the significance of evaluating the derivatives of g(x) at zero?

    -Evaluating the derivatives at zero simplifies the process of finding the coefficients for the power series representation of g(x), which is then used to find the power series for the derivative of arctangent of two x.

  • How does the video relate the function g(x) to the derivative of arctangent of two x?

    -The video shows that the derivative of arctangent of two x can be expressed as two times g(four x squared), allowing the power series of g(x) to be used to find the power series of the derivative.

  • What substitution is made in the power series of g(x) to find the power series of the derivative of arctangent of two x?

    -The substitution made is replacing x with four x squared in the power series of g(x) and then multiplying the entire series by two.

  • How is the final power series for arctangent of two x obtained in the video?

    -The final power series for arctangent of two x is obtained by integrating the power series of its derivative, adjusting the constant term to ensure the series is centered at zero, and ensuring that the arctangent of zero equals zero.

  • What are the first four nonzero terms of the power series for arctangent of two x as presented in the video?

    -The first four nonzero terms are 2x, -8/3 x cubed, 32/5 x to the fifth, and -128/7 x to the seventh.

Outlines
00:00
📚 Introduction to Power Series of Arctangent(2x)

The video begins with the host's intention to derive the power series representation of arctangent(2x), specifically the first four nonzero terms centered at zero. This is essentially the Maclaurin series for the function. The host encourages viewers to attempt the problem before revealing the solution. The approach involves understanding the derivative of arctangent(2x), which is simplified by recognizing it as 2/(1+4x^2), and then considering the complexity of deriving the series directly from this expression.

05:03
🔍 Insight on Simplifying the Derivative's Power Series

The host introduces a clever insight to simplify the process of finding the power series. Instead of directly deriving the series from the complex derivative, they propose considering a related function g(x) = 1/(1+x), which has a simpler derivative structure. By examining the derivatives of g(x), a pattern emerges that allows for easy calculation of its power series. The host then demonstrates how to find the first few terms of g(x)'s power series by evaluating its derivatives at zero.

10:06
🎯 Leveraging the Relationship Between f(x) and g(x) for Power Series

Building on the previous insight, the host reveals that f(x), the derivative of arctangent(2x), can be expressed as 2*g(4x^2). This relationship is key to simplifying the power series derivation. By substituting x with 4x^2 in the power series of g(x) and multiplying by two, the host derives the power series for f(x). This method avoids the complexity of directly deriving the series from the original function and results in a straightforward calculation of the first four nonzero terms.

📝 Constructing the Power Series for Arctangent(2x) Through Antiderivatives

With the power series for f(x) established, the host proceeds to find the power series for arctangent(2x) by integrating f(x). They carefully integrate each term of the power series for f(x), ensuring to adjust for the constant of integration, which must be zero to satisfy the condition that arctangent(2x) equals zero when x equals zero. The final step confirms the correctness of the constant term, completing the derivation of the power series for arctangent(2x) with the first four nonzero terms.

Mindmap
Keywords
💡Power Series
A power series is an infinite series of the form \( \sum_{n=0}^{\infty} a_n (x - x_0)^n \), where \( a_n \) are the coefficients and \( x_0 \) is the center of the series. In the context of the video, the power series is used to approximate functions, specifically the arctangent of two x centered at zero, which is the main theme of the video.
💡Maclaurin Series
A Maclaurin series is a special case of a power series where the center \( x_0 \) is zero. It is used to represent functions as an infinite sum of terms, which are calculated from the derivatives of the function at zero. The video is focused on finding the first four nonzero terms of the Maclaurin series for the arctangent of two x.
💡Arctan(x)
The arctangent function, often written as \( \text{arctan}(x) \) or \( \tan^{-1}(x) \), is the inverse function of the tangent. It represents the angle whose tangent is x. In the video, the script discusses finding the power series approximation of \( \text{arctan}(2x) \), which is a variation of the standard arctangent function.
💡Derivative
The derivative of a function measures how the function changes as its input changes. In the video, the derivative of \( \text{arctan}(2x) \) is calculated as \( \frac{2}{1 + 4x^2} \), which is a crucial step in finding the power series representation of the function.
💡Antiderivative
An antiderivative, also known as an indefinite integral, is a function that represents the reverse process of differentiation. The video involves taking the antiderivative of the power series representation of the derivative of \( \text{arctan}(2x) \) to find the power series of \( \text{arctan}(2x) \) itself.
💡Nonzero Terms
In the context of a power series, nonzero terms are those that contribute to the sum and do not cancel out. The video aims to find the first four nonzero terms of the power series for \( \text{arctan}(2x) \), which are essential for the approximation.
💡Chain Rule
The chain rule is a fundamental principle in calculus for differentiating composite functions. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. The video script mentions the chain rule when discussing the derivatives of the function \( g(x) \).
💡Factorial
The factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n. In the video, factorials are used in the denominators of terms when writing out the power series for \( g(x) \) and its derivatives.
💡Centered at Zero
When a power series is said to be 'centered at zero,' it means that the series is expanded around the point \( x_0 = 0 \). This is important for the Maclaurin series, which is the focus of the video, as it ensures that the first term of the series is the value of the function at zero.
💡Constant of Integration
The constant of integration arises when finding antiderivatives and represents an arbitrary constant added to the indefinite integral. In the video, the constant is adjusted to ensure that the power series approximation of \( \text{arctan}(2x) \) is correct when evaluated at zero.
Highlights

Introduction of the task to find the power series representation of arctangent(2x) centered at zero.

Encouragement for viewers to pause the video and attempt the problem themselves.

Derivation of the derivative of arctangent(2x) as 2/(1+4x^2).

Explanation of the complexity in finding the Maclaurin Series by direct differentiation.

Introduction of the key insight to simplify the problem by considering the antiderivative of the derivative.

Introduction of function g(x) = 1/(1+x) to simplify the differentiation process.

Derivation of g(x)'s derivatives to show the ease of finding the power series representation.

Calculation of the first three derivatives of g(x) and their evaluation at zero.

Formation of the power series for g(x) using the evaluated derivatives.

Revelation that f(x) can be expressed as 2 * g(4x^2) to connect the simplified problem with the original.

Substitution of x in the power series of g(x) with 4x^2 to find f(x)'s power series.

Derivation of the first four nonzero terms of the power series for f(x).

Explanation of the process to find the power series for arctangent(2x) by integrating f(x).

Integration of the power series terms to approximate arctangent(2x).

Adjustment of the constant term to ensure the series representation is accurate at x=0.

Final expression of the approximated power series for arctangent(2x).

Reflection on the problem-solving process and the simplification achieved through key insights.

Transcripts

Browse More Related Video

Worked example: power series from cos(x) | Series | AP Calculus BC | Khan Academy

First principles grade 12: Practice

Chain Rule Practice Problems

Finding function from power series by integrating | Series | AP Calculus BC | Khan Academy

Interpreting the Chain Rule Graphically

2023 AP Calculus BC FRQ #6

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Power SeriesArctangentDerivativeMaclaurin SeriesMathematicsCalculusSeries ApproximationIntegralEducationalProblem Solving